5.7: Hydrolysis of Salt Solutions
- Page ID
- 364675
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Predict whether a salt solution will be acidic, basic, or neutral
- Calculate the concentrations of the various species in a salt solution
- Describe the process that causes solutions of certain metal ions to be acidic
As we have seen in the section on chemical reactions, when an acid and base are mixed, they undergo a neutralization reaction. The word “neutralization” seems to imply that a stoichiometrically equivalent solution of an acid and a base would be neutral. This is sometimes true, but the salts that are formed in these reactions may have acidic or basic properties of their own, as we shall now see.
Acid-Base Neutralization
A solution is neutral when it contains equal concentrations of hydronium and hydroxide ions. When we mix solutions of an acid and a base, an acid-base neutralization reaction occurs. However, even if we mix stoichiometrically equivalent quantities, we may find that the resulting solution is not neutral. It could contain either an excess of hydronium ions or an excess of hydroxide ions because the nature of the salt formed determines whether the solution is acidic, neutral, or basic. The following four situations illustrate how solutions with various pH values can arise following a neutralization reaction using stoichiometrically equivalent quantities:
- A strong acid and a strong base, such as HCl(aq) and NaOH(aq) will react to form a neutral solution since the conjugate partners produced are of negligible strength: \[\ce{HCl}(aq)+\ce{NaOH}(aq)⇌\ce{NaCl}(aq)+\ce{H2O}(l)\]
- A strong acid and a weak base yield a weakly acidic solution, not because of the strong acid involved, but because of the conjugate acid of the weak base.
- A weak acid and a strong base yield a weakly basic solution. A solution of a weak acid reacts with a solution of a strong base to form the conjugate base of the weak acid and the conjugate acid of the strong base. The conjugate acid of the strong base is a weaker acid than water and has no effect on the acidity of the resulting solution. However, the conjugate base of the weak acid is a weak base and ionizes slightly in water. This increases the amount of hydroxide ion in the solution produced in the reaction and renders it slightly basic.
- A weak acid plus a weak base can yield either an acidic, basic, or neutral solution. This is the most complex of the four types of reactions. When the conjugate acid and the conjugate base are of unequal strengths, the solution can be either acidic or basic, depending on the relative strengths of the two conjugates. Occasionally the weak acid and the weak base will have the same strength, so their respective conjugate base and acid will have the same strength, and the solution will be neutral. To predict whether a particular combination will be acidic, basic or neutral, tabulated K values of the conjugates must be compared.
Salts of Weak Bases and Strong Acids
When we neutralize a weak base with a strong acid, the product is a salt containing the conjugate acid of the weak base. This conjugate acid is a weak acid. For example, ammonium chloride, NH4Cl, is a salt formed by the reaction of the weak base ammonia with the strong acid HCl:
\[\ce{NH3}(aq)+\ce{HCl}(aq)⟶\ce{NH4Cl}(aq)\]
A solution of this salt contains ammonium ions and chloride ions. The chloride ion has no effect on the acidity of the solution since HCl is a strong acid. Chloride is a very weak base and will not accept a proton to a measurable extent. However, the ammonium ion, the conjugate acid of ammonia, reacts with water and increases the hydronium ion concentration:
\[\ce{NH4+}(aq)+\ce{H2O}(l)⇌\ce{H3O+}(aq)+\ce{NH3}(aq)\]
The equilibrium equation for this reaction is simply the ionization constant. Ka, for the acid \(\ce{NH4+}\):
\[\ce{\dfrac{[H3O+][NH3]}{[NH4+]}}=K_\ce{a}\]
We will not find a value of Ka for the ammonium ion in Table E1. However, it is not difficult to determine Ka for \(\ce{NH4+}\) from the value of the ionization constant of water, Kw, and Kb, the ionization constant of its conjugate base, NH3, using the following relationship:
\[K_\ce{w}=K_\ce{a}×K_\ce{b}\]
This relation holds for any base and its conjugate acid or for any acid and its conjugate base.
Aniline is an amine that is used to manufacture dyes. It is isolated as aniline hydrochloride, \(\ce{[C6H5NH3+]Cl}\), a salt prepared by the reaction of the weak base aniline and hydrochloric acid. What is the pH of a 0.233 M solution of aniline hydrochloride?
\[\ce{C6H5NH3+}(aq)+\ce{H2O}(l)⇌\ce{H3O+}(aq)+\ce{C6H5NH2}(aq)\]
Solution
The new step in this example is to determine Ka for the \(\ce{C6H5NH3+}\) ion. The \(\ce{C6H5NH3+}\) ion is the conjugate acid of a weak base. The value of Ka for this acid is not listed in Table E1, but we can determine it from the value of Kb for aniline, C6H5NH2, which is given as 4.6 × 10−10 :
\[\mathrm{\mathit{K}_a(for\:C_6H_5NH_3^+)×\mathit{K}_b(for\:C_6H_5NH_2)=\mathit{K}_w=1.0×10^{−14}}\]
\[\mathrm{\mathit{K}_a(for\:C_6H_5NH_3^+)=\dfrac{\mathit{K}_w}{\mathit{K}_b(for\:C_6H_5NH_2)}=\dfrac{1.0×10^{−14}}{4.6×10^{−10}}=2.3×10^{−5}}\]
Now we have the ionization constant and the initial concentration of the weak acid, the information necessary to determine the equilibrium concentration of H3O+, and the pH:
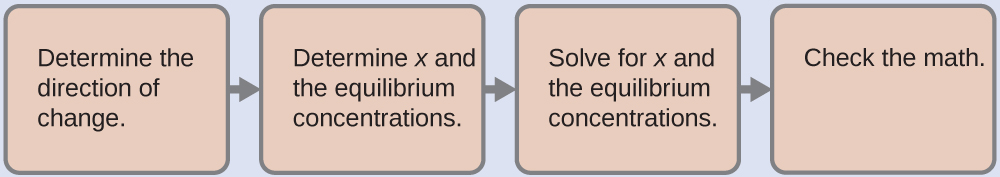
With these steps we find [H3O+] = 2.3 × 10−3 M and pH = 2.64
What is the hydronium ion concentration in a 0.100-M solution of ammonium nitrate, NH4NO3, a salt composed of the ions \(\ce{NH4+}\) and \(\ce{NO3-}\). The Kb for the ammonia is \(1.8×10^{−5}\).
- Answer
-
\(K_a\ce{(for\:NH4+)}=5.6×10^{−10}\), [H3O+] = \(7.5×10^{−6}\) M
Salts of Weak Acids and Strong Bases
When we neutralize a weak acid with a strong base, we get a salt that contains the conjugate base of the weak acid. This conjugate base is usually a weak base. For example, sodium acetate, NaCH3CO2, is a salt formed by the reaction of the weak acid acetic acid with the strong base sodium hydroxide:
\[\ce{CH3CO2H}(aq)+\ce{NaOH}(aq)⟶\ce{NaCH3CO2}(aq)+\ce{H2O}(aq)\]
A solution of this salt contains sodium ions and acetate ions. The sodium ion has no effect on the acidity of the solution. However, the acetate ion, the conjugate base of acetic acid, reacts with water and increases the concentration of hydroxide ion:
\[\ce{CH3CO2-}(aq)+\ce{H2O}(l)⇌\ce{CH3CO2H}(aq)+\ce{OH-}(aq)\]
The equilibrium equation for this reaction is the ionization constant, Kb, for the base \(\ce{CH3CO2-}\). The value of Kb can be calculated from the value of the ionization constant of water, Kw, and Ka, the ionization constant of the conjugate acid of the anion using the equation:
\[_\ce{w}=K_\ce{a}×K_\ce{b}\]
For the acetate ion and its conjugate acid we have:
\[\mathrm{\mathit{K}_b(for\:\ce{CH_3CO_2^-})=\dfrac{\mathit{K}_w}{\mathit{K}_a(for\:CH_3CO_2H)}=\dfrac{1.0×10^{−14}}{1.8×10^{−5}}=5.6×10^{−10}}\]
Some handbooks do not report values of Kb. They only report ionization constants for acids. If we want to determine a Kb value using one of these handbooks, we must look up the value of Ka for the conjugate acid and convert it to a Kb value.
Determine the acetic acid concentration in a solution with \(\ce{[CH3CO2- ]}=0.050\:M\) and [OH−] = 2.5 × 10−6 M at equilibrium. The reaction is:
\[\ce{CH3CO2-}(aq)+\ce{H2O}(l)⇌\ce{CH3CO2H}(aq)+\ce{OH-}(aq)\]
Solution
We are given two of three equilibrium concentrations and asked to find the missing concentration. If we can find the equilibrium constant for the reaction, the process is straightforward.
The acetate ion behaves as a base in this reaction; hydroxide ions are a product. We determine Kb as follows:
\[\mathrm{\mathit{K}_b(for\:\ce{CH_3CO_2^-})=\dfrac{\mathit{K}_w}{\mathit{K}_a(for\:CH_3CO_2H)}=\dfrac{1.0×10^{−14}}{1.8×10^{−5}}=5.6×10^{−10}}\]
Now find the missing concentration:
\[K_\ce{b}=\ce{\dfrac{[CH3CO2H][OH- ]}{[CH3CO2- ]}}=5.6×10^{−10}\]
\[=\dfrac{[\ce{CH3CO2H}](2.5×10^{−6})}{(0.050)}=5.6×10^{−10}\]
Solving this equation we get [CH3CO2H] = 1.1 × 10−5 M.
What is the pH of a 0.083-M solution of CN−? Use 4.9 × 10−10 as Ka for HCN. Hint: We will probably need to convert pOH to pH or find [H3O+] using [OH−] in the final stages of this problem.
- Answer
-
11.16
Equilibrium in a Solution of a Salt of a Weak Acid and a Weak Base
In a solution of a salt formed by the reaction of a weak acid and a weak base, to predict the pH, we must know both the Ka of the weak acid and the Kb of the weak base. If Ka > Kb, the solution is acidic, and if Kb > Ka, the solution is basic.
Determine whether aqueous solutions of the following salts are acidic, basic, or neutral:
- KBr
- NaHCO3
- NH4Cl
- Na2HPO4
- NH4F
Solution
Consider each of the ions separately in terms of its effect on the pH of the solution, as shown here:
- The K+ cation and the Br− anion are both spectators, since they are the cation of a strong base (KOH) and the anion of a strong acid (HBr), respectively. The solution is neutral.
- The Na+ cation is a spectator, and will not affect the pH of the solution; the \(\ce{HCO3-}\) anion is amphiprotic.The Ka of \(\ce{HCO3-}\) is 4.7 × 10−11, so the Kb of its conjugate base is \(\dfrac{1.0×10^{−14}}{4.7×10^{−11}}=2.1×10^{−4}\). Since Kb >> Ka, the solution is basic.
- The \(\ce{NH4+}\) ion is acidic and the Cl− ion is a spectator. The solution will be acidic.
- The Na+ ion is a spectator and will not affect the pH of the solution, while the \(\ce{HPO4^{2-}}\) ion is amphiprotic. The Ka of \(\ce{HPO_4^{2-}}\) is 4.2 × 10−13 and its Kb is \(\dfrac{1.0×10^{−14}}{4.2×10^{−13}}=2.4×10^{−2}\). Because Kb >> Ka, the solution is basic.
- The \(\ce{NH4+}\) ion is listed as being acidic, and the F− ion is listed as a base, so we must directly compare the Ka and the Kb of the two ions. Ka of \(\ce{NH4+}\) is 5.6 × 10−10, which seems very small, yet the Kb of F− is 1.4 × 10−11, so the solution is acidic, since Ka > Kb.
Determine whether aqueous solutions of the following salts are acidic, basic, or neutral:
- K2CO3
- CaCl2
- KH2PO4
- (NH4)2CO3
- AlBr3
- Answer a
-
basic
- Answer b
-
neutral
- Answer c
-
acidic
- Answer d
-
basic
- Answer e
-
acidic
The Ionization of Hydrated Metal Ions
If we measure the pH of the solutions of a variety of metal ions we will find that these ions act as weak acids when in solution. The aluminum ion is an example. When aluminum nitrate dissolves in water, the aluminum ion reacts with water to give a hydrated aluminum ion, \(\ce{Al(H2O)6^3+}\), dissolved in bulk water. What this means is that the aluminum ion has the strongest interactions with the six closest water molecules (the so-called first solvation shell), even though it does interact with the other water molecules surrounding this \(\ce{Al(H2O)6^3+}\) cluster as well:
\[\ce{Al(NO3)3}(s)+\ce{6H2O}(l)⟶\ce{Al(H2O)6^3+}(aq)+\ce{3NO3-}(aq)\]
We frequently see the formula of this ion simply as “Al3+(aq)”, without explicitly noting the six water molecules that are the closest ones to the aluminum ion and just describing the ion as being solvated in water (hydrated). This is similar to the simplification of the formula of the hydronium ion, H3O+ to H+. However, in this case, the hydrated aluminum ion is a weak acid (Figure \(\PageIndex{1}\)) and donates a proton to a water molecule. Thus, the hydration becomes important and we may use formulas that show the extent of hydration:
\[\ce{Al(H2O)6^3+}(aq)+\ce{H2O}(l)⇌\ce{H3O+}(aq)+\ce{Al(H2O)5(OH)^2+}(aq) \hspace{20px} K_\ce{a}=1.4×10^{−5}\]
As with other polyprotic acids, the hydrated aluminum ion ionizes in stages, as shown by:
\[\ce{Al(H2O)6^3+}(aq)+\ce{H2O}(l)⇌\ce{H3O+}(aq)+\ce{Al(H2O)5(OH)^2+}(aq)\]
\[\ce{Al(H2O)5(OH)^2+}(aq)+\ce{H2O}(l)⇌\ce{H3O+}(aq)+\ce{Al(H2O)4(OH)2+}(aq)\]
\[\ce{Al(H2O)4(OH)2+}(aq)+\ce{H2O}(l)⇌\ce{H3O+}(aq)+\ce{Al(H2O)3(OH)3}(aq)\]
Note that some of these aluminum species are exhibiting amphiprotic behavior, since they are acting as acids when they appear on the left side of the equilibrium expressions and as bases when they appear on the right side.
![A reaction is shown using ball and stick models. On the left, inside brackets with a superscript of 3 plus outside to the right is structure labeled “[ A l ( H subscript 2 O ) subscript 6 ] superscript 3 plus.” Inside the brackets is s central grey atom to which 6 red atoms are bonded in an arrangement that distributes them evenly about the central grey atom. Each red atom has two smaller white atoms attached in a forked or bent arrangement. Outside the brackets to the right is a space-filling model that includes a red central sphere with two smaller white spheres attached in a bent arrangement. Beneath this structure is the label “H subscript 2 O.” A double sided arrow follows. Another set of brackets follows to the right of the arrows which have a superscript of two plus outside to the right. The structure inside the brackets is similar to that on the left, except a white atom is removed from the structure. The label below is also changed to “[ A l ( H subscript 2 O ) subscript 5 O H ] superscript 2 plus.” To the right of this structure and outside the brackets is a space filling model with a central red sphere to which 3 smaller white spheres are attached. This structure is labeled “H subscript 3 O superscript plus.”](https://chem.libretexts.org/@api/deki/files/65679/CNX_Chem_14_04_hydronium.jpg?revision=1&size=bestfit&width=594&height=202)
The constants for the different stages of ionization are not known for many metal ions, so we cannot calculate the extent of their ionization. However, practically all hydrated metal ions other than those of the alkali metals ionize to give acidic solutions. Ionization increases as the charge of the metal ion increases or as the size of the metal ion decreases.
Summary
The characteristic properties of aqueous solutions of Brønsted-Lowry acids are due to the presence of hydronium ions; those of aqueous solutions of Brønsted-Lowry bases are due to the presence of hydroxide ions. The neutralization that occurs when aqueous solutions of acids and bases are combined results from the reaction of the hydronium and hydroxide ions to form water. Some salts formed in neutralization reactions may make the product solutions slightly acidic or slightly basic. Solutions that contain salts or hydrated metal ions have a pH that is determined by the extent of the hydrolysis of the ions in the solution. The pH of the solutions may be calculated using familiar equilibrium techniques, or it may be qualitatively determined to be acidic, basic, or neutral depending on the relative Ka and Kb of the ions involved.


