Effective Nuclear Charge
- Page ID
- 54334
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Skills to Develop
- Explain the difference between nuclear charge and effective nuclear charge
The reason electrons are attached to atoms is the Coulomb's law attraction between the positively charged nucleus and the negatively charged electrons. Without the nuclear charge holding on to the electrons, they would have no reason to stay in orbitals near nuclei. So it makes sense that energy of the orbitals and their size depend on the nuclear charge. For instance, equivalent orbitals get lower in energy and smaller (more density closer to the nucleus) when nuclear charge increases. (If you want to see where nuclear charge is in the orbital equations, follow this link and click on an orbital; then scroll down to see the equation. Zeff is the effective nuclear charge.)
There are 2 reasons an electron might not spend that much time actually right next to the nucleus, even though there is an attraction. One is angular momentum. Remember from physics that angular momentum is basically the momentum in the "around the center" direction multiplied by r, the distance from the center. Thus, if the electron is "in" the nucleus, r = 0, and angular momentum is 0. Remember also the second quantum number is the angular momentum quantum number ℓ, which corresponds to s, p, d and f orbitals. For s orbitals, ℓ = 0, and angular momentum is zero, so the electron can hang out at the nucleus. For the other shapes (p,d,f) angular momentum is not zero, and there is a node at the nucleus. So only s orbitals can hang out right at the nucleus.
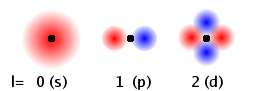
The other reason an electron might not be able to get that close to the nucleus is because of the other electrons. Electrons repel each other, and because of the exclusion principle there can only be 2 electrons in each orbital. So think about lithium, element 3. Its first 2 electrons are in 1s. The third electron is in 2s. Because the principal quantum number is bigger, the 2s electron is usually farther from the nucleus than the 1s electrons. That means that often the 1s electrons are between the 2s electron and the nucleus. This means that the total Coulomb attraction felt by the 2s electron is smaller than you would calculate using just nuclear charge and distance, because there is also the electron-electron repulsion. This is the basis of the effective nuclear charge. Because of the electron-electron repulsions, the outer electrons in a many-electron atom feel less attraction toward the nucleus, so they feel like the nuclear charge is smaller than it actually is. So effective nuclear charge is always smaller than actual nuclear charge.
Effective nuclear charge depends on the type of electron. Electrons in s orbitals, even 4s or 5s, still spend some time right at the nucleus, and when they are there, they feel the full nuclear charge, so on average the s electrons feel a nuclear charge closer to the actual nuclear charge. Electrons in d or f orbitals really don't get very close to the nucleus, so they really get blocked, or shielded by inner electrons. They feel a smaller effective nuclear charge than s electrons. And electrons in p orbitals are in between.
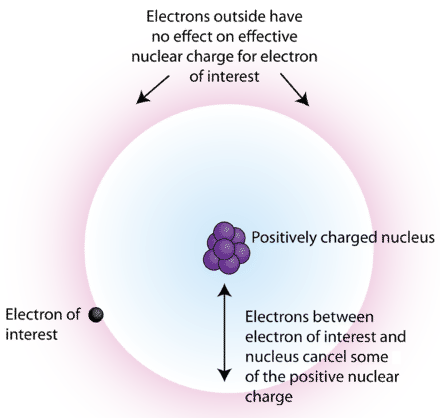
Usually if you do any calculation of orbitals for many-electron atoms, you will use effective nuclear charge instead of actual nuclear charge. The size and energy of the orbitals will depend on effective nuclear charge, not on actual nuclear charge. Size and energy of orbitals determines some very important chemical properties, including the size of the element (as an atom, ion, or in a molecule) and how easily it loses or gains electrons. Electrons take up most of the space in an atom, so orbital size tells you size. Losing electrons requires the energy by which they are bound, which is the roughly same as the orbital energy. Adding electrons only works if the orbital where they will go is lower energy than where they came from.
Outside Link
- Effective Nuclear Charge (10 min)
Contributors and Attributions
Emily V Eames (City College of San Francisco)

