Stoichiometry

Skills to Develop

• Perform stoichiometry calculations
• Distinguish dynamic equilibrium from equilibrium
• Describe why actual yield may be less than theoretical yield

Stoichiometry is a general term for relationships between amounts of substances in chemical reactions. It also describes calculations done to determine how much of a substance will be used in a reaction, left over after a reaction, produced by a reaction, etc.

How do you use it?

The calculations of theoretical yield in the previous section are simple stoichiometry calculations. To do stoichiometry calculations, you'll need:

1. A balanced chemical equation: you may need to write the equation and balance it yourself, based on what you know
2. Formula weights or molecular weights (abbreviated FW or MW) for relevant compounds: you will often need to calculate these for yourself using the periodic table and the formulas; just add up the atomic weights according to the formula
3. Usually, you'll need to figure out how many moles react; to do this, you'll need to decide what is the limiting reactant, by comparing the number of moles of each compound that are present and the coefficients in the equation
4. Finally, once you have all the parts you can do a dimensional analysis-style unit conversion to find the answer. Make sure you understand what each step does and that each step is multiplying by 1. You might also need to do some other addition or subtraction, depending on what the question asks for, such as subtracting the amount of a compound that reacts from the initial amount to find the part left over

An example of a stoichiometry calculation.

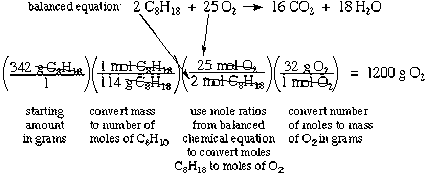
Stoichiometry and Reaction Concepts

Doing stoichiometry calculations isn't just a procedure to solve a problem in a book. It is a way to describe things that actually happen, and it is related to concepts and understanding of chemistry.

Stoichiometry calculations are based on the conservation of mass (see the Lavoisier page) and the idea that particles like nuclei and electrons aren't created or destroyed during reactions, just rearranged. These understandings allow us to make these calculations.

Stoichiometry calculations are also related to the concept of chemical equilibrium. Equilibrium means a stable state in which opposing forces are balanced. For instance, when you stand on one foot, to maintain your balance and not fall over, if you move a little to one side, you will need to correct that and move back a little in the other direction so you don't lose your balance. In chemistry, equilibrium means a state in which 2 opposite processes are occuring, but at the same rate. For instance, the reaction may go in the forward direction (to the right), and at the same time some molecules of product are turning into reactants, going in the reverse direction (to the left). In many chemical reactions, both directions are possible; when they are happening at the same rate, that is called dynamic equilibrium, which means "moving equilibrium", because the individual molecules are moving back and forth between "reactant" and "product" (which are really just defined by how you write the equation), but the total amounts of reactant and product aren't changing.

A reaction in a state of dynamic equilibrium.

When we do stoichiometry calculations, we assume that the reaction will be complete, meaning that the limiting reactant will react completely, so none is left, forming as much product as possible. However, this is only sometimes what happens. There are several reason why this might not happen.

Possible Reasons why Actual Yield is Less than Theoretical Yield

• Rate describes how fast a chemical reaction happens. Some chemical reactions are very slow, like the ones that dissolve rock and change the shape of mountains; others are very fast, or in between. In general, the rate of a reaction will depend on the conditions, such as temperature. Right now, we will mostly talk about reactions that happen pretty quickly, but if a reaction doesn't finish before you measure it, it could be because it's too slow. This is discussed more when you study chemical kinetics.

• The position of the equilibrium is another possible reason a reaction might not produce as much product as you calculate. For all reactions, equilibrium is reached before all the reactant is used; it might use almost all the reactant, so that you don't notice the tiny bit left over, or it might reach equilibrium with more than half the reactant left. If equilibrium is reached (if the reaction is fast enough), the predicted theoretical yield will only be reached if the equilibrium is almost all product. Later, you'll learn ways to predict where the equilibrium is. For now, just know that reactions can go both directions, and that the equilibrium isn't always all product.

Equilibrium may be like how many brownies you want to bake. If you want a lot, you use more ingredients (reactants). If you don't want as much, you use less ingredients, leaving more as excess.

The last possible complication is side or competing reactions. This means that some other reactions you haven't thought of or learned about might be happening, that use up some of the reactant or product. Because chemistry is really complicated and very few materials are actually truly pure, almost every real system or reaction will have some side reactions. Sometimes they are very important and prevent the thing you want to happen from happening; other times they don't matter at all.

Example of competing reactions during the formation of acyl azide from carboxylic acid.

For your calculations, you don't usually need to really worry about rate, equilibrium or side reactions, because we haven't learned strategies for dealing with them yet. However, to keep your mental models of chemical reactions matching real chemical systems, it's important to know that real reactions are more complicated than we make them seem in the first few weeks of chem class.

Contributors and Attributions

• Emily V Eames (City College of San Francisco)