13.2: Strong Monoprotic Acids and Bases

Learning Objectives

Make sure you thoroughly understand the following essential concepts that have been presented above.

• Estimate the pH of a solution of a strong acid or base, given its concentration.
• Explain the distinction between the concentration of a substance and its activity in a solution.
• Describe the origin and effects of ion-pairing in concentrated solutions of strong acids.
• Name and write formulas for the four major strong acids.

To a good approximation, strong acids, in the forms we encounter in the laboratory and in much of the industrial world, have no real existence; they are all really solutions of \(\ce{H3O^{+}}\). So if you think about it, the labels on those reagent bottles you see in the lab are not strictly true! However, if the strong acid is highly diluted, the amount of \(\ce{H3O^{+}}\) it contributes to the solution becomes comparable to that which derives from the autoprotolysis of water. Under these conditions, we need to develop a more systematic way of working out equilibrium concentrations.

At Moderate Concentrations, Forget About Equilibria

A strong acid, you will recall, is one that is stronger than the hydronium ion \(\ce{H3O^{+}}\). This means that in the presence of water, the proton on a strong acid such as HCl will "fall" into the "sink" provided by H₂O, converting the latter into its conjugate acid \(\ce{H3O^{+}}\). In other words, \(\ce{H3O^{+}}\) is the strongest acid that can exist in aqueous solution.

Figure \(\PageIndex{1}\)

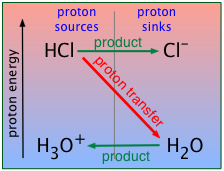
As we explained in the preceding lesson, all strong acids appear to be equally strong in aqueous solution because there are always plenty of H₂O molecules to accept their protons. This is called the "leveling effect".

Figure \(\PageIndex{2}\)

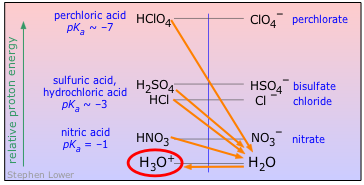
This greatly simplifies our treatment of strong acids because there is no need to deal with equilibria such as for hydrochloric acid

\[\ce{HCl + H_2O → H_3O^{+} + Cl^{–}}\]

The equilibrium constants for such reactions are so overwhelmingly large that we can usually consider the concentrations of acid species such as "HCl" to be indistinguishable from zero. As we will see further on, this is not strictly true for highly-concentrated solutions of strong acids (Figure \(\PageIndex{3}\)).

Figure \(\PageIndex{3}\)

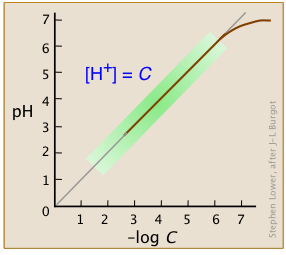
Over the normal range of concentrations we commonly work with (indicated by the green shading on this plot), the pH of a strong acid solution is given by the negative logarithm of its concentration in mol L^–1. Note that in very dilute solutions, the plot levels off, showing that this simple relation breaks down; there is no way you can make the solution alkaline by diluting an acid!

Example \(\PageIndex{1}\)

What will be the pH of a 0.025 mol/l solution of hydrochloric acid?

Solution

If we assume all the hydronium concentration originates from the added acid. So

\[\ce{H3O^{+}}\]

and we just find the negative logarithm of the concentration

\[pH = -\log_{10} [\ce{H3o^{+}}] = –\log_{10} 0.025 = 1.6\]

Since this pH is so far away from 7, our assumption is reasonable.

Major Strong Acids

Mineral acids are those that are totally inorganic. Not all mineral acids are strong; boric and carbonic acids are common examples of very weak ones. However, in ordinary usage, the term often implies one of those described below. With the exception of perchloric acid, which requires special handling, these are all widely used in industry and are almost always found in chemistry laboratories. Most have been known since ancient times

Table \(\PageIndex{1}\): Mineral Acid properties

acid	name	pKa	base	pKb
HClO4	perchloric acid	~ –7	ClO4^–	~ 21
HCl	hydrochloric acid	~ –7	Cl^–	~ 17
H2SO4	sulfuric acid	~ –7	HSO4^–	~ 17
HNO3	nitric acid	–1	NO3^–	15
H3O^+	hydronium ion	H2O	14

You should know the names and formulas of all four of these widely-encountered strong mineral acids.

• Hydrochloric acid \(HCl\): In contrast to the other strong mineral acids, no pure compound "hydrochloric acid" exists. One of the most commonly used acids, hydrochloric acid is usually sold as a constant-boiling 37% solution of hydrogen chloride gas in water, making its concentration about 10 M. Although higher concentrations are possible, the high partial pressures of HCl in equilibrium with the solution makes them difficult to store, ship and work with. Commercial-grade hydrochloric acid is often called muriatic acid.
• Nitric acid \(HNO_3\): Concentrated nitric acid is a 68% constant-boiling solution of HNO₃ in water, corresponding to about 11M. Although the acid itself is colorless, its slow decomposition into NO₂ (especially in the presence of light) often results in a yellow or orange color. More concentrated solutions are sold as "fuming nitric acid"; one form, available as "white fuming nitric acid" or "anhydrous nitricacid" contains 97.5% HNO₃ and only 2% water; the remainder consists of dissolved NO₂. Pure HNO₃ forms crystals that melt at –42°C. In addition to being a strong acid, nitric acid at high concentrations acts as a powerful oxidizing agent, a property that accounts for its use as a rocket fuel.
• Perchloric acid \(HClO_4\): This is the strongest of the mineral acids, thanks to the electron-withdrawing action of the oxygen atoms which make it energetically easier for HClO₄ to lose its proton. Its usual commercial form is a constant-boiling 72.5% solution in water. Concentrated perchloric acid is a powerful oxidizing agent that can react explosively with organic materials and certain metals. Its use in the laboratory requires special handling. A 1947 explosion of a 1000-L vat of HClO₄ in a Los Angeles electroplating plant killed 17 people and damaged over 250 homes and other buildings.
• Sulfuric acid \(H_2SO_4\): Sulfuric acid is by far the most industrially important acid, and is also the most concentrated one available. "Concentrated" sulfuric acid contains 98% by weight of H₂SO₄; its density of 1.83 kg/L and oil-like viscosity reflect this high concentration. "100% H₂SO₄" (which can be prepared However, is not stable) actually contains a variety of other species, all in equilibrium with H₂SO₄. These include H₂S₂O₇, H₂S₄O₁₃, and H₂SO₄'s autoprotolysis products H₃SO₄^– and HSO₄⁺.

Super acids

There is a class of super acids that are stronger than some of the common mineral acids. According to the classical definition, a superacid is an acid with an acidity greater than that of 100% pure sulfuric acid. Some, like fluorosulfuric acid \(FSO_3H\) are commercially available. Strong superacids are prepared by the combination of a strong Lewis acid and a strong Brønsted acid. The strongest known super acid is fluoroantimonic acid (\(H_2FSbF_6\)). This acid is so corrosive that the fumes alone will dissolve entire fume hoods, glass and plastic beakers, human skin, bone and most synthetic compounds.

Strong bases

The only strong bases that are commonly encountered are solutions of Group 1 hydroxides, mainly NaOH and KOH. Unlike most metal hydroxides, these solids are highly soluble in water, and can thus yield concentrated solutions of hydroxide ion, the strongest base that can exist in water — the ultimate aquatic proton sink. Sodium hydroxide is by far the most important of these strong bases; its common names "lye", "caustic soda" (or, in industry, often just "caustic"), reflect the diverse uses of NaOH.

Solid NaOH is usually sold in the form of pellets. When exposed to air, they become wet (deliquescence) and absorb CO₂, becoming contaminated with sodium carbonate. NaOH is the most soluble of the Group 1 hydroxides, dissolving in less than its own weight of water (111 g / 100 ml) to form a 2.8 M/L solution at 20°C. However, as with strong acids, the pH of such a solution cannot be reliably calculated from such a high concentration.

Acids at High Concentrations

At higher concentrations, intermolecular interactions and ion-pairing can cause the effective concentration (known as the activity) of \(\ce{H3O^{+}}\) to deviate from the value corresponding to the nominal or "analytical" concentration of the acid. Activities are important because only these work properly in equilibrium calculations. Also, pH is defined as the negative logarithm of the hydrogen ion activity, not its concentration. The relation between the concentration of a species and its activity is expressed by the activity coefficient \(\gamma\):

\[a = \gamma C\]

As a solution becomes more dilute, \(\gamma\) approaches unity. At ionic concentrations not exceeding about 2 M, concentrations of typical strong acids can generally be used in place of activities without serious error. Note that activities of single ions other than \(\ce{H3O^{+}}\) cannot be determined, so activity coefficients in ionic solutions are always the average, or mean, of those for the ionic species present. This quantity is denoted as \(\gamma_±\).

Table \(\PageIndex{2}\): Mean ionic activity coefficient of HCl at 25° C

molarity	\(\gamma_±\)
0.0005	0.975
0.01	0.904
0.10	0.796
1	0.809
2	1.01
5	2.38
10	10.44
12	17.25

Because activities of single ions cannot be measured, these mean values are the closest we can get to \(\{H^+\}\) in solutions of strong acids.

Activity is Important for Concentrated Samples

Activity is a practical consideration when dealing with strong mineral acids which are available at concentrations of 10 M or greater.

In a 12 M solution of hydrochloric acid, for example, the mean ionic activity coefficient is 17.25. This means that under these conditions with [H₃O⁺] = 12 M, the activity {H₃O⁺} = 12 × 17.25 = 207, corresponding to a pH of about –2.3, instead of –1.1 as might be predicted if concentrations were being used.

These very high activity coefficients also explain another phenomenon: why you can detect the odor of HCl(g) over a concentrated hydrochloric acid solution even though this acid is supposedly 100% dissociated. It turns out that the activity {HCl} (which represents the "escaping tendency" of HCl(g) from the solution) is almost 49,000 for a 10 M solution! The source of this gas is best described as the result of ion pairing.

Similarly, in a solution prepared by adding 0.5 mole of the very strong acid HClO₄ to sufficient water to make the volume 1 liter, freezing-point depression measurements indicate that the concentrations of hydronium and perchlorate ions are only about 0.4 M. This does not mean that the acid is only 80% dissociated; there is no evidence of HClO₄ molecules in the solution. What has happened is that about 20% of the \(\ce{H3O^{+}}\) and ClO₄^– ions have formed ion-pair complexes in which the oppositely-charged species are loosely bound by electrostatic forces (Figure \(\PageIndex{4}\)).

Ion-pairing reduces effective dissociation at high concentrations. If you have worked with concentrated hydrochloric acid in the lab, you will have noticed that the choking odor of hydrogen chloride gas is very apparent. How can this happen if this strong acid is really "100 percent dissociated" as all strong acids are said to be?

Figure \(\PageIndex{4}\):

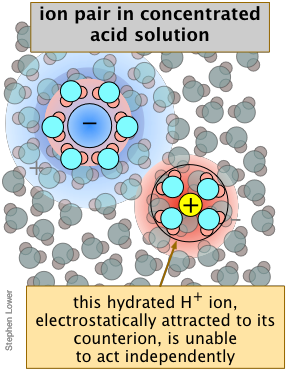
At very high concentrations, too few H₂O molecules are available to completely fill the extended hydration shells that normally help keep the ions apart, reducing the fraction of "free" \(\ce{H3O^{+}}\) ions capable of acting independently. Under these conditions, the term "dissociation" begins to lose its meaning. Although the concentration of HCl(aq) is never very high, its own activity coefficient can be as great as 2000 (Table \(\PageIndex{2}\)), which means that its escaping tendency from the solution is extremely high, so that the presence of even a tiny amount is very noticeable.

Example \(\PageIndex{2}\)

Estimate the pH of a 10.0 M solution of hydrochloric acid in which the mean ionic activity coefficient - is 10.4.

Solution

pH = – log {H⁺} ≈ – log (10.4 × 10.0) = – log 104 = – 2.0

Compare this result with what you would get by using – log[H⁺]

Systematic treatment of strong acids

In this section, we will derive expressions that relate the pH of solution of a strong acid to its concentration in a solution of pure water. We will use hydrochloric acid as an example. When HCl gas is dissolved in water, the resulting solution contains the ions H₃O⁺, OH^–, and Cl−, However, except in very concentrated solutions, the concentration of HCl is negligible; for all practical purposes, molecules of “hydrochloric acid”, HCl, do not exist in dilute aqueous solutions.

To specify the concentrations of the three species present in an aqueous solution of HCl, we need three independent relations between them. These relations are obtained by observing that certain conditions must always be true in any solution of HCl. These are:

1. The autoprotolysis equilibrium of water must always be satisfied:

\[[H_3O^+][OH^–] = K_w \label{4-1}\]

2. For any acid-base system, one can write a mass balance equation that relates the concentrations of the various dissociation products of the substance to its “nominal concentration”, which we designate here as C_a. For a solution of HCl, this equation would be

\[[HCl] + [Cl^–] = C_a \label{4-2}\]

However, since HCl is a strong acid and therefore no "HCl" exists in the solution, we can neglect the first term, so the mass balance equation becomes simply

\[[Cl^–] = C_a \label{4-3}\]

3. In any ionic solution, the sum of the positive and negative electric charges must be zero; in other words, all solutions are electrically neutral. This is known as the electroneutrality principle.

\[[H_3O^+] = [OH^–] + [Cl^–] \label{4-4}\]

The next step is to combine these three equations into a single expression that relates the hydronium ion concentration to \(C_a\). This is best done by starting with an equation that relates several quantities, such as Equation \(\ref{4-4}\), and substituting the terms that we want to eliminate. Thus we can get rid of the [Cl−] term by substituting Equation \(\ref{4-3}\) into Equation \(\ref{4-4}\) :

\[[H_3O^+] = [OH^–] + C_a \label{4-5}\]

The [OH^–] term can be eliminated by use of Equation \(\ref{4-3}\):

\[[H_3O^+] = C_a + \dfrac{K_w}{[H_3O^+]} \label{4-6}\]

This equation tells us that the hydronium ion concentration will be the same as the nominal concentration of a strong acid as long as the solution is not very dilute. Notice that Equation \(\ref{4-6}\) is a quadratic equation. Recalling that K_w = 10^–14, it is apparent that the final term of the above equation will ordinarily be very small in comparison to the other terms, so it can be ordinarily be dropped, yielding the simple relation

\[[H_3O^+] \approx C_a \label{4-7}\]

Figure \(\PageIndex{5}\)

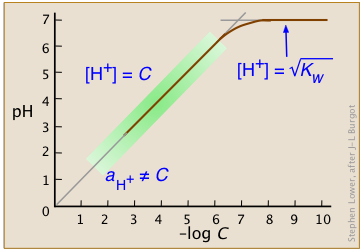
Only in extremely dilute solutions, around 10^–6 M or below (where the plot curves), does this approximation become untenable. However, even then, the effect is tiny. After all, the hydronium ion concentration in a solution of a strong acid can never fall below 10^–7 M; no amount of dilution can make the solution alkaline! So for almost all practical purposes, Equaton \(\ref{4-7}\) is all you will ever need for a solution of a strong acid.