1: INTRODUCTION

Mass Spectrometry is a powerful technique for identifying unknowns, studying molecular structure, and probing the fundamental principles of chemistry. Applications of mass spectrometry include identifying and quantitating pesticides in water samples, identifying steroids in athletes, determining metals at ppq (Parts Per Quadrillion) levels in water samples, carbon-14 dating the Shroud of Turin using only 40 mg of sample (1), looking for life on Mars, determining the mass of an ²⁸Si atom with an accuracy of 70 ppt (2), and studying the effect of molecular collision angle on reaction mechanisms.

Mass spectrometry is essentially a technique for finding the mass by weighing molecules. Obviously, this is not done with a conventional balance or scale. Instead, mass spectrometry is based up on the motion of a charged particle, called an ion, in an electric or magnetic field. The mass-to-charge ratio m/z^* of the ion effects this motion and it is actually the mass-to-charge ratio that is determined by the experiment. Since the charge of an electron is known, the mass to charge ratio a measurement of an ion’s mass. Typical mass spectrometry research focuses on the formation of gas phase ions, the chemistry of ions, and applications of mass spectrometry.

This paper covers the basics of mass spectrometry instrumentation and introduces the interpretation of mass spectra. It is only an introduction and interested readers are encouraged to consult more specialized books and journal articles for additional details. The articles and books referenced in this paper should be available at most college and university libraries.

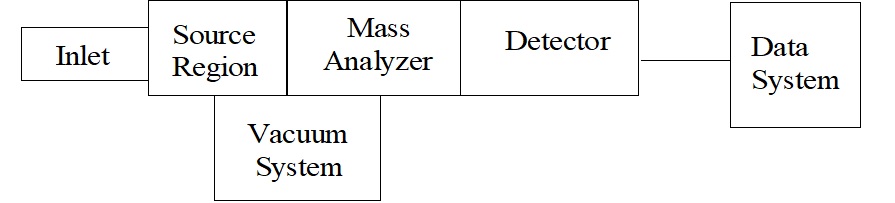
Figure \(\PageIndex{1}\) is a block diagram that shows the basic parts of a mass spectrometer. The inlet transfers the sample into the vacuum of the mass spectrometer. In the source region, neutral sample molecules are ionized and then accelerated into the mass analyzer. The mass analyzer is the heart of the mass spectrometer. This section separates ions, either in space or in time, according to their mass to charge ratio. After the ions are separated, they are detected and the signal is transferred to a data system for analysis. All mass spectrometers also have a vacuum system to maintain the low pressure, which is also called high vacuum, required for operation. High vacuum minimizes ion-molecule reactions, scattering, and neutralization of the ions. In some experiments, the pressure in the source region or a part of the mass spectrometer is intentionally increased to study these ion-molecule reactions. Under normal operation, however, any collisions will interfere with the analysis.

^* The mass to charge ratio, m/z, is used to describe ions observed in mass spectrometry. By convention, m is the numerical value for the mass of the ion and z is the numerical value for the charge of the ion. The unified atomic mass (u) and the elementary charge units e are used for these values. The unified atomic mass is defined as \(1 / 12\) the mass of an atom of ¹²C. Note: the amu is no longer an accepted term because there are conflicting definitions. Another unit, the dalton, is frequently used for polymers, peptides and other large molecules.

The elementary charge unit is defined as z is an integer equal to the number of electrons lost (or gained for negative ions). For many experiments one electron is lost during ionization so z is +1 and the m/z value is equivalent to the relative molecular mass of the ion. Because the unified atomic mass and the charge number are pure numbers the mass-to-charge ratio is a number and does not have any units. For calculations of the physical behavior of ions it is often necessary to use the actual mass (SI units of kilogram) and charge (SI units of coulomb).