6.6: Resonance

Last updated
Save as PDF

Page ID: 22186

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

An Atomic-Orbital Model of Benzene

Until now, we have discussed bonding only in terms of electron pair associated with two nuclei. These we may call localized electrons. In fact, bonding electrons can be associated with more than two nuclei, and there is a measure of stability to be gained by this because the degree of bonding increases when the electrons can distribute themselves over a greater volume. This effect often is called electron delocalization or resonance. It is important only if the component atomic orbitals overlap significantly, and this will depend in large part on the molecular geometry.

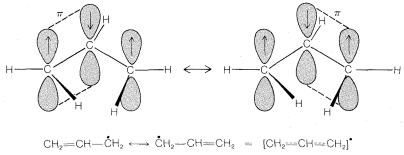
The classic example of resonance is provided by the \(\pi\) bonding of benzene. This compound was shown in Chapter 1 to have the molecular formula \(C_6H_6\), to be planar, and hexagonal with bond angles of \(120^\text{o}\), and to possess six equivalent \(C-C\) bonds and six equivalent \(C-H\) bonds. Benzene usually is written with a structural formula proposed by Kekulé:

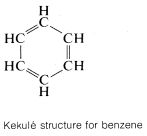
Kekule structure for benzene (all atoms are written out). Six-carbon ring with a double bond between every other carbon. — Figure 6-20. Because the \(\pi\) electrons are perfectly paired all around the ring, it is better to consider that the six electrons of benzene form a continuous \(\pi\) bond above and below the carbons of the ring. As mentioned previously, delocalization of the electrons indistinguishably over all six centers (as in benzene) corresponds to a more stable electron distribution than any in which the electrons are considered to be localized in pairs between adjacent carbons.

That benzene is more stable than a single Kekulé, or 1,3,5-cyclohexatriene, structure can be gauged by comparing the experimental heat of combustion

Benzene ring with six carbons. Each carbon has an orbital above and below it. Arrows alternate between going up/out of the orbital and down/in to the orbital. — Figure 6-19: Atomic-orbital model of benzene showing the arrangements of the \(p_z\) orbitals on each of the carbons

of benzene with the calculated value based on the average bond energies of Table 4-3:

\[\ce{C6H6(g) + 15/2 O2 -> 6CO2(g) + 3H2O(g)}\]

with

\[\begin{aligned}
&\Delta H_{\exp }^{0}=-789 \mathrm{kcal} \\
&\Delta H_{\text {calc }}^{0}=-827 \mathrm{kcal}
\end{aligned}\]

About \(38 \: \text{kcal}\) less energy is released on combustion than calculated. Benzene, therefore, is \(38 \: \text{kcal mol}^{-1}\) more stable than the cyclohexatriene structure predicts.

Left: benzene ring with orbital planes on every other carbon-carbon bond. Right: each orbital plane has shifted on over; still on every other carbon-carbon bond. — Figure 6-20: Alternative ways of forming \(\pi\) bonds in benzene through pairing of electrons in \(p\) orbitals on adjacent carbons

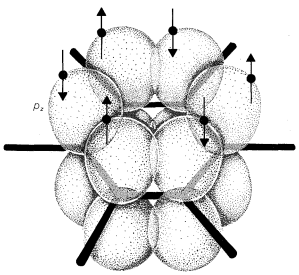
Representation of Resonance

Atomic-orbital models, like that shown for benzene, are useful descriptions of bonding from which to evaluate the potential for electron delocalization. But they are cumbersome to draw routinely. We need a simpler representation of electron delocalization.

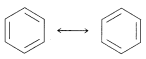
The method that commonly is used is to draw a set of structures, each of which represents a reasonable way in which the electrons (usually in \(p\) orbitals) could be paired. If more than one such structure can be written, the actual molecule, ion, or radical will have properties corresponding to some hybrid of these structures. A double-headed arrow \(\leftrightarrow\) is written between the structures that we consider to contribute to the hybrid. For example, the two Kekulé forms are two possible electron-pairing schemes or valence-bond structures that could contribute to the resonance hybrid of benzene:

Bond-line structure of benzene. 6 carbon ring with a double bond on every other carbon-carbon bond. Double sided arrows between original molecule and same ring with each double bond shifted over one. The structures are in equilibrium.

It is very important to know what attributes a reasonable set of valence-bond structures has to have to contribute to a hybrid structure. It is equally important to understand what is and what is not implied in writing a set of structures. Therefore we shall emphasize the main points to remember in the rest of this section.

1. The members of a set of structures, as the two Kekulé structures for benzene, have no individual reality. They are hypothetical structures representing different electron-pairing schemes. We are not to think of benzene as a 50:50 mixture of equilibrating Kekulé forms.

2. To be reasonable, all structures in a set representing a resonance hybrid must have exactly the same locations of the atoms in space. For example, formula \(7\) does not represent a valid member of the set of valence-bond structures of benzene, because the atoms of \(7\) have different positions from those of benzene (e.g., \(7\) is not planar):

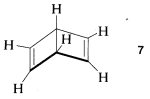
Structure \(7\) actually represents a known \(C_6H_6\) isomer that has a very different chemistry from that of benzene.

3. All members of the set must have the same number of paired or unpaired electrons. For the normal state of benzene, the six \(\pi\) electrons have three of one spin and three of the other. Structures such as \(8\), with four electrons of one spin and two of the other, are not valid contributors to the ground state of benzene:

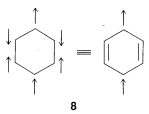
Cyclohexane molecule with arrows at every carbon. Top and bottom carbon have arrows pointing up. The two upper side carbons have arrows pointing down and the two lower side carbons have arrows pointing up. This diagram is the same has a cyclohexane molecule with two double bonds directly across from each other. The top and bottom carbon still have upward arrows.

4. The importance of resonance in any given case will depend on the energies of the contributing structures. The lower and more nearly equivalent the members of the set are in energy, the more important resonance becomes. That is to say, electron stabilization is greatest when there are two or more structures of lowest energy (as for the two Kekulé structures of benzene). As a corollary, the structure of a molecule is least likely to be satisfactorily represented by a conventional structural formula when two (or more) energetically equivalent, low-energy structures may be written.

5. If there is only one low-energy structure in the set then, to a first approximation, the resonance hybrid may be assigned properties like those expected for that structure. As an example, we show three possible pairing schemes for ethene, \(9\), \(10\), and \(11\):

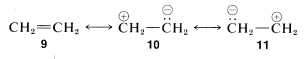
Left (figure 9) C H 2 double bonded to C H 2. This is in equilibrium with the middle figure (figure 10) which is a C H 2 cation single bonded to a C H 2 anion (has a lone pair of electrons). This is in equilibrium with figure 11; C H 2 anion singly bonded to a C H 2 cation.

Although \(10\) and \(11\) are equivalent, they are much higher in energy than \(9\) (see discussion in Section 4-4C). Therefore they do not contribute substantially to the structure of ethene that is best represented by \(9\).

Resonance is by no means restricted to organic molecules. The following sets of valence-bond structures represent the hybrid structures of nitrate ion, \(NO_3^\ominus\), carbonate ion \(CO_3^{2 \ominus}\), and nitrous oxide, \(N_2O\). These are only representative examples. We suggest that you check these structures carefully to verify that each member of a set conforms to the general rules for resonance summarized above.

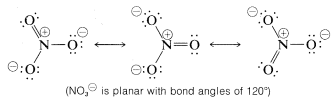
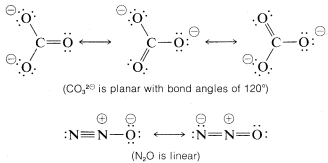
A shorthand notation of hybrid structures frequently is used in which the delocalized \(\pi\)-bonding is shown as a broken line. For benzene, an inscribed circle also is used to indicate continuous \(\pi\) bonding:

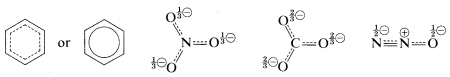
Resonance and Reactivity

Electron delocalization is an important factor in the reactivity (or lack of it) of organic molecules. As an example, recall from Chapter 4 that the bond energies of various types of \(C-H\) bonds differ considerably (see Table 4-6). In particular, the methyl \(C-H\) bond in propene is about \(9 \: \text{kcal}\) weaker than the methyl \(C-H\) bond of ethane or propane, and this difference can be explained by the use of the resonance concept. The following bond dissociations are involved:

Figure 6-21 as atomic-orbital and valence-bond structures. Consequently, the 2-propenyl radical is a resonance hybrid of two structures and is more stable than either one is expected to be. No such electron

Figure 6-21: Atomic-orbital model, valence-bond structures, and resonance-hybrid formula for the 2-propenyl radical

delocalization is possible for the propyl radical, propane, or propene. Accordingly, the methyl \(C-H\) bond strength in propene is less than in propane because of stabilization of the 2-propenyl radical.

The foregoing discussion adds further to our understanding of the selectivity observed in the halogenation reactions discussed in Chapter 4. When propene is chlorinated in sunlight, the product is 3-chloropropene, and we may explain this on the basis that the radical-chain reaction involves propagation steps in which a chlorine atom attacks the hydrogen corresponding to the weakest \(C-H\) bond:

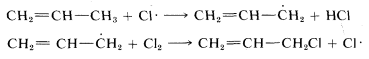
Top: C H 2 double bonded to C H single bonded to C H 3 plus C L isotope goes to C H 2 double bonded to C H single bonded to C H 2 isotope plus H C L. Bottom: C H 2 double bonded to C H single bonded to C H 2 isotope plus C L 2 goes to C H 2 double bonded to C H single bonded to C H 2 C L plus C L isotope.

The resonance theory is very useful in accounting for, and in many cases predicting, the behavior of substances with \(\pi\) bonds. However, it is not omnipotent. One example where it fails is cyclobutadiene, for which we can write two equivalent valence-bond structures corresponding to the Kekulé structures for benzene:

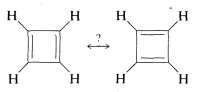
Cyclobutane molecule with two double bonds. Left: double bonds on the left and right sides. Right: Double bonds on the top and bottom.

Despite this, cyclobutadiene is an extremely unstable substance, reacting with itself almost instantly at temperatures above \(-250^\text{o}\). For better understanding of this and some related problems, we provide a more detailed discussion of electron delocalization in Chapter 21.

Contributors and Attributions

John D. Robert and Marjorie C. Caserio (1977) Basic Principles of Organic Chemistry, second edition. W. A. Benjamin, Inc. , Menlo Park, CA. ISBN 0-8053-8329-8. This content is copyrighted under the following conditions, "You are granted permission for individual, educational, research and non-commercial reproduction, distribution, display and performance of this work in any format."