\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Electron Spin or Spin Quantum Number is the fourth quantum number for electrons in atoms and molecules. Denoted as \(m_s\), the electron spin is constituted by either upward (\(m_s=+1/2\)) or downward (\(m_s=-1/2\)) arrows.
In 1920, Otto Stern and Walter Gerlach designed an experiment, which unintentionally led to the discovery that electrons have their own individual, continuous spin even as they move along their orbital of an atom. Today, this electron spin is indicated by the fourth quantum number, also known as the Electron Spin Quantum Number and denoted by ms. In 1925, Samuel Goudsmit and George Uhlenbeck made the claim that features of the hydrogen spectrum that were unexamined might by explained by assuming electrons act as if it has a spin. This spin can be denoted by an arrow pointing up, which is +1/2, or an arrow pointing down, which is -1/2.
The experiment mentioned above by Otto Stern and Walter Gerlach was done with silver which was put in an oven and vaporized. The result was that silver atoms formed a beam that passed through a magnetic field in which it split in two.
An explanation of this is that an electron has a magnetic field due to its spin. When electrons that have opposite spins are put together, there is no net magnetic field because the positive and negative spins cancel each other out. The silver atom used in the experiment has a total of 47 electrons, 23 of one spin type, and 24 of the opposite. Because electrons of the same spin cancel each other out, the one unpaired electron in the atom will determine the spin. There is a high likelihood for either spin due to the large number of electrons, so when it went through the magnetic field it split into two beams.
Note: In this module, capital "L" will be used instead of small case "l" for angular momentum quantum number.
A total of four quantum numbers were developed to better understand the movement and pathway of electrons in its designated orbital within an atom.
(For more information about the three quantum numbers above, see Quantum Number.)
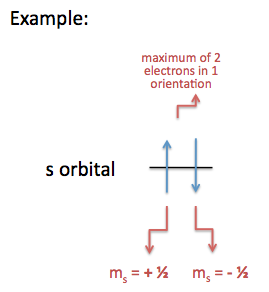
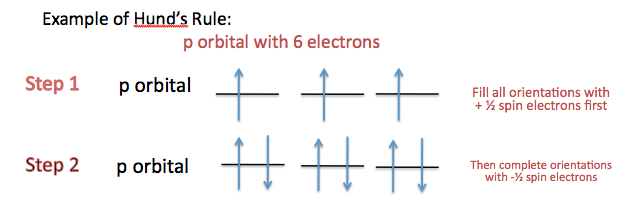
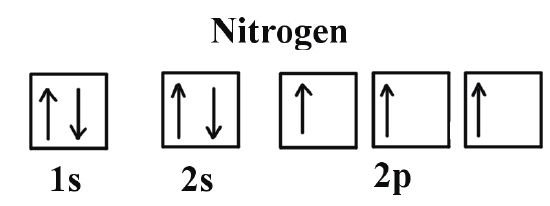
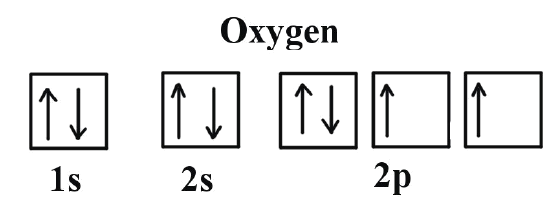
The lines represent how many orientations each orbital has, (e.g. the s-orbital has one orientation, a p-orbital has three orientations, etc.) and each line can hold up to two electrons, represented by up and down arrows. An electron with an up arrow means it has an electron spin of +\(\frac{1}{2}\), and an electron with a down arrow means it has an electron spin of -\(\frac{1}{2}\).
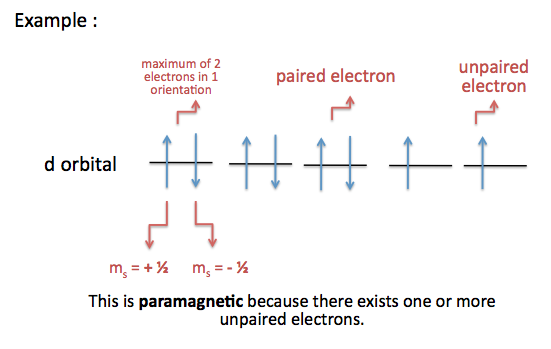
Significance: determines if an atom will or will not generate a magnetic field (For more information, scroll down to Magnetic Spin, Magnetism, and Magnetic Field Lines). Although the electron spin is limited to +½ or –½, certain rules apply when assigning electrons of different spins to fill a subshell (orientations of an orbital) . For more information, scroll down to Assigning Spin Direction.
An atom with unpaired electrons are termed as paramagnetic
An atom with paired electrons are termed as diamagnetic
Applying concepts of magnetism with liquid nitrogen and liquid oxygen:
The magnetic spin of an electron follows in the direction of the magnetic field lines as shown below.
.jpg?revision=1)
{{media("www.youtube.com/watch?v=uj0DFDfQajw\)
An effective visual on how to assign spin directions can be represented by the orbital diagram (shown previously and below.) Restrictions apply when assigning spin directions to electrons, so the following Pauli Exclusion Principle and Hund's Rule help explain this. The direction of the electron spin magnetic quantum number, ms, can only be determined its interaction with a magnetic field. Electrons whose spin is parallel to an external magnetic field (spin up with +\(\frac{1}{2}\) spin) have a lower energy than those whose magnetic spin is anti-parallel (spin down with -\(\frac{1}{2}\) spin) to the external magnetic and fill first.
When one is filling an orbital, such as the p orbital, you must fill all orbitals possible with spin up electrons before assigning the opposite spin. For example, when filling the fluorine, which will have a total of two electrons in the s orbital and a total of five electrons in the p orbital, one will start with the s orbital which will contain two electrons. So, the first electron one assigns will be spin up and the next spin down. Moving on to the three p orbitals that one will start by assigning a spin up electron in each of the three orbitals. That takes up three of the five electrons, so with the remaining two electrons, one returns to the first and second p orbital and assigns the spin down electron. This means there will be one unpaired electron in fluorine so it will be paramagnetic.
The Pauli exclusion principle declares that there can only be a maximum of two electrons for every one orientation, and the two electrons must be opposite in spin direction; meaning one electron has \(m_s = +\frac{1}{2}\) and the other electron has \(m_s = -\frac{1}{2}\).
Hund's Rule declares that the electrons in the orbital are filled up first by electrons whose spins are parallel to each other. Once all the orbitals are filled with unpaired +\(\frac{1}{2}\) spins, the orbitals are then filled with -\(\frac{1}{2}\) spin. (See examples below, labeled electronic configuration.)
(For more information on Pauli Exclusion Principle and Hund's Rules, see Electronic Configuration.)
Sulfur - S (16 electrons)
Electronic Configuration:
When given a principal quantum number, n, with either the s, p, d or f-orbital, identify all the possibilities of L, mL and ms.
Given 5f, identify all the possibilities of the four quantum numbers.
In this problem, the principal quantum number is n = 5 (the subshell number placed in front of the orbital, the f-orbital in this case). Since we are looking at the f-obital, therefore L = 3. (Look under "Subshells" in the module Quantum Numbers for more information) Knowing L = 3, we can interpret that mL = 0, \(\pm\) 1, \(\pm\) 2, \(\pm\) 3 since mL = -L,...,-1, 0, 1,...+L. As for ms, since it isn't specified in the problem as to whether it is -\(\frac{1}{2}\) or +\(\frac{1}{2}\), therefore for this problem, it could be both; meaning that the electron spin quantum number is \(\pm\)\(\frac{1}{2}\).
Given 6s and mL= +1, identify all the possibilities of the four quantum numbers.
The principal quantum number is n = 6. Looking at the s-orbital, we know that L = 0. Knowing that mL = -L,...,-1, 0, 1,...+L, therefore mL = +1 is not possible since in this problem, the interval of mL can only equal to 0 according to the angular momentum quantum number, L.
Given 4d and ms = +\(\frac{1}{2}\), identify all the possibilities of the four quantum numbers.
The principal quantum number is n = 4. Given that it is a d-orbital, we know that L = 2. Therefore, mL = 0, \(\pm\) 1, \(\pm\) 2 since mL = -L,...,-1, 0, 1,...+L. For ms, this problem specifically said ms = +\(\frac{1}{2}\); meaning that the electron spin quantum number is +\(\frac{1}{2}\).
First, draw a table labeled n, L, mLand ms, as shown below:
| n | L | mL | ms |
Then, depending on what the question is asking for, fill in the boxes accordingly. Finally, determine the number of electrons for the given quantum number, n, with regards to L, mL and ms.
How many electrons can have n = 5 and L = 1? 6
| n | L | mL | ms |
|
5 |
1 |
-1 | -\(\frac{1}{2}\), +\(\frac{1}{2}\) |
| 0 | -\(\frac{1}{2}\), +\(\frac{1}{2}\) | ||
| +1 | -\(\frac{1}{2}\), +\(\frac{1}{2}\) |
This problem includes both -\(\frac{1}{2}\), +\(\frac{1}{2}\), therefore the answer is 6 electrons based on the three possible values of mL.
How many electrons can have n = 5 and ms = -\(\frac{1}{2}\)? 9
| n | L | mL | ms |
|
5 |
4 | \(\pm\) 4 | -\(\frac{1}{2}\) |
| 3 | \(\pm\) 3 | -\(\frac{1}{2}\) | |
| 2 | \(\pm\) 2 | -\(\frac{1}{2}\) | |
| 1 | \(\pm\) 1 | -\(\frac{1}{2}\) | |
| 0 | 0 | -\(\frac{1}{2}\) |
This problem only wants the Spin Quantum Number to be -\(\frac{1}{2}\), the answer is 9 electrons based on the 9 different values of mL .
How many electrons can have n = 3, L = 2 and mL = 3? zero
| n | L | mL | ms |
| 3 | 2 | NOT POSSIBLE |
How many electrons can have n = 3, mL = +2 and ms = +\(\frac{1}{2}\)? 1
| n | L | mL | ms |
|
3 |
2 |
+2 |
+\(\frac{1}{2}\) |
This problem only wants the Spin Quantum Number to be +\(\frac{1}{2}\), the answer is 1 electron based on the value of mL and ms.
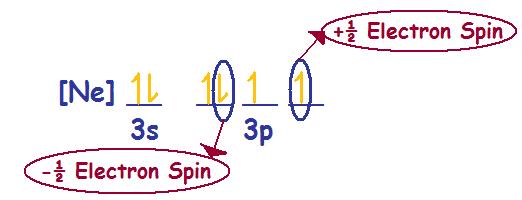
Problem (1): Sodium (Na) --> Electronic Configuration [Ne] 3s1
Spin direction for the valence electron or ms = +\(\frac{1}{2}\)
Sodium (Na) with a neutral charge of zero is paramagnetic, meaning that the electronic configuration for Na consists of one or more unpaired electrons.
Problem (2): Chlorine (Cl) --> Electronic Configuration [Ne] 3s2 3p5
Spin direction for the valence electron or ms = +\(\frac{1}{2}\)
Chlorine (Cl) with a neutral charge of zero is paramagnetic.
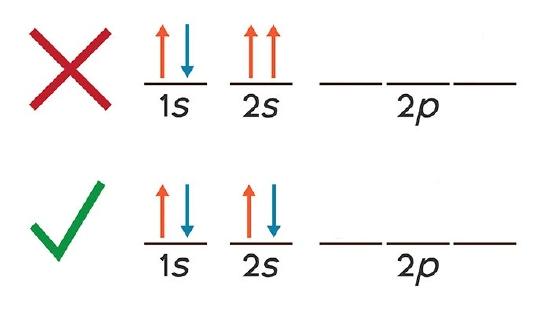
Problem (3): Calcium (Ca) --> Electronic Configuration [He] 4s2
Spin direction for the valence electron or ms = \(\pm\)\(\frac{1}{2}\)
Whereas for Calcium (Ca) with a neutral charge of zero, it is diamagnetic; meaning that ALL the electrons are paired as shown in the image above.
Problem (4): Given 5p and ms = -\(\frac{1}{2}\), identify all the possibilities of the four quantum numbers.
The principal quantum number is n = 5. Given that it is a p-orbital, we know that L = 1. And based on L, mL = 0, \(\pm\) 1 since mL = -L,...,-1, 0, 1,...+L. As for ms, this problem specifically says ms = -\(\frac{1}{2}\), meaning that the spin direction is -\(\frac{1}{2}\), pointing downwards ("down" spin).
Problem (5): Given 6f, identify all the possibilities of the four quantum numbers.
The principal quantum number is n = 6. Given that it is a f-orbital, we know that L = 3. Based on L, mL = 0, \(\pm\) 1, \(\pm\) 2, \(\pm\) 3 since mL = -L,...,-1, 0, 1,...+L. As for ms, since it isn't specified in the problem as to whether it is -\(\frac{1}{2}\) or +\(\frac{1}{2}\), therefore for this problem, it could be both; meaning that the electron spin quantum number is \(\pm\)\(\frac{1}{2}\).
Problem (6): How many electrons can have n = 4 and L = 1? 6
| n | L | mL | ms |
| 4 | 1 | \(\pm\) 1 | -\(\frac{1}{2}\), + \(\frac{1}{2}\) |
| 0 |
This problem includes both -\(\frac{1}{2}\), +\(\frac{1}{2}\), therefore the answer is 6 electrons based on the mL.
Problem (7): How many electrons can have n = 4, L = 1, mL = -2 and ms = +\(\frac{1}{2}\)? zero
| n | L | mL | ms |
| 4 | 1 | NOT POSSIBLE | + \(\frac{1}{2}\) |
Since mL = -L...-1, 0, +1...+L, mL is not possible because L = 1, so it is impossible for mL to be equal to 2 when mL MUST be with the interval of -L and +L. So, there is zero electron.
Problem (8): How many electrons can have n = 5, L = 3, mL = \(\pm\) 2 and ms = +\(\frac{1}{2}\)? 2
| n | L | mL | ms |
| 5 | 3 | \(\pm\) 2 | + \(\frac{1}{2}\) |
| \(\pm\) 1 | |||
| 0 |
This problem only wants the Spin Quantum Number to be +\(\frac{1}{2}\) and mL = \(\pm\) 2, therefore 2 electrons can have n = 5, L = 3, mL = \(\pm\) 2 and ms = +\(\frac{1}{2}\).
Problem (9): How many electrons can have n = 5, L = 4 and mL = +3? 2
| n | L | mL | ms |
| 5 | 4 | -3, +3 | -\(\frac{1}{2}\), + \(\frac{1}{2}\) |
| \(\pm\) 2 | |||
| \(\pm\) 1 | |||
| 0 |
This problem includes both -\(\frac{1}{2}\) and +\(\frac{1}{2}\) and given that mL = +3, therefore the answer is 2 electrons.
Problem (10): How many electrons can have n = 4, L = 2 and mL = \(\pm\) 1? 4
| n | L | mL | ms |
| 4 | 2 | \(\pm\) 1 | -\(\frac{1}{2}\), + \(\frac{1}{2}\) |
| 0 |
This problem includes both -\(\frac{1}{2}\) and +\(\frac{1}{2}\) and given that mL = \(\pm\) 1, therefore the answer is 4 electrons.
Problem (11): How many electrons can have n = 3, L = 3, mL = +2 and ms = -\(\frac{1}{2}\)? zero
| n | L | mL | ms |
| 3 | 3 (NOT POSSIBLE) | \(\pm\) 2 | -\(\frac{1}{2}\) |
| \(\pm\) 1 | |||
| 0 |
Since L = n - 1, there is zero electron, not possible because in this problem, n = L = 3.
The Pauli Exclusion Principle states that, in an atom or molecule, no two electrons can have the same four electronic quantum numbers. As an orbital can contain a maximum of only two electrons, the two electrons must have opposing spins. This means if one electron is assigned as a spin up (+1/2) electron, the other electron must be spin-down (-1/2) electron.
Electrons in the same orbital have the same first three quantum numbers, e.g., \(n=1\), \(l=0\), \(m_l=0\) for the 1s subshell. Only two electrons can have these numbers, so that their spin moments must be either \(m_s = -1/2\) or \(m_s = +1/2\). If the 1s orbital contains only one electron, we have one \(m_s\) value and the electron configuration is written as 1s1 (corresponding to hydrogen). If it is fully occupied, we have two \(m_s\) values, and the electron configuration is 1s2 (corresponding to helium). Visually these two cases can be represented as
As you can see, the 1s and 2s subshells for beryllium atoms can hold only two electrons and when filled, the electrons must have opposite spins. Otherwise they will have the same four quantum numbers, in violation of the Pauli Exclusion Principle.
The Aufbau section discussed how electrons fill the lowest energy orbitals first, and then move up to higher energy orbitals only after the lower energy orbitals are full. However, there is a problem with this rule. Certainly, 1s orbitals should be filled before 2s orbitals, because the 1s orbitals have a lower value of \(n\), and thus a lower energy. What about filling the three different 2p orbitals? In what order should they be filled? The answer to this question involves Hund's rule.
Hund's rule states that:
When assigning electrons to orbitals, an electron first seeks to fill all the orbitals with similar energy (also referred to as degenerate orbitals) before pairing with another electron in a half-filled orbital. Atoms at ground states tend to have as many unpaired electrons as possible. In visualizing this process, consider how electrons exhibit the same behavior as the same poles on a magnet would if they came into contact; as the negatively charged electrons fill orbitals, they first try to get as far as possible from each other before having to pair up.
Consider the correct electron configuration of the nitrogen (Z = 7) atom: 1s2 2s2 2p3
The p orbitals are half-filled; there are three electrons and three p orbitals. This is because the three electrons in the 2p subshell will fill all the empty orbitals first before pairing with electrons in them.
Keep in mind that elemental nitrogen is found in nature typically as molecular nitrogen, \(\ce{N2}\), which requires molecular orbitals instead of atomic orbitals as demonstrated above.
Next, consider oxygen (Z = 8) atom, the element after nitrogen in the same period; its electron configuration is: 1s2 2s2 2p4
Oxygen has one more electron than nitrogen; as the orbitals are all half-filled, the new electron must pair up. Keep in mind that elemental oxygen is found in nature typically as molecular oxygen, \(\ce{O_2}\), which has molecular orbitals instead of atomic orbitals as demonstrated above.
According to the first rule, electrons always enter an empty orbital before they pair up. Electrons are negatively charged and, as a result, they repel each other. Electrons tend to minimize repulsion by occupying their own orbitals, rather than sharing an orbital with another electron. Furthermore, quantum-mechanical calculations have shown that the electrons in singly occupied orbitals are less effectively screened or shielded from the nucleus. Electron shielding is further discussed in the next section.
For the second rule, unpaired electrons in singly occupied orbitals have the same spins. Technically speaking, the first electron in a sublevel could be either "spin-up" or "spin-down." Once the spin of the first electron in a sublevel is chosen, however, the spins of all of the other electrons in that sublevel depend on that first spin. To avoid confusion, scientists typically draw the first electron, and any other unpaired electron, in an orbital as "spin-up."
Consider the electron configuration for carbon atoms: 1s22s22p2: The two 2s electrons will occupy the same orbital, whereas the two 2p electrons will be in different orbital (and aligned the same direction) in accordance with Hund's rule.
Consider also the electron configuration of oxygen. Oxygen has 8 electrons. The electron configuration can be written as 1s22s22p4. To draw the orbital diagram, begin with the following observations: the first two electrons will pair up in the 1s orbital; the next two electrons will pair up in the 2s orbital. That leaves 4 electrons, which must be placed in the 2p orbitals. According to Hund’s rule, all orbitals will be singly occupied before any is doubly occupied. Therefore, two p orbital get one electron and one will have two electrons. Hund's rule also stipulates that all of the unpaired electrons must have the same spin. In keeping with convention, the unpaired electrons are drawn as "spin-up", which gives (Figure 1).
When atoms come into contact with one another, it is the outermost electrons of these atoms, or valence shell, that will interact first. An atom is least stable (and therefore most reactive) when its valence shell is not full. The valence electrons are largely responsible for an element's chemical behavior. Elements that have the same number of valence electrons often have similar chemical properties.
Electron configurations can also predict stability. An atom is most stable (and therefore unreactive) when all its orbitals are full. The most stable configurations are the ones that have full energy levels. These configurations occur in the noble gases. The noble gases are very stable elements that do not react easily with any other elements. Electron configurations can assist in making predictions about the ways in which certain elements will react, and the chemical compounds or molecules that different elements will form.
