14.1: Brønsted-Lowry Acids and Bases

 Learning Objectives

• Identify acids, bases, and conjugate acid-base pairs according to the Brønsted-Lowry definition
• Write equations for acid and base ionization reactions
• Use the ion-product constant for water to calculate hydronium and hydroxide ion concentrations
• Describe the acid-base behavior of amphiprotic substances

Acids and bases have been known for a long time. When Robert Boyle characterized them in 1680, he noted that acids dissolve many substances, change the color of certain natural dyes (for example, they change litmus from blue to red), and lose these characteristic properties after coming into contact with alkalis (bases). In the eighteenth century, it was recognized that acids have a sour taste, react with limestone to liberate a gaseous substance (now known to be CO₂), and interact with alkalis to form neutral substances. In 1815, Humphry Davy contributed greatly to the development of the modern acid-base concept by demonstrating that hydrogen is the essential constituent of acids. Around that same time, Joseph Louis Gay-Lussac concluded that acids are substances that can neutralize bases and that these two classes of substances can be defined only in terms of each other. The significance of hydrogen was reemphasized in 1884 when Svante Arrhenius defined an acid as a compound that dissolves in water to yield hydrogen cations (now recognized to be hydronium ions) and a base as a compound that dissolves in water to yield hydroxide anions.

In an earlier chapter on chemical reactions, we defined acids and bases as Arrhenius did: We identified an acid as a compound that dissolves in water to yield hydronium ions (H₃O⁺) and a base as a compound that dissolves in water to yield hydroxide ions (\(\ce{OH-}\)). This definition is not wrong; it is simply limited.

Later, we extended the definition of an acid or a base using the more general definition proposed in 1923 by the Danish chemist Johannes Brønsted and the English chemist Thomas Lowry. Their definition centers on the proton, \(\ce{H^+}\). A proton is what remains when a normal hydrogen atom, \(\ce{^1_1H}\), loses an electron. A compound that donates a proton to another compound is called a Brønsted-Lowry acid, and a compound that accepts a proton is called a Brønsted-Lowry base. An acid-base reaction is the transfer of a proton from a proton donor (acid) to a proton acceptor (base). In a subsequent chapter of this text we will introduce the most general model of acid-base behavior introduced by the American chemist G. N. Lewis.

Acids may be compounds such as HCl or H₂SO₄, organic acids like acetic acid (\(\ce{CH_3COOH}\)) or ascorbic acid (vitamin C), or H₂O. Anions (such as \(\ce{HSO_4^-}\), \(\ce{H_2PO_4^-}\), \(\ce{HS^-}\), and \(\ce{HCO_3^-}\)) and cations (such as \(\ce{H_3O^+}\), \(\ce{NH_4^+}\), and \(\ce{[Al(H_2O)_6]^{3+}}\)) may also act as acids. Bases fall into the same three categories. Bases may be neutral molecules (such as \(\ce{H_2O}\), \(\ce{NH_3}\), and \(\ce{CH_3NH_2}\)), anions (such as \(\ce{OH^-}\), \(\ce{HS^-}\), \(\ce{HCO_3^-}\), \(\ce{CO_3^{2−}}\), \(\ce{F^-}\), and \(\ce{PO_4^{3−}}\)), or cations (such as \(\ce{[Al(H_2O)_5OH]^{2+}}\)). The most familiar bases are ionic compounds such as \(\ce{NaOH}\) and \(\ce{Ca(OH)_2}\), which contain the hydroxide ion, \(\ce{OH^-}\). The hydroxide ion in these compounds accepts a proton from acids to form water:

\[\ce{H^+ + OH^- \rightarrow H_2O} \label{14.11} \]

We call the product that remains after an acid donates a proton the conjugate base of the acid. This species is a base because it can accept a proton (to re-form the acid):

\[\text{acid} \rightleftharpoons \text{proton} + \text{conjugate base}\label{14.12a} \]

\[\ce{HF \rightleftharpoons H^+ + F^-} \label{14.12b} \]

\[\ce{H_2SO_4 \rightleftharpoons H^+ + HSO_4^{−}}\label{14.12c} \]

\[\ce{H_2O \rightleftharpoons H^+ + OH^-}\label{14.12d} \]

\[\ce{HSO_4^- \rightleftharpoons H^+ + SO_4^{2−}}\label{14.12e} \]

\[\ce{NH_4^+ \rightleftharpoons H^+ + NH_3} \label{14.12f} \]

We call the product that results when a base accepts a proton the base’s conjugate acid. This species is an acid because it can give up a proton (and thus re-form the base):

\[\text{base} + \text{proton} \rightleftharpoons \text{conjugate acid} \label{14.13a} \]

\[\ce{OH^- +H^+ \rightleftharpoons H2O}\label{14.13b} \]

\[\ce{H_2O + H^+ \rightleftharpoons H3O+}\label{14.13c} \]

\[\ce{NH_3 +H^+ \rightleftharpoons NH4+}\label{14.13d} \]

\[\ce{S^{2-} +H^+ \rightleftharpoons HS-}\label{14.13e} \]

\[\ce{CO_3^{2-} +H^+ \rightleftharpoons HCO3-}\label{14.13f} \]

\[\ce{F^- +H^+ \rightleftharpoons HF} \label{14.13g} \]

In these two sets of equations, the behaviors of acids as proton donors and bases as proton acceptors are represented in isolation. In reality, all acid-base reactions involve the transfer of protons between acids and bases. For example, consider the acid-base reaction that takes place when ammonia is dissolved in water. A water molecule (functioning as an acid) transfers a proton to an ammonia molecule (functioning as a base), yielding the conjugate base of water, \(\ce{OH^-}\), and the conjugate acid of ammonia, \(\ce{NH4+}\):

 

This figure has three parts in two rows. In the first row, two diagrams of acid-base pairs are shown. On the left, a space filling model of H subscript 2 O is shown with a red O atom at the center and two smaller white H atoms attached in a bent shape. Above this model is the label “H subscript 2 O (acid)” in purple. An arrow points right, which is labeled “Remove H superscript plus.” To the right is another space filling model with a single red O atom to which a single smaller white H atom is attached. The label in purple above this model reads, “O H superscript negative (conjugate base).” Above both of these red and white models is an upward pointing bracket that is labeled “Conjugate acid-base pair.” To the right is a space filling model with a central blue N atom to which three smaller white H atoms are attached in a triangular pyramid arrangement. A label in green above reads “N H subscript 3 (base).” An arrow labeled “Add H superscript plus” points right. To the right of the arrow is another space filling model with a blue central N atom and four smaller white H atoms in a tetrahedral arrangement. The green label above reads “N H subscript 3 superscript plus (conjugate acid).” Above both of these blue and white models is an upward pointing bracket that is labeled “Conjugate acid-base pair.” The second row of the figure shows the chemical reaction, H subscript 2 O ( l ) is shown in purple, and is labeled below in purple as “acid,” plus N H subscript 3 (a q) in green, labeled below in green as “base,” followed by a double sided arrow arrow and O H superscript negative (a q) in purple, labeled in purple as “conjugate base,” plus N H subscript 4 superscript plus (a q)” in green, which is labeled in green as “conjugate acid.” The acid on the left side of the equation is connected to the conjugate base on the right with a purple line. Similarly, the base on the left is connected to the conjugate acid on the right side.

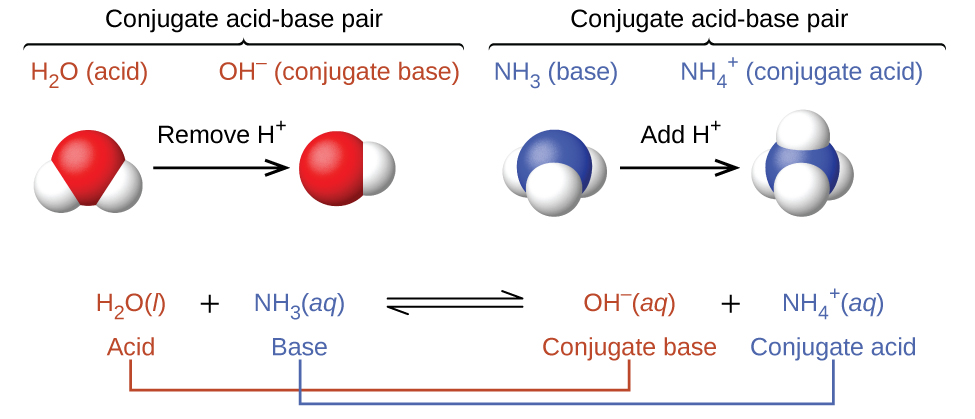
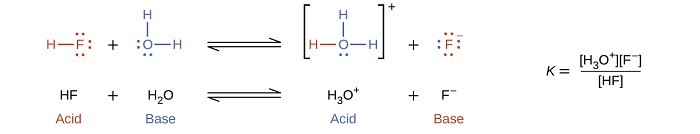
The reaction between a Brønsted-Lowry acid and water is called acid ionization. For example, when hydrogen fluoride dissolves in water and ionizes, protons are transferred from hydrogen fluoride molecules to water molecules, yielding hydronium ions and fluoride ions:

 

This figure has two rows. In both rows, a chemical reaction is shown. In the first, structural formulas are provided. In this model, in purple, an H atom is connected to an F atom with a single bond. The F atom has pairs of electron dots at the top, right, and bottom. This is followed by a plus sign, which is followed in green by an O atom which has H atoms singly bonded above and to the right. The O atom has pairs of electron dots on its left and lower sides. A double arrow follows. To the right, in brackets is a structure with a central O atom in green, with green H atoms singly bonded above and to the right. A pair of green electron dots is on the lower side of the O atom. To the left of the green O atom, a purple H atom is singly bonded. This is followed by a plus sign and an F atom in purple with pairs of electron dots above, right, below, and to the left. This atom also has a superscript negative sign. The reaction is written in symbolic form below. H F is labeled in purple below as “Acid subscript 1.” This is followed by plus H subscript 2 O, which is labeled in green below as “Base subscript 2.” A double sided arrow follows. To the right is H subscript 3 O superscript plus, which is labeled in green as below in as “Acid subscript 2.” This is followed by plus and F surrounded by 4 pairs of dots and superscript negative. The label below in purple reads, “Base subscript 1.” To the right of the reactions is the formula, K subscript a equals left bracket H subscript 3 O superscript plus right bracket left bracket F superscript negative right bracket all over left bracket H F right bracket.

When we add a base to water, a base ionization reaction occurs in which protons are transferred from water molecules to base molecules. For example, adding pyridine to water yields hydroxide ions and pyridinium ions:

Notice that both these ionization reactions are represented as equilibrium processes. The relative extent to which these acid and base ionization reactions proceed is an important topic treated in a later section of this chapter. In the preceding paragraphs we saw that water can function as either an acid or a base, depending on the nature of the solute dissolved in it. In fact, in pure water or in any aqueous solution, water acts both as an acid and a base. A very small fraction of water molecules donate protons to other water molecules to form hydronium ions and hydroxide ions:

 

This figure has two rows. In both rows, a chemical reaction is shown. In the first, structural formulas are provided. In this model, in purple, O atom which has H atoms singly bonded above and to the right. The O atom has pairs of electron dots on its left and lower sides. This is followed by a plus sign, which is followed in green by an O atom which has H atoms singly bonded above and to the right. The O atom has pairs of electron dots on its left and lower sides. A double arrow follows. To the right, in brackets is a structure with a central O atom in green, with green H atoms singly bonded above and to the right. A pair of green electron dots is on the lower side of the O atom. To the left of the green O atom, a purple H atom is singly bonded. Outside the brackets to the right is a superscript plus. This is followed by a plus sign and an O atom in purple with pairs of electron dots above, left, and below. An H atom is singly bonded to the right. This atom has a superscript negative sign. The reaction is written in symbolic form below. H subscript 2 O is labeled in purple below as “Acid subscript 1.” This is followed by plus H subscript 2 O, which is labeled in green below as “Base subscript 2.” A double sided arrow follows. To the right is H subscript 3 O superscript plus, which is labeled in green as below in as “Acid subscript 2.” This is followed by plus and O with pairs of dots above, below, and to the left with a singly bonded H on the right with a superscript negative. The label below in purple reads, “ Base subscript 1.”

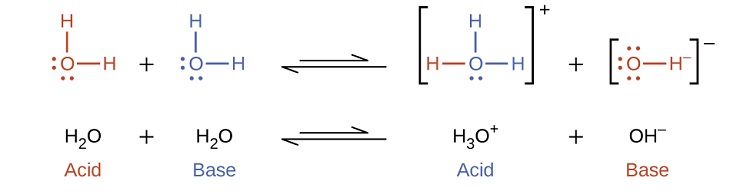
This type of reaction, in which a substance ionizes when one molecule of the substance reacts with another molecule of the same substance, is referred to as autoionization. Pure water undergoes autoionization to a very slight extent. Only about two out of every \(10^9\) molecules in a sample of pure water are ionized at 25 °C. The equilibrium constant for the ionization of water is called the ion-product constant for water (K_w):

\[\ce{H_2O}_{(l)}+\ce{H_2O}_{(l)} \rightleftharpoons \ce{H_3O^+}_{(aq)}+\ce{OH^-}_{(aq)}\;\;\; K_\ce{w}=\ce{[H_3O^+][OH^- ]} \label{14.14} \]

The slight ionization of pure water is reflected in the small value of the equilibrium constant; at 25 °C, Kw has a value of \(1.0 \times 10^{−14}\). The process is endothermic, and so the extent of ionization and the resulting concentrations of hydronium ion and hydroxide ion increase with temperature. For example, at 100 °C, the value for \(K_\ce{w}\) is approximately \(5.1 \times 10^{−13}\), roughly 50-times larger than the value at 25 °C.

Example \(\PageIndex{1}\): Ion Concentrations in Pure Water

What are the hydronium ion concentration and the hydroxide ion concentration in pure water at 25 °C?

Solution

The autoionization of water yields the same number of hydronium and hydroxide ions. Therefore, in pure water, \(\ce{[H_3O^+]} = \ce{[OH^- ]}\). At 25 °C:

\[K_\ce{w}=\ce{[H_3O^+][OH^- ]}=\ce{[H_3O^+]^2}=\ce{[OH^- ]^2}=1.0 \times 10^{−14} \nonumber \]

So:

\[\ce{[H_3O^+]}=\ce{[OH^- ]}=\sqrt{1.0 \times 10^{−14}} =1.0 \times 10^{−7}\; M \nonumber \]

The hydronium ion concentration and the hydroxide ion concentration are the same, and we find that both equal \(1.0 \times 10^{−7}\; M\).

Exercise \(\PageIndex{1}\)

The ion product of water at 80 °C is \(2.4 \times 10^{−13}\). What are the concentrations of hydronium and hydroxide ions in pure water at 80 °C?

Answer

\(\ce{[H_3O^+]} = \ce{[OH^- ]} = 4.9 \times 10^{−7}\; M\)

It is important to realize that the autoionization equilibrium for water is established in all aqueous solutions. Adding an acid or base to water will not change the position of the equilibrium. Example 14.12 demonstrates the quantitative aspects of this relation between hydronium and hydroxide ion concentrations.

Example \(\PageIndex{2}\): The Inverse Proportionality of \(\ce{[H_3O^+]}\) and \(\ce{[OH^- ]}\)

The Inverse Proportionality of [H₃O⁺] and [OH^-] A solution of carbon dioxide in water has a hydronium ion concentration of \(2.0 \times 10^{−6}\; M\). What is the concentration of hydroxide ion at 25 °C?

Solution

We know the value of the ion-product constant for water at 25 °C:

\[\ce{2 H_2O}_{(l)} \rightleftharpoons \ce{H_3O^+}_{(aq)} + \ce{OH^-}_{(aq)} \nonumber \]

\[K_\ce{w}=\ce{[H3O+][OH^- ]}=1.0 \times 10^{−14} \nonumber \]

Thus, we can calculate the missing equilibrium concentration.

Rearrangement of the K_w expression yields that \([\ce{OH^- }]\) is directly proportional to the inverse of [H₃O⁺]:

\[[\ce{OH^- }]=\dfrac{K_{\ce w}}{[\ce{H_3O^+}]}=\dfrac{1.0 \times 10^{−14}}{2.0 \times 10^{−6}}=5.0 \times 10^{−9} \nonumber \]

The hydroxide ion concentration in water is reduced to \(5.0 \times 10^{−9}\: M\) as the hydrogen ion concentration increases to \(2.0 \times 10^{−6}\; M\). This is expected from Le Chatelier’s principle; the autoionization reaction shifts to the left to reduce the stress of the increased hydronium ion concentration and the \(\ce{[OH^- ]}\) is reduced relative to that in pure water.

A check of these concentrations confirms that our arithmetic is correct:

\[\begin{align*} K_\ce{w} &=\ce{[H_3O^+][OH^- ]} \\[4pt] &=(2.0 \times 10^{−6})(5.0 \times 10^{−9})\\[4pt] &=1.0 \times 10^{−14} \end{align*} \nonumber \]

Exercise \(\PageIndex{2}\)

What is the hydronium ion concentration in an aqueous solution with a hydroxide ion concentration of 0.001 M at 25 °C?

Answer

\[\ce{[H3O+]} = 1 \times 10^{−11} M \nonumber \]

Amphiprotic Species

Like water, many molecules and ions may either gain or lose a proton under the appropriate conditions. Such species are said to be amphiprotic. Another term used to describe such species is amphoteric, which is a more general term for a species that may act either as an acid or a base by any definition (not just the Brønsted-Lowry one). Consider for example the bicarbonate ion, which may either donate or accept a proton as shown here:

\[\ce{HCO^-}_{3(aq)} + \ce{H_2O}_{(l)} \rightleftharpoons \ce{CO^{2-}}_{3(aq)} + \ce{H_3O^+}_{(aq)} \label{14.15a} \]

\[ \ce{HCO^-}_{3(aq)} + \ce{H_2O}_{(l)} \rightleftharpoons \ce{H_2CO}_{3(aq)} + \ce{OH^-}_{(aq)} \label{14.15b} \]

Example \(\PageIndex{3}\): The Acid-Base Behavior of an Amphoteric Substance

Write separate equations representing the reaction of \(\ce{HSO3-}\)

1. as an acid with \(\ce{OH^-}\)
2. as a base with HI

Solution

1. \(\ce{HSO3-}(aq)+ \ce{OH^-}(aq)\rightleftharpoons \ce{SO3^2-}(aq)+ \ce{H_2O}_{(l)} \)
2. \(\ce{HSO3-}(aq)+\ce{HI}(aq)\rightleftharpoons \ce{H2SO3}(aq)+\ce{I-}(aq)\)

Exercise \(\PageIndex{3}\)

Write separate equations representing the reaction of \(\ce{H2PO4-}\)

1. as a base with HBr
2. as an acid with \(\ce{OH^-}\)

Answer a

\(\ce{H2PO4-}(aq)+\ce{HBr}(aq)\rightleftharpoons \ce{H3PO4}(aq)+\ce{Br-}(aq)\)

Answer b

\(\ce{H2PO4-}(aq)+\ce{OH^-} (aq)\rightleftharpoons \ce{HPO4^2-}(aq)+ \ce{H_2O}_{(l)} \)

Summary

A compound that can donate a proton (a hydrogen ion) to another compound is called a Brønsted-Lowry acid. The compound that accepts the proton is called a Brønsted-Lowry base. The species remaining after a Brønsted-Lowry acid has lost a proton is the conjugate base of the acid. The species formed when a Brønsted-Lowry base gains a proton is the conjugate acid of the base. Thus, an acid-base reaction occurs when a proton is transferred from an acid to a base, with formation of the conjugate base of the reactant acid and formation of the conjugate acid of the reactant base. Amphiprotic species can act as both proton donors and proton acceptors. Water is the most important amphiprotic species. It can form both the hydronium ion, H₃O⁺, and the hydroxide ion, \(\ce{OH^-}\) when it undergoes autoionization:

\[\ce{2 H_2O}_{(l)} \rightleftharpoons \ce{H_3O^+}(aq)+\ce{OH^-} (aq) \nonumber \]

The ion product of water, K_w is the equilibrium constant for the autoionization reaction:

\[K_\ce{w}=\mathrm{[H_3O^+][OH^- ]=1.0 \times 10^{−14} \; at\; 25°C} \nonumber \]

Key Equations

• \[K_{\ce w} = \ce{[H3O+][OH^- ]} = 1.0 \times 10^{−14}\textrm{ (at 25 °C)} \nonumber \]

Glossary

acid ionization

reaction involving the transfer of a proton from an acid to water, yielding hydronium ions and the conjugate base of the acid

amphiprotic

species that may either gain or lose a proton in a reaction

amphoteric

species that can act as either an acid or a base

autoionization

reaction between identical species yielding ionic products; for water, this reaction involves transfer of protons to yield hydronium and hydroxide ions

base ionization

reaction involving the transfer of a proton from water to a base, yielding hydroxide ions and the conjugate acid of the base

Brønsted-Lowry acid

proton donor

Brønsted-Lowry base

proton acceptor

conjugate acid

substance formed when a base gains a proton

conjugate base

substance formed when an acid loses a proton

ion-product constant for water (K_w)

equilibrium constant for the autoionization of water