16.10: Electrolytic Cells and Electrolysis

Learning Objectives

Make sure you thoroughly understand the following essential ideas.

• In electrolysis, an external power source supplies the free energy required to drive a cell reaction in its non-spontaneous direction. An electrolytic cell is in this sense the opposite of a galvanic cell. In practice, the products of electrolysis are usually simpler than the reactants, hence the term electro-lysis.
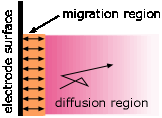
• Transport of ions in the electrolyte in response to the potential difference between the electrodes ("drift") is largely restricted to the regions very close to the electrodes. Ionic transport in the greater part of the electrolyte is by ordinary thermal diffusion— the statistical tendency of concentrations to become uniform.
• When an aqueous solution is subjected to electrolysis, the oxidation or reduction of water can be a competing process and may dominate if the applied voltage is sufficiently great. Thus an attempt to electrolyze a solution of NaNO₃ will produce only H₂ and O₂.
• A large number of electrolysis processes are employed by industry to refine metals and to produce both inorganic and organic products. The largest of these are the chloralkali industry (chlorine and "caustic"), and the refining of aluminum; the latter consumes approximately 5% of the electrical power generated in North America.

Electrolysis refers to the decomposition of a substance by an electric current. The electrolysis of sodium and potassium hydroxides, first carried out in 1808 by Sir Humphrey Davey, led to the discovery of these two metallic elements and showed that these two hydroxides which had previously been considered un-decomposable and thus elements, were in fact compounds.

Electrolysis of molten alkali halides is the usual industrial method of preparing the alkali metals:

Na^+ + e^– → Na(l)	E° = –2.71 V
Cl^– → ½ Cl2(g) + e^–	E° = –1.36 V
Na^+ + Cl^– → Na(l) + ½ Cl2(g)	E° = –4.1 V

Ions in aqueous solutions can undergo similar reactions. Thus if a solution of nickel chloride undergoes electrolysis at platinum electrodes, the reactions are

Ni^2^+ + 2 e^– → Ni(s)	E° = –0.24 V
2 Cl^– → Cl2(g) + 2 e^–	E° = –1.36 V
Ni^2^+ + 2 Cl^– → Ni(s) + Cl2(g)	E° = –1.60 v

Both of these processes are carried out in electrochemical cells which are forced to operate in the "reverse", or non-spontaneous direction, as indicated by the negative for the above cell reaction. The free energy is supplied in the form of electrical work done on the system by the outside world (the surroundings). This is the only fundamental difference between an electrolytic cell and the galvanic cell in which the free energy supplied by the cell reaction is extracted as work done on the surroundings.

A common misconception about electrolysis is that "ions are attracted to the oppositely-charged electrode." This is true only in the very thin interfacial region near the electrode surface. Ionic motion throughout the bulk of the solution occurs mostly by diffusion, which is the transport of molecules in response to a concentration gradient. Migration— the motion of a charged particle due to an applied electric field, is only a minor player, producing only about one non-random jump out of around 100,000 random ones for a 1 volt cm^–1 electric field. Only those ions that are near the interfacial region are likely to undergo migration.

Electrolysis in aqueous solutions

Water is capable of undergoing both oxidation

\[H_2O \rightarrow O_{2(g)} + 4 H^+ + 2 e^– \;\;\; E^o = -1.23 V\]

and reduction

\[2 H_2O + 2 e^– \rightarrow H_{2(g)} + 2 OH^– \;\;\; E^o = -0.83 \;V\]

Thus if an aqueous solution is subjected to electrolysis, one or both of the above reactions may be able to compete with the electrolysis of the solute. For example, if we try to electrolyze a solution of sodium chloride, hydrogen is produced at the cathode instead of sodium:

	H2O + 2 e^– → H2(g) + 2 OH^–	E =+0.41 V ([OH^–] = 10-7 M)
	Cl^– → ½ Cl2(g) + e^–	E° = –1.36 V
	Cl^– + H2O → 2 H2(g) + ½ Cl2(g) + 2 OH^–	E = –0.95 V

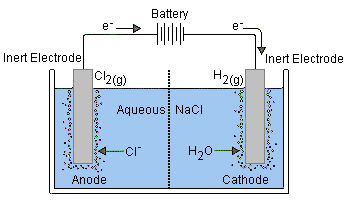
[This illustration is taken from the excellent Purdue University Chemistry site]Reduction of Na+ (E° = –2.7 v) is energetically more difficult than the reduction of water (–1.23 V), so in aqueous solution the latter will prevail.

Electrolysis of salt ("brine") is carried out on a huge scale and is the basis of the chloralkali industry.

Electrolysis of water

Pure water is an insulator and cannot undergo significant electrolysis without adding an electrolyte. If the object is to produce hydrogen and oxygen, the electrolyte must be energetically more difficult to oxidize or reduce than water itself. Electrolysis of a solution of sulfuric acid or of a salt such as NaNO₃ results in the decomposition of water at both electrodes:

	H2O + 2 e^– → H2(g) + 2 OH^–	E =+0.41 V ([OH^–] = 10^-7 M)
	2 H2O → O2(g) + 4 H+ + 2 e^–	E° = -0.82 V
	2 H2O(l) → 2 H2(g) + O2(g)	E = -1.23 V

Electrolytic production of hydrogen is usually carried out with a dilute solution of sulfuric acid. This process is generally too expensive for industrial production unless highly pure hydrogen is required. However, it becomes more efficient at higher temperatures, where thermal energy reduces the amount of electrical energy required, so there is now some interest in developing high-temperature electrolytic processes. Most hydrogen gas is manufactured by the steam reforming of natural gas.

Faraday's laws of electrolysis

One mole of electric charge (96,500 coulombs), when passed through a cell, will discharge half a mole of a divalent metal ion such as Cu²⁺. This relation was first formulated by Faraday in 1832 in the form of two laws of electrolysis:

1. The weights of substances formed at an electrode during electrolysis are directly proportional to the quantity of electricity that passes through the electrolyte.
2. The weights of different substances formed by the passage of the same quantity of electricity are proportional to the equivalent weight of each substance.

The equivalent weight of a substance is defined as the molar mass, divided by the number of electrons required to oxidize or reduce each unit of the substance. Thus one mole of V³⁺ corresponds to three equivalents of this species, and will require three faradays of charge to deposit it as metallic vanadium.

Most stoichiometric problems involving electrolysis can be solved without explicit use of Faraday's laws. The "chemistry" in these problems is usually very elementary; the major difficulties usually stem from unfamiliarity with the basic electrical units:

• current (amperes) is the rate of charge transport; 1 amp = 1 C/sec.
• power (watts) is the rate of energy production or consumption; \[1 W = 1 J/sec = 1 volt-amp; 1 watt-sec = 1 J, 1 kW-h = 3600 J.\]

Example \(\PageIndex{1}\)

A metallic object to be plated with copper is placed in a solution of CuSO₄.

1. To which electrode of a direct current power supply should the object be connected?
2. What mass of copper will be deposited if a current of 0.22 amp flows through the cell for 1.5 hours?

Solution

1. Since Cu²⁺ ions are being reduced, the object acts as a cathode and must be connected to the negative terminal (where the electrons come from!)
2. The amount of charge passing through the cell is

(0.22 amp) × (5400 sec) = 1200 C

or

(1200 C) ÷ (96500 c F^–1) = 0.012 F

Since the reduction of one mole of Cu²⁺ ion requires the addition of two moles of electrons, the mass of Cu deposited will be

(63.54 g mol^–1) (0.5 mol Cu/F) (.012 F) = 0.39 g of copper

Example \(\PageIndex{2}\)

How much electric power is required to produce 1 metric ton (1000 kg) of chlorine from brine, assuming the cells operate at 2.0 volts and assuming 100 % efficiency?

Solution

• moles of Cl₂ produced: (10⁶ g) ÷ 70 g mol^–1 = 14300 mol Cl₂
• faradays of charge: (2 F/mol) × (14300 mol) = 28600 F
• charge in coulombs: (96500 C/F) × (28600 F) = 2.76 × 10⁹ C
• duration of electrolysis: (3600 s/h) x (24 h) = 86400 s
• current (rate of charge delivery): (2.76 × 10⁹ amp-sec) ÷ (86400 sec) = 32300 amps
• power (volt-amps): (2.0 V) × (32300 amp) = 64.6 kW
• energy in kW-h: (64.6 kW) × (24 h) = 1550 kW-h
• energy in joules: (1550 kW-h) × (3.6 MJ/kW-h) = 5580 MJ (megajoules)

(In the last step, recall that 1 W = 1 J/s, so 1 kW-h = 3.6 MJ)

Industrial Electrolytic Processes

For many industrial-scale operations involving the oxidation or reduction of both inorganic and organic substances, and especially for the production of the more active metals such as sodium, calcium, magnesium, and aluminum, the most cost-effective reducing agent is electrons supplied by an external power source. The two most economically important of these processes are described below.

The chloralkali industry

The electrolysis of brine is carried out on a huge scale for the industrial production of chlorine and caustic soda (sodium hydroxide). Because the reduction potential of Na⁺ is much higher than that of water, the latter substance undergoes decomposition at the cathode, yielding hydrogen gas and OH^–.

anode reactions	2 Cl^– → Cl2(g) + 2 e^–	-1.36 V	i
	4 OH^– → O2(g) + 2 H2O + 4 e^–	-0.40 V	ii
cathode reactions	Na+ + e^– → Na(s)	-2.7 V	iii
	H2O + 2 e^– → H2(g) + 2 OH^–	+.41 V	iv

A comparison of the E°s would lead us to predict that the reduction (ii) would be favored over that of (i). This is certainly the case from a purely energetic standpoint, but as was mentioned in the section on fuel cells, electrode reactions involving O₂ are notoriously slow (that is, they are kinetically hindered), so the anodic process here is under kinetic rather than thermodynamic control. The reduction of water (iv) is energetically favored over that of Na⁺ (iii), so the net result of the electrolysis of brine is the production of Cl₂ and NaOH ("caustic"), both of which are of immense industrial importance:

\[\ce{2 NaCl + 2 H2O -> 2 NaOH + Cl2(g) + H2(g)} \nonumber\]

Since chlorine reacts with both OH^– and H₂, it is necessary to physically separate the anode and cathode compartments. In modern plants this is accomplished by means of an ion-selective polymer membrane, but prior to 1970 a more complicated cell was used that employed a pool of mercury as the cathode. A small amount of this mercury would normally find its way into the plant's waste stream, and this has resulted in serious pollution of many major river systems and estuaries and devastation of their fisheries.

Electrolytic refining of aluminum

Aluminum is present in most rocks and is the most abundant metallic element in the earth's crust (eight percent by weight.) However, its isolation is very difficult and expensive to accomplish by purely chemical means, as evidenced by the high E° (–1.66 V) of the Al³⁺/Al couple. For the same reason, aluminum cannot be isolated by electrolysis of aqueous solutions of its compounds, since the water would be electrolyzed preferentially. And if you have ever tried to melt a rock, you will appreciate the difficulty of electrolyzing a molten aluminum ore! Aluminum was in fact considered an exotic and costly metal until 1886, when Charles Hall (U.S.A) and Paul Hérault (France) independently developed a practical electrolytic reduction process.

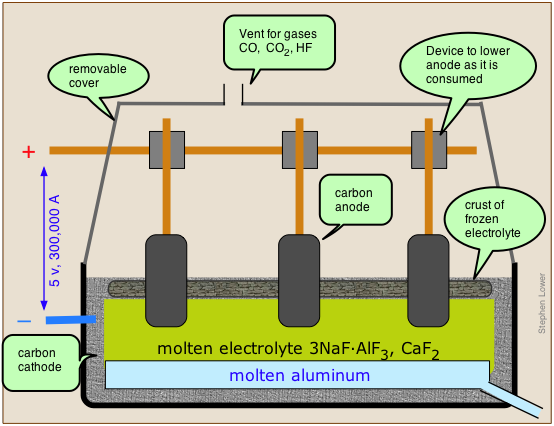
The Hall-Hérault process takes advantage of the principle that the melting point of a substance is reduced by admixture with another substance with which it forms a homogeneous phase. Instead of using the pure alumina ore Al₂O₃ which melts at 2050°C, it is mixed with cryolite, which is a natural mixture of NaF and AlF₃, thus reducing the temperature required to a more manageable 1000°C. The anodes of the cell are made of carbon (actually a mixture of pitch and coal), and this plays a direct role in the process; the carbon gets oxidized (by the oxide ions left over from the reduction of Al³⁺ to CO, and the free energy of this reaction helps drive the aluminum reduction, lowering the voltage that must be applied and thus reducing the power consumption. This is important, because aluminum refining is the largest consumer of industrial electricity, accounting for about 5% of all electricity generated in North America. Since aluminum cells commonly operated at about 100,000 amperes, even a slight reduction in voltage can result in a large saving of power.

The net reaction is

\[\ce{2 Al_2O_3 + 3 C \rightarrow 4 Al + 3 CO_2} \nonumber\]

However, large quantities of CO and of HF (from the cryolite), and hydrocarbons (from the electrodes) are formed in various side reactions, and these can be serious sources of environmental pollution.