Atomic Force Microscopy
- Page ID
- 333
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Atomic force microscopy utilizes a microscale probe to produce three dimensional image of surfaces at sub nanometer scales. The atomic force microscope obtains images by measurement of the attractive and repulsive forces acting on a microscale probe interacting with the surface of a sample. Ideally the interaction occurs at an atomically fine probe tip being attracted and repulsed by the atoms of the surface giving atomically resolved surface images.
Introduction
The atomic force microscope (AFM) probe is mounted onto a flexible cantilever that is manipulated by a vertical piezoelectric into interacting with the sample. The piezoelectric expands and exerts a force on the cantilever proportional to the potential voltage. The force is balanced by those acting on the probe by its interaction with the surface and the stain on the cantilever.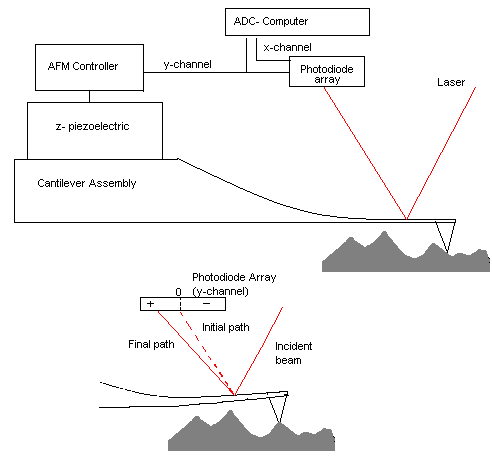
The flexible cantilever will bend proportionally to the force acting upon it in accordance to Hooke's law. By measuring the reflection of a laser source on the cantilever it is possible to determine the degree of the bend and by a feedback loop control the force exerted by the cantilever. By using the strain as a restoring force, a piezoelectric element can be used to drive the probe as a mechanical oscillator with a calculable resonate frequency, allowing for tapping mode microscopy. The laser acts on a photodiode array to give measurement of the deflection both horizontally and vertically. Using the deflection it is possible to calculate the quantity of force acting on the probe in both the horizontal and vertical directions. From the force applied by the vertical piezoelectric and the force acting on the probe it is possible to obtain a measure of the relative height of the probe.
As the probe encounters a feature, it raises with the feature, causing a deflection measured by the photodiode and a change in the force on the vertical piezoelectric. The potential may be adjusted to minimize the deflection, by feedback from the photodiode array, knowing the expansion rate of the vertical piezoelectric allows for a direct computation of the height, this is the z-sensor. By computation from the total deflection and thus strain on the cantilever, it is also possible to obtain the relative height of the feature given a known spring constant of the cantilever.
The probe is scanned across the surface, with either the probe or the sample being moved by piezoelectric elements. This allows for the measure of the interaction of the forces across the entire sample, allowing the surface to be rendered as a three dimensional image. The force exerted by the probe has the potential to alter the surface by etching or simply moving loosely bound surface features. As such, this microscopy technique can be potentially used to write (etch) as well as read, atomic scale surface features. The strain on the probe tip may cause deformation that leads to loss in resolution due to flattening or the generation of artifacts due to secondary tips.
The probe tip is idealized to be an atomically perfect spherical surface with a nanoscopic radius of curvature, leading to a single point of contact between the probe tip and the surface. Tips however may have multiple points of contact, leading to image artifacts such as doubled images or shadowing. Alternatively tips may be flattened or event indented, causing a lower resolution as smaller surface features are passed over. Significant error may arise from the expansion of the piezoelectric materials as they become heated. This problem is typically mitigated by attempting to maintain an isothermal environment. Drift may be measured and accounted for by repeat measurement of a known point and normalizing the data from that known height.
Modes of Operation
There are mutiple methods of imaging a surface using an atomic force microscope, these imaginging modes uniquely utilize the probe and its interaction with the surface to obtain data.
Contact
If a constant potential is maintained for the vertical piezoelectric, the probe will maintain continuous contact with the surface. It is then possible to use deflection and z-sensor to yield accurate height information of surface features.
Friction
When the probe is in contact with the sample the resistance acting on the probe's horizontal motion causes it to be strained horizontally. This strain is proportional to the resistance, friction, allowing for a direct measurement of the friction of the sample and probe surface.
Adhesion
Also known as non-contact mode, this method utilizes the attractive forces acting on the probe. To measure this, the probe is pulled from the sample by the vertical piezoelectric with a set force, as a feature is encountered the attractive force acting on the probe increases causing it to deflect downward. The downward deflection is counteracted by the vertical piezoelectric in a similar manner to contact mode, reaching the same equilibrium of forces acting on the cantilever.
Tapping
By using a piezoelectric device it is possible to use the cantilever as a harmonic oscillator with a resonance frequency proportional to the known spring constant. The probe then moves with an amplitude proportional to the driving force which is controlled and a frequency which is proportional to the spring constant. When the probe tip contact the surface the effective restoring force increases, increasing the frequency.
The total change in frequency is proportional to the feature's height, and vertical piezoelectric can then be used to raise the cantilever and restore the frequency to the original giving additional data from the z-sensor regarding the feature's height.
Additionally, as the probe contacts the surface it acts as a driving force, deforming the surface which is restored by the internal stress (that acts on the probe to repel it). The phenomenon is proportional to the Young's modulus of compressibility of the sample and will case a phase shift between the oscillation of the piezoelectric driver of the probe and the probe itself.
Other uses of AFM
Use of specialized probes allows a further expansion of atomic force microscopy's role in nanoscience. By alterations in probe design it is possible to: directly obtain data about the surface interaction with other functional groups, alter the surface by etching or causing chemical change, or deposit substrates onto a surface; all at the nanoscopic scale.
Chemical Force Microscopy
Functionalizing a probe can be accomplished by binding a protein or functional groups to the probe tip surface. The probe now takes direct measure of the iteration between the surface and the functional group. This technique is particularly useful for biological application, e.g. affinity of a protein to the binding site of a membrane.
Etching
The strain exerted by the tip on the surface has the potential to manipulate or alter the surface features, allowing for the mechanical etching of the system. By using specialized thermal tips, it becomes possible to heat the surface. Either technique can be used to precisely carve into or otherwise alter a surface at the scales necessary for many nanotechnologic advances.
Depositing
Use of a special tip similar to a fountain pen head, it is possible to deposit units of a substrate onto a surface. Precise deposition allows for building very precise surface structures, e.g. protien binding sites, onto an atomically flat surface.
Data recording
AFM has been demonstrated as a potential means of data storage by the IBM corperation. By using a heated tip it is possible to alter a polymer surface by a reversible polymerization reaction. The indentation created may be read by contact or tapping mode allowing for written data to be read. Data may be erased by use of the thermal tip to cause a polymerization on the surface, sealing the indentation.

