15.1: Chemical Equilibrium

Introduction

It was believed that all chemical reactions were irreversible until 1803, when French chemist Claude Louis Berthollet introduced the concept of reversible reactions. Initially he observed that sodium carbonate and calcium chloride react to yield calcium carbonate and sodium chloride; however, after observing sodium carbonate formation around the edges of salt lakes, he realized that large amount of salts in the evaporating water reacted with calcium carbonate to form sodium carbonate, indicating that the reverse reaction was occurring.

Chemical reactions are represented by chemical equations. These equations typically have a unidirectional arrow (\(\rightarrow\)) to represent irreversible reactions. Other chemical equations may have a bidirectional harpoons (\(\rightleftharpoons\)) that represent reversible reactions (not to be confused with the double headed arrows \(\leftrightarrow\) used to indicate resonance of Lewis dot structures).

Before proceeding, we need to define some conventions for reversible reactions, as either reactants or products can be created.

\[ Reactants \underset{k_{Backwards}} {\overset{k_{Forward}} {\rightleftharpoons}} Products \]

This means reactants are on the left side of the chemical equation and products are on the right, even if products react and produce reactants.

Irreversible Reactions

A fundamental concept of chemistry is that chemical reactions occurred when reactants reacted with each other to form products. These unidirectional reactions are known as irreversible reactions, reactions in which the reactants convert to products and where the products cannot convert back to the reactants. These reactions are essentially like baking. The ingredients, acting as the reactants, are mixed and baked together to form a cake, which acts as the product. This cake cannot be converted back to the reactants (the eggs, flour, etc.), just as the products in an irreversible reaction cannot convert back into the reactants.

An example of an irreversible reaction is combustion. Combustion involves burning an organic compound—such as a hydrocarbon—​and oxygen to produce carbon dioxide and water. Because water and carbon dioxide are stable, they do not react with each other to form the reactants. Combustion reactions take the following form:

\[ C_xH_y + O_2 \rightarrow CO_2 + H_2O \]

Reversible Reactions

In reversible reactions, the reactants and products are never fully consumed; they are each constantly reacting and being produced. A reversible reaction can take the following summarized form:

\[ A + B \underset{k_{-1}} {\overset{k_1} {\rightleftharpoons}} C + D \]

This reversible reaction can be broken into two reactions.

Reaction 1: \[ A + B \xrightarrow{k_1}C+D \]

Reaction 2: \[ C + D \xrightarrow{k_{-1}}A+B \]

These two reactions are occurring simultaneously, which means that the reactants are reacting to produce the products, as the products are reacting to produce the reactants. 

Video\(\PageIndex{1}\) 1:47 min Youtube from University of Surrey. This youtube shows a dynamic equilibrium involing the evaporation and condensation of water in a closed system (https://youtu.be/JsoawKguU6A ).

Kinetics and Equilibrium

Consider the simplest reversible reaction of the form

\[ A\underset{k_{-1}} {\overset{k_1} {\rightleftharpoons}} B\]

The Rates are

\[\underbrace{R_{F}=k_{1}[A]^{m}}_{\text{Forward Rate}} \; \; \text{and} \; \; \underbrace{R_{B}=k_{-1}[B]^{n}}_{\text{Backward Rate}}\]

This system is described in figure 15.1.1, where initially (at t=0), [A] = [A]₀ and [B] = 0, and the rate of consumption of A production of B is the greatest (the curves are steepest at t=0).

As soon as B accumulates the rate of the back reaction increases (producing A and consuming B) and this occurs until equilibrium is achieved, when, the forward rate equals the backward rate (also called the reverse rate). At this point the slope of the line equals zero as each species is being produced and consumed at the same rate.

Figure\(\PageIndex{1}\): graph showing relationship between kinetics and equilibrium.

At equilibrium, the backward rate equals the forward rate.

\[\begin{align} R_{F} & = R_{B} \nonumber \\ \nonumber \\ k_{1}[A]^{m} & = k_{-1}[B]^{n} \end{align}\]

Exercise \(\PageIndex{1}\)

What would figure 15.1.1 look like if the reaction was irreversible?

Answer

All the reactant would disappear, and you could have 100% yield.

Chapter 14 described Kinetics and how fast a reaction proceeds. If a reaction is reversible, it will proceed until equilbrium is achieved, and at the point in time, the concentration of the reactants and products stays constant.

At equilibrium, the forward reaction rate is equal to the reverse reaction rate.

Example \(\PageIndex{1}\)

The three reaction systems (1, 2, and 3) depicted in the accompanying illustration can all be described by the equation:

\[2A \rightleftharpoons B\]

where the blue circles are \(A\) and the purple ovals are \(B\). Each set of panels shows the changing composition of one of the three reaction mixtures as a function of time. Which system took the longest to reach chemical equilibrium?

Given: three reaction systems

Asked for: relative time to reach chemical equilibrium

Strategy:

Compare the concentrations of A and B at different times. The system whose composition takes the longest to stabilize took the longest to reach chemical equilibrium.

Solution:

In systems 1 and 3, the concentration of A decreases from \(t_0\) through \(t_2\) but is the same at both \(t_2\) and \(t_3\). Thus systems 1 and 3 are at equilibrium by \(t_3\). In system 2, the concentrations of A and B are still changing between \(t_2\) and \(t_3\), so system 2 may not yet have reached equilibrium by \(t_3\). Thus system 2 took the longest to reach chemical equilibrium.

Exercise \(\PageIndex{2}\)

In the following illustration, A is represented by blue circles, B by purple squares, and C by orange ovals; the equation for the reaction is A + B ⇌ C. The sets of panels represent the compositions of three reaction mixtures as a function of time. Which, if any, of the systems shown hdo we know for a fact has reached equilibrium?

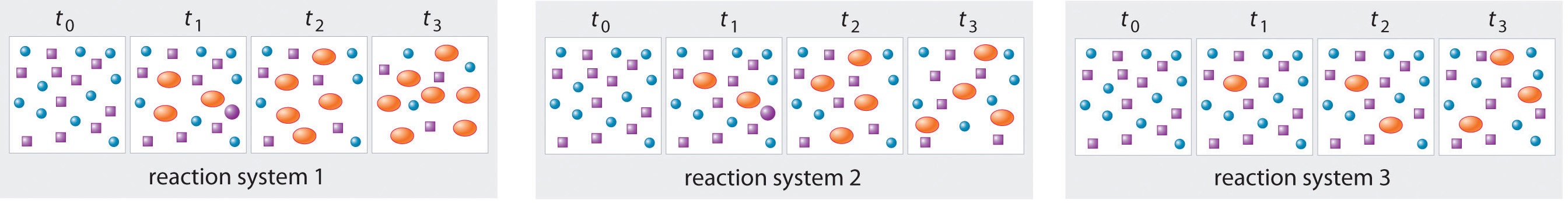
Answer

system 2

 

Test Yourself

Homework

Query \(\PageIndex{1}\)

 

 

 

Robert E. Belford (University of Arkansas Little Rock; Department of Chemistry). The breadth, depth and veracity of this work is the responsibility of Robert E. Belford, [e-mail]. You should contact him if you have any concerns. This material has both original contributions, and content built upon prior contributions of the LibreTexts Community and other resources, including but not limited to: