1.8: Density

Which one weighs more, a kilogram of feathers or a kilogram of bricks? Though many people will say that a kilogram of bricks is heavier, they actually weigh the same! However, many people are caught up by the concept of density, which causes them to answer the question incorrectly. A kilogram of feathers clearly takes up more space, but this is because it is less "dense." But what is density, and how can we determine it?

Introduction

Density (\(\rho\)) is a physical property found by dividing the mass of an object by its volume. Regardless of the sample size, density is always constant. For example, the density of a pure sample of tungsten is always 19.25 grams per cubic centimeter. This means that whether you have one gram or one kilogram of the sample, the density will never vary. The equation is as follows:

\[ Density = \dfrac{Mass}{Volume} \nonumber \]

or just

\[\rho = \dfrac{m}{v} \label{dens} \]

Based on Equation \(\ref{dens}\), it's clear that density can, and does, vary from element to element and substance to substance due to differences in the relation of mass and volume. Let us break it down one step further. What are mass and volume? We cannot understand density until we know its parts: mass and volume. The following two sections will teach you all the information you need to know about volume and mass to properly solve and manipulate the density equation.

Mass

Mass concerns the quantity of matter in an object. The SI unit for mass is the kilogram (kg), although grams (g) are commonly used in the laboratory to measure smaller quantities. Often, people mistake weight for mass. Weight concerns the force exerted on an object as a function of mass and gravity. This can be written as

\[\text{Weight} = \text{mass} \times \text{gravity} \nonumber \]

\(Weight = {m}{g}\)

Hence, weight changes due to variations in gravity and acceleration. For example, the mass of a 1 kg cube will continue to be 1 kg whether it is on the top of a mountain, the bottom of the sea, or on the moon, but its weight will differ. Another important difference between mass and weight is how they are measured. Weight is measured with a scale, while mass must be measured with a balance. Just as people confuse mass and weight, they also confuse scales and balances. A balance counteracts the effects of gravity while a scale incorporates it. There are two types of balances found in the laboratory: electronic and manual. With a manual balance, you find the unknown mass of an object by adjusting or comparing known masses until equilibrium is reached.

Volume

Volume describes the quantity of three dimensional space than an object occupies. The SI unit for volume is meters cubed (m³), but milliliters (mL), centimeters cubed (cm³), and liters (L) are more common in the laboratory. There are many equations to find volume. Here are just a few of the easy ones:

Volume = (length)³ or (length)(width)(height) or (base area)(height)

Density: A Further Investigation

We know all of density's components, so let's take a closer look at density itself. The unit most widely used to express density is g/cm³ or g/mL, though the SI unit for density is technically kg/m³. Grams per centimeter cubed is equivalent to grams per milliliter (g/cm³ = g/mL). To solve for density, simply follow the equation d = m/v. For example, if you had a metal cube with mass 7.0 g and volume 5.0 cm³, the density would be

\[\rho = \dfrac{7\,g}{5\,cm^3}= 1.4\, g/cm^3 \nonumber \]

Sometimes, you have to convert units to get the correct units for density, such as mg to g or in³ to cm³.

Density can be used to help identify an unknown element. Of course, you have to know the density of an element with respect to other elements. Below is a table listing the density of a few elements from the Periodic Table at standard conditions for temperature and pressure, or STP corresponding to a temperature of 273 K (0° Celsius) and 1 atmosphere of pressure.

Table \(\PageIndex{1}\): Density of elements

Element Name and Symbol	Density (g/cm^3)	Atomic Number
Hydrogen (H)	0.000089 \((8.9 \times 10^{-5})\) at 0 °C and 1 Atm. pressumre	1
Helium (He)	0.000164 \((1.64 \times 10^{-4})\) at 0 °C and 1 Atm. pressure	2
Aluminum (Al)	2.7	13
Zinc (Zn)	7.13	30
Tin (Sn)	7.31	50
Iron (Fe)	7.87	26
Nickel (Ni)	8.9	28
Cobalt (Co)	8.9	27
Copper (Cu)	8.96	29
Silver (Ag)	10.5	47
Lead (Pb)	11.35	82
Mercury (Hg)	11.55	80
Gold (Au)	19.32	79
Platinum (Pt)	21.45	78
Osmium (Os)	22.6	76

As can be seen from the table, the most dense element is Osmium (Os) with a density of 22.6 g/cm³. The least dense element is Hydrogen (H) with a density of 0.09 g/cm³.

Density and Temperature

Density generally decreases with increasing temperature and likewise increases with decreasing temperatures. This is because volume differs according to temperature. Volume increases with increasing temperature. If you are curious as to why the density of a pure substance could vary with temperature, check out the ChemWiki page on Van Der Waal interactions. Below is a table showing the density of pure water with differing temperatures.

Table \(\PageIndex{2}\): Density of water as a function of temperature

Temperature (C)	Density (g/cm^3)
100	0.9584
80	0.9718
60	0.9832
40	0.9922
30	0.9957
25	0.997
22	0.9978
20	0.9982
15	0.9991
10	0.9997
4	1.000
0 (liquid)	.9998
0 (solid)	0.9150

As can be seen from Table \(\PageIndex{2}\), the density of water decreases with increasing temperature. Liquid water also shows an exception to this rule from 0 degrees Celsius to 4 degrees Celsius, where it increases in density instead of decreasing as expected. Looking at the table, you can also see that ice is less dense than water. This is unusual as solids are generally denser than their liquid counterparts. Ice is less dense than water due to hydrogen bonding. In the water molecule, the hydrogen bonds are strong and compact. As the water freezes into the hexagonal crystals of ice, these hydrogen bonds are forced farther apart and the volume increases. With this volume increase comes a decrease in density. This explains why ice floats to the top of a cup of water: the ice is less dense.

Even though the rule of density and temperature has its exceptions, it is still useful. For example, it explains how hot air balloons work.

Density and Pressure

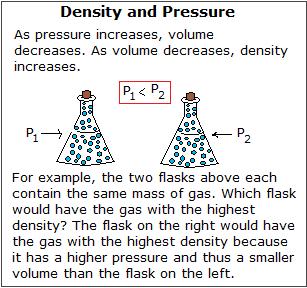
Density increases with increasing pressure because volume decreases as pressure increases. And since density=mass/volume , the lower the volume, the higher the density. This is why all density values in the Periodic Table are recorded at STP, as mentioned in the section "Density and the Periodic Table." The decrease in volume as related to pressure is explained in Boyle's Law: \(P_1V_1 = P_2V_2\) where P = pressure and V = volume. This idea is explained in the figure below. More about Boyle's Law, as well as the other gas laws, can be found here.

Archimedes' Principle

The Greek scientist Archimedes made a significant discovery in 212 B.C. The story goes that Archimedes was asked to find out for the King if his goldsmith was cheating him by replacing his gold for the crown with silver, a cheaper metal. Archimedes did not know how to find the volume of an irregularly shaped object such as the crown, even though he knew he could distinguish between elements by their density. While meditating on this puzzle in a bath, Archimedes recognized that when he entered the bath, the water rose. He then realized that he could use a similar process to determine the density of the crown! He then supposedly ran through the streets naked shouting "Eureka," which means "I found it!" in Latin.

Archimedes then tested the king's crown by taking a genuine gold crown of equal mass and comparing the densities of the two. The king's crown displaced more water than the gold crown of the same mass, meaning that the king's crown had a greater volume and thus had a smaller density than the real gold crown. The king's "gold" crown, therefore, was not made of pure gold. Of course, this tale is disputed today because Archimedes was not precise in all his measurements, which would make it hard to determine accurately the differences between the two crowns.

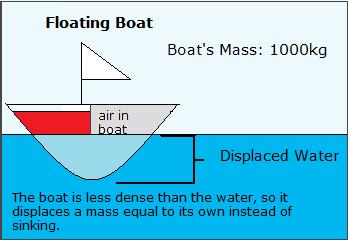
Archimedes' Principle states that if an object has a greater density than the liquid that it is placed into, it will sink and displace a volume of liquid equal to its own. If it has a smaller density, it will float and displace a mass of liquid equal to its own. If the density is equal, it will not sink or float. This principle also explains why balloons filled with helium float. Balloons, as we learned in the section concerning density and temperature, float because they are less dense than the surrounding air. Helium is less dense than the atmospheric air, so it rises. Archimedes' Principle can also be used to explain why boats float. Boats, including all the air space, within their hulls, are far less dense than water. Boats made of steel can float because they displace their mass in water without submerging all the way.

Table \(\PageIndex{3}\) below gives the densities of a few liquids to put things into perspective.

Table \(\PageIndex{3}\): Density of select liquids

Liquid	Density in kg/m^3	Density in g/cm^3
2-Methoxyethanol	964.60	0.9646
Acetic Acid	1049.10	1.049
Acetone	789.86	0.7898
Alcohol, ethyl	785.06	0.7851
Alcohol, methyl	786.51	0.7865
Ammonia	823.35	0.8234
Benzene	873.81	0.8738
Water, pure	1000.00	1.000

Percent Composition

Percent composition is very simple. Percent composition tells you by mass what percent of each element is present in a compound. A chemical compound is the combination of two or more elements. If you are studying a chemical compound, you may want to find the percent composition of a certain element within that chemical compound. The equation for percent composition is (mass of element/molecular mass) x 100.

Steps to calculating the percent composition of the elements in an compound

1. Find the molar mass of all the elements in the compound in grams per mole.
2. Find the molecular mass of the entire compound.
3. Divide the component's molar mass by the entire molecular mass.
4. You will now have a number between 0 and 1. Multiply it by 100% to get percent composition.

Tips for solving:

1. The percent composition of all elements in a compounds must add up to 100%. In a binary compound, you can find the % of the first element, then do 100%-(% first element) to get (% second element)
2. If using a calculator, you can store the overall molar mass to a variable such as "A". This will speed up calculations, and reduce errors.

Example \(\PageIndex{1}\): Phosphorus Pentachloride

What is the percent composition of phosphorus and chlorine in \(PCl_5\)?

Solution

Find the molar mass of all the elements in the compound in grams per mole.

• \(P\): \(1 \times 30.975 \,g/mol = 30.75\, g/mol\)
• \(Cl\): \(5 \times 35.453 \, g/mol = 177.265\, g/mol\)

Find the molecular mass of the entire compound.

• \(PCl_5\): \(1 \times 30.975 \,g/mol + 5 \times 35.453 \, g/mol = 208.239 \, g/mol\)

Divide the component's molar mass by the entire molecular mass.

• \(P\): \(\dfrac{30.75 \, g/mol}{208.239\, g/mol} \times 100\% = 14.87\%\)
• \(Cl\): \(\dfrac{177.265 \, g/mol}{208.239\, g/mol} \times 100\% = 85.13 \%\)

Therefore, in \(PCl_5\) is 14.87% phosphorus and 85.13% chlorine by mass.

Example \(\PageIndex{2}\): HCl

What is the percent composition of each element in hydrochloric acid (HCl).

Solution

First find the molar mass of hydrogen.

\[H = 1.00794 \,g \nonumber \]

Now find the molecular mass of the HCl molecule:

\[1.00794\,g + 35.4527\,g = 36.46064\,g \nonumber \]

Follow steps 3 and 4:

\[ \left(\dfrac{1.00794\,g}{36.46064\,g}\right) \times 100\% = 2.76\% \nonumber \]

Now just subtract to find the percent by mass of chlorine in the compound:

\[100\%-2.76\% = 97.24\% \nonumber \]

Therefore, \(HCl\) is 2.76% hydrogen and 97.24% chlorine by mass.

Percent Composition in Everyday Life

Percent composition plays an important role in everyday life. It is more than just the amount of chlorine in your swimming pool because it concerns everything from the money in your pocket to your health and how you live. The next two sections describe percent composition as it relates to you.

Nutrition Labels

The nutrition label found on the container of every bit of processed food sold by the local grocery store employs the idea of percent composition. On all nutrition labels, a known serving size is broken down in five categories: Total Fat, Cholesterol, Sodium, Total Carbohydrate, and Protein. These categories are broken down into further subcategories, including Saturated Fat and Dietary Fiber. The mass for each category, except Protein, is then converted to percent of Daily Value. Only two subcategories, Saturated Fat and Dietary Fiber are converted to percent of Daily Value. The Daily Value is based on a the mass of each category recommended per day per person for a 2000 calorie diet. The mass of protein is not converted to percent because their is no recommended daily value for protein. Following is a picture outlining these ideas.

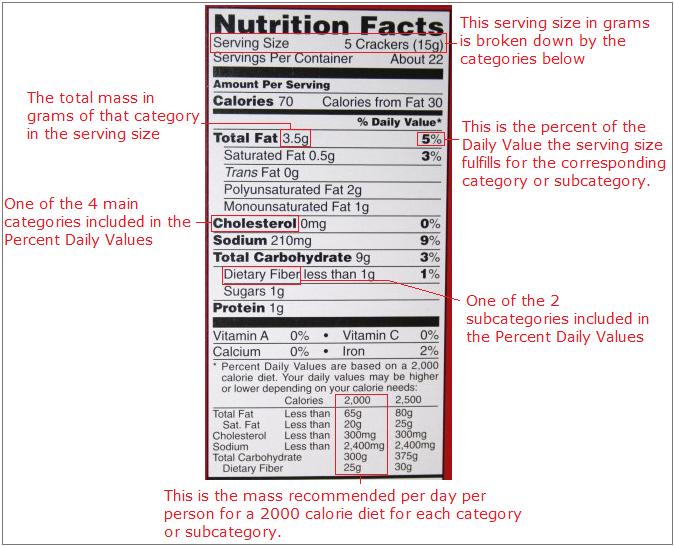
For example, if you wanted to know the percent by mass of the daily value for sodium you are eating when you eat one serving of the food with this nutrition label, then go to the category marked sodium. Look across the same row and read the percent written. If you eat one serving of this food, then you will have consumed about 9% of your daily recommended value for sodium. To find the percent mass of fat in the whole food, you could divide 3.5 grams by 15 grams, and see that this snack is 23.33% fat.

Penny: The Lucky Copper Coin

The penny should be called "the lucky copper coated coin." The penny has not been made of solid copper since part of 1857. After 1857, the US government started adding other cheaper metals to the mix. The penny, being only one cent, is literally not worth its weight in copper. People could melt copper pennies and sell the copper for more than the pennies were worth. After 1857, nickel was mixed with the more expensive copper. After 1864, the penny was made of bronze. Bronze is 95% copper and 5% zinc and tin. For one year, 1943, the penny had no copper in it due to the expense of the World War II. It was just zinc coated steel. After 1943 until 1982, the penny went through periods where it was brass or bronze.

Today, the penny in America is 2.5% copper with 97.5% zinc. The copper coats the outside of the penny while the inner portion is zinc. For comparison's sake, the penny in Canada is 94% steel, 1.5% nickel, and 4.5% copper.

The percent composition of a penny may actually affect health, particularly the health of small children and pets. Since the newer pennies are made mainly of zinc instead of copper, they are a danger to a child's health if ingested. Zinc is very susceptible to acid. If the thin copper coating is scratched and the hydrochloric acid present in the stomach comes into contact with the zinc core it could cause ulcers, anemia, kidney and liver damage, or even death in severe cases. Three important factors in penny ingestion are time, pH of the stomach, and amount of pennies ingested. Of course, the more pennies swallowed, the more danger of an overdose of zinc. The more acidic the environment, the more zinc will be released in less time. This zinc is then absorbed and sent to the liver where it begins to cause damage. In this kind of situation, time is of the essence. The faster the penny is removed, the less zinc is absorbed. If the penny or pennies are not removed, organ failure and death can occur.

Below is a picture of a scratched penny before and after it had been submerged in lemon juice. Lemon juice has a similar pH of 1.5-2.5 when compared to the normal human stomach after food has been consumed. Time elapsed: 36 hours.

As you can see, the copper is vastly unharmed by the lemon juice. That's why pennies made before 1982 with mainly copper (except the 1943 penny) are relatively safe to swallow. Chances are they would pass through the digestive system naturally before any damage could be done. Yet, it is clear that the zinc was partially dissolved even though it was in the lemon juice for only a limited amount of time. Therefore, the percent composition of post 1982 pennies is hazardous to your health and the health of your pets if ingested.

Summary

Density and percent composition are important concepts in chemistry. Each have basic components as well as broad applications. Components of density are: mass and volume, both of which can be more confusing than at first glance. An application of the concept of density is determining the volume of an irregular shape using a known mass and density. Determining Percent Composition requires knowing the mass of entire object or molecule and the mass of its components. In the laboratory, density can be used to identify an element, while percent composition is used to determine the amount, by mass, of each element present in a chemical compound. In daily life, density explains everything from why boats float to why air bubbles will try to escape from soda. It even affects your health because bone density is very important. Similarly, percent composition is commonly used to make animal feed and compounds such as the baking soda found in your kitchen.

Density Problems

These problems are meant to be easy in the beginning and then gradually become more challenging. Unless otherwise stated, answers should be in g/mL or the equivalent g/cm³.

1. If you have a 2.130 mL sample of acetic acid with mass .002234 kg, what is the density?
2. Calculate the density of a .03020 L sample of ethyl alcohol with a mass of 23.71002 g.
3. Find the density of a sample that has a volume of 36.5 L and a mass of 10.0 kg.
4. Find the volume in mL of an object that has a density of 10.2 g/L and a mass of 30.0 kg.
5. Calculate the mass in grams of an object with a volume of 23.5 mL and density of 10.0 g/L.
6. Calculate the density of a rectangular prism made of metal. The dimensions of the prism are: 5cm by 4cm by 5cm. The metal has a mass of 50 grams.
7. Find the denstiy of an unknown liquid in a beaker. The beaker's mass is 165g when there is no liquid present. With the unknown liquid, the total mass is 309g. The volume of the unknown is 125mL.
8. Determine the density in g/L of an unknown with the following information. A 55 gallon tub weighs 137.5lb when empty and 500.0 lb when filled with the unknown.
9. A ring has a mass of 5.00g and a volume of 0.476 mL. Is it pure silver?
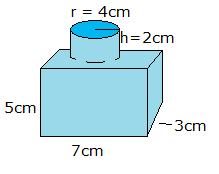
10. What is the density of the solid in the image if the mass is 40 g? Make your answer have 3 significant figures.

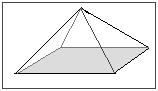
11) Below is a model of a pyramid found at an archeological dig made of an unknown substance. It is too large to find the volume by submerging it in water. Also, the scientists refuse to remove a piece to test because this pyramid is a part of history. Its height is 150.0m. The length of its base is 75.0m and the width is 50.0m. The mass of this pyramid is 5.50x10⁵kg. What is the density?

Density Problem Solutions

1. 1.049 g/mL
2. 0.7851 g/mL
3. 0.274 g/mL
4. 2.94 x 10⁶ mL
5. 0.3.27 kg
6. 0.5 g/cm³
7. 1.15 g/mL
8. 790 g/L
9. Yes
10. 0.195 g/cm³
11. 29.3 g/cm³

Percent Composition Problems

These problems will follow the same pattern of difficulty as those of density.

1. Calculate the percent by mass of each element in Cesium Fluoride (CsF).
2. Calculate the percent by mass of each element present in carbon tetrachloride (CCl₄)
3. A solution of salt and water is 33.0% salt by mass and has a density of 1.50 g/mL. What mass of the salt in grams is in 5.00L of this solution?
4. A solution of water and HCl contains 25% HCl by mass. The density of the solution is 1.05 g/mL. If you need 1.7g of HCl for a reaction, what volume of this solution will you use?
5. A solution containing 42% NaOH by mass has a density of 1.30 g/mL. What mass, in kilograms, of NaOH is in 6.00 L of this solution?

Percent Composition Problem Solutions

1. CsF is 87.5% Cs and 12.5% F by mass
2. CCl₄is 92.2% Cl and 7.8% C by mass
3. 2480g
4. 6.5mL
5. 2.38 kg

References

1. AUTOR , ARQUIMEDES , and Thomas Little . The Works of Archimedes . Courier Dover Publications, 2002.
2. Chande, D. and T. Fisher (2003). "Have a Penny? Need a Penny? Eliminating the One-Cent Coin from Circulation." Canadian Public Policy/Analyse de Politiques 29(4): 511-517.
3. Jefferson, T. (1999). "A Thought for Your Pennies." JAMA 281(2): 122.
4. Petrucci , Ralph , William Harwood , and Geoffrey Herring . Principles and Modern Application. ninth . New Jersey : Peason Eduation , 2007.
5. Rauch, F., H. Plotkin, et al. (2003). "Bone Mass, Size, and Density in Children and Adolescents with Osteogenesis Imperfecta: Effect of Intravenous Pamidronate Therapy." Journal of Bone and Mineral Research 18: 610-614.
6. Richardson, J., S. Gwaltney-Brant, et al. (2002). "Zinc Toxicosis from Penny Ingestion in Dogs." Vet Med 97(2): 96-99.
7. Tate, J. "Archimedes’ Discoveries: A Closer Look."