Chapter 14.2: The Equilibrium Constant
- Page ID
- 42119
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\) |
Prince George's Community College |
 |
| Unit I: Atoms Unit II: Molecules Unit III: States of Matter Unit IV: Reactions Unit V: Kinetics & Equilibrium Unit VI: Thermo & Electrochemistry Unit VII: Nuclear Chemistry |
||
Learning Objectives
- To know the relationship between the equilibrium constant and the rate constants for the forward and reverse reactions.
- To write an equilibrium constant expression for any reaction.
To know the relationship between the equilibrium constant and the rate constants for the forward and reverse reactions.
To write an equilibrium constant expression for any reaction.
Because an equilibrium state is achieved when the forward reaction rate equals the reverse reaction rate, under a given set of conditions there must be a relationship between the composition of the system at equilibrium and the kinetics of a reaction (represented by rate constants). We can show this relationship using the system described in Equation 14.1.1, the decomposition of N2O4 to NO2. Both the forward and reverse reactions for this system consist of a single elementary reaction, so the reaction rates are as follows:
\[\text{forward rate} = k_f[N_2O_4] \tag{14.2.1}\]
and
\[\text{reverse rate} = k_r[NO_2]^2 \tag{14.2.2}\]
At equilibrium, the forward rate equals the reverse rate:
\[ k_f[N_2O_4] = k_r[NO_2]^2 \tag{14.2.3}\]
so
\[\dfrac{k_f}{k_r}=\dfrac{[NO_2]^2}{[N_2O_4]} \tag{14.2.4}\]
The ratio of the rate constants gives us a new constant, the equilibrium constant (K)The ratio of the rate constants for the forward reaction and the reverse reaction; that is, K =kf/kr It is also the equilibrium constant calculated from solution concentrations: K =[C]c[D]d/[A]a[B]b for the general reaction aA + bB ⇌cC +dD in which each component is in solution. which is defined as follows:
\[K=\dfrac{k_f}{k_r} \tag{14.2.5}\]
Hence there is a fundamental relationship between chemical kinetics and chemical equilibrium: under a given set of conditions, the composition of the equilibrium mixture is determined by the magnitudes of the rate constants for the forward and the reverse reactions.
Note the Pattern
The equilibrium constant is equal to the rate constant for the forward reaction divided by the rate constant for the reverse reaction.
Table 14.2.1 lists the initial and equilibrium concentrations from five different experiments using the reaction system described by Equation 14.1.1. At equilibrium the magnitude of the quantity [NO2]2/[N2O4] is essentially the same for all five experiments. In fact, no matter what the initial concentrations of NO2 and N2O4 are, at equilibrium the quantity [NO2]2/[N2O4] will always be 6.53 ± 0.03 × 10−3 at 25°C, which corresponds to the ratio of the rate constants for the forward and reverse reactions. That is, at a given temperature, the equilibrium constant for a reaction always has the same value, even though the specific concentrations of the reactants and products vary depending on their initial concentrations.
Table 14.2.1 Initial and Equilibrium Concentrations for \(NO_2:N_2O_4\) Mixtures at 25°C
| Initial Concentrations | Concentrations at Equilibrium | ||||
|---|---|---|---|---|---|
| Experiment | [N2O4] (M) | [NO2] (M) | [N2O4] (M) | [NO2] (M) | K = [NO2]2/[N2O4] |
| 1 | 0.0500 | 0.0000 | 0.0417 | 0.0165 | 6.54 × 10−3 |
| 2 | 0.0000 | 0.1000 | 0.0417 | 0.0165 | 6.54 × 10−3 |
| 3 | 0.0750 | 0.0000 | 0.0647 | 0.0206 | 6.56 × 10−3 |
| 4 | 0.0000 | 0.0750 | 0.0304 | 0.0141 | 6.54 × 10−3 |
| 5 | 0.0250 | 0.0750 | 0.0532 | 0.0186 | 6.50 × 10−3 |
Developing an Equilibrium Constant Expression
In 1864, the Norwegian chemists Cato Guldberg (1836–1902) and Peter Waage (1833–1900) carefully measured the compositions of many reaction systems at equilibrium. They discovered that for any reversible reaction of the general form
\[aA+bB \rightleftharpoons cC+dD \tag{14.2.6}\]
where A and B are reactants, C and D are products, and a, b, c, and d are the stoichiometric coefficients in the balanced chemical equation for the reaction, the ratio of the product of the equilibrium concentrations of the products (raised to their coefficients in the balanced chemical equation) to the product of the equilibrium concentrations of the reactants (raised to their coefficients in the balanced chemical equation) is always a constant under a given set of conditions. This relationship is known as the law of mass action and can be stated as follows:
\[K=\dfrac{[C]^c[D]^d}{[A]^a[B]^b} \tag{14.2.7}\]
where K is the equilibrium constant for the reaction. Equation 14.2.7 is called the equilibrium equation, and the right side of Equation 14.2.7 is called the equilibrium constant expression. The relationship shown in Equation 14.2.7 is true for any pair of opposing reactions regardless of the mechanism of the reaction or the number of steps in the mechanism.
The equilibrium constant can vary over a wide range of values. The values of K shown in Table 14.2.2 , for example, vary by 60 orders of magnitude. Because products are in the numerator of the equilibrium constant expression and reactants are in the denominator, values of K greater than 103 indicate a strong tendency for reactants to form products. In this case, chemists say that equilibrium lies to the right as written, favoring the formation of products. An example is the reaction between H2 and Cl2 to produce HCl, which has an equilibrium constant of 1.6 × 1033 at 300 K. Because H2 is a good reductant and Cl2 is a good oxidant, the reaction proceeds essentially to completion. In contrast, values of K less than 10−3 indicate that the ratio of products to reactants at equilibrium is very small. That is, reactants do not tend to form products readily, and the equilibrium lies to the left as written, favoring the formation of reactants.
Table 14.2.2 Equilibrium Constants for Selected Reactions*
| Reaction | Temperature (K) | Equilibrium Constant (K) |
|---|---|---|
| \(S_{(s)}+O_{2(g)} \rightleftharpoons SO_{2(g)}\) | 300 | 4.4 × 1053 |
| \(2H_{2(g)}+O_{2(g)} \rightleftharpoons 2H2O_{(g)}\) | 500 | 2.4 × 1047 |
| \(H_{2(g)}+Cl_{2(g)} \rightleftharpoons 2HCl_{(g)}\) | 300 | 1.6 × 1033 |
| \(H_{2(g)}+Br_{2(g)} \rightleftharpoons 2HBr_{(g)}\) | 300 | 4.1 × 1018 |
| \(2NO_{(g)}+O_{2(g)} \rightleftharpoons 2NO_{2(g)}\) | 300 | 4.2 × 1013 |
| \(3H_{2(g)}+N_{2(g)} \rightleftharpoons 2NH_{3(g)}\) | 300 | 2.7 × 108 |
| \(H_{2(g)}+D_{2(g)} \rightleftharpoons 2HD_{(g)}\) | 100 | 1.92 |
| \(H_{2(g)}+I_{2(g)} \rightleftharpoons 2HI_{(g)}\) | 300 | 2.9 × 10−1 |
| \(I_{2(g)} \rightleftharpoons 2I_{(g)}\) | 800 | 4.6 × 10−7 |
| \(Br_{2(g)} \rightleftharpoons 2Br_{(g)}\) | 1000 | 4.0 × 10−7 |
| \(Cl_{2(g)} \rightleftharpoons 2Cl_{(g)}\) | 1000 | 1.8 × 10−9 |
| \(F_{2(g)} \rightleftharpoons 2F_{(g)}\) | 500 | 7.4 × 10−13 |
| *Equilibrium constants vary with temperature. The K values shown are for systems at the indicated temperatures. | ||
You will also notice in Table 14.2.2 that equilibrium constants have no units, even though Equation 14.2.7 suggests that the units of concentration might not always cancel because the exponents may vary. In fact, equilibrium constants are calculated using “effective concentrations,” or activities, of reactants and products, which are the ratios of the measured concentrations to a standard state of 1 M. As shown in Equation 14.2.8, the units of concentration cancel, which makes K unitless as well:
\[ \dfrac{[A]_{measured}}{[A]_{standard\; state}}=\dfrac{\cancel{M}}{\cancel{M}} = \dfrac{\cancel{\frac{mol}{L}}}{\cancel{\frac{mol}{L}}} \tag{14.2.8}\]
Many reactions have equilibrium constants between 1000 and 0.001 (103 ≥ K ≥ 10−3), neither very large nor very small. At equilibrium, these systems tend to contain significant amounts of both products and reactants, indicating that there is not a strong tendency to form either products from reactants or reactants from products. An example of this type of system is the reaction of gaseous hydrogen and deuterium, a component of high-stability fiber-optic light sources used in ocean studies, to form HD:
\[H_{2(g)}+D_{2(g)} \rightleftharpoons 2HD_{(g)} \tag{14.2.9}\]
The equilibrium constant expression for this reaction is
\[K= \dfrac{[HD]^2}{[H_2][D_2]}\]
with K varying between 1.9 and 4 over a wide temperature range (100–1000 K). Thus an equilibrium mixture of H2, D2, and HD contains significant concentrations of both product and reactants.
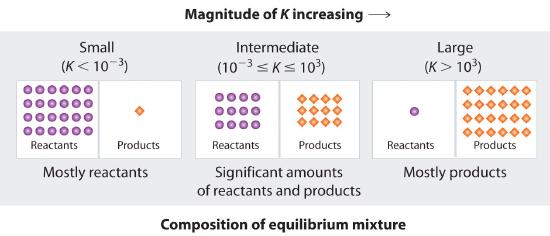
Figure 14.2.1 summarizes the relationship between the magnitude of K and the relative concentrations of reactants and products at equilibrium for a general reaction, written as reactants ⇌ products Because there is a direct relationship between the kinetics of a reaction and the equilibrium concentrations of products and reactants (Equation 14.2.8 and Equation 14.2.7), when kf >> kr, K is a large number, and the concentration of products at equilibrium predominate. This corresponds to an essentially irreversible reaction. Conversely, when kf << kr, K is a very small number, and the reaction produces almost no products as written. Systems for which kf ≈ kr have significant concentrations of both reactants and products at equilibrium.
Figure 14.2.1 The Relationship between the Composition of the Mixture at Equilibrium and the Magnitude of the Equilibrium ConstantThe larger the K, the farther the reaction proceeds to the right before equilibrium is reached, and the greater the ratio of products to reactants at equilibrium.
Note the Pattern
A large value of the equilibrium constant K means that products predominate at equilibrium; a small value means that reactants predominate at equilibrium.
Example 14.2.1
Write the equilibrium constant expression for each reaction.
- \(N_{2(g)}+3H_{2(g)} \rightleftharpoons 2NH_{3(g)}\)
- \(CO_{(g)}+\frac{1}{2}O_{2(g)} \rightleftharpoons CO_{2(g)}\)
- \(2CO_{2(g)} \rightleftharpoons 2CO_{(g)}+O_{2(g)}\)
Given: balanced chemical equations
Asked for: equilibrium constant expressions
Strategy:
Refer to Equation 14.2.7. Place the arithmetic product of the concentrations of the products (raised to their stoichiometric coefficients) in the numerator and the product of the concentrations of the reactants (raised to their stoichiometric coefficients) in the denominator.
Solution:
-
The only product is ammonia, which has a coefficient of 2. For the reactants, N2 has a coefficient of 1 and H2 has a coefficient of 3. The equilibrium constant expression is as follows:
\[\dfrac{[NH_3]^2}{[N_2][H_2]^3}\] -
The only product is carbon dioxide, which has a coefficient of 1. The reactants are CO, with a coefficient of 1, and O2, with a coefficient of 1/2 Thus the equilibrium constant expression is as follows:
\[\dfrac{[CO_2]}{[CO][O_2]^{1/2}}\] -
This reaction is the reverse of the reaction in part b, with all coefficients multiplied by 2 to remove the fractional coefficient for O2. The equilibrium constant expression is therefore the inverse of the expression in part b, with all exponents multiplied by 2:
\[\dfrac{[CO]^2[O_2]}{[CO_2]^2}\]
Exercise
Write the equilibrium constant expression for each reaction.
- \(N_2O_{(g)} \rightleftharpoons N_{2(g)}+\frac{1}{2}O_{2(g)}\)
- \(2C_8H_{18(g)}+25O_{2(g)} \rightleftharpoons 16CO_{2(g)}+18H_2O_{(g)}\)
- \(H_{2(g)}+I_{2(g)} \rightleftharpoons 2HI_{(g)}\)
Answer:
- \(K=\dfrac{[N_2][O_2]^{1/2}}{[N_2O]}\)
- \(K=\dfrac{[CO_2]^{16}[H_2O]^{18}}{[C_8H_{18}]^2[O_2]^{25}}\)
- \(K=\dfrac{[HI]^2}{[H_2][I_2]}\)
Example 14.2.2
Predict which systems at equilibrium will (a) contain essentially only products, (b) contain essentially only reactants, and (c) contain appreciable amounts of both products and reactants.
- \(H_{2(g)}+I_{2(g)} \rightleftharpoons 2HI_{(g)}\;\;\; K_{(700K)}=54\)
- \(2CO_{2(g)} \rightleftharpoons 2CO_{(g)}+O_{2(g)}\;\;\; K_{(1200K)}=3.1 \times 10^{−18}\)
- \(PCl_{5(g)} \rightleftharpoons PCl_{3(g)}+Cl_{2(g)}\;\;\; K_{(613K)}=97\)
- \(2O_{3(g)} \rightleftharpoons 3O_{2(g)} \;\;\; K_{(298 K)}=5.9 \times 10^{55}\)
Given: systems and values of K
Asked for: composition of systems at equilibrium
Strategy:
Use the value of the equilibrium constant to determine whether the equilibrium mixture will contain essentially only products, essentially only reactants, or significant amounts of both.
Solution:
- Only system 4 has K >> 103, so at equilibrium it will consist of essentially only products.
- System 2 has K << 10−3, so the reactants have little tendency to form products under the conditions specified; thus, at equilibrium the system will contain essentially only reactants.
- Both systems 1 and 3 have equilibrium constants in the range 103 ≥ K ≥ 10−3, indicating that the equilibrium mixtures will contain appreciable amounts of both products and reactants.
Exercise
Hydrogen and nitrogen react to form ammonia according to the following balanced chemical equation:
\[3H_{2(g)}+N_{2(g)} \rightleftharpoons 2NH_{3(g)}\]
Values of the equilibrium constant at various temperatures were reported as K25°C = 3.3 × 108, K177°C = 2.6 × 103, and K327°C = 4.1.
- At which temperature would you expect to find the highest proportion of H2 and N2 in the equilibrium mixture?
- Assuming that the reaction rates are fast enough so that equilibrium is reached quickly, at what temperature would you design a commercial reactor to operate to maximize the yield of ammonia?
Answer:
- 327°C, where K is smallest
- 25°C
Variations in the Form of the Equilibrium Constant Expression
Because equilibrium can be approached from either direction in a chemical reaction, the equilibrium constant expression and thus the magnitude of the equilibrium constant depend on the form in which the chemical reaction is written. For example, if we write the reaction described in Equation 14.2.6 in reverse, we obtain the following:
\[cC+dD \rightleftharpoons aA+bB \tag{14.2.10}\]
The corresponding equilibrium constant \(K′\) is as follows:
\[K'=\dfrac{[A]^a[B]^b}{[C]^c[D]^d} \tag{14.2.11}\]
This expression is the inverse of the expression for the original equilibrium constant, so K′ = 1/K. That is, when we write a reaction in the reverse direction, the equilibrium constant expression is inverted. For instance, the equilibrium constant for the reaction N2O4 ⇌2NO2 is as follows:
\[K=\dfrac{[NO_2]^2}{[N_2O_4]} \tag{14.2.12}\]
but for the opposite reaction, 2NO2 ⇌ N2O4 the equilibrium constant K′ is given by the inverse expression:
\[K'=\dfrac{[N_2O_4]}{[NO_2]^2} \tag{14.2.13}\]
Consider another example, the formation of water: 2H2(g) + O2(g) ⇌ 2H2O(g) Because H2 is a good reductant and O2 is a good oxidant, this reaction has a very large equilibrium constant (K = 2.4 × 1047 at 500 K). Consequently, the equilibrium constant for the reverse reaction, the decomposition of water to form O2 and H2, is very small: K′ = 1/K = 1/(2.4 × 1047) = 4.2 × 10−48. As suggested by the very small equilibrium constant, and fortunately for life as we know it, a substantial amount of energy is indeed needed to dissociate water into H2 and O2.
Note the Pattern
The equilibrium constant for a reaction written in reverse is the inverse of the equilibrium constant for the reaction as written originally.
Writing an equation in different but chemically equivalent forms also causes both the equilibrium constant expression and the magnitude of the equilibrium constant to be different. For example, we could write the equation for the reaction
\[2NO_2 \rightleftharpoons N_2O_4\]
as
\[NO_2 \rightleftharpoons \frac{1}{2}N_2O_4\]
with the equilibrium constant K″ is as follows:
\[ K′′=\dfrac{[N_2O_4]^{1/2}}{[NO_2]} \tag{14.2.14}\]
The values for K′ (Equation 14.2.13) and K″ are related as follows:
\[ K′′=(K')^{1/2}=\sqrt{K'} \tag{14.2.15}\]
In general, if all the coefficients in a balanced chemical equation are subsequently multiplied by n, then the new equilibrium constant is the original equilibrium constant raised to the nth power.
Example 14.2.3
At 745 K, K is 0.118 for the following reaction:
\[N_{2(g)}+3H_{2(g)} \rightleftharpoons 2NH_{3(g)}\]
What is the equilibrium constant for each related reaction at 745 K?
- \(2NH_{3(g)} \rightleftharpoons N2(g)+3H_{2(g)}\)
- \(\frac{1}{2}N_{2(g)}+\frac{3}{2}H_{2(g)} \rightleftharpoons NH_{3(g)}\)
Given: balanced equilibrium equation, K at a given temperature, and equations of related reactions
Asked for: values of K for related reactions
Strategy:
Write the equilibrium constant expression for the given reaction and for each related reaction. From these expressions, calculate K for each reaction.
Solution:
The equilibrium constant expression for the given reaction of N2(g) with H2(g) to produce NH3(g) at 745 K is as follows:
\[K=\dfrac{[NH_3]^2}{[N_2][H_2]^3}=0.118\]
-
This reaction is the reverse of the one given, so its equilibrium constant expression is as follows:
\[K'=\dfrac{1}{K}=\dfrac{[N_2][H_2]^3}{[NH_3]^2}=\dfrac{1}{0.118}=8.47\] -
In this reaction, the stoichiometric coefficients of the given reaction are divided by 2, so the equilibrium constant is calculated as follows:
\[K′′=\dfrac{[NH_3]}{[N_2]^{1/2}[H_2]^{3/2}}=K^{1/2}=\sqrt{K}=\sqrt{0.118} = 0.344\]
Exercise
At 527°C, the equilibrium constant for the reaction
\[2SO_{2(g)}+O_{2(g)} \rightleftharpoons 2SO_{3(g)}\]
is 7.9 × 104. Calculate the equilibrium constant for the following reaction at the same temperature:
\[SO_{3(g)} \rightleftharpoons SO_{2(g)}+\frac{1}{2}O_{2(g)}\]
Answer: 3.6 × 10−3
Equilibrium Constant Expressions for Systems that Contain Gases
For reactions that involve species in solution, the concentrations used in equilibrium calculations are usually expressed in moles/liter. For gases, however, the concentrations are usually expressed in terms of partial pressures rather than molarity, where the standard state is 1 atm of pressure. The symbol KpAn equilibrium constant expressed as the ratio of the partial pressures of the products and reactants, each raised to its coefficient in the chemical equation. is used to denote equilibrium constants calculated from partial pressures. For the general reaction aA + bB ⇌ cC + dD in which all the components are gases, we can write the equilibrium constant expression as the ratio of the partial pressures of the products and reactants (each raised to its coefficient in the chemical equation):
\[K_p=\dfrac{(P_C)^c(P_D)^d}{(P_A)^a(P_B)^b} \tag{14.2.16}\]
Thus Kp for the decomposition of N2O4 (Equation 14.1.1) is as follows:
\[K_p=\dfrac{(P_{NO_2})^2}{P_{N_2O_4}} \tag{14.2.17}\]
Like K, Kp is a unitless quantity because the quantity that is actually used to calculate it is an “effective pressure,” the ratio of the measured pressure to a standard state of 1 bar (approximately 1 atm), which produces a unitless quantity.The “effective pressure” is called the fugacity, just as activity is the effective concentration.
Because partial pressures are usually expressed in atmospheres or Torr, the molar concentration of a gas and its partial pressure do not have the same numerical value. Consequently, the numerical values of K and Kp are usually different. They are, however, related by the ideal gas constant (R) and the temperature (T):
\[K_p = K(RT)^{Δn} \tag{14.2.18}\]
where K is the equilibrium constant expressed in units of concentration and Δn is the difference between the numbers of moles of gaseous products and gaseous reactants (np − nr). The temperature is expressed as the absolute temperature in kelvins. You can prove this by starting from the ideal gas law for the partial pressure of each gas piV = niRT and the concentration of each gas is given by ni/V.
According to Equation 14.2.18, Kp = K only if the moles of gaseous products and gaseous reactants are the same (i.e., Δn = 0). For the decomposition of N2O4, there are 2 mol of gaseous product and 1 mol of gaseous reactant, so Δn = 1. Thus, for this reaction, Kp = K(RT)1 = KRT.
Example 14.2.4
The equilibrium constant for the reaction of nitrogen and hydrogen to give ammonia is 0.118 at 745 K. The balanced equilibrium equation is as follows:
\[N_{2(g)}+3H_{2(g)} \rightleftharpoons 2NH_{3(g)}\]
What is Kp for this reaction at the same temperature?
Given: equilibrium equation, equilibrium constant, and temperature
Asked for: K p
Strategy:
Use the coefficients in the balanced chemical equation to calculate Δn. Then use Equation 14.2.18 to calculate K from Kp.
Solution:
This reaction has 2 mol of gaseous product and 4 mol of gaseous reactants, so Δn = (2 − 4) = −2. We know K, and T = 745 K. Thus, from Equation 14.2.15, we have the following:
\[K_p=K(RT)^{−2}=\dfrac{K}{(RT)^2}=\dfrac{0.118}{\{ [0.08206(L \cdot atm)/(mol \cdot K)][745\; K]\}^2}=3.16 \times 10^{−5}\]
Because Kp is a unitless quantity, the answer is Kp = 3.16 × 10−5.
Exercise
Calculate Kp for the reaction
\[2SO_{2(g)}+O_{2(g)} \rightleftharpoons 2SO_{3(g)}\]
at 527°C, if K = 7.9 × 104 at this temperature.
Answer: Kp = 1.2 × 103
Homogeneous and Heterogeneous Equilibriums
When the products and reactants of an equilibrium reaction form a single phase, whether gas or liquid, the system is a homogeneous equilibriumAn equilibrium in which the reactants and products of an equilibrium reaction form a single phase, whether gas or liquid.. In such situations, the concentrations of the reactants and products can vary over a wide range. In contrast, a system whose reactants, products, or both are in more than one phase is a heterogeneous equilibriumAn equilibrium in which the reactants of an equilibrium reaction, the products, or both are in more than one phase., such as the reaction of a gas with a solid or liquid.
Because the molar concentrations of pure liquids and solids normally do not vary greatly with temperature, their concentrations are treated as constants, which allows us to simplify equilibrium constant expressions that involve pure solids or liquids.The reference states for pure solids and liquids are those forms stable at 1 bar (approximately 1 atm), which are assigned an activity of 1. (Recall from Chapter 7, for example, that the density of water, and thus its volume, changes by only a few percentage points between 0°C and 100°C.)
Consider the following reaction, which is used in the final firing of some types of pottery to produce brilliant metallic glazes:
\[CO_{2(g)}+C_{(s)} \rightleftharpoons 2CO_{(g)} \tag{14.2.19}\]
The glaze is created when metal oxides are reduced to metals by the product, carbon monoxide. The equilibrium constant expression for this reaction is as follows:
\[K=\dfrac{[CO]^2}{[CO_2][C]} \tag{14.2.20}\]
Because graphite is a solid, however, its molar concentration, determined from its density and molar mass, is essentially constant and has the following value:
\[ [C] =\dfrac{2.26 \cancel{g}/{\cancel{cm^3}}}{12.01\; \cancel{g}/mol} \times 1000 \; \cancel{cm^3}/L = 188 \; mol/L = 188\;M \tag{14.2.21}\]
We can rearrange Equation 14.2.20 so that the constant terms are on one side:
\[ K[C]=K(188)=\dfrac{[CO]^2}{[CO_2]} \tag{14.2.22}\]
Incorporating the constant value of [C] into the equilibrium equation for the reaction in Equation 14.2.19,
\[K'=\dfrac{[CO]^2}{[CO_2]} \tag{14.2.23}\]
The equilibrium constant for this reaction can also be written in terms of the partial pressures of the gases:
\[K_p=\dfrac{(P_{CO})^2}{P_{CO_2}} \tag{14.2.24}\]
Incorporating all the constant values into K′ or Kp allows us to focus on the substances whose concentrations change during the reaction.
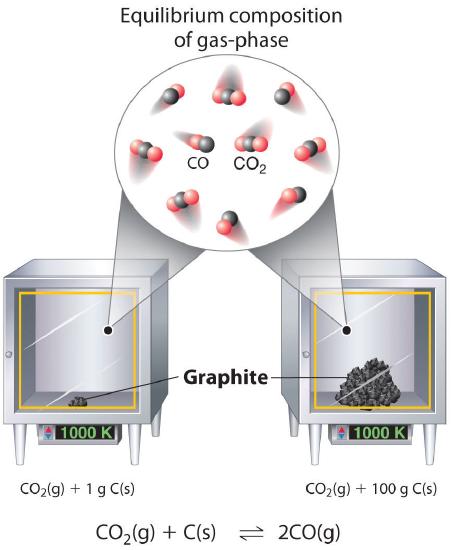
Although the concentrations of pure liquids or solids are not written explicitly in the equilibrium constant expression, these substances must be present in the reaction mixture for chemical equilibrium to occur. Whatever the concentrations of CO and CO2, the system described in Equation 14.2.19 will reach chemical equilibrium only if a stoichiometric amount of solid carbon or excess solid carbon has been added so that some is still present once the system has reached equilibrium. As shown in Figure 14.2.2, it does not matter whether 1 g or 100 g of solid carbon is present; in either case, the composition of the gaseous components of the system will be the same at equilibrium.
Figure 14.2.2 Effect of the Amount of Solid Present on Equilibrium in a Heterogeneous Solid–Gas System In the system, the equilibrium composition of the gas phase at a given temperature, 1000 K in this case, is the same whether a small amount of solid carbon (left) or a large amount (right) is present.
Example 14.2.5
Write each expression for K, incorporating all constants, and Kp for the following equilibrium reactions.
- \(PCl_{3(l)}+Cl_{2(g)} \rightleftharpoons PCl_{5(s)}\)
- \(Fe_3O_{4(s)}+4H_{2(g)} \rightleftharpoons 3Fe_{(s)}+4H_2O_{(g)}\)
Given: balanced equilibrium equations
Asked for: expressions for K and Kp
Strategy:
Find K by writing each equilibrium constant expression as the ratio of the concentrations of the products and reactants, each raised to its coefficient in the chemical equation. Then express Kp as the ratio of the partial pressures of the products and reactants, each also raised to its coefficient in the chemical equation.
Solution:
-
This reaction contains a pure solid (PCl5) and a pure liquid (PCl3). Their concentrations do not appear in the equilibrium constant expression because they do not change significantly. So
\[K=\dfrac{1}{[Cl_2]}\]and
\[K_p=\dfrac{1}{P_{Cl_2}}\]
-
This reaction contains two pure solids (Fe3O4 and Fe), which do not appear in the equilibrium constant expressions. The two gases do, however, appear in the expressions:
\[K=\dfrac{[H_2O]^4}{[H_2]^4}\]
Exercise
Write the expressions for K and Kp for the following reactions.
- \(CaCO_{3(s)} \rightleftharpoons CaO_{(s)}+CO_{2(g)}\)
- \( \underset{glucose}{C_6H_{12}O_{6(s)}} + 6O_{2(g)} \rightleftharpoons 6CO_{2(g)}+6H_2O_{(g)}\)
Answer:
- K = [CO2]; and Kp = P(CO2)
- \(K=\dfrac{[CO_2]^6[H_2O]^6}{[O_2]^6}\) and \(K_p=\dfrac{(P_{CO_2})^6(P_{H_2O})^6}{(P_{O_2})^6}\)
For reactions carried out in solution, the concentration of the solvent is omitted from the equilibrium constant expression even when the solvent appears in the balanced chemical equation for the reaction. The concentration of the solvent is also typically much greater than the concentration of the reactants or products (recall that pure water is about 55.5 M, and pure ethanol is about 17 M). Consequently, the solvent concentration is essentially constant during chemical reactions, and the solvent is therefore treated as a pure liquid. The equilibrium constant expression for a reaction contains only those species whose concentrations could change significantly during the reaction.
Note the Pattern
The concentrations of pure solids, pure liquids, and solvents are omitted from equilibrium constant expressions because they do not change significantly during reactions when enough is present to reach equilibrium.
Equilibrium Constant Expressions for the Sums of Reactions
Chemists frequently need to know the equilibrium constant for a reaction that has not been previously studied. In such cases, the desired reaction can often be written as the sum of other reactions for which the equilibrium constants are known. The equilibrium constant for the unknown reaction can then be calculated from the tabulated values for the other reactions.
To illustrate this procedure, let’s consider the reaction of N2 with O2 to give NO2. As we stated in Section 14.1, this reaction is an important source of the NO2 that gives urban smog its typical brown color. The reaction normally occurs in two distinct steps. In the first reaction (1), N2 reacts with O2 at the high temperatures inside an internal combustion engine to give NO. The released NO then reacts with additional O2 to give NO2 (2). The equilibrium constant for each reaction at 100°C is also given.
- \(N_{2(g)}+O_{2(g)} \rightleftharpoons 2NO_{(g)}\;\; K_1=2.0 \times 10^{−25}\)
- \(2NO_{(g)}+O_{2(g)} \rightleftharpoons 2NO_{2(g)}\;\;\;K_2=6.4 \times 10^9\)
-
Summing reactions (1) and (2) gives the overall reaction of N2 with O2:
- \(N_{2(g)}+2O_{2(g)} \rightleftharpoons 2NO_{2(g)} \;\;\;K_3=?\)
The equilibrium constant expressions for the reactions are as follows:
\[K_1=\dfrac{[NO]^2}{[N_2][O_2]}\;\;\; K_2=\dfrac{[NO_2]^2}{[NO]^2[O_2]}\;\;\; K_3=\dfrac{[NO_2]^2}{[N_2][O_2]^2}\]v
What is the relationship between K1, K2, and K3, all at 100°C? The expression for K1 has [NO]2 in the numerator, the expression for K2 has [NO]2 in the denominator, and [NO]2 does not appear in the expression for K3. Multiplying K1 by K2 and canceling the [NO]2 terms,
\[ K_1K_2=\dfrac{\cancel{[NO]^2}}{[N_2][O_2]} \times \dfrac{[NO_2]^2}{\cancel{[NO]^2}[O_2]}=\dfrac{[NO_2]^2}{[N_2][O_2]^2}=K_3\]
Thus the product of the equilibrium constant expressions for K1 and K2 is the same as the equilibrium constant expression for K3:
\[K_3 = K_1K_2 = (2.0 \times 10^{−25})(6.4 \times 10^9) = 1.3 \times 10^{−15}\]
The equilibrium constant for a reaction that is the sum of two or more reactions is equal to the product of the equilibrium constants for the individual reactions. In contrast, recall that according to Hess’s Law, ΔH for the sum of two or more reactions is the sum of the ΔH values for the individual reactions.
Note the Pattern
To determine K for a reaction that is the sum of two or more reactions, add the reactions but multiply the equilibrium constants.
Example 14.2.6
The following reactions occur at 1200°C:
- \(CO_{(g)}+3H_{2(g)} \rightleftharpoons CH_{4(g)}+H_2O_{(g)} \;\;\;K_1=9.17 \times 10^{−2}\)
- \(CH_{4(g)}+2H_2S_{(g)} \rightleftharpoons CS_{2(g)}+4H_{2(g})\;\;\; K_2=3.3 \times 10^4\)
Calculate the equilibrium constant for the following reaction at the same temperature.
- \(CO_{(g)}+2H_2S_{(g)} \rightleftharpoons CS_{2(g)}+H_2O_{(g)}+H_{2(g)}\;\;\; K_3=?\)
Given: two balanced equilibrium equations, values of K, and an equilibrium equation for the overall reaction
Asked for: equilibrium constant for the overall reaction
Strategy:
Arrange the equations so that their sum produces the overall equation. If an equation had to be reversed, invert the value of K for that equation. Calculate K for the overall equation by multiplying the equilibrium constants for the individual equations.
Solution:
The key to solving this problem is to recognize that reaction 3 is the sum of reactions 1 and 2:
\[CO_{(g)}+ \cancel{3H_{2(g)}} \rightleftharpoons \cancel{CH_{4(g)}} + H_2O_{(g)}\]
\[\cancel{CH_{4(g)}} +2H_2S_{(g)} \rightleftharpoons CS_{2(g)} + \cancel{3H_{2(g)}} + H_{2(g)}\]
\[ CO_{(g)} + 3H_{2(g)} \rightleftharpoons CS_{2(g)}+H_2O_{(g)}+H_{2(g)}\]
The values for K1 and K2 are given, so it is straightforward to calculate K3:
\[K_3 = K_1K_2 = (9.17 \times 10^{−2})(3.3 \times 10^4) = 3.03 \times 10^3\]
Exercise
In the first of two steps in the industrial synthesis of sulfuric acid, elemental sulfur reacts with oxygen to produce sulfur dioxide. In the second step, sulfur dioxide reacts with additional oxygen to form sulfur trioxide. The reaction for each step is shown, as is the value of the corresponding equilibrium constant at 25°C. Calculate the equilibrium constant for the overall reaction at this same temperature.
- \(\frac{1}{8}S_{8(s)}+O_{2(g)} \rightleftharpoons SO_{2(g)}\;\;\; K_1=4.4 \times 10^{53}\)
- \(SO_{2(g)}+\frac{1}{2}O_{2(g)} \rightleftharpoons SO_{3(g)}\;\;\; K_2=2.6 \times 10^{12}\)
- \(\frac{1}{8}S_{8(s)}+\frac{3}{2}O_{2(g)} \rightleftharpoons SO_{3(g)}\;\;\; K_3=?\)
Answer: K3 = 1.1 × 1066
Summary
The ratio of the rate constants for the forward and reverse reactions at equilibrium is the equilibrium constant (K), a unitless quantity. The composition of the equilibrium mixture is therefore determined by the magnitudes of the forward and reverse rate constants at equilibrium. Under a given set of conditions, a reaction will always have the same K. For a system at equilibrium, the law of mass action relates K to the ratio of the equilibrium concentrations of the products to the concentrations of the reactants raised to their respective powers to match the coefficients in the equilibrium equation. The ratio is called the equilibrium constant expression. When a reaction is written in the reverse direction, K and the equilibrium constant expression are inverted. For gases, the equilibrium constant expression can be written as the ratio of the partial pressures of the products to the partial pressures of the reactants, each raised to a power matching its coefficient in the chemical equation. An equilibrium constant calculated from partial pressures (Kp) is related to K by the ideal gas constant (R), the temperature (T), and the change in the number of moles of gas during the reaction. An equilibrium system that contains products and reactants in a single phase is a homogeneous equilibrium; a system whose reactants, products, or both are in more than one phase is a heterogeneous equilibrium. When a reaction can be expressed as the sum of two or more reactions, its equilibrium constant is equal to the product of the equilibrium constants for the individual reactions.
Key Takeaways
- The law of mass action describes a system at equilibrium in terms of the concentrations of the products and the reactants.
- For a system involving one or more gases, either the molar concentrations of the gases or their partial pressures can be used.
Key Equations
Definition of equilibrium constant in terms of forward and reverse rate constants
Equation 14.2.5: \( K=\dfrac{k_f}{k_r} \)
Equilibrium constant expression (law of mass action)
Equation 14.2.7: \( K=\dfrac{\left [ C \right ]^c \left [ D \right ]^d}{\left [ A \right ]^a \left [ B \right ]^b} \)
Equilibrium constant expression for reactions involving gases using partial pressures
Equation 14.2.16: \( K_p=\dfrac{P_C^c P_D^d}{P_A^aP_B^b} \)
Relationship between K p and K
Equation 14.2.18: \( K_p=K\left ( RT \right )^{\Delta n} \)
Conceptual Problems
-
For an equilibrium reaction, what effect does reversing the reactants and products have on the value of the equilibrium constant?
-
Which of the following equilibriums are homogeneous and which are heterogeneous?
- \(2HF_{(g)} \rightleftharpoons H_{2(g)}+F_{2(g)}\)
- \(C_{(s)} + 2H_{2(g)} \rightleftharpoons CH_{4(g)}\)
- \(\ce{H_2C=CH_{2(g)}} + H_{2(g)} \rightleftharpoons C_2H_{6(g)}\)
- \(2Hg_{(l)} + O_{2(g)} \rightleftharpoons 2HgO_{(s)}\)
-
Classify each equilibrium system as either homogeneous or heterogeneous.
- \(NH_4CO_2NH_{2(s)} \rightleftharpoons 2NH_{3(g)}+CO_{2(g)}\)
- \(C_{(s)} + O_{2(g)} \rightleftharpoons CO_{2(g)}\)
- \(2Mg_{(s)} + O_{2(g)} \rightleftharpoons 2MgO_{(s)}\)
- \(AgCl_{(s)} \rightleftharpoons Ag^+_{(aq)}+Cl^−_{(aq)}\)
-
If an equilibrium reaction is endothermic, what happens to the equilibrium constant if the temperature of the reaction is increased? if the temperature is decreased?
-
Industrial production of NO by the reaction <math xml:id="av_1.0-15_m114" display="inline"><semantics><mrow><msub><mtext>N</mtext><mn>2</mn></msub><mtext>(g)</mtext><mo>+</mo><msub><mtext>O</mtext><mn>2</mn></msub><mtext>(g)</mtext><mo>⇌</mo><mtext>2NO(g)</mtext></mrow></semantics></math> is carried out at elevated temperatures to drive the reaction toward the formation of product. After sufficient product has formed, the reaction mixture is quickly cooled. Why?
-
How would you differentiate between a system that has reached chemical equilibrium and one that is reacting so slowly that changes in concentration are difficult to observe?
-
What is the relationship between the equilibrium constant, the concentration of each component of the system, and the rate constants for the forward and reverse reactions?
-
Write the equilibrium constant expressions for K and Kp for each reaction.
- \(CO_{(g)} + H_2O_{(g)} \rightleftharpoons CO_{2(g)}+H_{2(g)}\)
- \(PCl_{3(g)}+Cl_{2(g)} \rightleftharpoons PCl_{5(g)}\)
- \(2O_{3(g)} \rightleftharpoons 3O_{2(g)}\)
-
Write the equilibrium constant expressions for K and Kp as appropriate for each reaction.
- \(2NO_{(g)}+O_{2(g)} \rightleftharpoons 2NO_{2(g)}\)
- \(\frac{1}{2}H_{2(g)}+12I_{2(g)} \rightleftharpoons HI_{(g)}\)
- \(cis-stilbene_{(soln)} \rightleftharpoons trans-stilbene_{(soln)}\)
-
Why is it incorrect to state that pure liquids, pure solids, and solvents are not part of an equilibrium constant expression?
-
Write the equilibrium constant expressions for K and Kp for each equilibrium reaction.
- \(2S_{(s)}+3O_{2(g)} \rightleftharpoons 2SO_{3(g)}\)
- \(C_{(s)} + CO_{2(g)} \rightleftharpoons 2CO_{(g)}\)
- \(2ZnS_{(s)}+3O_{2(g)} \rightleftharpoons 2ZnO_{(s)}+2SO_{2(g)}\)
-
Write the equilibrium constant expressions for K and Kp for each equilibrium reaction.
- \(2HgO_{(s)} \rightleftharpoons 2Hg_{(l)}+O_{2(g)}\)
- \(H_{2(g)}+I_{2(s)} \rightleftharpoons 2HI_{(g)}\)
- \(NH_4CO_2NH_{2(s)} \rightleftharpoons 2NH_{3(g)}+CO_{2(g)}\)
-
At room temperature, the equilibrium constant for the reaction <math xml:id="av_1.0-15_m134" display="inline"><semantics><mrow><mn>2</mn><mtext>A(g)</mtext><mo>⇌</mo><mtext>B(g)</mtext></mrow></semantics></math> is 1. What does this indicate about the concentrations of A and B at equilibrium? Would you expect K and Kp to vary significantly from each other? If so, how would their difference be affected by temperature?
-
For a certain series of reactions, if [OH−][HCO3−]/[CO32−] = K1 and [OH−][H2CO3]/[HCO3−] = K2, what is the equilibrium constant expression for the overall reaction? Write the overall equilibrium equation.
-
In the equation for an enzymatic reaction, ES represents the complex formed between the substrate S and the enzyme protein E. In the final step of the following oxidation reaction, the product P dissociates from the ESO2 complex, which regenerates the active enzyme:
\[E + S \rightleftharpoons ES\;\;\; K_1\]\[ES + O_2 \rightleftharpoons ESO_2 \;\;\; K_2\]\[ESO_2 \rightleftharpoons E+P\;\;\;\; K_3\]Give the overall reaction equation and show that K = K1 × K2 × K3.
Answers
-
The equilibrium constant for the reaction written in reverse: K′ = 1/K.
-
Each system is heterogeneous.
-
Rapid cooling “quenches” the reaction mixture and prevents the system from reverting to the low-temperature equilibrium composition that favors the reactants.
-
\(K=\dfrac{k_f}{k_r}\;\;\; K=\dfrac{[C]^c[D]^d}{[A]^a[B]^b}\])
-
- \(K=\dfrac{[NO_2]^2}{[NO]^2[O_2]}\;\;\; K_p=\dfrac{(P_{N_2O})^2}{(P_{NO})^2(P_{O_2}})\)
- \(K=\dfrac{[HI]}{[H_2]^{1/2}[I_2]^{1/2}}\;\;\; K_p=\dfrac{P_{HI}}{(P_{H_2})^{1/2}(P_{I_2})}\)
- \(K=\dfrac{[\text{trans-stilbene}]}{[\text{cis-stilbene}]}\)
-
- \(K=\dfrac{[SO_3]^2}{[O_2]^3}\;\;\; K_p=\dfrac{(P_{SO_3})^2}{(P_{O_2})^3}\)
- \(K=\dfrac{[CO]^2}{[CO_2]}\;\;\; K_p=\dfrac{(P_{CO})^2}{P_{CO_2}}\)
- \(K=\dfrac{[SO_2]^2}{[O_2]^3}\;\;\; K_p=\dfrac{(P_{SO_2})^2}{(P_{O_2})^3}\)
-
At equilibrium,
\([A]=\sqrt{B}\) and \(Δn =−1\) so \(K_p=K(RT)^{Δn}=\dfrac{K}{RT}\)
the difference increases as T increases.
Numerical Problems
-
Explain what each of the following values for K tells you about the relative concentrations of the reactants versus the products in a given equilibrium reaction: K = 0.892; K = 3.25 × 108; K = 5.26 × 10−11. Are products or reactants favored at equilibrium?
-
Write the equilibrium constant expression for each reaction. Are these equilibrium constant expressions equivalent? Explain.
- \(N_2O_{4(g)} \rightleftharpoons 2NO_{2(g)}\)
- \(\frac{1}{2}N_2O_{4(g)} \rightleftharpoons NO_{2(g)}\)
-
Write the equilibrium constant expression for each reaction.
- \(\frac{1}{2}N_{2(g)}+\frac{3}{2}H_{2(g)} \rightleftharpoons NH_{3(g)}\)
- \(\frac{1}{3}N_{2(g)}+H_{2(g)} \rightleftharpoons \frac{2}{3}NH_{3(g)}\)
How are these two expressions mathematically related to the equilibrium constant expression for
\[N_{2(g)}+3H_{2(g)} \rightleftharpoons 2NH_{3(g)} ?\]
-
Write an equilibrium constant expression for each reaction.
- \(C_{(s)} + 2H_2O_{(g)}⇌CO_{2(g)}+2H_{2(g)}\)
- \(SbCl_{3(g)}+Cl_{2(g)}⇌SbCl_{5(g)}\)
- \(2O_{3(g)}⇌3O_{2(g)}\)
-
Give an equilibrium constant expression for each reaction.
- \(2NO_{(g)} + O_{2(g)} \rightleftharpoons 2NO_{2(g)}\)
- \(\frac{1}{2}H_{2(g)}+\frac{1}{2}I_{2(g)}⇌HI_{(g)}\)
- \(CaCO_{3(s)} + 2HOCl_{(aq)} \rightleftharpoons Ca^{2+}_{(aq)} + 2OCl^−_{(aq)} + H_2O_{(l)} + CO_{2(g)}\)
-
Calculate K and Kp for each reaction.
- \(2NOBr_{(g)}⇌2NO_{(g)}+Br_{(g)}\) at 727°C, the equilibrium concentration of NO is 1.29 M, Br2 is 10.52 M, and NOBr is 0.423 M.
- \(C_{(s)} + CO_{2(g)}⇌2CO_{(g)}\): at 1200 K, a 2.00 L vessel at equilibrium has partial pressures of 93.5 atm CO2 and 76.8 atm CO, and the vessel contains 3.55 g of carbon.
-
Calculate K and Kp for each reaction.
- \(N_2O4_{(g)}⇌2NO_{2(g)}\): at the equilibrium temperature of −40°C, a 0.150 M sample of N2O4 undergoes a decomposition of 0.456%.
- \(CO_{(g)}+2H_{2(g)}⇌CH_3OH_{(g)}\): an equilibrium is reached at 227°C in a 15.5 L reaction vessel with a total pressure of 6.71 × 102 atm. It is found to contain 37.8 g of hydrogen gas, 457.7 g of carbon monoxide, and 7193 g of methanol.
-
Determine K and Kp (where applicable) for each reaction.
- \(2H_2S_{(g)}⇌2H_{2(g)}+S_{2(g)}\): at 1065°C, an equilibrium mixture consists of \(1.00 \times 10^{−3}\) M \(H_2\), \(1.20 \times 10^{−3}\) M \(S_2\), and \(3.32 \times 10^{−3}\) M \(H_2S\).
- \(Ba(OH)_{2(s)}⇌2OH^−_{(aq)}+Ba^{2+}_{(aq)}\): at 25°C, a 250 mL beaker contains 0.330 mol of barium hydroxide in equilibrium with 0.0267 mol of barium ions and 0.0534 mol of hydroxide ions.
-
Determine K and Kp for each reaction.
- \(2NOCl_{(g)}⇌2NO_{(g)}+Cl_{2(g)}\): at 500 K, a 24.3 mM sample of \(NOCl\) has decomposed, leaving an equilibrium mixture that contains 72.7% of the original amount of \(NOCl\).
- \(Cl_{2(g)}+PCl_{3(g)}⇌PCl_{5(g)}\): at 250°C, a 500 mL reaction vessel contains 16.9 g of \(Cl_2\) gas, 0.500 g of \(PCl_3\), and 10.2 g of \(PCl_5\) at equilibrium.
-
The equilibrium constant expression for a reaction is [CO2]2/[SO2]2[O2]. What is the balanced chemical equation for the overall reaction if one of the reactants is Na2CO3(s)?
-
The equilibrium constant expression for a reaction is [NO][H2O]3/2/[NH3][O2]5/4. What is the balanced chemical equation for the overall reaction?
-
Given K = kf/kr, what happens to the magnitude of the equilibrium constant if the reaction rate of the forward reaction is doubled? What happens if the reaction rate of the reverse reaction for the overall reaction is decreased by a factor of 3?
-
The value of the equilibrium constant for
\[ 2H_{2(g)}+S_{2(g)}⇌2H_2S_{(g)}\]
is 1.08 × 107 at 700°C. What is the value of the equilibrium constant for the following related reactions?
- \(H_{2(g)}+12S_{2(g)}⇌H_2S_{(g)}\)
- \(4H_{2(g)}+2S_{2(g)}⇌4H_2S_{(g)}\)
- \(H_2S_{(g)}⇌H_{2(g)}+12S_{2(g)}\)
Answers
-
K = 0.892: the concentrations of the products and the reactants are approximately equal at equilibrium so neither is favored; K = 3.25 × 108: the ratio of the concentration of the products to the reactants at equilibrium is very large so the formation of products is favored; K = 5.26 × 10−11: the ratio of the concentration of the products to the reactants at equilibrium is very small so the formation of products is not favored.
-
- \(K'=\dfrac{[NH_3]}{[N_2]^{1/2}[H_2]^{3/2}}\)
- \(K''=\dfrac{[NH_3]^{2/3}}{[N_2]^{1/3}[H_2]}\;\;\; K=\dfrac{[NH_3]^2}{[N_2][H_2]^3}\;\;\; K′ = K^{1/2},\text{ and} K″ = K^{1/3}\)
-
- \(K=\dfrac{[NO_2]^2}{[NO]^2[O_2]}\)
- \(K=\dfrac{[HI]}{[H_2]^{1/2}[I_2]^{1/2}}\)
- \(K=\dfrac{[Ca^{2+}][OCl^−]^2[P_{CO_2}]}{[HOCl]^2}\)
-
- \(K = 1.25 \times 10^{−5}\;\;\; K_p = 2.39 \times 10^{−4}\)
- \(K = 9.43\;\;\; K_p = 5.60 \times 10^{−3}\)
-
- \(K=\dfrac{[Cl_2][NO]^2}{[NOCl]^2}=4.59 \times 10^{−4}\;\;\; K_p=1.88 \times 10^{−2}\)
- \(K=\dfrac{[PCl_5]}{[PCl_3][Cl_2]}=28.3\;\;\; K_p=0.658\)
-
\(NH_3 + \frac{5}{4}O_2⇌NO +\frac{3}{2}H_2O\), which can also be written as follows: \[4NH_{3(g)} + 5O_{2(g)}⇌4NO_{(g)} + 6H_2O_{(g)}\]
-
- 3.29 × 103
- 1.17 × 1014
- 3.04 × 10−4
Contributors
- Anonymous
Modified by Joshua Halpern (Howard University), Scott Sinex, and Scott Johnson (PGCC)