16.3: The Ultraviolet Catastrophe

Last updated
Save as PDF

Page ID: 416075

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

The ultraviolet (UV) catastrophe, also called the Rayleigh–Jeans catastrophe, is the prediction of classical electromagnetism that the intensity of the radiation emitted by an ideal black body at thermal equilibrium goes to infinity as wavelength decreases (see figure \(\PageIndex{1}\) )\(^1\).

Figure \(\PageIndex{1}\): The ultraviolet catastrophe is the error at short wavelengths in the Rayleigh–Jeans law for the energy emitted by an ideal black body. The error, much more pronounced for short wavelengths, is the difference between the Rayleigh–Jeans law —black—and Planck’s law—blue.

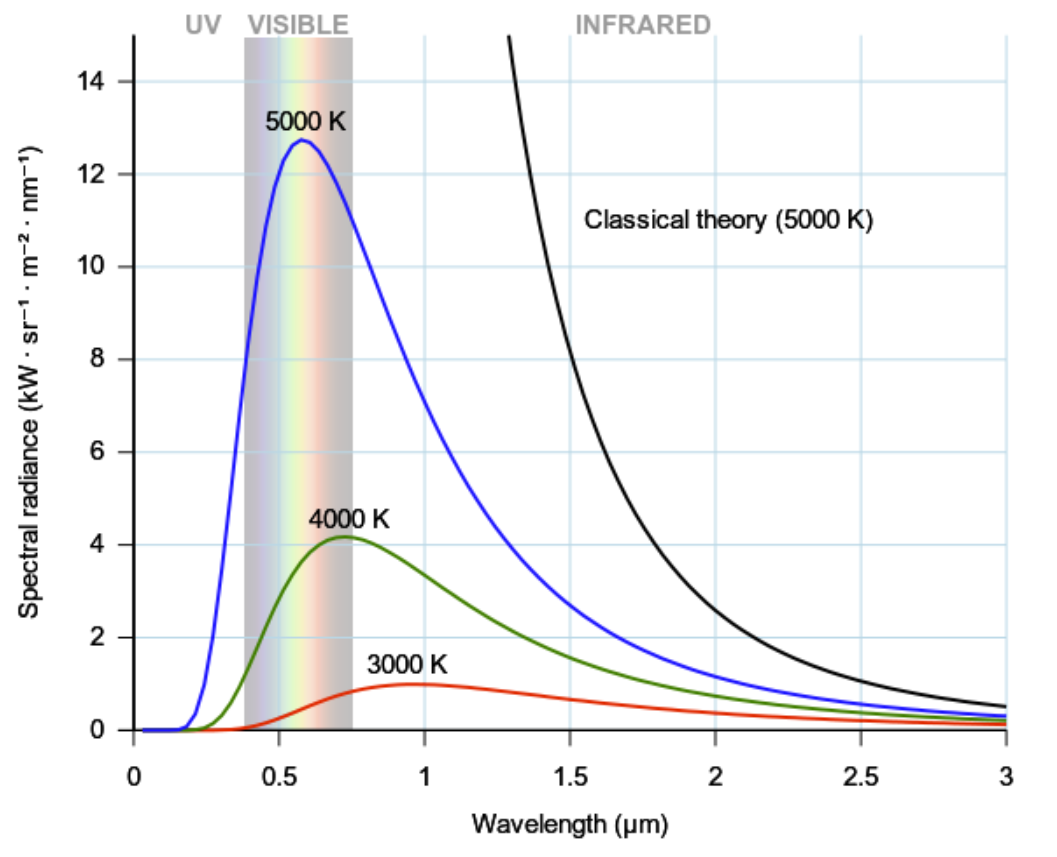
A black body is an idealized object that absorbs and emits all frequencies. Classical physics can be used to derive an approximated equation describing the intensity of a black body radiation as a function of frequency for a fixed temperature. The result is known as the Rayleigh-Jeans law, which for wavelength \(\lambda\), is:

\[ B_{\lambda }(T)={\dfrac {2ck_{\mathrm {B} }T}{\lambda ^{4}}} \label{17.3.1} \]

where \(B_{\lambda }\) is the intensity of the radiation —expressed as the power emitted per unit emitting area, per steradian, per unit wavelength (spectral radiance)— \(c\) is the speed of light, \(k_{\mathrm{B}}\) is the Boltzmann constant, and \(T\) is the temperature in kelvins. The paradox —or rather the breakdown of the Rayleigh–Jeans formula— happens at small wavelength \(\lambda\). If we take the limit for \(\lambda \rightarrow 0\) in Equation \ref{17.3.1}, we obtain that \(B_{\lambda } \rightarrow \infty\). In other words, as the wavelength of the emitted light gets smaller (approaching the UV range), the intensity of the radiation approaches infinity, and the black body emits an infinite amount of energy. This divergence for low wavelength (high frequencies) is called the ultraviolet catastrophe, and it is clearly unphysical.

Max Planck explained the black body radiation in 1900 by assuming that the energies of the oscillations of the electrons responsible for the radiation must be proportional to integral multiples of the frequency, i.e.,

\[ E = n h \nu = n h \dfrac{c}{\lambda} \label{17.3.2} \]

Planck’s assumptions led to the correct form of the spectral function for a black body: \[ B_{\lambda }(\lambda ,T)={\dfrac {2hc^{2}}{\lambda ^{5}}}{\dfrac {1}{e^{hc/(\lambda k_{\mathrm {B} }T)}-1}}. \label{17.3.3} \]

If we now take the limit for \(\lambda \rightarrow 0\) of Equation \ref{17.3.3}, it is easy to prove that \(B_{\lambda }\) goes to zero, in agreement with the experimental results, and our intuition. Planck also found that for \(h = 6.626 \times 10^{-34} \; \text{J s}\), the experimental data could be reproduced exactly. Nevertheless, Planck could not offer a good justification for his assumption of energy quantization. Physicists did not take this energy quantization idea seriously until Einstein invoked a similar assumption to explain the photoelectric effect.

This picture is taken from Wikipedia by user Darth Kule, and in in the Public Domain