Intermolecular Forces in Mixtures And Solutions
- Page ID
- 1622
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Some forces that interact within pure liquids are also present during mixtures and solutions. Forces such as Cohesive as well as Adhesive forces still apply to mixtures; however, more importantly we focus on the interaction between different molecules. Why is oil only soluble in benzene and not water? Why do only "like" molecules dissolve in "like" molecules?
The process of Mixing
Before we go on to the more specific mechanisms of mixing, let's discuss its process. Mixing is a spontaneous process that increases the entropy of the solution. In order to form a mixture of homogenous solutions by distributing the solute molecules evenly within the solvent molecules, heat transfers are inevitable. This heat transfer is denoted ΔHsoln for our general comprehension. ΔH is the change in heat energy found by subtracting the enthalpy of the reactant from that of the product:
\[H_{products} - H_{reactants}= ΔH_{soln}.\]
Enthalpy of Solution
What then is the significance of \(ΔH_{soln}\)? It presents a clear indication of the magnitude as well as direction of the heat transfer so that when:
- ΔH>0 : Endothermic Reaction (positive), because the products encompass more energy than the reactants
- ΔH<0 : Exothermic Reaction (negative), because the reactants consist of more energy than the products.
What we have to supply for our understanding for this equation is that the extra energy is seized either from or give to the surrounding. And to ascertain the enthalpy of solution, we take the three step approach in enthalpy when a solute is mixed with the solvent.
Three Step Approach to Finding the Enthalpy of Solution: ΔHsoln = ΔH1 + ΔH2+ ΔH3
- Each molecule of solute is Separated from each other (expand the solute), endothermic reaction. (ΔH1)
- Each molecule of solvent is separated from each other (expand solvent), endothermic reaction. (ΔH2) Now the molecules of solute and molecules of solvent can be permitted to attract one another in solution.
- The molecules of solute and solvent react with each other and a solution will result. exothermic reaction (ΔH3)
note that usually \(ΔH_1\) and \(ΔH_2\) are opposite in sign as \(ΔH_3\). Separating the solute and the solvent solutions alone are usually endothermic reactions in that their cohesive forces are broken while letting the molecules to react freely is an exothermic reaction. The figure below explains pictorially how positive and negative \(ΔH\) can be obtained through the three step process of mixing solution.
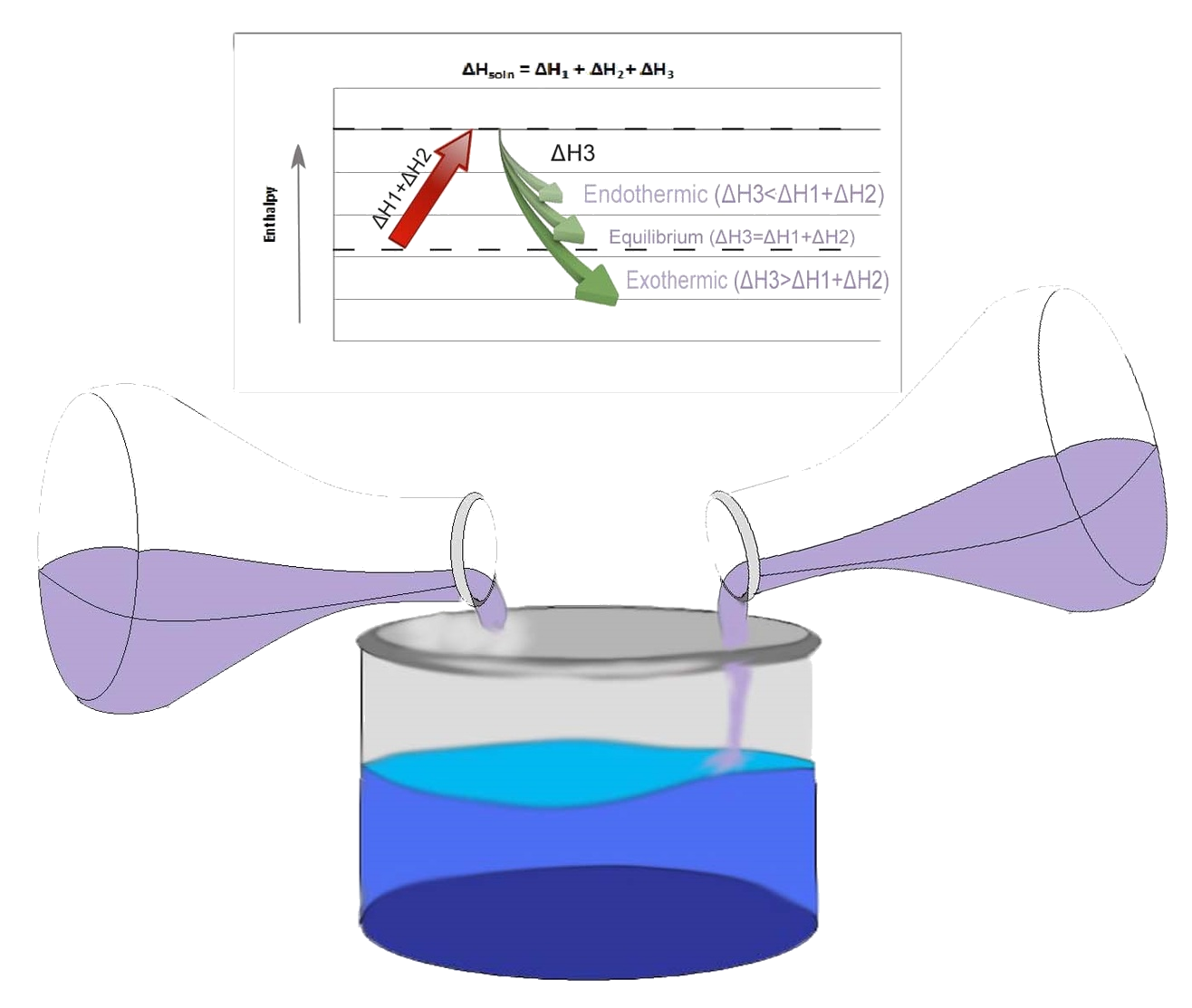
ΔH's Relationship to the Behavior of the Solution
Ideal solution is the mixture that has little to no net intermolecular interactions that differentiates it from its ideal behavior. Thus if the intermolecular forces of attraction are the same and have the same strength, both the solvent and solute will mix at random. This solution is called an ideal solution, which means that \(ΔH_{soln} = 0\). If the intermolecular forces of attraction of different molecules are greater than the forces of attraction of like molecules, then it is called a nonideal solution. This will result in an exothermic process (\(ΔH_{soln}<0\)). If the intermolecular forces of attraction of different molecules are a bit weaker than the forces of attraction of like molecules. This solution is a nonideal solution, has bigger enthalpy value than pure components, and it goes through an endothermic process. Lastly, if the intermolecular forces of attraction of different molecules is a lot weaker than the forces of attraction of like molecules, the solution becomes a heterogeneous mixture (e.g., water and olive oil).
The Effects of Intermolecular Forces in Solution.
The epitome of intermolecular forces in solution is the miracle of solubility, because when a matter precipitates it no longer interacts with the solvent. So what is the attraction between "like" molecules that makes them attract to each other? Let's take a phospholipid, the building block of a cell's membrane, as an example. This molecule is amphipathic, meaning that it is both hydrophilic and hydrophobic. Beginning with the structure of a phospholipid, it has a polar head which is hydrophilic and a nonpolar tail which is hydrophobic as the picture below.
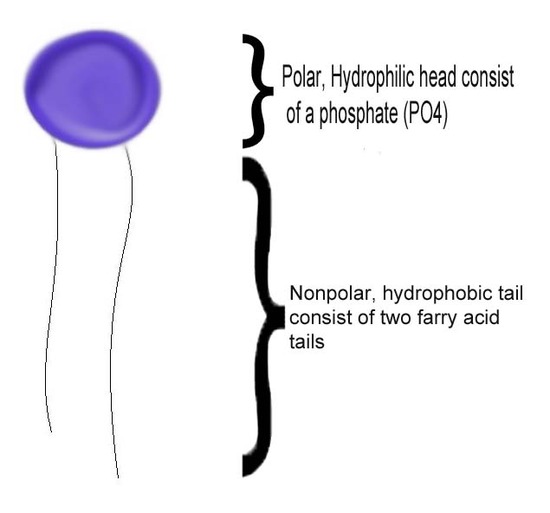
How can a single molecule be both polar molecule loving and polar molecule disliking at the same time? This is because at the polar head, the phosphate has a net negative charge thus attracting the partial positive charge of the hydrogen molecules of water. Its nonpolar tails on the other hand, is a very organized form of hydrocarbon, consisting of no net charges. The tail is then repelled by water as it struggles to fit between the partial positive and partial negative of the water molecule.
Another side effect of the interactions of molecules is reflected by the use of the activity coefficient during thermodynamic equilibrium constant calculations. This constant differentiates ideal and nonideal solutions so that interactions for solution equilibrium can be more accurately estimated. Most versions of the equilibrium constant K utilizes activity instead of concentration so that the units would disappear more fluently. For an ideal solution, the activity coefficient is 1 [x]/ oCelcius, thus when the concentration is dived by it to yield activity, it is unaltered.
Based on the concept of intermolecular interactions, ascertain the reason behind freezing-point depression and boiling-point elevation.
Solution
When an ion is added into solution, it exerts an intermolecular force which binds loosely to the water molecules in solution. This weak force then increases the energy necessary to break each molecule loose, thus increasing temperature in relationship to vapor pressure. It now takes more energy input to obtain the same vapor pressure, thus elevating the boiling point. For freezing-point depression, the same force that is holding the water molecules from evaporating is holding them against being placed into an organized solid form. It now takes more energy to form the weak bonds between each water molecule because the intermolecular forces between water and the ions first have to be overcome, hence reducing the freezing point.
Give examples that present the involvement of intermolecular forces thus differentiating ideal from nonideal solutions.
Solution
Gibbs free energy (relating ΔG with ΔGo), calculating thermodynamic equilibrium constants, boiling-point elevation, and freezing-point depression
References
- Petrucci, Harwood, Herring. General Chemistry: Principles & Modern Applications. 8th ed. Upper Saddle River, New Jersey: Pearson/Prentice Hall, 2002.
- Zumdahl, Steven S. Chemical Principles. 4th ed. Boston: Houghton Mifflin Company, 2002. pg 812

