1.1: An Approach to Quantum Mechanics
- Page ID
- 5131
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The purpose of this tutorial is to introduce the basics of quantum mechanics using Dirac bracket notation while working in one dimension. Dirac notation is a succinct and powerful language for expressing quantum mechanical principles; restricting attention to one-dimensional examples reduces the possibility that mathematical complexity will stand in the way of understanding. A number of texts make extensive use of Dirac notation (1-5).
Wave-particle duality is the essential concept of quantum mechanics. DeBroglie expressed this idea mathematically as \(\lambda = h/m \nu = h/p\). On the left is the wave property \(\lambda\), and on the right the particle property m\(\nu\), momentum. The most general coordinate space wavefunction for a free particle with wavelength \(\lambda\) is the complex Euler function shown below.
\[\langle x | \lambda \rangle = \exp \left(i2 \pi \frac{x}{\lambda}\right) = \cos \left(2 \pi \frac{x}{\lambda}\right)+i \sin \left(2 \pi \frac{x}{\lambda}\right) \label{Equation 1} \]
Feynman called this equation “the most remarkable formula in mathematics.” He referred to it as “our jewel.” A nd indeed it is, because when it is enriched with de Broglie’s relation it serves as the foundation of quantum mechanics.
According to de Broglie=s hypothesis, a particle with a well-defined wavelength also has a well-defined momentum. Therefore, we can obtain the momentum wavefunction (unnormalized) of the particle in coordinate space by substituting the deBroglie relation into Equation \ref{Equation 1}.
\[\langle x | p \rangle = \exp \left(\dfrac{ipx}{\hbar}\right) \label{Equation 2} \]
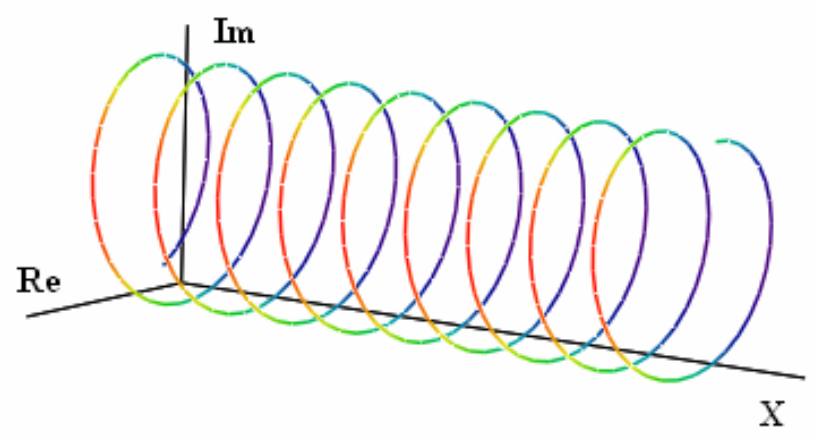
When Equation \ref{Equation 2} is graphed it creates a helix about the axis of propagation (X-axis). Z is the imaginary axis and Y is the real axis. It is the simplest example of a fourier transform, translating momentum into coordinate language. It also has in it the heart of the uncertainty principle.
Everyday examples of this important mathematical formula include telephone cords, spiral notebooks and slinkies.
Quantum mechanics teaches that the wavefunction contains all the physical information about a system that can be known, and that one extracts information from the wavefunction using quantum mechanical operators. There is, therefore, an operator for each observable property.
For example, in momentum space if a particle has a well-defined momentum we write its state as \(|p \rangle\). If we operate on this state with the momentum operator \(\hat{p}\), the following eigenvalue equation is satisfied.
\[\hat{p} |p \rangle = p|p \rangle \label{Equation 3} \]
We say the system is in a state which is an eigenfunction of the momentum operator with eigenvalue p. In other words, operating on the momentum eigenfunction with the momentum operator, in momentum space, returns the momentum eigenvalue times the original momentum eigenfunction. From
\[ \langle p | \hat{p} |p \rangle = p \langle p|p \rangle \label{Equation 4} \]
it follows that,
\[ \langle p | \hat{p} = p \langle p| \label{Equation 5} \]
Equations (3) and (5) show that in its own space the momentum operator is a multiplicative operator, and can operate either to the right on a ket, or to the left on a bra. The same is true of the position operator in coordinate space.
To obtain the momentum operator in coordinate space, equation (3) is projected onto coordinate space by operating on the left with \(\langle x | \). After inserting equation (2) we have,
\[ \langle x | \hat{p} |p \rangle = p \langle x|p \rangle = p \exp \left(\dfrac{ipx}{h}\right) = \frac{\hbar}{i} \frac{d}{dx} \exp \left(\dfrac{ipx}{\hbar}\right) = \frac{\hbar}{i} \frac{d}{dx} \langle x | p \rangle \label{Equation 6} \]
Comparing the first and last terms reveals that
\[ \langle x | \hat{p} = \frac{\hbar}{i} \frac{d}{dx} \langle x | \label{Equation 7} \]
and that \(\frac{\hbar}{i} \frac{d}{dx} \langle x | \) is the momentum operator in coordinate space.
The position wavefunction in momentum space is the complex conjugate of the momentum wavefunction coordinate space.
\[ \langle p|x \rangle = \langle x|p \rangle^{*} = \exp \left(\dfrac{-ipx}{\hbar}\right) \label{Equation 8} \]
Starting with the coordinate-space eigenvalue equation
\[ \hat{x} |x \rangle = x|x \rangle \label{Equation 9} \]
and using the same approach as with momentum, it is easy to show that
\[ \langle x| \hat{x} = x \langle x| \label{Equation 10} \]
\[ \langle p| \hat{x} = - \frac{\hbar}{i} \frac{d}{dp} \langle p| \label{Equation 11} \]
In summary, the two fundamental dynamical operators are position and momentum, and the two primary representations are coordinate space and momentum space. The results achieved thus far are shown in the following table.
| Coordinate Space | Momentum Space | |
|---|---|---|
| position operator: \(\hat{x}\) | \( x \langle x|\) | \( - \frac{\hbar}{i} \frac{d}{dp} \langle p | \) |
| momentum operator: \(\hat{p}\) | \( \frac{\hbar}{i} \frac{d}{dx} \langle x |\) | \( p \langle p| \) |
Other quantum mechanical operators can be constructed from \(\hat{x}\) and \(\hat{p}\) in the appropriate representation, position or momentum. To illustrate this, Schrödinger's equation for the one-dimensional harmonic oscillator will be set up in both coordinate and momentum space using the information in the table. Schrödinger's equation is the quantum mechanical energy eigenvalue equation, and for the harmonic oscillator it looks like this initially,
the information in the table. Schrödinger's equation is the quantum mechanical energy eigenvalue equation, and for the harmonic oscillator it looks like this initially,
\[\bigg[ \frac{\hat{p}^{2}}{2m} + \frac{1}{2} k \hat{x} \bigg] | \Psi \rangle = E | \Psi \rangle \label{Equation 12} \]
The term in brackets on the left is the classical energy written as an operator without a commitment to a representation (position or momentum) for the calculation.
Most often, chemists solve Schrödinger's equation in coordinate space. Therefore, to prepare Schrödinger's equation for solving, equation (12) is projected onto coordinate space by operating on the left with \(\langle x |\).
\[\langle x | \bigg[ \frac{\hat{p}^{2}}{2m} + \frac{1}{2} k \hat{x} \bigg] | \Psi \rangle = \langle x | E | \Psi \rangle \label{Equation 13} \]
Using the information in the table this yields,
\[\bigg[ - \frac{\hbar^{2}}{2m} \frac{d^{2}}{dx^{2}} + \frac{1}{2} kx^{2} \bigg] \langle x| \Psi \rangle = E \langle x| \Psi \rangle \label{Equation 14} \]
The square bracket on the left contains the quantum mechanical energy operator in coordinate space. Before proceeding we illustrate how the kinetic energy operator emerges as a differential operator in coordinate space using equation (7).
\[\frac{1}{2m} \langle x| \hat{p} \hat{p} | \Psi \rangle = \frac{1}{2m} \frac{\hbar}{i} \frac{d}{dx} \langle x| \hat{p} | \Psi \rangle = \frac{1}{2m} \frac{\hbar}{i} \frac{d}{dx} \frac{\hbar}{i} \frac{d}{dx} \langle x| \Psi \rangle = - \frac{hbar^{2}}{2m} \frac{d^{2}}{dx^{2}} \langle x | \Psi \rangle \label{Equation 15} \]
Equation (10) is used in a similar fashion to show that potential energy is a multiplicative operator in coordinate space.
\[ \frac{1}{2} k \langle x| \hat{x} \hat{x} | \Psi \rangle = \frac{1}{2} kx \langle x| \hat{x} | \Psi \rangle = \frac{1}{2} kx^{2} \langle x| \Psi \rangle \label{Equation 16} \]
Obviously the calculation could also have been set up in momentum space. It is easy to show that in the momentum representation Schrödinger=s equation is
\[ \bigg[ \frac{p^{2}}{2m} - \frac{\hbar^{2} k}{2} \frac{d^{2}}{dp^{2}} \bigg] \langle p | \Psi \rangle = E \langle p | \Psi \rangle \label{Equation 17} \]
In momentum space the kinetic energy operator is multiplicative and the potential energy operator is differential. The one-dimensional simple harmonic oscillator problem is exactly soluble in both coordinate and momentum space. The solution can be found in any chemistry and physics text dealing with quantum mechanics, and will not be dealt with further here, other than to say that equations (14) and (17) reveal an appealing symmetry.
Unfortunately, for most applications the potential energy term in momentum space presents more of a mathematical challenge than it does for the harmonic oscillator problem. A general expression for the potential energy in the momentum representation when its form in the coordinate representation is specified is given below.
\[\langle p | \hat{V} | Psi \rangle = \int \int \exp \bigg[ \frac{i(p'-p)x}{\hbar} \bigg] V(x) \langle p' | \Psi \rangle dp' dx \label{Equation 18} \]
To see how this integral is handled for a specific case see reference (10).
If a system is in a state which is an eigenfunction of an operator, we say the system has a well-defined value for the observable associated with the operator, for example, position, momentum, energy, etc. Every time we measure we get the same result. However, if the system is in a state that is not an eigenfunction of the operator, for example, if \(\hat{o} | \Psi \rangle = | \Phi \rangle\), the system does not have a well-defined value for the observable. Then the measurement results have a statistical character and each measurement gives an unpredictable result in spite of the fact that the system is in a well-defined state \(| \Psi \rangle\). Under these circumstances, all we can do is calculate a mean value for the observable. This is unheard of in classical physics where, if a system is in a well-defined state, all its physical properties are precisely determined. In quantum mechanics a system can be in a state which has a well-defined energy, but its position and momentum are un-determined.
The quantum mechanical recipe for calculating the mean value of an observable is now derived. Consider a system in the state \(| \Psi \rangle\), which is not an eigenfunction of the energy operator, \(\hat{H}\). A statistically meaningful number of such states are available for the purpose of measuring the energy. Quantum mechanical principles require that an energy measurement must yield one of the energy eigenvalues, \(\epsilon_{i}\), of the energy operator. Therefore, the average value of the energy measurements is calculated as,
\[ \langle E \rangle = \frac{\sum_{i} n_{i} \epsilon_{i}}{N} \label{Equation 19} \]
where ni is the number of times \(\epsilon_{i}\) is observed, and N is the total number of measurements. Therefore, pi = ni/N, is the probability that \(\epsilon_{i}\) is observed. Equation (19) becomes
\[ \langle E \rangle = \sum_{i} p_{i} \epsilon_{i} \label{Equation 20} \]
According to quantum mechanics, for a system in the state \(| \Psi \rangle, p_{i} = \langle \Psi | i \rangle \langle i | \Psi \rangle\), where the \(| i\rangle\) are the eigenfunctions of the energy operator. Equation (20) can now be re-written as,
\[ \langle E \rangle = \sum_{i} \langle \Psi | i \rangle \langle i | \Psi \rangle \epsilon_{i} = \sum_{i} \langle \Psi | i \rangle \epsilon_{i} \langle i | \Psi \rangle \label{Equation 21} \]
However, it is also true that
\[ \hat{H} | i \rangle = \epsilon_{i} | i \rangle = | i \rangle \epsilon_{i} \label{Equation 22} \]
Substitution of equation (22) into (21) yields
\[ \langle E \rangle = \sum_{i} \langle \Psi | \hat{H} | i \rangle \langle i | \Psi \rangle \label{Equation 23} \]
As eigenfunctions of the energy operator, the \(| i \rangle\) form a complete basis set, making available the discrete completeness condition, \(\sum_{i} | i \rangle \langle i | = 1\), the use of which in equation (23) yields
\[ \langle E \rangle = \langle \Psi | \hat{H} | \Psi \rangle \label{Equation 24} \]
This formalism is general and applies to any operator-observable pair. The average value for the observed property may always be calculated as,
\[ \langle o \rangle = \langle \Psi | \hat{o} | \Psi \rangle \label{Equation 25} \]
These principles are now applied to a very simple problem B the particle in a box. Schrödinger's equation in coordinate space,
\[ - \frac{\hbar^{2}}{2m} \frac{d^{2}}{dx^{2}} \langle x | \Psi \rangle = E \langle x | \Psi \rangle \label{Equation 26} \]
can be solved exactly, yielding the following eigenvalues and eigenfunctions,
\[ E_{n} = \frac{n^{2} h^{2}}{8ma^{2}} \label{Equation 27} \]
\[ \langle x | \Psi_{n} \rangle = \sqrt{\frac{2}{a}} \sin \left(\dfrac{n \pi x}{a}\right) \label{Equation 28} \]
where a is the box dimension, m is the particle mass, and n is a quantum number restricted to integer values starting with 1.
Substitution of equation (28) into (26) confirms that it is an eigenfunction with the manifold of allowed eigenvalues given by equation (27). However, equation (28) is not an eigenfunction of either the position or momentum operators, as is shown below.
\[ \langle x | \hat{x} | \Psi_{n} \rangle = x \langle x | \Psi_{n} \rangle = x \sqrt{2}{a} \sin \left(\dfrac{n \pi x}{a}\right) \label{Equation 29} \]
\[ \langle x | \hat{p} | \Psi_{n} \rangle = \frac{\hbar}{i} \frac{d}{dx} \langle x | \Psi_{n} \rangle = \frac{n \pi}{a} \sqrt{2}{a} \cos \left(\dfrac{n \pi x}{a}\right) \label{Equation 30} \]
To summarize, the particle in a box has a well-defined energy, but the same is not true for its position or momentum. In other words, it is not buzzing around the box executing a classical trajectory. The outcome of an energy measurement is certain, but position and momentum measurements are uncertain. All we can do is calculate the expectation value for these observables and compare the calculations to the mean values found through a statistically meaningful number of measurements.
Next we set up the calculation for the expectation value for position utilizing the recipe expressed in equation (25).
\[ \langle x \rangle_{n} = \langle \Psi_{n} | \hat{x} | \Psi_{n} \rangle \label{Equation 31} \]
Evaluation of (31) in coordinate space requires the continuous completeness condition.
\[ \int_{0}^{a} | x \rangle \langle x | dx = 1 \label{Equation 32} \]
Substitution of (32) into (31) gives
\[ \langle x \rangle_{n} = \int_{0}^{a} \langle \Psi_{n} | x \rangle \langle x | \hat{x} | \Psi_{n} \rangle dx = \int_{0}^{a} \langle \Psi_{n} | x \rangle x \langle x | \Psi_{n} \rangle dx = \frac{a}{2} \label{Equation 33} \]
The expectation value for momentum is calculated in a similar fashion,
\[ \langle p \rangle_{n} = \int_{0}^{a} \langle \Psi_{n} | x \rangle \langle x | \hat{p} | \Psi_{n} \rangle dx = \int_{0}^{a} \langle \Psi_{n} | x \rangle \frac{\hbar}{i} \frac{d}{dx} \langle x | \Psi_{n} \rangle dx = 0 \label{Equation 34} \]
In other words, the expectation values for position and momentum are the same for all the allowed quantum states of the particle in a box.
It is now necessary to explore the meaning of \(\langle x | \Psi \rangle\). It is the probability amplitude that a system in the state \(| \Psi \rangle\), will be found at position x. \(| \langle x | \Psi \rangle |^{2}\) or \(\langle \Psi | x \rangle \langle x | \Psi \rangle\), is the probability density that a system in the state \(| \Psi \rangle\) will be found at position x. Thus equation (28) is an algorithm for calculating probability amplitudes and probability densities for the position of the particle in a one-dimensional box. This, of course, is true only if \(| \Psi \rangle\) is normalized.
\[ \langle \Psi | \Psi \rangle = \int_{0}^{a} \langle \Psi | x \rangle \langle x | \Psi \rangle dx = 1 \label{Equation 35} \]
There are two ways to arrive at the integral in equation (34). One can insert the continuous completeness relation (32) at the | on the left side, or, equivalently one can express \(| \Psi \rangle\), as a linear superposition in the continuous basis set x,
\[ | \Psi \rangle = \int_{0}^{a} | x \rangle \langle x | \Psi \rangle dx \label{Equation 36} \]
and projecting this expression onto \(\langle \Psi |\).
A quantum particle is described by its wavefunction rather than by its instantaneous position and velocity; a confined quantum particle, such as a particle in a box, is not moving in any classical sense, and must be considered to be present at all points in space, properly weighted by \(| \langle x | \Psi \rangle |^{2}\).
Thus, \(\langle x | \Psi \rangle \) allows us to examine the coordinate space probability distribution and to calculate expectation values for observables such as was done in equations (33) and (34). Plots of \(| \langle x | \Psi_{n} \rangle |^{2}\) show that the particle is distributed symmetrically in the box, and \(\langle x | \Psi_{n} \rangle \), allows us to calculate the probability of finding the particle anywhere inside the box.
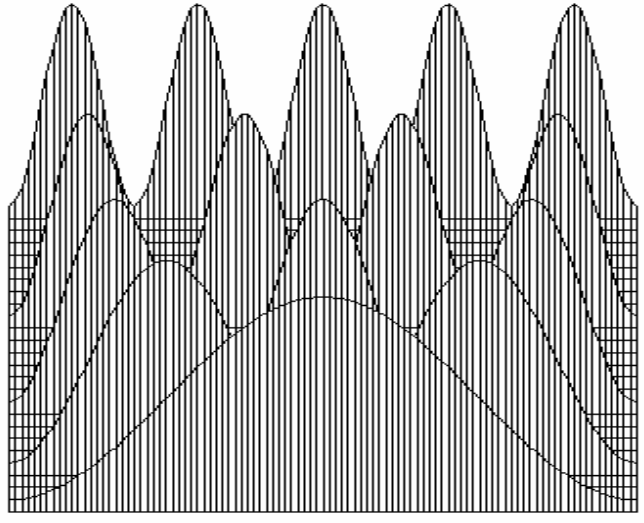
Position_Distribution
The coordinate-space wavefunction does not say much about momentum, other than its average value is zero (see equation 34). However, a momentum-space wavefunction, \(\langle p | \Psi \rangle \), can be generated by a Fourier transform of \(\langle x | \Psi \rangle \). This is accomplished by projecting equation (36) onto momentum space by multiplication on the left by \(\langle p | \).
\[\langle p | \Psi_{n} \rangle = \int_{0}^{a} \langle p|x \rangle \langle x| \Psi_{n} \rangle dx = \frac{1}{\sqrt{2 \pi \hbar}} \int_{0}^{a} \exp \left(- \dfrac{ipx}{\hbar}\right) \sqrt{\frac{2}{a}} \sin \left(\dfrac{n \pi x}{a}\right) dx \label{Equation 37} \]
The term preceding the integral is the normalization constant (previously ignored) for the momentum wavefunction. Evaluation of the integral on the right side yields,
\[\langle p | \Psi_{n} \rangle = n \sqrt{a \pi \hbar^{3}} \bigg[ \frac{1 - \cos (n \pi) \exp (- \frac{ipa}{\hbar})}{n^{2} \pi^{2} \hbar^{2} - a^{2} p^{2}} \bigg] \label{Equation 38} \]
Now with the continuous completeness relationship for momentum,
\[\int_{- \infty}^{\infty} | p \rangle \langle p | dp = 1 \label{Equation 39} \]
one can re-calculate \(\langle x \rangle_{n}\) and \(\langle p \rangle_{n}\) in momentum space.
\[\langle x \rangle_{n} = \int_{- \infty}^{\infty} \langle \Psi_{n} | p \rangle \langle p | \hat{x} | \Psi_{n} \rangle dp = \int_{- \infty}^{\infty} \langle \Psi_{n} | p \rangle \frac{- \hbar}{i} \frac{d}{dp} \langle p | \Psi_{n} \rangle dp = \frac{a}{2} \label{Equation 40} \]
\[\langle p \rangle_{n} = \int_{- \infty}^{\infty} \langle \Psi_{n} | \hat{p} | p \rangle \langle p | \Psi_{n} \rangle dp = \int_{- \infty}^{\infty} \langle \Psi_{n} | p \rangle p \langle p | \Psi_{n} \rangle dp = 0 \label{Equation 41} \]
It is clear that \(\langle x | \Psi_{n} \rangle\) and \(\langle p | \Psi_{n} \rangle\) contain the same information; they just present it in different languages (representations). The coordinate space distribution functions for the particle in a box shown above are familiar to anyone who has studied quantum theory, however, because chemists work mainly in coordinate space, the momentum distributions are not so well known. A graphical representation of \(| \langle p | \Psi_{n} \rangle |^{2}\) for the first five momentum states is shown below. The distribution functions are offset by small increments for clarity of presentation.
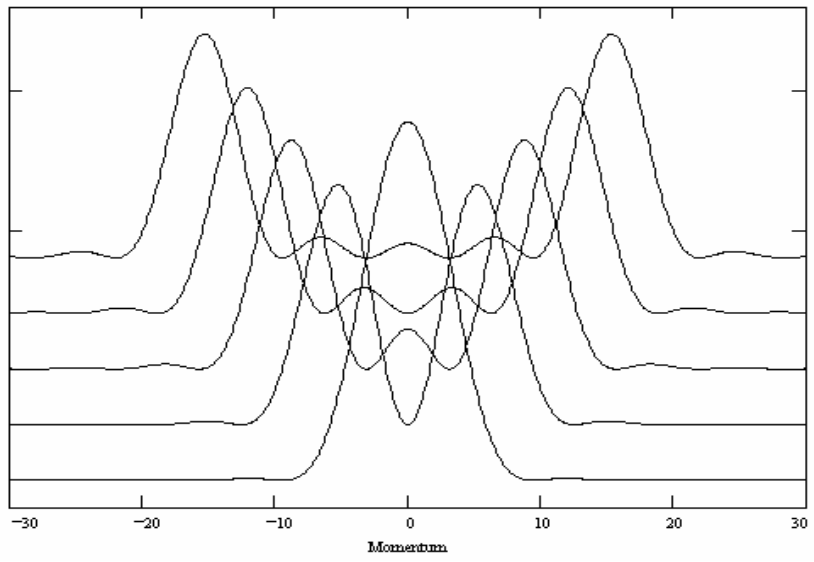
As just shown, the particle in a box can be used to illustrate many fundamental quantum mechanical concepts. To demonstrate that some systems can be analyzed without solving Schrödinger’s equation we will briefly consider the particle on a ring. This model has been used to study the behavior of the \(\pi\)-electrons of benzene.
In order to satisfy the requirement of being single-valued, the momentum wavefunction in coordinate space for a particle on a ring of radius R must satisfy the following condition,
\[\langle x + 2 \pi R | p \rangle = \langle x | p \rangle \label{Equation 42} \]
This leads to,
\[\exp \left(\dfrac{i2 \pi Rp}{\hbar}\right) \exp \left(\dfrac{ipx}{\hbar}\right) = \exp \left(\dfrac{ipx}{\hbar}\right) \label{Equation 43} \]
This equation can be written as,
\[\exp \left(\dfrac{i2 pi Rp}{\hbar}\right) = 1 = \exp(i2 \pi m) \quad where\; m = 0, \pm 1, \pm 2, \ldots \label{Equation 44} \]
Comparison of the left with the far right of this equation reveals that,
\[\frac{Rp}{\hbar} = m \label{Equation 45} \]
It is easy to show that the energy manifold associated with this quantum restriction is,
\[E_{m} = m^{2} \left(\dfrac{\hbar^{2}}{2m_{e} R^{2}} \right) \label{Equation 46} \]
The corresponding wavefunctions can be found in the widely used textbook authored by Atkins and de Paula (11).
There are, of course, many formulations of quantum mechanics, and all of them develop quantum mechanical principles in different ways from diverse starting points, but they are all formally equivalent. In the present approach the key concepts are de Broglie’s hypothesis as stated in equation (2), and the eigenvalue equations (3) and (9) expressed in the momentum and coordinate representations, respectively.
Another formulation (Heisenberg’s approach) identifies the commutation relation of equation (47) as the basis of quantum theory, and adopts operators for position and momentum that satisfy the equation.
\[[ \hat{p}, \hat{x}] = \hat{p} \hat{x} - \hat{x} \hat{p} = \frac{\hbar}{i} \label{Equation 47} \]
Equation (47) can be confirmed in both coordinate and momentum space for any state function \(| \Psi \rangle\) using the operators in the table above.
\[\langle x | (\hat{p} \hat{x} - \hat{x} \hat{p}) | \Psi \rangle = \frac{\hbar}{i} \left(\dfrac{d}{dx} x - x \dfrac{d}{dx}\right) \langle x | \Psi \rangle = \frac{\hbar}{i} \langle x | \Psi \rangle \label{Equation 48} \]
\[\langle p | (\hat{p} \hat{x} - \hat{x} \hat{p}) | \Psi \rangle = i \hbar \left(p \dfrac{d}{dd} - \dfrac{d}{dp} p \right) \langle p | \Psi \rangle = \frac{\hbar}{i} \langle p | \Psi \rangle \label{Equation 49} \]
The meaning associated with equations (48) and (49) is that the observables associated with non-commuting operators cannot simultaneously have well-defined values. This, of course, is just another statement of the uncertainty principle.
The famous double-slit experiment illustrates the uncertainty principle in a striking way. To illustrate this it is mathematically expedient to begin with infinitesimally thin slits. Later this restriction will be relaxed.
A screen with infinitesimally thin slits (6) at x1 and x2 projects the incident beam into a linear superposition of position eigenstates.
\[| \Psi \rangle = \frac{1}{\sqrt{2}} [ | x_{1} \rangle + | x_{2} \rangle ] \label{Equation 50} \]
Expressing this state in the coordinate representation yields the following superposition of Dirac delta functions.
\[\langle x | \Psi \rangle = \frac{1}{\sqrt{2}} [ \langle x | x_{1} \rangle + \langle x | x_{2} \rangle ] = \frac{1}{\sqrt{2}} [ \delta (x-x_{1}) + \delta(x-x_{2})] \label{Equation 51} \]
According to the uncertainty principle this localization of the incident beam in coordinate space is accompanied by a delocalization of the x-component of the momentum, px. This can be seen by projecting \(| \Psi \rangle\) onto momentum space.
\[\langle p_{x} | \Psi \rangle = \frac{1}{\sqrt{2}} [ \langle p_{x} | x_{1} \rangle + \langle p_{x} | x_{2} \rangle ] = \frac{1}{2 \sqrt{\pi \hbar}} \bigg[ \exp \left(- \dfrac{ip_{x}x_{1}}{\hbar}\right) + \exp \left(- \dfrac{ip_{x} x_{2}}{\hbar}\right) \bigg] \label{Equation 52} \]
The momentum probability distribution in the x-direction, \(P(p_{x})=| \langle p_{x} | \Psi \rangle |^{2}\), reveals the required spread in momentum, plus the interesting interference pattern in the momentum distribution that will ultimately be projected onto the detection screen. As Marcella (6) points out the detection screen is actually measuring the x-component of the momentum.
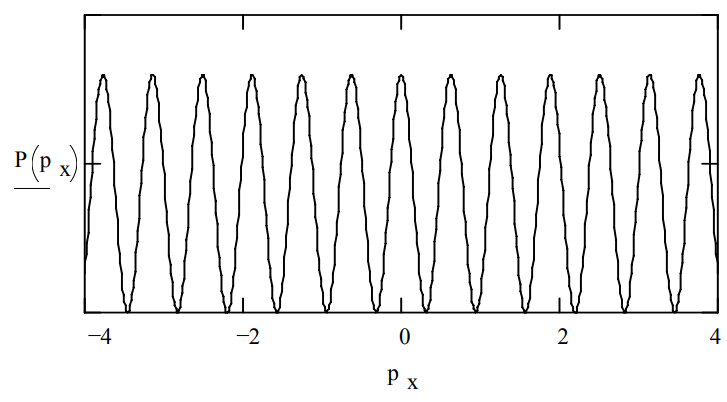
Of course, in the actual experiment the slits are not infinitesimally thin and the diffraction pattern takes on the more familiar appearance reported in the literature (7) and textbooks (8). For example, a linear superposition of Gaussian functions can be used to represent the coordinate-space wavefunction at a screen with two slits of finite width.
\[\langle x | \Psi \rangle = \exp [-(x-x_{1})^{2}]+ \exp [-(x-x_{2})^{2}] \label{Equation 53} \]
The Fourier transform of this state into momentum space leads to the momentum distribution shown in the figure below (9).
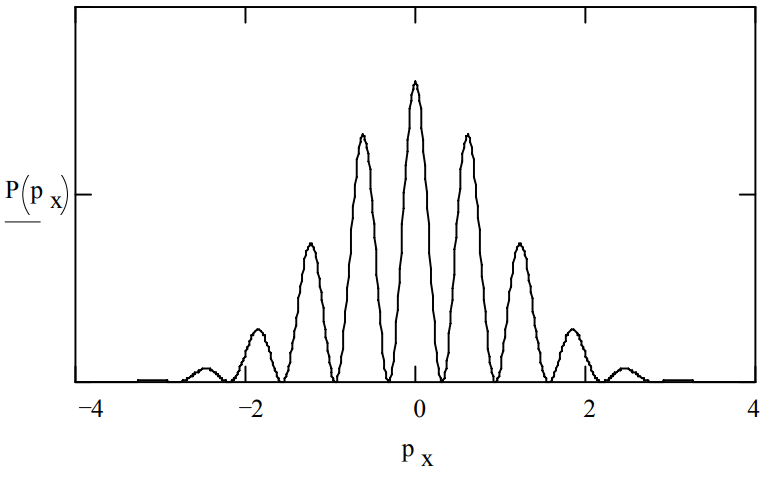
The double-slit experiment reveals the three essential steps in a quantum mechanical experiment:
- state preparation (interaction of incident beam with the slit-screen)
- measurement of observable (arrival of scattered beam at the detection screen)
- calculation of expected results of the measurement step
The Dirac delta function appeared in equation (51). It expresses the fact that the position eigenstates form a continuous orthogonal basis. The same, of course, is true for the momentum eigenstates.
The bracket \(\langle x | x' \rangle\) is zero unless x = x′. This expresses the condition that an object at x′ is not at x. It is instructive to expand this bracket in the momentum representation.
\[\langle x | x' \rangle = \int_{- \infty}^{\infty} \langle x|p \rangle \langle p|x' \rangle dp = \frac{1}{2 \pi \hbar} \int_{- \infty}^{\infty} \exp \left(\dfrac{ip(x-x')}{\hbar}\right)dp = \delta (x-x') \label{Equation 54} \]
The same approach for momentum yields,
\[\langle p|p' \rangle = \int_{- \infty}^{\infty} \langle p|x \rangle \langle x|p' \rangle dx = \frac{1}{2 \pi \hbar} \int_{- \infty}^{\infty} \exp \left(\dfrac{-i(p-p')x}{\hbar}\right) dx = \delta (p-p') \label{Equation 55} \]
The Dirac delta function has great utility in quantum mechanics, so it is important to be able to recognize it in its several guises.
The time-dependent energy operator can be obtained by adding time dependence to equation (1) so that it represents a classical one-dimensional plane wave moving in the positive x-direction.
\[\langle x | \lambda \rangle \langle t | \nu \rangle = \exp \left( i2 \pi \frac{x}{\lambda}\right) \exp (-i2 \pi \nu t) \label{Equation 56} \]
This classical wave equation is transformed into a quantum mechanical wavefunction by using (as earlier) the de Broglie relation and E = h\(\nu\).
\[\langle x|p \rangle \langle t|E \rangle = \exp \left(\dfrac{ipx}{\hbar}\right) \exp \left(- \dfrac{iEt}{\hbar}\right) \label{Equation 57} \]
From this equation we obtain the important Dirac bracket relating energy and time.
\[\langle t|E \rangle = \exp \left(- \dfrac{iEt}{\hbar}\right) \label{Equation 58} \]
The time-dependent energy operator is found by projecting the energy eigenvalue equation,
\[\hat{H} |E \rangle = E|E \rangle \label{Equation 59} \]
into the time domain.
\[\langle t| \hat{H} |E \rangle = E \langle t|E \rangle = E \exp \left(- \dfrac{iEt}{\hbar}\right) = i \hbar \frac{d \langle t|E \rangle}{dt} \label{Equation 60} \]
Comparison of the first and last terms reveals that the time-dependent energy operator is
\[\langle t | \hat{H} = i \hbar \frac{d}{dt} \langle t | \label{Equation 61} \]
We see also from equation (60) that
\[i \hbar \frac{d}{dt} \langle t | = E \langle t | \label{Equation 62} \]
So that in general,
\[i \hbar \frac{d}{dt} \langle t | \Psi \rangle = E \langle t | \Psi \rangle \label{Equation 63} \]
Integration of equation (63) yields a general expression for the time-dependence of the wavefunction.
\[\langle t | \Psi \rangle = \exp \left(- \dfrac{iE(t-t_{0})}{\hbar}\right) \langle t_{0} | \Psi \rangle \label{Equation 64} \]
References
- Chester, M. Primer of Quantum Mechanics; Krieger Publishing Co.:Malabar, FL, 1992.
- Das, A.; Melissinos, A. C. Quantum Mechanics: A Modern Introduction; Gordon and Breach Science Publishers: New York, 1986.
- Feynman, R. P.; Leighton, R. B.; Sands, M. The Feynman Lectures on Physics, Vol.3; Addison-Wesley: Reading, 1965.
- Martin, J. L. Basic Quantum Mechanics; Claredon Press, Oxford, 1981.
- Townsend, J. S. A Modern Approach to Quantum Mechanics; University Science Books, Sausalito, 2000.
- Marcella, T. V. “Quantum interference with slits,” European Journal of Physics 23, 615-621 (2002).
- Tonomura A.; Endo, J.; Matsuda, T.; Kawasaki, T.; Ezawa, H. “Demonstration of single-electron buildup of an interference pattern,” American Journal of Physics 57,117-120 (1989).
- French, A. P.; Taylor, E. F. An Introduction to Quantum Physics; W. W. Norton & Co., Inc. New York, 1978.
- For further detail visit: www.users.csbsju.edu/~frioux/...t/new-slit.htm. For different approach see: www.users.csbsju.edu/~frioux/...other2slit.pdf. A description of a recent example of a temporal double-slit is available at www.users.csbsju.edu/~frioux/...poral2slit.pdf.
- Lieber, M. “Quantum mechanics in momentum space: An illustration,” American Journal of Physics 43, 486-487 (1975).
- Atkins, P.; de Paulo, J. Physical Chemistry; 7th ed. W. H. Freeman & Co., New York, 2002, p. 347.

