1: Spectroscopy
- Page ID
- 4468
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Spectroscopy generally is defined as the area of science concerned with the absorption, emission, and scattering of electromagnetic radiation by atoms and molecules, which may be in the gas, liquid, or solid phase. Visible electromagnetic radiation is called light, although the terms light, radiation, and electromagnetic radiation can be used interchangeably. You will discover some properties of electromagnetic radiation in Activities 1 and 2.
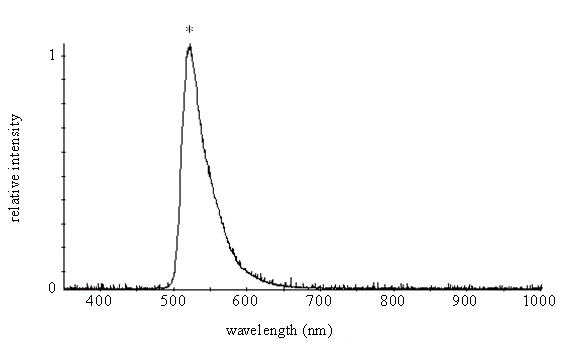
Spectroscopy played a key role in the development of quantum mechanics and is essential to understanding molecular properties and the results of spectroscopic experiments. It is used as a “stepping stone” to take us to the concepts of quantum mechanics and the quantum mechanical description of molecular properties in order to make the discussion more concrete and less abstract and mathematical. A spectrum is a graph that shows the intensity of radiation at different wavelengths or the response of the atomic or molecular system to different wavelengths of the radiation. Examples of absorption and fluorescence spectra are shown in Figures \(\PageIndex{1}\) and \(\PageIndex{2}\).
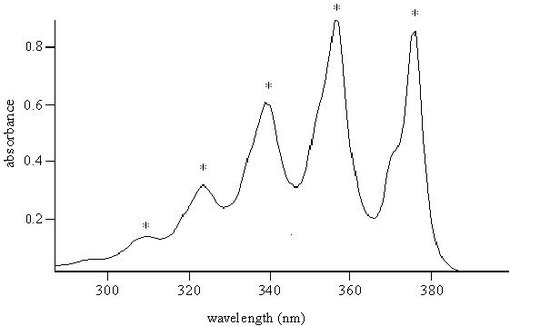
An absorption spectrum shows how much light is absorbed by a sample at each wavelength of the radiation. Absorption spectra generally are displayed in one of three different ways: as a plot of either the transmission (T), absorbance (A), or the absorption coefficient (ε) on the y-axis with the wavelength on the x-axis. Sometimes the absorbance is called the optical density (OD). If we define I0 as the intensity of light incident on a sample, I as the intensity of the light transmitted by the sample, d as the thickness of the sample, and c as the concentration of the absorbing species in the sample, then
\[T = \dfrac {I}{I_0} \label {1.1}\]
\[A = log \dfrac {I_0}{I} \label {1.2}\]
\[\epsilon = \dfrac {1}{dc} log_{10} \left (\dfrac {I_0}{I} \right ) \label {1.3}\]
Equation \(\ref{1.3}\) is a rearranged form of Beer’s law, as developed in a Problem at the end of this chapter. Each of the quantities I, \(I_0\), and ε are functions of the wavelength of the light being used.
Three different ways of plotting absorption spectra are used because each has particular advantages. The transmission function is simple. The absorbance condenses large variations by using a logarithm so reasonably-sized graphs show both large and small variations in light intensity. Also, the absorbance is proportional to a fundamental property, which is the absorption coefficient. The absorption coefficient is of interest because it can be calculated from the transition moment, which is a quantum mechanical quantity. In Chapter 4, we will use quantum mechanics to calculate transition moments for some molecules.
Energy often is released from atoms, molecules, and solids as light. This light is called luminescence in general and fluorescence and phosphorescence in particular situations that are identified by the decay time of the luminescence and the nature of the excited state. The decay time is the characteristic time it takes for the luminescence to disappear after the source of energy is removed or turned off. Fluorescence decays quickly (in microseconds or faster), and phosphorescence decays slowly (milliseconds to minutes). The concepts of angular momentum and a transition moment that are developed in other chapters will help you understand why these decay times are so different and depend on the nature of the excited state. The fluorescence spectrum in Figure \(\PageIndex{2}\). shows how the intensity of the light emitted by fluorescein varies with wavelength. This spectrum is an example of a distribution function. It shows how the fluorescence intensity is distributed over a range of wavelengths.
The idea of a distribution function is an important one that you may have encountered previously (e.g. the Maxwell-Boltzmann velocity distribution) and will encounter again. The term spectroscopy also is used in electron spectroscopy and mass spectroscopy where the energy distribution of electrons and the mass distribution of ions are the quantities of interest. These distributions give the absolute or relative number of particles with a given energy or mass. In general, any function that shows how some property is distributed (i.e. a distribution function) can be called a spectrum.
In scattering, light incident on an atomic or molecular system is deflected to some other direction, and in the process the wavelength of the light may or may not change. When the wavelength does not change, the scattering is called elastic or Rayleigh scattering, and when the wavelength does change, it is called inelastic scattering or Raman scattering. Scattering spectra show the intensity of radiation that is scattered in some direction as a function of the wavelength of the scattered radiation. Rather than plotting the absolute wavelength on the x-axis, it is common to plot the change in wavenumber value for the radiation, because this quantity is proportional to the energy left behind in the molecule during the scattering process.
The spectra in Figures \(\PageIndex{1}\) and \(\PageIndex{2}\) are characterized by intense features, which are called spectral bands or lines, at some points on the x-axis. The peaks of spectral bands are indicated by a star in Figures \(\PageIndex{1}\) and \(\PageIndex{2}\). The spectral bands are characterized by three quantities: their location on the x-axis, their intensity or height, and their width or shape. Quantum mechanics is needed to understand and explain these characteristics. From this book, you will learn how to interpret and calculate the positions of the bands on the x-axis in terms of the energy level structure of molecules and the intensities in terms of the transition moments. The band widths and shapes are due to dynamical effects that are unfortunately beyond the scope of this book.
The above discussion of spectroscopy brings us to the question: What is electromagnetic radiation? During the nineteenth century, research in the areas of optics, electricity, and magnetism and the unification of the resulting concepts by Maxwell provided convincing evidence that electromagnetic radiation consists of two sinusoidally oscillating fields or waves, an electric field and a magnetic field. In the simplest situation, which is radiation in a vacuum, these fields oscillate perpendicular to each other and perpendicular to the direction of propagation of the wave.
Various units are used in discussing electromagnetic radiation, and you must be familiar with conversions between them. Tables 3-5 provide the most frequently used units and their relationships. These units include hertz, joules, electron volts, wavenumbers, Angstroms, and nanometers. Any of these units, not just wavelength, can be used when plotting a spectrum.
The electromagnetic spectrum commonly is viewed as split into different regions. These regions are classified by the nature of the instrumentation (sources, wavelength selectors and detectors) that are used in the different frequency ranges. The different radiation frequencies correspond to different kinds of motions or degrees of freedom within a molecule, e.g. rotational motion (microwave region), vibrational motion (infrared region), electronic motion (generally visible through soft x-ray regions) and nuclear and electron spin motion (radio and microwave regions). After a description of the historical development of quantum mechanics and the introduction of some key concepts associated with it, this book uses quantum mechanics to account for the spectra associated with these motions and identify what can be learned about these degrees of freedom from the spectra.
Table 2 lists the parameters that characterize electromagnetic radiation. As you can see from this table, Greek letters often are used to represent physical quantities. The use of symbols makes writing equations and derivations and showing relationships much shorter and quicker than using words, but we pay a price for this convenience. We have to remember what the symbols mean, and since there are more quantities than there are symbols, even with the use of both Latin and Greek letters, some symbols mean more than one thing. Consequently, we must deduce their meaning from the context. Tables at the end of this chapter provide you with information about Greek letters and other items such as units and physical constants that will prove useful to you.
While spectra often are plotted with the wavelength, and sometimes with the wavenumber values or the frequency, on the x-axis, usually the energy associated with a photon at a particular wavelength is needed in order to relate spectra to the energy level structure of molecules. The following relationships convert wavelength λ, wavenumbers \(\bar {\nu}\), and frequency ν to photon energy E.
\[E = \dfrac {hc}{\lambda} \label {1.4}\]
\[E = hc \bar {\nu} \label {1.5}\]
\[E = h \nu \label {1.6}\]
where \(c\) is the speed of light in a vacuum.
Since wavenumbers and frequency are proportional to energy, sometimes spectroscopists measure energy in these units for convenience.


