V. Examples of Radical Philicity in Reactions of Carbohydrates

A. Hydrogen-atom abstraction

The reactions shown in equations 4 and 5 illustrate the impor­tance of radical philicity in hy­dro­gen-abstraction reactions by showing that the nucleo­philic radical R· abstracts the elec­tron-de­ficient hydrogen atom attached to sulfur (eq 5) more rapidly than the electron-rich hydro­gen atom bonded to tin (eq 4).^33–35 The differences in rate constants and enthalpies for these two reac­tions underscore the fact that radical philicity affects the stability of the transition-state struc­ture in a reac­tion but not the overall energy changes due to bond breaking and bond forma­tion.

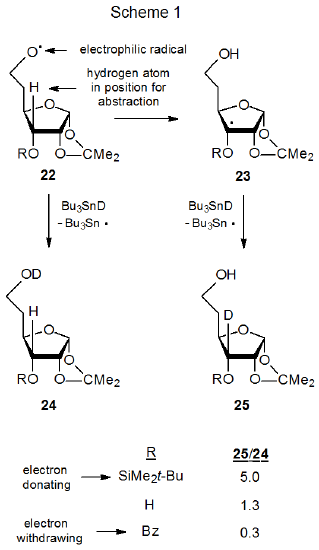
Comparing the three reactions pictured in Scheme 1 draws attention to the effect of radical philicity on hydrogen-atom abstraction from carbo­hy­drates. In each of these reactions the oxygen-cen­tered radical 22 either abstracts a deuterium atom from Bu₃SnD to give the deuterated alco­hol 24, or it reacts internally with H-3 to generate the carbon-centered radical 23.³⁶ After the radical 23 forms, it then abstracts a deu­terium atom for Bu₃SnD to give the second reaction product (25). The relative amounts of products 24 and 25 provide a measure of external (deuterium) versus internal (hydrogen) abstrac­tion. As H‑3 becomes less electron rich, internal reaction (22\(\rightarrow\)23) becomes less com­pet­i­tive. External abstraction ­(22\(\rightarrow\)24), on the other hand, should not be notice­ably affected by changes in substituents at C-3. If, as expected, the transition states for the internal hydro­gen-abstraction reactions shown in Scheme 1 are early, these reactions support the idea that polarity matching has a critical role in deter­mining the favored reaction pathway for the radical 22.

B. Radical Addition

Pyranos-1-yl radicals add readily to electron-deficient, carbon-carbon double bonds but are much less reactive toward double bonds lacking electron-withdrawing substituents.^37,38 A group of reactions that illustrates this difference in reactivity is found in Scheme 2.³⁷ The ability of the pyran­os-1-yl radical 26 to add to the unsaturated compounds shown in Scheme 2 correlates with the reduction potentials of these com­pounds; that is, addition to com­pounds with less negative reduction potentials occurs more rapidly than addi­tion to compounds with more negative reduction potentials.³⁷

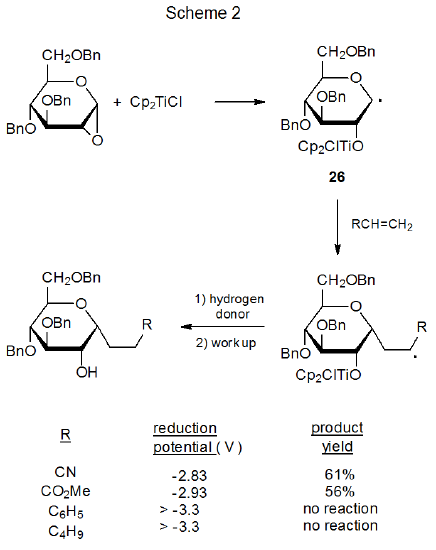
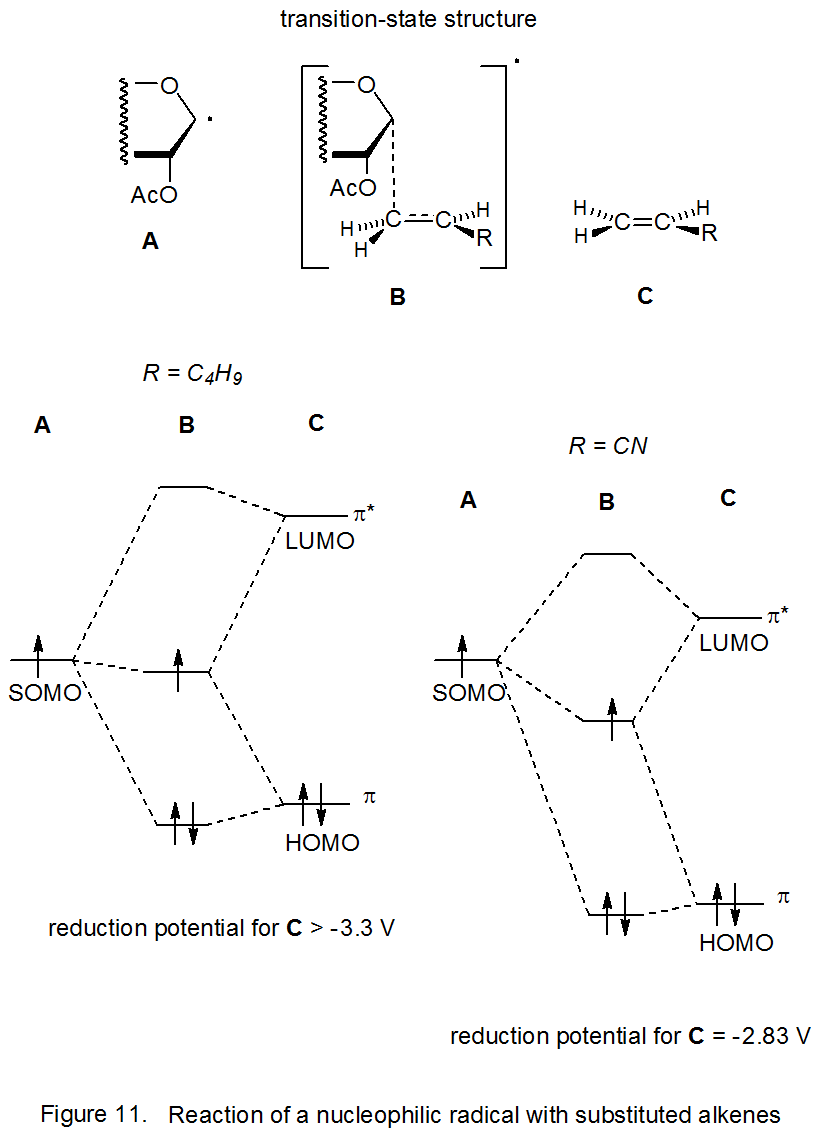
Since the reduction potential in a substituted alkene is a measure of the ease of introducing an electron into a π* orbital, this poten­tial becomes an indicator of energy-level positioning. When comparing two reduc­tion potent­ials, the less negative one has a lower energy level for the π* orbi­tal. Because this lower energy level causes the π* orbital (LUMO) to interact more effectively with the SOMO of the adding radical, transition-state stabilization due to frontier-orbital inter­action increases as the reduction potential for the substituted alkene becomes less negative (Figure 11).

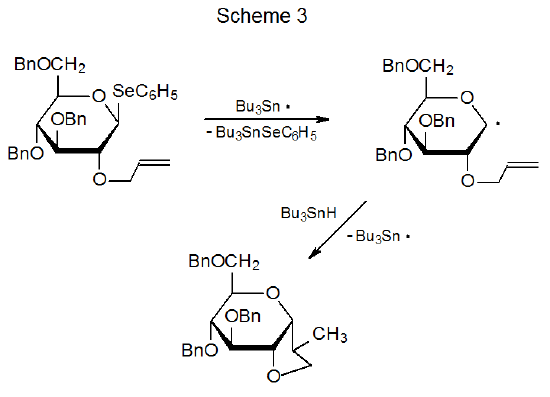
Although the addition of a nucleophilic radical to a π bond that is not electron-deficient is too slow to be observed in the reactions shown in Scheme 2, the situation changes when reactions become intramolecular. For π bonds that are 1,5- or 1,6-related to a radical center, intramolecular addi­tion can take place even if the π bond is not decidedly electron-deficient (Scheme 3).³⁹ As far as overall reaction rate is concerned, forced, close proximity of the radical center to the π bond can compensate for a small transition-state sta­bil­i­zation caused by a large separation in energy levels of inter­acting, frontier orbitals.

C. Polarity-Reversal Catalysis

The philicity of radicals involved in hydrogen-abstraction reactions provides the basis for a phe­nomenon known as polarity-reversal catalysis.^4,33–35,40 This type of catalysis, which is respon­sible for the effect that thiols have on the reactions of carbohydrate acetals and ethers, is discussed in Section III of Chapter 5 in Volume II.