1.4: Sigmatropic Rearrangements

Objectives

After completing this section, you should be able to:

1. Identify sigmatropic rearrangements including hyrdride shifts and Cope, Claisen, and Wittig rearrangements
2. Understand the orbital analysis of sigmatropic rearrangements
3. Draw curved arrows to illustrate electron flow for sigmatropic rearrangements
4. Given a sigmatropic rearrangement starting material, accurately predict the product including stereochemistry
5. Use retrosynthetic analysis to determine starting materials given a sigmatropic rearrangement product

Key Terms

Make certain that you can define, and use in context, the key terms below.

• Suprafacial
• Antarafacial
• Hydride shift
• Cope rearrangement
• Claisen rearrangement
• Wittig rearrangement

Study Notes

Sigmatropic rearrangements are pericyclic reactions that, no surprise, provide rearranged products. The most common include hydrogen shifts across pi systems and formation of new carbon-carbon bonds across allyl-type structural fragments. The most synthetically useful are the Cope and Claisen rearrangements which are formally classified as [3,3] rearrangements. (See Chapter 1.1: Introduction to Pericyclic Reactions for an explanation of naming sigmatropic rearrangements.) Your goal is to understand the theoretical basis of these reactions using a molecular orbital analysis and to apply these reactions in synthesis. You should focus on accurately predicting products with a focus on stereochemistry and, given a complicated product, to accurately predict the starting material used to generate it.

Chapter 1.1 provided an overview of pericyclic reactions, including sigmatropic rearrangements. Sigmatropic rearrangements are intramolecular reactions involving the migration of a sigma bond across a pi system. Examples include hydrogen atoms migrating across dienes upon heating (some even do this spontaneously at room temperature) and hydrogen atoms migrating across alkenes with light. Double allyl-type systems also commonly react via sigmatropic rearrangements, with 1,5-dienes participating in Cope rearrangements while ally vinyl ethers produce 1,4-enones after Claisen rearrangements. Most of our attention will focus on the applications of Cope and Claisen reactions in synthesis.

Thermal and Photochemical Hydride Shifts

Building on the introduction to pericyclic reactions presented in Chapter 1.1, we must understand the following results: [1,5] hydrogen shifts occur thermally while [1,3] hydrogen shifts happen photochemically. Labeling substrates with deuterium makes it possible to see the outcomes of these reactions which could otherwise often be invisible. Examples are shown below. As we have seen in previous sections, we need to use molecular orbital diagrams to explain these results.

Molecular Orbital Explanation for Sigmatropic Rearrangements

If we think about the transition states for the hydride shift reactions shown above, we can depict the reactions as a hydrogen (or deuterium) atom (a radical) moving across either a pentadienyl radical (heat) or an allyl radical (light).

This means that we need to draw a 5-atom and a 3-atom pi molecular orbital diagram so that we can determine the HOMO for each reaction. We can then use those diagrams to explain the experimental results. As shown below, the excited state HOMO for the 3 atom system is psi 3* and the ground state HOMO for the 5 atom system is psi 3.

What does it look like when a hydrogren atom moves across these two orbital systems? As shown below, the H is able to migrate across the same face of the pi system. As mentioned previously, this is called a suprafacial process. If the H moved from the bottom face to the top face (or vice versa), that would be an antarafacial process. Antarafacial hydrogen shifts are possible, but only for rings that are 7-membered or larger. So, the two reactions are explained by the MO diagrams showing that a thermal [1,5] H shift is suprafacial and a photochemical [1,3] H shift is suprafacial. Antarafacial [1,3] or [1,5] H shifts are too high in energy, so they do not occur.

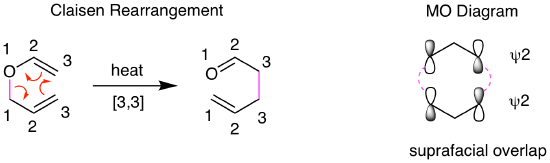
A similar analysis helps explain why thermal [3,3] sigmatropic rearrangements are common. These reactions involve suprafacial overlap between two allyl systems. Looking at the reaction we introduced in Chapter 1.1, the simplest version of the Claisen rearrangement, we can see that the key sigma bond moves across two three-atom systems. For the MO diagram, this means that allyl HOMO psi 2 overlaps in a suprafacial fashion with another allyl HOMO psi 2. We can generalize the orbital analyses of sigmatropic rearrangements as shown in the following section.

Generalized Statement of Woodward-Hoffmann Rules for Sigmatropic Rearrangements

Number of Electrons	Thermal	Photochemical
4n + 2	Suprafacial	Antarafacial
4n	Antarafacial	Suprafacial

Cope Rearrangement

The Cope rearrangement is a [3,3] sigmatropic rearrangement of a 1,5-diene. It was discovered at Bryn Mawr College by Elizabeth Hardy, a graduate student in Arthur Cope's research lab. As shown below in its simplist form, this is a reversible reaction. Consequently, the Cope retron is also a 1,5-diene. To make the Cope rearrangement synthetically useful, we must introduce a driving force that will favor formation of the product. The two most popular strategies are to provide relief of ring strain and to incorporate a tautomerization as an irreversible final step. The following problems provide examples of these strategies.

Exercise \(\PageIndex{1}\)

Predict the Cope rearrangement product of this reaction.

Answer

As mentioned above, this reaction is favorable because it relieves the strain in the cyclopropane ring. This common strategy is a useful way to generate a new 7-membered ring.

Exercise \(\PageIndex{2}\)

Predict the product of this reaction.

Answer

This is an example of an Oxy-Cope rearrangement. In Oxy-Cope reactions, placement of an OH on one of the sp³ carbons connecting the 1,5-diene results in an enol after the [3,3] sigmatropic rearrangement. An irreversible tautomerization yields the final product.

Oxy-Cope Rearrangement

As seen in the previous problem, the Oxy-Cope rearrangement is an important synthetic tool. The product of this reaction is a 1,5-enone. This is the Oxy-Cope retron which will be important when approaching synthesis problems. One way to promote Oxy-Cope reactions is to deprotonate the starting alcohol with a hydride base. These reactions are referred to as oxy-anion accelerated Cope rearrangements.

Cope Rearrangement Application

The most amazing application of the Cope rearrangement is the molecule known as bullvalene. This molecule was designed and synthesized in the lab of William von Eggers Doering and published in 1963. If you play around with the molecule, you see that there are several Cope rearrangements that are initially possible. If you draw those products, you see that even more become apparent. The net result is that this is a fluxional molecule; it does not have a set structure at room temperature. In fact, when heated, its NMR spectra are a singlet at 4.2 in the proton NMR and one peak at 86 in the carbon spectrum. For an excellent article about the bullvalene origin story, check out Addison Ault's paper in the Journal of Chemical Education.

Claisen Rearrangement

As mentioned above, the Claisen rearrangement is the conversion of an allyl vinyl ether into a 1,4-enone via a [3,3] sigmatropic rearrangement. The simplist Claisen rearrangement is picture below. The forward direction is favored because the product contains a carbonyl, so this is not a reversible reaction. The following two problems provide practice predicting products of Claisen rearrangements.

Exercise \(\PageIndex{3}\)

Predict the product of the following Claisen rearrangement.

Answer

First, find the allyl vinyl ether. It is highlighted in magenta below. Second, rotate the bonds so that carbons 1 and 6 are near each other. Third, draw the curved arrows. Fourth, draw the product. As with many reactions, numbering your atoms is helpful to ensure you get the correct product.

Exercise \(\PageIndex{4}\)

Predict the product of the following Claisen rearrangement. Hint: Think carefully about the most stable structure for your product.

Answer

Draw the curved arrows for the allyl vinyl ether to generate the 1,4-enone. The tricky part of this problem is recognizing that this product is not the lowest energy molecule possible. A keto to enol tautomerization yields the final product. This is another example of the aromatic stability of benzene. We are used to converting enols to ketones. In this case the enol is more stable because of the aromaticity of benzene.

The above reaction is a very common strategy to make a C-C bond at the ortho position of a phenol. Keep this is mind when thinking about synthesis problems. (The starting material above is easily synthesized by treating phenol with sodium hydride and allyl bromide.)

Often the most challenging aspect of the Claisen rearrangement is synthesizing the starting material. Allyl vinyl ethers are difficult to obtain and chemists have developed several useful strategies to make them. How would you do it? The answer to Problem #4 above mentions starting with phenol, a vinyl ether, and reacting it with allyl bromide. However, vinyl ethers are very rare since they readily tautomerize to carbonyls unless they are part of an aromatic ring. One solution is to start with an acetal or ketal in place of the vinyl ether. An example of this strategy is shown below. What is the mechanism for this reaction? This variant of the Claisen rearrangement forms ketones or aldehydes (R=H). We will see below that other common Claisen strategies form esters, carboxylic acids, and amides.

Exercise \(\PageIndex{5}\)

Propose a mechanism for the example Claisen reaction shown above. Remember, your mechanism must generate an allyl vinyl ether so that the Claisen rearrangement can occur. Don't forget that AcOH is acetic acid.

Answer

This is a good review of acid catalyzed ketal mechanistic steps that you learned in intro organic chemistry. The mechanism starts with those standard steps. In the second to last step, the acetate anion (generated in the first step) can deprotonate to form the neutral allyl vinyl ether (this deprotonation looks like the mechanistic step we use to form enamines from secondary amines plus ketones) that undergoes the Claisen rearrangement in the final step.

Johnson-Claisen Rearrangement

A reaction analogous to the one we just discussed above is the Johnson-Claisen rearrangement that features the unusual orthoester functional group as one of its starting materials and produces ester products. An orthoester is the ester equivalent of a ketal. Using the exact same mechanism as in the answer to Problem #5 above (with R = OMe), we can understand the Johnson-Claisen rearrangement shown below. Thus, the retron for a Johson-Claisen rearrangement is a 1,4-enester.

Ireland-Claisen Rearrangement

The Ireland-Claisen rearrangement results in the formation of carboxylic acid products and proceeds via a silyl enol ether generated after forming an enolate. The reaction and mechanism are shown below. Continuing our trend of starting with allyl alcohol, we treat it with acetic anhydride to form an allyl ester. Combining that with LDA yields an enolate that reacts with trimethylsilyl chloride (TMSCl) on the oxygen of the enolate (this is standard reactivity for silyl electrophiles with enolates) to yield the key silyl enol ether. This undergoes the Ireland-Claisen rearrangement to yield a silyl ester that is easily converted to the desired carboxylic acid upon workup with aqueous acid. (This step is analogous to acidic deprotection of silyl ethers to yield alcohols.)

Exercise \(\PageIndex{6}\)

What is the product of the following reaction?

Answer

This is an example of the Eschenmoser-Claisen rearrangement. Using an orthoamide in place of the orthoester in the Johnson-Claisen rearrangement results in the production of an unsaturated amide product by the same mechanism.

Claisen Rearrangement Alkene Geometry

One final point about the Claisen rearrangement relates to the alkene geometry formed in the reaction. The example below highlights that trans alkenes are formed while cis alkenes are not. Why? This is another example of the importance of chair-like transition states. (We first saw this with the ene reaction in the cycloadditions chapter.) Putting the methyl substituent in the more stable equatorial position leads to the trans product. With the methyl in the less stable axial position, the cis product would form.

Wittig Rearrangement

All of the sigmatropic rearrangements that we have seen so far are reactions of neutral molecules. That is the case for most sigmatropic rearrangements, but charged molecules also participate in these transformations. The Wittig rearrangement is an anionic [2,3] sigmatropic rearrangement of an allylic ether to yield a homoallylic alcohol. So, the retron for this reaction is the same as the oxo-ene reaction. A generic version of this reaction is shown below. The critical components of the starting material are an allyl ether containing an electron withdrawing "Z" group. Thus, treatment with a strong base results in deprotonation next to the Z group. The resulting anion can participate in a [2,3] sigmatropic rearrangement (numbering from the sigma bond broken to the sigma bond formed) to yield a homoallylic alcohol product, after quenching with acid.

An application of the Wittig reaction is shown below where the electron withdrawing group is a resonance stabilizing alkyne. As this example shows, the Wittig rearrangement can be a powerful ring contraction reaction. In this case, converting an 18-membered ring ether into a 15-membered ring alcohol.

Exercise \(\PageIndex{7}\)

What is the product of this Wittig rearrangement?

Answer

The phenyl is the electron withdrawing group in this molecule. So, draw the anion, then the rearrangement arrows, and finally add the proton to generate the product.

Summary Problems

Exercise \(\PageIndex{8}\)

Identify the retron in the following molecule then draw the starting material (go back only one step) that would yield the target.

Answer

This molecule contains a 1,5-diene which is the retron for the Cope rearrangement. Drawing the curved arrows for the reaction enables you to determine the starting material used to make the target. Note: There is another 1,5-diene in the molecule; however, the one shown is what was used in the 2010 Organic Letters paper to make this molecule.

Exercise \(\PageIndex{9}\)

The following transformation appeared in a paper focused on the synthesis of the novel antibiotic platensimycin. Propose a synthetic route for the conversion of the starting allylic alcohol into the triene synthetic intermediate. Then, propose a mechanism for the conversion of this material into the polycyclic product. Hints: One of the steps in your mechanism is a cycloaddition. It might help to think retrosynthetically, going backwards one step from the target for this mechanism.

Answer

The synthesis portion of this problem involves three reaction types: oxidation, Wittig, and deprotection. Both oxidations are primary alcohols to aldehydes, so feel free to use PCC, Swern, or Dess-Martin interchangeably. The key Wittig reagents are shown below. After adding the first alkene, then it's time for the deprotection with fluoride (any F minus reagent will work). This reveals the second primary alcohol for the final two steps.

For the mechanism part of the problem, spotting the Diels-Alder retron in the product is the key. This disconnection gets you back to a molecule that is very similar to the starting material. Performing a thermal [1,5] H shift on the starting molecule generates the intramolecular Diels-Alder substrate. This is an excellent example of the importance of hydrogen shifts and the amazing complexity that can be synthesized using intramolecular cycloadditions.

Reference: Journal of Organic Chemistry 2009

Exercise \(\PageIndex{10}\)

Propose a mechanism for the following reaction. Hints: The mechanism is 4 steps and involves two sigmatropic rearrangements.

Answer

Looking at the reactants, it's important to recognize that we are starting with an allyl vinyl ether, the starting material for the Claisen rearrangement, and a modified Wittig reagent. This is a nitrogen Wittig reagent which is commonly called an aza Wittig reagent. It reacts just like the Wittig reagents you have seen before but produces an imine instead of an alkene when reacting with a ketone or aldehyde. There is no carbonyl at the start, so our only option is to do the Claisen rearrangement. This yields a molecule with a 4-membered ring and an aldehyde! So, we can now do the aza Wittig reaction. Comparing that imine product with the target 8-membered ring shows that we can do the reverse of our first step, a retro aza (because of the nitrogen) Claisen rearrangement, to generate the target. So, in the end it's just substituting NBn for O, but there's a lot going on to make that possible.

Reference: Organic Letters 2010

Exercise \(\PageIndex{11}\)

Propose a synthesis of the following target starting with any compounds containing six carbons or fewer. Feel free to ignore any carbons that might be in the alcohol protecting groups (PG). Hint: Retrosynthetic analysis should be very helpful.

Answer

Let's focus on retrons for this problem. The target contains a 1,4-enamide which is the retron for the Eschenmoser-Claisen rearrangement. Doing that retrosynthetic step reveals a second 1,4-enamide, so we can do another Eschenmoser-Claisen rearrangement. This yields a double amino enol ether that we can disconnect back to a 6-carbon diol and the ortho amide that we have used before for Claisen rearrangements.

For the synthesis part of the problem, all we need to do is heat the diol with excess ortho amide. This yields the allyl vinyl ether we need for the Eschenmoser-Claisen rearrangement, and the entire process can happen again to yield our target. An excellent example of creative synthetic planning!

Reference: Organic Letters 2010

Contributors

• Prof. Kevin Shea (Smith College)