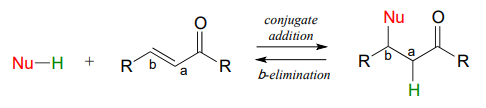
13.5: Conjugate Addition and Elimination
- Page ID
- 106375
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In this section, we will look at two more common biochemical reactions that proceed through enolate intermediates. In a typical conjugate addition, a nucleophile and a proton are 'added' to the two carbons of an alkene which is conjugated to a carbonyl (i.e. in the \(\alpha-\beta\) position). an \(\beta\)-elimination step, the reverse process occurs:
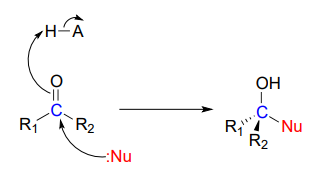
In chapter 9 we learned about nucleophilic carbonyl addition reactions, including the formation of hemiacetals, hemiketals, and imines. In all of these reactions, a nucleophile directly attacks a carbonyl carbon.
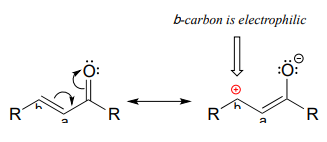
If, however, the electrophilic carbonyl is \(\beta\)-unsaturated - if, in other words, it contains a double bond conjugated to the carbonyl - a different reaction pathway is possible. A resonance structure can be drawn in which the \(\beta\)-carbon has a positive charge, meaning that the \(\beta\)-carbon also has the potential to be an electrophilic target.
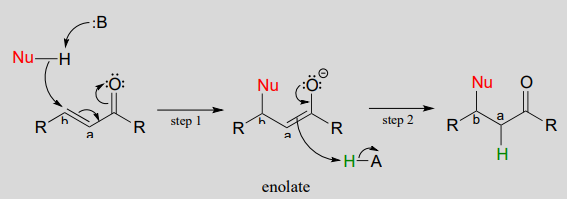
If a nucleophile attacks at the b-carbon, an enol or enolate intermediate results (step 1 below). In many cases this intermediate collapses and the a-carbon is protonated (step 2). This type of reaction is known as a conjugate addition.
Mechanism of a conjugate addition reaction
The reverse of a conjugate addition is a \(\beta\)-elimination, and is referred to mechanistically the abbreviation \(E1cb\).
Mechanism of an E1cB elimination
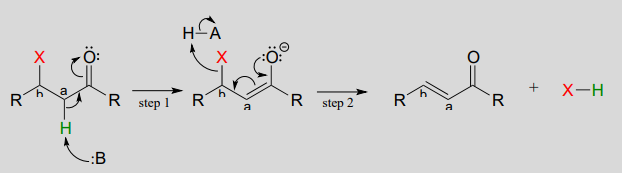
The \(E\) stands for 'elimination'; the numeral 1 refers to the fact that, like the \(S_N1\) mechanism, it is a stepwise reaction with first order kinetics. '\(cB\)' designation refers to the intermediate, which is the conjugate base of the starting compound. In step 1, an \(\alpha\)-carbon is deprotonated to produce an enolate, just like in aldol and Claisen reactions we have already seen. In step 2, the excess electron density on the enolate expels a leaving group at the \(\beta\) position (designated 'X' in the figure above). Notice that the \(\alpha\) and \(\beta\) carbons change from \(sp^3\) to \(sp^2\) hybridization with the formation of a conjugated double bond.
(In chapter 14 we will learn about alternate mechanisms for alkene addition and \(\beta\)-elimination reactions in which there is not an adjacent carbonyl (or imine) group, and in which the key intermediate species is a resonance-stabilized carbocation. )
Step II of fatty acid degradation is a conjugate addition of water, or hydration.
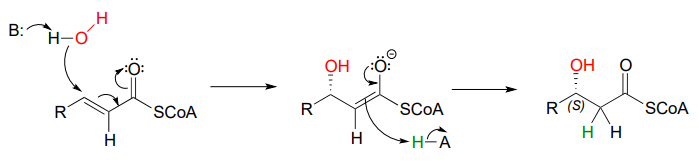
Note the specific stereochemical outcome: in the active site, the nucleophilic water is bound behind the plane of the conjugated system (as drawn in the figure above), and the result is \(S\) configuration in the \(\beta\)-hydroxy thioester product.
In step III of the fatty acid synthesis cycle we saw an \(E1cb\) \(\beta\)-elimination of water (dehydration):
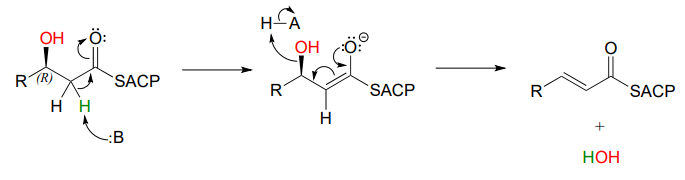
Notice that the stereochemistry at the \(\beta \)-carbon of the starting alcohol is R, whereas the hydration pathway (step II) reaction in the fatty acid degradation cycle pathway results in the \(S\) stereoisomer. These two reactions are not the reverse of one another!
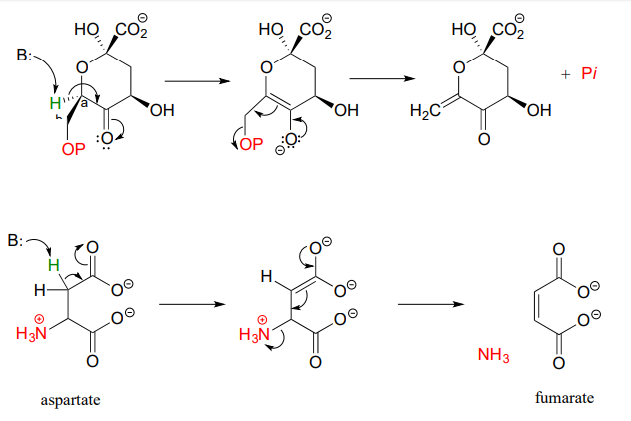
Here are two more examples of \(\beta \)-elimination reactions, with phosphate and ammonium respectively, as leaving groups. The first, 3-dehydroquinate synthase (EC 4.2.3.4) is part of the biosynthesis of aromatic amino acids, the second, aspartate ammonia lyase (EC 4.3.1.1) is part of amino acid catabolism.
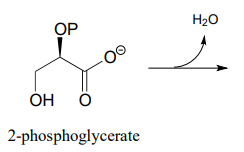
In the glycolysis pathway, the enzyme 'enolase' (EC 4.2.1.11) catalyzes the \(E1cb\) dehydration of 2-phosphoglycerate. Predict the product of this enzymatic step.
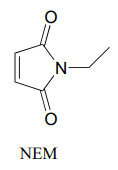
N-ethylmaleimide (NEM) is an irreversible inhibitor of many enzymes that contain active site cysteine residues. Inactivation occurs through conjugate addition of cysteine to NEM: show the structure of the labeled residue. (Michael addition)
Argininosuccinate lyase (4.3.2.1), an enzyme in the metabolic pathway that serves to eliminate nitrogen from your body in the form of urea in urine, catalyzes this \(\beta\)-elimination step:
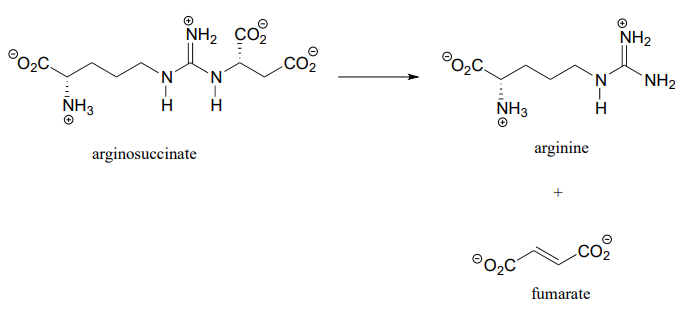
Propose a complete mechanism.
- Hint
-
Don't be intimidated by the size or complexity of the substrate - review the \(\beta\)-elimination mechanism, then identify the leaving group and breaking bond, the \(\alpha\)-carbon which loses a proton, the carbonyl that serves to stabilize the negatively-charged (enolate) intermediate, and the double bond that forms as a result of the elimination. You may want to designate an appropriate 'R' group to reduce the amount of drawing.


