8.4: Gas Laws
- Page ID
- 16103
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- To predict the properties of gases using the gas laws.
Experience has shown that several properties of a gas can be related to each other under certain conditions. The properties are pressure (P), volume (V), temperature (T, in kelvins), and amount of material expressed in moles (n). What we find is that a sample of gas cannot have any random values for these properties. Instead, only certain values, dictated by some simple mathematical relationships, will occur.
Boyle’s Law
The first simple relationship, referred to as a gas law, is between the pressure of a gas and its volume. If the amount of gas in a sample and its temperature are kept constant, then as the pressure of a gas is increased, the volume of the gas decreases proportionately. Mathematically, this is written as
\[\mathrm{P \propto \dfrac{1}{V}} \nonumber \]
where the “∝” symbol means “is proportional to.” This is one form of Boyle’s law, which relates the pressure of a gas to its volume.
A more useful form of Boyle’s law involves a change in conditions of a gas. For a given amount of gas at a constant temperature, if we know the initial pressure and volume of a gas sample and the pressure or volume changes, we can calculate what the new volume or pressure will be. That form of Boyle’s law is written
\[P_iV_i = P_fV_f \label{Eq1} \]
where the subscript \(i\) refers to initial conditions and the subscript \(f\) refers to final conditions.
To use \(\ref{Eq1}\), you need to know any three of the variables so that you can algebraically calculate the fourth variable. Also, the pressure quantities must have the same units, as must the two volume quantities. If the two similar variables don’t have the same variables, one value must be converted to the other value’s unit.
What happens to the volume of a gas if its pressure is increased? Assume all other conditions remain the same.
Solution
If the pressure of a gas is increased, the volume decreases in response.
What happens to the pressure of a gas if its volume is increased? Assume all other conditions remain the same.
- Answer
-
If the volume of a gas is increased, the pressure decreases.
If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is reduced to 0.987 atm? Assume that the amount and the temperature of the gas remain constant.
Solution
The key in problems like this is to be able to identify which quantities represent which variables from the relevant equation. The way the question is worded, you should be able to tell that 1.56 atm is Pi, 7.02 L is Vi, and 0.987 atm is Pf. What we are looking for is the final volume—Vf. Therefore, substituting these values into PiVi = PfVf:
(1.56 atm)(7.02 L) = (0.987 atm) × Vf
The expression has atmospheres on both sides of the equation, so they cancel algebraically:
(1.56)(7.02 L) = (0.987) × Vf
Now we divide both sides of the expression by 0.987 to isolate Vf, the quantity we are seeking:
\(\mathrm{\dfrac{(1.56)(7.02\: L)}{0.987}=V_f}\)
Performing the multiplication and division, we get the value of Vf, which is 11.1 L. The volume increases. This should make sense because the pressure decreases, so pressure and volume are inversely related.
If a sample of gas has an initial pressure of 3.66 atm and an initial volume of 11.8 L, what is the final pressure if the volume is reduced to 5.09 L? Assume that the amount and the temperature of the gas remain constant.
- Answer
-
8.48 atm
If the units of similar quantities are not the same, one of them must be converted to the other quantity’s units for the calculation to work out properly. It does not matter which quantity is converted to a different unit; the only thing that matters is that the conversion and subsequent algebra are performed properly. The following example illustrates this process.
If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is changed to 1,775 torr? Does the answer make sense? Assume that the amount and the temperature of the gas remain constant.
Solution
This example is similar to Example \(\PageIndex{2}\), except now the final pressure is expressed in torr. For the math to work out properly, one of the pressure values must be converted to the other unit. Let us change the initial pressure to torr:
\(\mathrm{1.56\: atm\times\dfrac{760\: torr}{1\: atm}=1,190\: torr}\)
Now we can use Boyle’s law:
(1,190 torr)(7.02 L) = (1,775 torr) × Vf
Torr cancels algebraically from both sides of the equation, leaving
(1,190)(7.02 L) = (1,775) × Vf
Now we divide both sides of the equation by 1,775 to isolate Vf on one side. Solving for the final volume,
\(\mathrm{V_f=\dfrac{(1,190)(7.02\: L)}{1,775}=4.71\: L}\)
Because the pressure increases, it makes sense that the volume decreases.
The answer for the final volume is essentially the same if we converted the 1,775 torr to atmospheres: \(\mathrm{1,775\: torr\times\dfrac{1\: atm}{760\: torr}=2.336\: atm}\). Using Boyle’s law: (1.56 atm)(7.02 L) = (2.335 atm) × Vf; \(\mathrm{V_f=\dfrac{(1.56\: atm)(7.02\: L)}{2.336\: atm}=4.69\: L}\).
If a sample of gas has an initial pressure of 375 torr and an initial volume of 7.02 L, what is the final pressure if the volume is changed to 4,577 mL? Does the answer make sense? Assume that amount and the temperature of the gas remain constant.
- Answer
-
575 torr
Breathing certainly is a major contribution to your health! Without breathing, we could not survive. Curiously, the act of breathing itself is little more than an application of Boyle’s law.
The lungs are a series of ever-narrowing tubes that end in a myriad of tiny sacs called alveoli. It is in the alveoli that oxygen from the air transfers to the bloodstream and carbon dioxide from the bloodstream transfers to the lungs for exhalation. For air to move in and out of the lungs, the pressure inside the lungs must change, forcing the lungs to change volume—just as predicted by Boyle’s law.
The pressure change is caused by the diaphragm, a muscle that covers the bottom of the lungs. When the diaphragm moves down, it expands the size of our lungs. When this happens, the air pressure inside our lungs decreases slightly. This causes new air to rush in, and we inhale. The pressure decrease is slight—only 3 torr, or about 0.4% of an atmosphere. We inhale only 0.5–1.0 L of air per normal breath.
Exhaling air requires that we relax the diaphragm, which pushes against the lungs and slightly decreases the volume of the lungs. This slightly increases the pressure of the air in the lungs, and air is forced out; we exhale. Only 1–2 torr of extra pressure is needed to exhale. So with every breath, our own bodies are performing an experimental test of Boyle’s law.
Charles’s Law
Another simple gas law relates the volume of a gas to its temperature. Experiments indicate that as the temperature of a gas sample is increased, its volume increases as long as the pressure and the amount of gas remain constant. The way to write this mathematically is
\[\mathrm V \propto T \nonumber \]
At this point, the concept of temperature must be clarified. Although the Kelvin scale is the preferred temperature scale, the Celsius scale is also a common temperature scale used in science. The Celsius scale is based on the melting and boiling points of water and is actually the common temperature scale used by most countries around the world (except for the United States, which still uses the Fahrenheit scale). The value of a Celsius temperature is directly related to its Kelvin value by a simple expression:
Kelvin temperature = Celsius temperature + 273
Thus, it is easy to convert from one temperature scale to another.
The Kelvin scale is sometimes referred to as the absolute scale because the zero point on the Kelvin scale is at absolute zero, the coldest possible temperature. On the other temperature scales, absolute zero is −260°C or −459°F.
The expression relating a gas volume to its temperature begs the following question: to which temperature scale is the volume of a gas related? The answer is that gas volumes are directly related to the Kelvin temperature. Therefore, the temperature of a gas sample should always be expressed in (or converted to) a Kelvin temperature.
What happens to the volume of a gas if its temperature is decreased? Assume that all other conditions remain constant.
SolutionIf the temperature of a gas sample is decreased, the volume decreases as well.
What happens to the temperature of a gas if its volume is increased? Assume that all other conditions remain constant.
- Answer
-
The temperature increases.
As with Boyle’s law, the relationship between volume and temperature can be expressed in terms of initial and final values of volume and temperature, as follows:
\[\mathrm{\dfrac{V_i}{T_i}=\dfrac{V_f}{T_f}} \nonumber \]
where Vi and Ti are the initial volume and temperature, and Vf and Tf are the final volume and temperature. This is Charles’s law. The restriction on its use is that the pressure of the gas and the amount of gas must remain constant. (Charles’s law is sometimes referred to as Gay-Lussac’s law, after the scientist who promoted Charles’s work.)
A gas sample at 20°C has an initial volume of 20.0 L. What is its volume if the temperature is changed to 60°C? Does the answer make sense? Assume that the pressure and the amount of the gas remain constant.
Solution
Although the temperatures are given in degrees Celsius, we must convert them to the kelvins before we can use Charles’s law. Thus,
20°C + 273 = 293 K = Ti 60°C + 273 = 333 K = Tf
Now we can substitute these values into Charles’s law, along with the initial volume of 20.0 L:
\(\mathrm{\dfrac{20.0\: L}{293\: K}=\dfrac{V_f}{333\: K}}\)
Multiplying the 333 K to the other side of the equation, we see that our temperature units will cancel:
\(\mathrm{\dfrac{(333\: K)(20.0\: L)}{293\: K}=V_f}\)
Solving for the final volume, Vf = 22.7 L. So, as the temperature is increased, the volume increases. This makes sense because volume is directly proportional to the absolute temperature (as long as the pressure and the amount of the remain constant).
A gas sample at 35°C has an initial volume of 5.06 L. What is its volume if the temperature is changed to −35°C? Does the answer make sense? Assume that the pressure and the amount of the gas remain constant.
- Answer
-
3.91 L
Combined Gas Law
Other gas laws can be constructed, but we will focus on only two more. The combined gas law brings Boyle’s and Charles’s laws together to relate pressure, volume, and temperature changes of a gas sample:
\[\mathrm{\dfrac{P_iV_i}{T_i}=\dfrac{P_fV_f}{T_f}} \nonumber \]
To apply this gas law, the amount of gas should remain constant. As with the other gas laws, the temperature must be expressed in kelvins, and the units on the similar quantities should be the same. Because of the dependence on three quantities at the same time, it is difficult to tell in advance what will happen to one property of a gas sample as two other properties change. The best way to know is to work it out mathematically.
A sample of gas has Pi = 1.50 atm, Vi = 10.5 L, and Ti = 300 K. What is the final volume if Pf = 0.750 atm and Tf = 350 K?
SolutionUsing the combined gas law, substitute for five of the quantities:
\(\mathrm{\dfrac{(1.50\: atm)(10.5\: L)}{300\: K}=\dfrac{(0.750\: atm)(V_f)}{350\: K}}\)
We algebraically rearrange this expression to isolate Vf on one side of the equation:
\(\mathrm{V_f=\dfrac{(1.50\: atm)(10.5\: L)(350\: K)}{(300\: K)(0.750\: atm)}=24.5\: L}\)
Note how all the units cancel except the unit for volume.
Exercise \(\PageIndex{6}\)
A sample of gas has Pi = 0.768 atm, Vi = 10.5 L, and Ti = 300 K. What is the final pressure if Vf = 7.85 L and Tf = 250 K?
- Answer
-
0.856 atm
A balloon containing a sample of gas has a temperature of 22°C and a pressure of 1.09 atm in an airport in Cleveland. The balloon has a volume of 1,070 mL. The balloon is transported by plane to Denver, where the temperature is 11°C and the pressure is 655 torr. What is the new volume of the balloon?
Solution
The first task is to convert all quantities to the proper and consistent units. The temperatures must be expressed in kelvins, and the pressure units are different so one of the quantities must be converted. Let us convert the atmospheres to torr:
22°C + 273 = 295 K = Ti
11°C + 273 = 284 K = Tf
\(\mathrm{1.09\: atm\times\dfrac{760\: torr}{1\: atm}=828\: torr = P_i}\)
Now we can substitute the quantities into the combined has law:
\(\mathrm{\dfrac{(828\: torr)(1,070\: mL)}{295\: K}=\dfrac{(655\: torr)\times V_f}{284\: K}}\)
To solve for Vf, we multiply the 284 K in the denominator of the right side into the numerator on the left, and we divide 655 torr in the numerator of the right side into the denominator on the left:
\(\mathrm{\dfrac{(828\: torr)(1,070\: mL)(284\: K)}{(295\: K)(655\: torr)}=V_f}\)
Notice that torr and kelvins cancel, as they are found in both the numerator and denominator. The only unit that remains is milliliters, which is a unit of volume. So Vf = 1,300 mL. The overall change is that the volume of the balloon has increased by 230 mL.
Exercise \(\PageIndex{7}\)
A balloon used to lift weather instruments into the atmosphere contains gas having a volume of 1,150 L on the ground, where the pressure is 0.977 atm and the temperature is 18°C. Aloft, this gas has a pressure of 6.88 torr and a temperature of −15°C. What is the new volume of the gas?
- Answer
-
110,038 L
The Ideal Gas Law
So far, the gas laws we have used have focused on changing one or more properties of the gas, such as its volume, pressure, or temperature. There is one gas law that relates all the independent properties of a gas under any particular condition, rather than a change in conditions. This gas law is called the ideal gas law. The formula of this law is as follows:
PV = nRT
In this equation, P is pressure, V is volume, n is amount of moles, and T is temperature. R is called the ideal gas law constant and is a proportionality constant that relates the values of pressure, volume, amount, and temperature of a gas sample. The variables in this equation do not have the subscripts i and f to indicate an initial condition and a final condition. The ideal gas law relates the four independent properties of a gas under any conditions.
The value of R depends on what units are used to express the other quantities. If volume is expressed in liters and pressure in atmospheres, then the proper value of R is as follows:
\[\mathrm{R=0.08205 \: \dfrac{L\cdot atm}{mol\cdot K}} \nonumber \]
This may seem like a strange unit, but that is what is required for the units to work out algebraically.
What is the volume in liters of 1.45 mol of N2 gas at 298 K and 3.995 atm?
SolutionUsing the ideal gas law where P = 3.995 atm, n = 1.45, and T = 298,
\(\mathrm{(3.995\: atm)\times V=(1.45\: mol)\left(0.08205\: \dfrac{L\cdot atm}{mol\cdot K}\right)(298\: K)}\)
On the right side, the moles and kelvins cancel. Also, because atmospheres appear in the numerator on both sides of the equation, they also cancel. The only remaining unit is liters, a unit of volume. So
3.995 × V = (1.45)(0.08205)(298) L
Dividing both sides of the equation by 3.995 and evaluating, we get V = 8.87 L. Note that the conditions of the gas are not changing. Rather, the ideal gas law allows us to determine what the fourth property of a gas (here, volume) must be if three other properties (here, amount, pressure, and temperature) are known.
Exercise \(\PageIndex{8}\)
What is the pressure of a sample of CO2 gas if 0.557 mol is held in a 20.0 L container at 451 K?
- Answer
-
1.03 atm
For convenience, scientists have selected 273 K (0°C) and 1.00 atm pressure as a set of standard conditions for gases. This combination of conditions is called standard temperature and pressure (STP). Under these conditions, 1 mol of any gas has about the same volume. We can use the ideal gas law to determine the volume of 1 mol of gas at STP:
\[\mathrm{(1.00\: atm)\times V=(1.00\: mol)\left(0.08205\: \dfrac{L\cdot atm}{mol\cdot K}\right)(273\: K)} \nonumber \]
This volume is 22.4 L. Because this volume is independent of the identity of a gas, the idea that 1 mol of gas has a volume of 22.4 L at STP makes a convenient conversion factor:
1 mol gas = 22.4 L (at STP)
Cyclopropane (C3H6) is a gas that formerly was used as an anesthetic. How many moles of gas are there in a 100.0 L sample if the gas is at STP?
Solution
We can set up a simple, one-step conversion that relates moles and liters:
\(\mathrm{100.0\: L\: C_3H_6\times \dfrac{1\: mol}{22.4\: L}=4.46\: mol\: C_3H_6}\)
There are almost 4.5 mol of gas in 100.0 L.
Note: Because of its flammability, cyclopropane is no longer used as an anesthetic gas.
Exercise \(\PageIndex{9}\)
Freon is a trade name for a series of fluorine- and chlorine-containing gases that formerly were used in refrigeration systems. What volume does 8.75 mol of Freon have at STP?
Note: Many gases known as Freon are no longer used because their presence in the atmosphere destroys the ozone layer, which protects us from ultraviolet light from the sun.
- Answer
-
196 L
Airbags (Figure \(\PageIndex{3}\)) are a safety feature provided in most automobiles since the 1990s. The effective operation of an airbag requires that it be rapidly inflated with an appropriate amount (volume) of gas when the vehicle is involved in a collision. This requirement is satisfied in many automotive airbag systems through use of explosive chemical reactions, one common choice being the decomposition of sodium azide, NaN3. When sensors in the vehicle detect a collision, an electrical current is passed through a carefully measured amount of NaN3 to initiate its decomposition:
This reaction is very rapid, generating gaseous nitrogen that can deploy and fully inflate a typical airbag in a fraction of a second (~0.03–0.1 s). Among many engineering considerations, the amount of sodium azide used must be appropriate for generating enough nitrogen gas to fully inflate the air bag and ensure its proper function. For example, a small mass (~100 g) of NaN3 will generate approximately 50 L of N2.

Dalton's Law of Partial Pressures
The ideal gas equation of state applies to mixtures just as to pure gases. It was in fact with a gas mixture, ordinary air, that Boyle, Gay-Lussac and Charles did their early experiments. The only new concept we need in order to deal with gas mixtures is the partial pressure, a concept invented by the famous English chemist John Dalton (1766-1844). Dalton reasoned that the low density and high compressibility of gases indicates that they consist mostly of empty space; from this it follows that when two or more different gases occupy the same volume, they behave entirely independently. The contribution that each component of a gaseous mixture makes to the total pressure of the gas is known as the partial pressure of that gas.
The definition of Dalton's Law of Partial Pressures that address this is:
The total pressure of a gas is the sum of the partial pressures of its components
which is expressed algebraically as
\[P_{total}=P_1+P_2+P_3 ... = \sum_i P_i \nonumber \]
or, equivalently
\[ P_{total} = \dfrac{RT}{V} \sum_i n_i \nonumber \]
There is also a similar relationship based on volume fractions, known as Amagat's law of partial volumes. It is exactly analogous to Dalton's law, in that it states that the total volume of a mixture is just the sum of the partial volumes of its components. But there are two important differences: Amagat's law holds only for ideal gases which must all be at the same temperature and pressure. Dalton's law has neither of these restrictions. Although Amagat's law seems intuitively obvious, it sometimes proves useful in chemical engineering applications. We will make no use of it in this course.
Three flasks having different volumes and containing different gases at various pressures are connected by stopcocks as shown. When the stopcocks are opened,
- What will be the pressure in the system?
- Which gas will be most abundant in the mixture?
Assume that the temperature is uniform and that the volume of the connecting tubes is negligible.
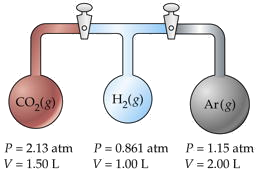
Solution
The trick here is to note that the total number of moles nT and the temperature remain unchanged, so we can make use of Boyle's law PV = constant. We will work out the details for CO2 only, denoted by subscripts a.
For CO2,
\[P_aV_a = (2.13\; atm)(1.50\; L) = 3.20\; L \cdot atm \nonumber \]
Adding the PV products for each separate container, we obtain
\[\sum_i P_iV_i = 6.36\; L \cdot atm = n_T RT \nonumber \]
We will call this sum P1V1. After the stopcocks have been opened and the gases mix, the new conditions are denoted by P2V2.
From Boyle's law (\(\ref{Eq1}\),
\[P_1V_1 = P_2V_2 = 6.36\; L \cdot atm \nonumber \]
\[V_2 = \sum_i V_i = 4.50\; L \nonumber \]
Solving for the final pressure P2 we obtain (6.36 L-atm)/(4.50 L) = 1.41 atm.
For part (b), note that the number of moles of each gas is n = PV/RT. The mole fraction of any one gas is Xi = ni /nT . For CO2, this works out to (3.19/RT) / (6.36/RT) = 0.501. Because this exceeds 0.5, we know that this is the most abundant gas in the final mixture.
Dalton’s law states that in a gas mixture (\(P_{total}\)) each gas will exert a pressure independent of the other gases (\(P_n\)) and each gas will behave as if it alone occupies the total volume. By extension, the partial pressure of each gas can be calculated by multiplying the total pressure (\(P_{total}\)) by the gas percentage (%).
\[P_{Total} = P_1 + P_2 + P_3 + P_4 + ... + P_n \nonumber \]
or
\[P_n = \dfrac{\text{% of individual gas}_n}{P_{Total}} \nonumber \]
| Gas | Partial Pressure (mm Hg) | Percentage (%) |
|---|---|---|
| Nitrogen, (N_2\) | \(P_{N_2}\) = 594 | 78 |
| Oxygen, \(O_2\) | \(P_{O_2}\)= 160 | 21 |
| Carbon Dioxide, \(CO_2\) | \(P_{CO_2}\) = 0.25 | 0.033 |
| Water Vapor, \(H_2O\) | \(P_{H_2O}\) = 5.7 | 0.75 |
| Other trace gases | \(P_{Other}\) = 0.05 | 0.22 |
| Total air | \(P_{Total}\) = 760 | 1 |
Application of Dalton's Law: Collecting Gases over Water
A common laboratory method of collecting the gaseous product of a chemical reaction is to conduct it into an inverted tube or bottle filled with water, the opening of which is immersed in a larger container of water. This arrangement is called a pneumatic trough, and was widely used in the early days of chemistry. As the gas enters the bottle it displaces the water and becomes trapped in the upper part.
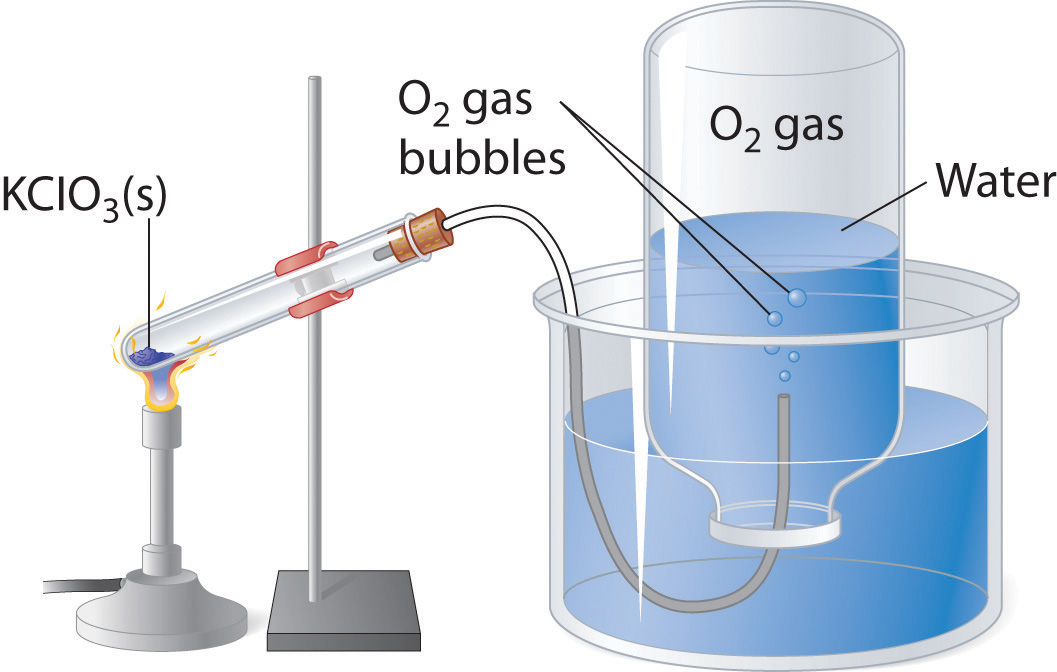
The volume of the gas can be observed by means of a calibrated scale on the bottle, but what about its pressure? The total pressure confining the gas is just that of the atmosphere transmitting its force through the water. (An exact calculation would also have to take into account the height of the water column in the inverted tube.) But liquid water itself is always in equilibrium with its vapor, so the space in the top of the tube is a mixture of two gases: the gas being collected, and gaseous H2O. The partial pressure of H2O is known as the vapor pressure of water and it depends on the temperature. In order to determine the quantity of gas we have collected, we must use Dalton's Law to find the partial pressure of that gas.
Oxygen gas was collected over water as shown above. The atmospheric pressure was 754 torr, the temperature was 22°C, and the volume of the gas was 155 mL. The vapor pressure of water at 22°C is 19.8 torr. Use this information to estimate the number of moles of \(O_2\) produced.
Solution
From Dalton's law,
\[P_{O_2} = P_{total} – P_{H_2O} = 754 – 19.8 = 734 \; torr = 0.966\; atm \nonumber \]
Now use the Ideal Gas Law to convert to moles
\[ n =\dfrac{PV}{RT} = \dfrac{(0.966\; atm)(0.155\;L)}{(0.082\; L atm mol^{-1} K^{-1})(295\; K)}= 0.00619 \; mol \nonumber \]
Henry’s Law
Henry's law is one of the gas laws formulated by William Henry in 1803. It states: "At a constant temperature, the amount of a given gas that dissolves in a given type and volume of liquid is directly proportional to the partial pressure of that gas in equilibrium with that liquid." An equivalent way of stating the law is that the solubility of a gas in a liquid is directly proportional to the partial pressure of the gas above the liquid.
To explain this law, Henry derived the equation:
\[ C =k P_{gas} \nonumber \]
where
Henry’s Law tells us that the greater the pressure of gas above the surface of a liquid, the higher the concentration of the gas in the liquid. Also, Henry’s law tells us that gases diffuse from areas of high gas concentration to areas of low gas concentration.
- Henry's law only works if the molecules are at equilibrium.
- Henry's law does not work for gases at high pressures (e.g., \(N_{2\;(g)}\) at high pressure becomes very soluble and harmful when in the blood supply).
- Henry's law does not work if there is a chemical reaction between the solute and solvent (e.g., \(HCl_{(g)}\) reacts with water by a dissociation reaction to generate \(H_3O^+\) and \(Cl^-\) ions).
Application of Henry's Law: Scuba diving
Our respiratory systems are designed to maintain the proper oxygen concentration in the blood when the partial pressure of O2 is 0.21 atm, its normal sea-level value. Below the water surface, the pressure increases by 1 atm for each 10.3 m increase in depth; thus a scuba diver at 10.3 m experiences a total of 2 atm pressure pressing on the body. In order to prevent the lungs from collapsing, the air the diver breathes should also be at about the same pressure.

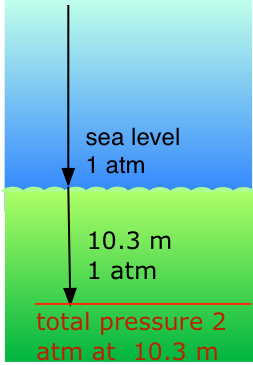
But at a total pressure of 2 atm, the partial pressure of \(O_2\) in ordinary air would be 0.42 atm; at a depth of 100 ft (about 30 m), the \(O_2\) pressure of 0.8 atm would be far too high for health. For this reason, the air mixture in the pressurized tanks that scuba divers wear must contain a smaller fraction of \(O_2\). This can be achieved most simply by raising the nitrogen content, but high partial pressures of N2 can also be dangerous, resulting in a condition known as nitrogen narcosis. The preferred diluting agent for sustained deep diving is helium, which has very little tendency to dissolve in the blood even at high pressures.
Certain diseases—such as emphysema, lung cancer, and severe asthma—primarily affect the lungs. Respiratory therapists help patients with breathing-related problems. They can evaluate, help diagnose, and treat breathing disorders and even help provide emergency assistance in acute illness where breathing is compromised.
Most respiratory therapists must complete at least two years of college and earn an associate’s degree, although therapists can assume more responsibility if they have a college degree. Therapists must also pass state or national certification exams. Once certified, respiratory therapists can work in hospitals, doctor’s offices, nursing homes, or patient’s homes. Therapists work with equipment such as oxygen tanks and respirators, may sometimes dispense medication to aid in breathing, perform tests, and educate patients in breathing exercises and other therapy.
Because respiratory therapists work directly with patients, the ability to work well with others is a must for this career. It is an important job because it deals with one of the most crucial functions of the body.
Key Takeaway
- The physical properties of gases are predictable using mathematical formulas known as gas laws.
- \(C\) is the solubility of a gas at a fixed temperature in a particular solvent (in units of M or mL gas/L)
- \(k\) is Henry's law constant (often in units of M/atm)
- \(P_{gas}\) is the partial pressure of the gas (often in units of Atm)


