1.15: Vibrational Spectroscopy of Linear Molecules
- Page ID
- 204716
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Groups with axial symmetry are also known as continuous groups due to the infinite amount of rotations and reflections that leave the molecule invariant. Due to the sheer number of symmetry operations, determining irreducible representations is not feasible. There is one aspect of group theory that can be taken advantage of and these are the group, subgroup relationships.
Groups and Subgroups
A subgroup is a set of elements within a group that also form their own group, such that every operation in the subgroup stays in the subgroup. Consider a concrete example. The \({C_{2h}}\) point group contains 4 elements: {\(E\), \(C_2\), \(i\), \(σ\)}. However, the elements \(E\) and \(C_2\) form the \(C_2\) group. Hence \(C_2\) is a point group of the \(C_{2h}\) point group, and this is designated as, \({ C_{2}\subseteq C_{2h}}\).
The character tables of subgroups are appropriately reduced down. Consider \(D_{4h}\). We know that \(C_{4v}\) is a subgroup of \(D_{4h}\). On Figure 2, the C4v elements are boxed with their respective characters. Notice that \(D_{4h}\) has many more possible irreducible representations. However, there are different irreducible representations in \(D_{4h}\) that become identical due to the fact that the characters are the same with the elements of the \(C_{4v}\) point group. Conversely, degenerate irreducible reps may become nondegenerate.
One has to figure out properly classify the irreducible representations. For example, \(A_{1g}\) and \(A_{2u}\) in \(D_{4h}\) are both \(A_1\) in \(C_{4v}\). The complete relationship between different irreducible representations is a correlation diagram and essentially matches identical sets of characters to one another.
The importance of this is that a physical property, i.e. a wavefunction, that serves as a basis for one of the irreducible representations for a point group will transform as its correlated irreducible representation in its subgroup.
Subgroups of Linear Molecules
The electronic energy terms of a linear molecule are classified by the angular momentum that lies along the principal axis of symmetry. The physical reason for this is that only angular momentum about the axis is conserved around the axis. Therefore, only the m quantum number classifies a linear molecule. In analogy with s,p,d.., the new designations for the energy levels are \(Σ\), \(Π\), \(Δ\), etc. A (\(±\)) subscript is reserved for \(Σ\) energies which have no angular momentum about the axis, so it designates between wavefunctions symmetric and antisymmetric with respect to reflection about the principal axis. \(g\) and \(u\) designate wavefunctions that are symmetric and antisymmetric with respect to inversion. In order to completely determine energy levels, one must Figure out how reflections, inversion, and rotations affect the Hamiltonian of a wavefunction. The various symmetry operations produce physically distinct, but degenerate, energy levels. This method of analysis is free from approximations from molecular orbitals, but molecular orbitals of diatomic molecules must still adhere to these symmetry rules hence a pattern.
The important aspect of this discussion is that these physically realized energy levels help simplify the issue of dealing with an infinite point group. There are an infinite amount of subgroups but the correct subgroup are point groups with two fold rotational symmetry. For a \({ D_{\infty h}}\), the appropriate subgroup is a \({ D_{2h}}\), and for a \({ C_{\infty v}}\), the appropriate subgroup is a \({ C_{2v}}\).
The technique for solving the irreducible representations of linear groups is straightforward.
- Identify the point group of the molecule and then treat the point group as the appropriate subgroup as designated in the above tables.
- Solve for the reducible as the subgroup and reduce down to the irreducible representations.
- Use the correlation diagram to convert back to the proper form and then pull out relevant physical information.
Vibrations of Linear Molecules
The continuous rotation of linear molecules is not physically meaningful as a degree of freedom, hence the total number of vibrational degrees of freedom is \(3N-5\), subtracting away two rotational degrees of freedom instead of 3. Using the method outlined above, determine the molecule’s irreducible representation in the corresponding two-fold rotation group and then convert back to the infinite point group. Because the correlation diagram links between irreducible representations that transform the same way, one can use the irreducible representations in the two-fold rotation group to Figure out which ones are IR and Raman active.
Both infrared and electron diffraction studies have argue that carbon suboxide (\(\ce{C_{3}O_{2}}\)) has a bent structure in the gas phase, however, it exhibits an average linear geometry in the solid phase and would be in the \(D_{ꝏh}\) point group.
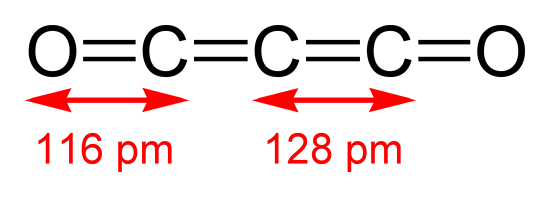
Solving this as a \(D_{2h}\) point group just as we solve a vibrational problem, by appropriately tossing out translational and rotational degrees of freedom, one can arrive at an irreducible representation of
\[2A_g + 2B_{2g} + 2B_{3g} + 3B_{1u} +3B_{2u} + 3B_{3u}. \nonumber \]
Using the correlation table above, we find that the true irreducible representation is actually
\[ 2\Sigma _{g}^{+} + \Pi _{g} + 2\Sigma _{u}^{+} + 2\Pi _{u}. \nonumber \]
- \(\Sigma _{g}^{+}\) transforms as \({ A_{g}}\)
- \({ \Pi _{g}}\) transforms as both \({ B_{2g}}\) and \({ B_{3g}}\).
Therefore \({ A_{g},B_{2g},}\) and \({ B_{3g}}\) are all Raman active.
- \({ \Sigma _{u}^{+}}\) transforms as \({ B_{1u}}\)
- \({ \Pi _{u}}\) transforms as \({ B_{2u}}\) and \({ B_{3u}}\).
Therefore \({ B_{1u},B_{2u}}\), and \({ B_{3u}}\) are IR active.
Making these correlations allow us to effectively treat this molecule as a typical vibrational problem and pull out all the same relevant information.


