9.11: The Strange Case of the Alkali Oxides
- Page ID
- 189354
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The alkali oxides, made by reacting alkali metals (Li, Na, K, Rb, Cs) with oxygen, show an unusual trend. When lithium reacts with oxygen we obtain the binary oxide Li2O, as expected from combining an element in group I with one in group VI. Curiously, the oxide that forms most readily when sodium metal is oxidized is not Na2O, but is instead the peroxide Na2O2, which we can formulate as (Na+)2(O22-). With potassium, rubidium, and cesium we obtain the superoxides MO2, which contain the superoxide radical anion (O2-.) and should be formulated as (M+)(O2-). While it is possible to make Na2O, K2O, Rb2O, and Cs2O by reaction of the appropriate metal nitrate (MNO3) with elemental alkali metal M,[16] it is curious that these "normal valent" compounds do not form by direct reaction of the metal with oxygen.
|
Sodium metal is oxided in air to sodium peroxide, Na2O2 |
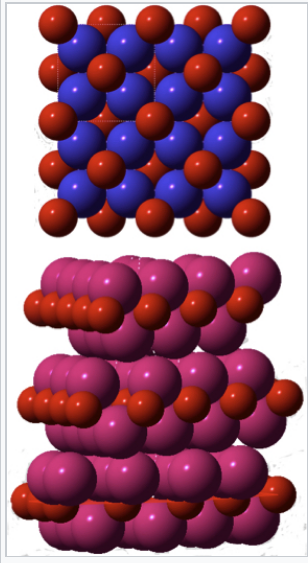
Because the alkali metals are all very electropositive (χ = 0.8-1.0), and oxygen is very electronegative (χ = 3.5), we expect all the compounds we make by combining them to be reliably ionic. Consistent with this picture we find that Li2O (along with Na2O, K2O, and Rb2O) adopts the antifluorite structure (8:4 coordination - see problem 8.8.2), which we expect to find with relatively ionic M2X compounds. Strangely however, Cs2O crystallizes in the anti-CdCl2 structure. This is odd because CdCl2 has a layered structure that we normally associate with polar covalent MX2 compounds (see section 8.4). In Cs2O, six Cs+ cations surround each O2- anion in an octahedron. Each Cs+ is coordinated to three O2- ions, and the Cs+ ions contact each other across a van der Waals gap. The juxtaposition of Cs+ ions near each other is clearly electrostatically unfavorable, so why does Cs2O prefer the anti-CdCl2 structure to antifluorite?
The answer has to do with the crowding of alkali ions around oxygen, as illustrated for K2O at the right. Because eight large K+ ions surround each O2- ion in the structure, the cations are essentially in contact. Indeed, the metal-oxygen bonds are "stretched" in Na2O, K2O, and Rb2O relative to M-O bonds with the same bond order in other structures.[17] The situation is so extreme for Cs2O that it finds an (electrostatically unfavorable) structure in which the coordination is lowered to 6:3. This packing problem is relieved somewhat in the peroxides, where the coordination is still 8:4 but the anion is larger, and especially in the superoxides where the cation:anion ratio is 1:1 and the coordination is 6:6. Thus the larger alkali ions (K+, Rb+, Cs+) tend to form superoxides.
|
Space-filling models of the crystal structures of K2O (top) and Cs2O (bottom). Oxygen atoms are red, potassium ions are blue, and cesium ions are magenta |
Another way that we can rationalize this trend is through the energetics of forming the oxides, peroxides, and superoxides.
Let's calculate the enthalpy change (per mole of metal) for forming a metal oxide M2O from the metal and oxygen:
\(\ce{M_{(s)} + \frac{1}{4} O2 _{(g)} -> \frac{1}{2} M2O_{(s)}}\)
We can use Hess' law to write this as a sum of reactions:
| Reaction | ΔH |
|---|---|
| \(\ce{M_{(s)}-> M_{(g)} -> M^{+}_{(g)}}\) | \(\Delta_{s} + IE = \Delta_{f, M^{+} (g)}\) |
| \(\ce{\frac{1}{4} O2_{(g)} -> \frac{1}{2}O_{(g)} -> \frac{1}{2} O^{2-}}\) | \(\frac{1}{4} \Delta H_{d} + \frac{1}{2} EA_{1} + \frac{1}{2}EA_{2} = \frac{1}{2} \Delta H_{f, O^{2-} (g)}\) |
| \(\ce{M^{+}_{(g)} + \frac{1}{2}O^{2-}_{(g)} -> \frac{1}{2}M2O_{(s)}}\) | \(\frac{1}{2} E_{L, M_{2}O} - \frac{3}{2}RT\) |
| Overall: | |
| \(\ce{M_{(s)} + \frac{1}{4}O2_{(g)} -> \frac{1}{2}M2O_{(s)}}\) | \(\Delta H_{f, M^{+}(g)} + \frac{1}{2} \Delta H_{f, O^{2-} (g)} + \frac{1}{2} E_{L, M_{2}O} -\frac{3}{2} RT\) |
To get the enthalpy change for the overall reaction (the heat of formation of 1/2 mole of M2O) we will need the heats of formation of M+(g) and O2-(g), which are available from tabulated values, and EL, which we can calculate from Kapustinskii's equation.
Similarly, we can write for the formation of the alkali peroxides:
\(\ce{M_{(s)} + \frac{1}{2}O2_{(g)} -> \frac{1}{2}M2O2_{(s)}}\)
Reaction
| Reaction | ΔH |
|---|---|
| \(\ce{M_{(s)} -> M_{(g)} -> M^{+}_{(g)}}\) | \(\Delta H _{(f, M^{+} (g)}\) |
| \(\ce{\frac{1}{2} O2_{(g)} -> \frac{1}{2}O2^{2-}_{(g)}}\) | \(\frac{1}{2} \Delta H_{f, O_{2}^{2-} (g)}\) |
| \(\ce{M^{+}_{(g)} + \frac{1}{2} O2^{2-}_{(g)} -> \frac{1}{2} M2O2_{(s)}}\) | \(\frac{1}{2} E_{L, M_{2}O_{2}} - \frac{3}{2} RT\) |
| Overall: | |
| \(\ce{M_{(s)} + \frac{1}{2}O2_{(g)} ->\frac{1}{2}M2O2_{(s)}}\) | \(\Delta H_{f, M^{+} (g)} + \frac{1}{2} \Delta H_{f, O_{2}^{2-} (g)} + \frac{1}{2} E_{L, M_{2}O_{2}} - \frac{3}{2} RT\) |
and for the superoxides:
\(\ce{M_{(s)} + O2_{(g)} -> MO2_{(s)}}\)
| Reaction | ΔH |
|---|---|
| \(\ce{M_{(s)} -> M_{(g)} -> M^{+}_{(g)}}\) | \(\Delta H_{f, M^{+} (g)} \) |
| \(\ce{O2_{(g)} -> O2^{-}_{(g)}}\) | \(\Delta H_{f, O_{2}^{-} (g)}\) |
| \(\ce{M^{+}_{(g)} + O2^{-}_{(g)} -> MO2_{(s)}}\) | \(E_{L, MO_{2}} - 2RT\) |
| Overall: | |
| \(\ce{M_{(s)} + O2_{(g)} -> MO2_{(s)} }\) | \(\Delta H_{f, M^{+} (g)} + \Delta H_{(f, O_{2}^{-}(g)} + E_{L, MO_{2}} - 2RT\) |
For the gaseous anions and cations, we have the following heats of formation and ionic radii (CN=6):
| Ion | ΔHf, kJ | ionic radius, Å |
|---|---|---|
| Li+ | 678 | 0.76 |
| Na+ | 602 | 1.02 |
| K+ | 506 | 1.38 |
| Rb+ | 485 | 1.52 |
| Cs+ | 473 | 1.67 |
| O2- | 500 | 1.20 |
| O22- | 519 | 1.59 |
| O2- | -88 | 1.49 |
Now using Kapustinskii's equation, we can calculate the lattice energies for each compound; these have been converted to lattice enthalpies by subtracting 2 RT or 3 RT as appropriate:
\[\mathbf{E}_{L} = \frac{1213.8z_{+}z_{-}n}{r_{+}+r_{-}}(1 - \frac{0.345}{r_{+}+r_{-}})\]
| Metal | ΔHL,M2O | ΔHL,M2O2 | ΔHL,MO2 |
|---|---|---|---|
| Li | -3,065 kJ | -2,651 kJ | -918 kJ |
| Na | -2,776 | -2,433 | -838 |
| K | -2,454 | -2,178 | -751 |
| Rb | -2,345 | -2,090 | -721 |
| Cs | -2,241 | -2,007 | -678 |
As expected, the lattice energies for M2O and M2O2 are comparable, the latter being somewhat smaller in magnitude because of the larger size of the O22- anion. The lattice energies of the superoxides, MO2, are about 1/3 those of the corresponding peroxides because both the anion and cation are singly charged, and there are only two ions per formula unit.
Now, putting it all together, we can use the lattice energies and heats of formation of the individual ions to compare the heats of formation (per mole of metal) of each of the oxides:
| Metal | 1/2 ΔHf,M2O | 1/2 ΔHf,M2O2 | ΔHf,MO2 |
|---|---|---|---|
| Li | -404 kJ | -388 kJ | -328 kJ |
| Na | -338 | -354 | -324 |
| K | -271 | -321 | -328 |
| Rb | -241 | -300 | -324 |
| Cs | -53 | -70 | -81 |
We can see that for Li, the formation of Li2O is favored over Li2O2 or LiO2 because of the very favorable lattice energy of Li2O. As the lattice energy becomes less negative with increasing cation size, the peroxide becomes the most stable at Na. For the heavier alkalis, M2O becomes quite unstable and the superoxides MO2 are the most stable. This is consistent with our observations of the chemistry of the group I oxides.
Metal-air batteries
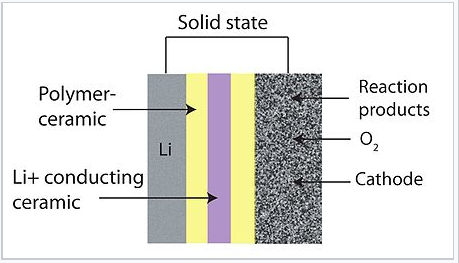
The alkali oxides are quite interesting in the context of metal-air batteries because of their potential for extremely high energy storage on a mass basis. Such batteries have alkali metal (typically Li) or Zn anodes and utilize oxygen from the air at the cathode. Although lithium is the lightest and therefore the most energy-dense alkali metal, there are materials problems associated with the formation of Li dendrites when the battery is recharged, and also with the slow kinetics of the four-electron interconversion between O2(g) and 2 O2- at the cathode. For this reason, superoxide batteries are currently being studied as alternatives. The one-electron cathode reaction O2 + e- = O2- is kinetically fast, and potassium[18] and sodium[19] represent potentially viable alternatives to lithium for the anode of these air-breathing batteries. Recently, it has been shown that LiO2 can be kinetically stabilized by template growth on iridium nanoparticles, potentially opening the door to very high energy density lithium-air batteries.[20]
|
Schematic of an air-breathing lithium battery |