2.7: Ions and Ionic Compounds
- Page ID
- 21703
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Explain the bonding nature of ionic compounds.
- Relating microscopic bonding properties to macroscopic solid properties.
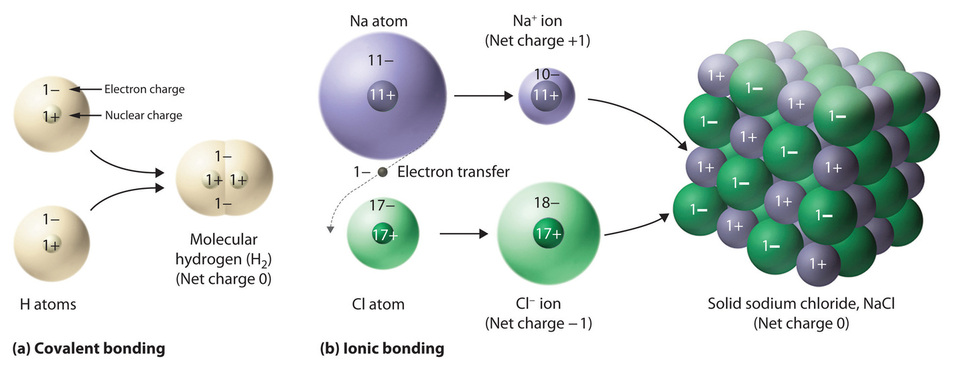
The substances described in the preceding discussion are composed of molecules that are electrically neutral; that is, the number of positively-charged protons in the nucleus is equal to the number of negatively-charged electrons. In contrast, ions are atoms or assemblies of atoms that have a net electrical charge. Ions that contain fewer electrons than protons have a net positive charge and are called cations. Conversely, ions that contain more electrons than protons have a net negative charge and are called anions. Ionic compounds contain both cations and anions in a ratio that results in no net electrical charge.
In covalent compounds, electrons are shared between bonded atoms and are simultaneously attracted to more than one nucleus. In contrast, ionic compounds contain cations and anions rather than discrete neutral molecules. Ionic compounds are held together by the attractive electrostatic interactions between cations and anions. In an ionic compound, the cations and anions are arranged in space to form an extended three-dimensional array that maximizes the number of attractive electrostatic interactions and minimizes the number of repulsive electrostatic interactions (Figure \(\PageIndex{1}\)). As shown in Equation \(\ref{Eq1}\), the electrostatic energy of the interaction between two charged particles is proportional to the product of the charges on the particles and inversely proportional to the distance between them:
\[ \text {electrostatic energy} \propto {Q_1Q_2 \over r} \label{Eq1} \]
where \(Q_1\) and \(Q_2\) are the electrical charges on particles 1 and 2, and \(r\) is the distance between them. When \(Q_1\) and \(Q_2\) are both positive, corresponding to the charges on cations, the cations repel each other and the electrostatic energy is positive. When \(Q_1\) and \(Q_2\) are both negative, corresponding to the charges on anions, the anions repel each other and the electrostatic energy is again positive. The electrostatic energy is negative only when the charges have opposite signs; that is, positively charged species are attracted to negatively charged species and vice versa.
Ionic compounds contain both cations and anions in a ratio that results in zero electrical charge.
As shown in Figure \(\PageIndex{2}\), the strength of the interaction is proportional to the magnitude of the charges and decreases as the distance between the particles increases. These energetic factors are discussed in greater quantitative detail later.

If the electrostatic energy is positive, the particles repel each other; if the electrostatic energy is negative, the particles are attracted to each other.
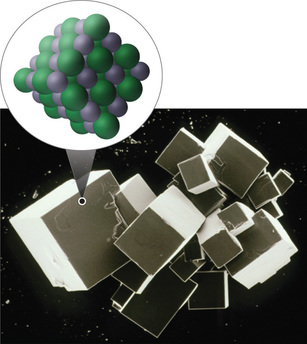
One example of an ionic compound is sodium chloride (NaCl; Figure \(\PageIndex{3}\)), formed from sodium and chlorine. In forming chemical compounds, many elements have a tendency to gain or lose enough electrons to attain the same number of electrons as the noble gas closest to them in the periodic table. When sodium and chlorine come into contact, each sodium atom gives up an electron to become a Na+ ion, with 11 protons in its nucleus but only 10 electrons (like neon), and each chlorine atom gains an electron to become a Cl− ion, with 17 protons in its nucleus and 18 electrons (like argon), as shown in part (b) in Figure \(\PageIndex{1}\). Solid sodium chloride contains equal numbers of cations (Na+) and anions (Cl−), thus maintaining electrical neutrality. Each Na+ ion is surrounded by 6 Cl− ions, and each Cl− ion is surrounded by 6 Na+ ions. Because of the large number of attractive Na+Cl− interactions, the total attractive electrostatic energy in NaCl is great.
Consistent with a tendency to have the same number of electrons as the nearest noble gas, when forming ions, elements in groups 1, 2, and 3 tend to lose one, two, and three electrons, respectively, to form cations, such as Na+ and Mg2+. They then have the same number of electrons as the nearest noble gas: neon. Similarly, K+, Ca2+, and Sc3+ have 18 electrons each, like the nearest noble gas: argon. In addition, the elements in group 13 lose three electrons to form cations, such as Al3+, again attaining the same number of electrons as the noble gas closest to them in the periodic table. Because the lanthanides and actinides formally belong to group 3, the most common ion formed by these elements is M3+, where M represents the metal. Conversely, elements in groups 17, 16, and 15 often react to gain one, two, and three electrons, respectively, to form ions such as Cl−, S2−, and P3−. Ions such as these, which contain only a single atom, are called monatomic ions. The charges of most monatomic ions derived from the main group elements can be predicted by simply looking at the periodic table and counting how many columns an element lies from the extreme left or right. For example, barium (in Group 2) forms Ba2+ to have the same number of electrons as its nearest noble gas, xenon; oxygen (in Group 16) forms O2− to have the same number of electrons as neon; and cesium (in Group 1) forms Cs+, which has the same number of electrons as xenon. Note that this method is ineffective for most of the transition metals. Some common monatomic ions are listed in Table \(\PageIndex{1}\).
Elements in Groups 1, 2, and 3 tend to form 1+, 2+, and 3+ ions, respectively; elements in Groups 15, 16, and 17 tend to form 3−, 2−, and 1− ions, respectively.
| Group 1 | Group 2 | Group 3 | Group 13 | Group 15 | Group 16 | Group 17 |
|---|---|---|---|---|---|---|
| Li+ lithium | Be2+ beryllium | N3− nitride (azide) | O2− oxide | F− fluoride | ||
| Na+ sodium | Mg2+ magnesium | Al3+ aluminum | P3− phosphide | S2− sulfide | Cl− chloride | |
| K+ potassium | Ca2+ calcium | Sc3+ scandium | Ga3+ gallium | As3− arsenide | Se2− selenide | Br− bromide |
| Rb+ rubidium | Sr2+ strontium | Y3+ yttrium | In3+ indium | Te2− telluride | I− iodide | |
| Cs+ cesium | Ba2+ barium | La3+ lanthanum |
Predict the charge on the most common monatomic ion formed by each element.
- aluminum, used in the quantum logic clock, the world’s most precise clock
- selenium, used to make ruby-colored glass
- yttrium, used to make high-performance spark plugs
Given: element
Asked for: ionic charge
Strategy:
- Identify the group in the periodic table to which the element belongs. Based on its location in the periodic table, decide whether the element is a metal, which tends to lose electrons; a nonmetal, which tends to gain electrons; or a semimetal, which can do either.
- After locating the noble gas that is closest to the element, determine the number of electrons the element must gain or lose to have the same number of electrons as the nearest noble gas.
Solution:
- A Aluminum is a metal in group 13; consequently, it will tend to lose electrons. B The nearest noble gas to aluminum is neon. Aluminum will lose three electrons to form the Al3+ ion, which has the same number of electrons as neon.
- A Selenium is a nonmetal in group 16, so it will tend to gain electrons. B The nearest noble gas is krypton, so we predict that selenium will gain two electrons to form the Se2− ion, which has the same number of electrons as krypton.
- A Yttrium is in group 3, and elements in this group are metals that tend to lose electrons. B The nearest noble gas to yttrium is krypton, so yttrium is predicted to lose three electrons to form Y3+, which has the same number of electrons as krypton.
Predict the charge on the most common monatomic ion formed by each element.
- calcium, used to prevent osteoporosis
- iodine, required for the synthesis of thyroid hormones
- zirconium, widely used in nuclear reactors
- Answer a
-
Ca2+
- Answer b
-
I−
- Answer c
-
Zr4+
Ions of Atoms: Ions of Atoms, YouTube(opens in new window) [youtu.be]
Physical Properties of Ionic and Covalent Compounds
In general, ionic and covalent compounds have different physical properties. Ionic compounds form hard crystalline solids that melt at high temperatures and are resistant to evaporation. These properties stem from the characteristic internal structure of an ionic solid, illustrated schematically in Figure \(\PageIndex{4a}\) which shows the three-dimensional array of alternating positive and negative ions held together by strong electrostatic attractions. In contrast, as shown in Figure \(\PageIndex{4b}\) most covalent compounds consist of discrete molecules held together by comparatively weak intermolecular forces (the forces between molecules), even though the atoms within each molecule are held together by strong intramolecular covalent bonds (the forces within the molecule).

Covalent substances can be gases, liquids, or solids at room temperature and pressure, depending on the strength of the intermolecular interactions. Covalent molecular solids tend to form soft crystals that melt at low temperatures and evaporate easily. Some covalent substances, however, are not molecular but consist of infinite three-dimensional arrays of covalently bonded atoms and include some of the hardest materials known, such as diamond. This topic will be addressed elsewhere. The covalent bonds that hold the atoms together in the molecules are unaffected when covalent substances melt or evaporate, so a liquid or vapor of independent molecules is formed. For example, at room temperature, methane, the major constituent of natural gas, is a gas that is composed of discrete \(\ce{CH4}\) molecules. A comparison of the different physical properties of ionic compounds and covalent molecular substances is given in Table \(\PageIndex{2}\).
| Ionic Compounds | Covalent Molecular Substances |
|---|---|
| hard solids | gases, liquids, or soft solids |
| high melting points | low melting points |
| nonvolatile | volatile |
Summary
The atoms in chemical compounds are held together by attractive electrostatic interactions known as chemical bonds. Ionic compounds contain positively and negatively charged ions in a ratio that results in an overall charge of zero. The ions are held together in a regular spatial arrangement by electrostatic forces. Atoms or groups of atoms that possess a net electrical charge are called ions; they can have either a positive charge (cations) or a negative charge (anions). Ions can consist of one atom (monatomic ions) or several (polyatomic ions). The charges on monatomic ions of most main group elements can be predicted from the location of the element in the periodic table. Ionic compounds usually form hard crystalline solids with high melting points.

