Skip to main content
Chemistry LibreTexts

Gas Laws

  1. Last updated
  2. Save as PDF
  • Page ID
    53599

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Skills to Develop

    • Explain the following laws within the Ideal Gas Law

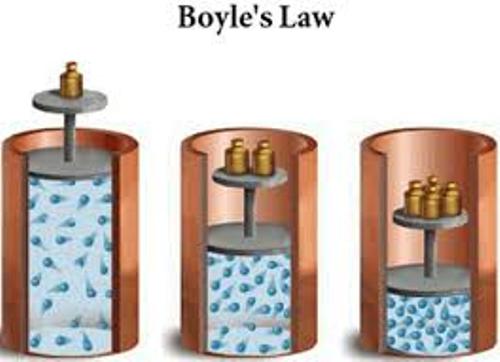
    Boyle's Law

    Boyle was an Irish nobleman who is often described as one of the first modern chemists, as opposed to the old alchemists. However, many of his ideas and experiments came from earlier chemist/alchemists.

    Boyle observed that for a particular sample of gas at a constant temperature, if the pressure or volume is changed, the initial and final pressure and volume are related:

    \[P_{0}V_{0} = P_{1}V_{1}\]

    This is now called Boyle's Law. Later it was shown that Boyle's law is an approximate law, not an exact law. Most gases follow it pretty closely at normal pressures, but less closely at large pressures.

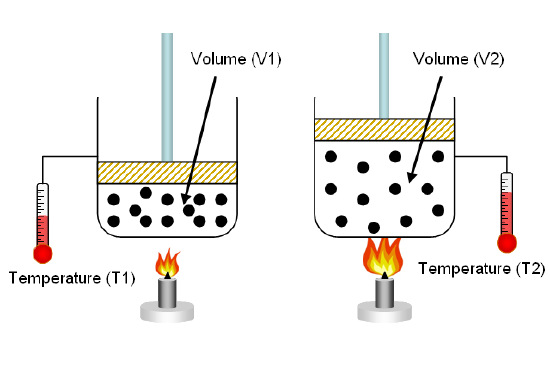
    A model demonstrating how volume decreases as pressure increases.

    Charles' Law and Absolute Temperature

    Charles made the first solo flight in a hot-air balloon filled with hydrogen. He also observed that at constant pressure, the volume of a gas increases linearly with temperature. This can be written

    \[V = kT\]

    Notably, although he couldn't measure volumes at very low temperatures, all the lines for different gases pointed to the same temperature point. The temperature at which each gas was predicted to have V = 0 was the same, although the slopes were different. This temperature is now called 0 K = -273 °C, or absolute zero. Since gases can't have negative volume, this temperature seems to be special: the lowest possible temperature. Although in fact gases won't have zero volume at absolute zero (they'll be solids, and solids have volume), modern theory does still consider absolute zero special. In fact, we have to use temperature in Kelvin for any gas law problem.

    A diagram showing how volume increases as temperature increases.

    Avogadro's Hypothesis

    We discussed this earlier. Although often called Avogadro's Law, it was actually a hypothesis. The hypothesis is that at the same temperature and pressure, all gases have the same number of particles (molecules). Avogadro guessed that this was true based on Gay-Lussac's law, but he had no way to measure it directly, so it couldn't really be called a law. However, now we can be pretty sure that it is approximately true.

    Ideal Gas Law

    The Ideal Gas Law combines Boyle, Charles and Avogadro's laws. The Ideal Gas Law says that

    \[PV = nRT\]

    where P is pressure, V is volume, T is temperature, n is the number of moles, and R is the molar gas constant. We can express it another way too:

    \[PV = nk_{B}T\]

    where everything is the same except n is now the number of particles, and kB is the Boltzmann constant. As you can see, the gas constant R is just the Boltzmann constant multiplied by Avogadro's number (the number of particles in a mole). The Boltzman constant essentially provides a conversion factor between temperature in K and energy in J. Because temperature and energy are closely connected, kB appears in many important equations.

    You can use the Ideal Gas Law to make predictions about how gases will react when you change pressure, volume or temperature. It gives you a good intuition for what gases do. The predictions it makes aren't always very accurate: they're pretty good at normal temperature and pressure, but actually for most engineering work they aren't good enough, so people use other equations or data tables instead. The Ideal Gas Law is a scientific law: it describes mathematically what happens under certain conditions, in this case low pressure. In the next section we'll describe the theory that explains the behavior of gases, which will also tell us when we should expect the Ideal Gas Law to be inaccurate.

    You can use the ideal gas law to make various calculations, including with density and molar mass. We need to be careful with the units, because there are so many pressure units. Check that your value of R has the right unit for pressure, and isn't using an energy unit (because sometimes it's convenient to use energy units for R, but not in the ideal gas equation). Also make sure you use temperature in K. For example of a calculation, the ideal gas law says that the molar volume of any gas should be almost the same, and under standard conditions (1 atm and 0 °C) it should be close to 22.4 L.

    Contributors and Attributions

    Gas Laws is shared under a CC BY license and was authored, remixed, and/or curated by LibreTexts.