5.5: Wave Mechanics
- Page ID
- 49287
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)During the middle of the nineteen-twenties some scientists began to realize that electrons must move around the nucleus in a very different way from that in which planets move around the sun. They abandoned the idea that an electron traces out a definite orbit or trajectory. Instead they adopted the point of view that it was impossible to describe the exact path of a particle whose mass was as small as that of an electron. Rather than think of the motion in planetary terms, they suggested it was much more useful to think of this motion in terms of a wave which could fold itself around the nucleus only in certain specific three-dimensional patterns. This new way of approaching the behavior of electrons (and other particles too) became known as wave mechanics or quantum mechanics. In order to familiarize you with some of the concepts and terminology of wave mechanics, we shall consider the simple, though somewhat artificial, example illustrated in Figure 1. This is usually referred to as a particle in a one-dimensional box. We consider the particle (which could be an electron) to have a mass m and to be restricted in its movement by a narrow but absolutely straight tube of length d into which it can just fit. This container, or box, is closed at both ends and insures that the particle can move in only one dimension within its length.
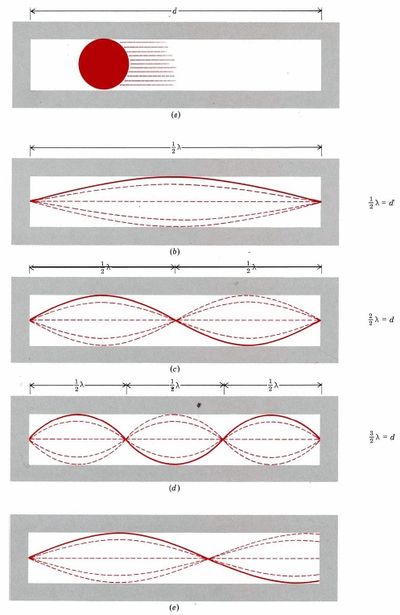
An everyday object like a marble or a billiard ball could move back and forth in this container, bouncing off either end. If there were no friction to slow it down, the particle would oscillate indefinitely, maintaining a constant speed v and a constant kinetic energy Ek of value ½ mv2. The actual magnitude of v (and hence Ek) would depend on how large or how small a “push” the particle was given initially, to start it moving.
In order to look at this particle from a wave-mechanical point of view, we apply an idea originally suggested in 1924 by Louis de Broglie (1892 to 1987). He proposed that a wave of wavelength λ is associated with every particle. The larger the mass of the particle and the faster it is moving, the smaller this wavelength becomes. The exact relationship is given by the formula
\[\lambda =\dfrac{h}{\mu} \label{1} \]
where μ is the momentum of the electron, the product of its mass and velocity (μ = m * v) and the constant of proportionality h is known as Planck’s constant (h = 6.626 × 10–34 J s). The wave-mechanical view no longer pictures the particle as the oscillating billiard ball of Figure \(\PageIndex{1}\)a. Instead we must begin to think of it as having a behavior similar to that of the guitar string illustrated in Figure \(\PageIndex{1}\)b,c or d. Elsewhere you can see a series of videos demonstrate and describe the aspects of wave mechanics. They show the different wavelengths that can be formed by a string anchored at both ends. If the string is attached to both ends of the box, only those waves or vibrations in which the ends of the string do not move are possible. The length d of the box can thus correspond to a single half wavelength (Figure \(\PageIndex{1}\)b), to two half wavelengths (Figure \(\PageIndex{1}\)c), to three half wavelengths (Figure \(\PageIndex{1}\)d, etc., but not to the intermediate situation shown in Figure \(\PageIndex{1}\)e. In other words, the length of the tube must correspond to an integral number of half wavelengths, or
\[d=n\dfrac{\lambda}{\text{2}} \label{2} \]
where n = 1, 2, 3, 4, or some larger whole number. If n = 1, d = λ/2; if n = 2, d = λ; and so on. Rearranging Equation \(\ref{2}\), we then obtain
\[\lambda =\dfrac{\text{2}d}{n} \label{3} \]
Since the right-hand sides of Equations \(\ref{1}\) and \(\ref{3}\) are both equal to λ, they may be set equal to each other, giving
\[\dfrac{\text{2}}{n} d = \dfrac{h}{\mu} = \dfrac{h}{mv} \nonumber \]
which rearranges to give
\[v=\dfrac{nh}{\text{2}md} \text{ } n = \text{1, 2, 3, 4,} \ldots \nonumber \]
We can now calculate the kinetic energy of our wave-particle. It is given by the formula
\[E_{k}=\tfrac{\text{1}}{\text{2}}mv^{\text{2}}=\tfrac{\text{1}}{\text{2}}m\left( \dfrac{nh}{\text{2}md} \right)^{\text{2}} \nonumber \]
or
\[E_{k}=n^{\text{2}}\left( \dfrac{h^{\text{2}}}{\text{8}md^{\text{2}}} \right) \label{4} \]
Since the value of n is restricted to positive whole numbers, we arrive at the interesting result that the kinetic energy of the electron can have only certain values and not others. Thus, if our particle is an electron (m = 9.1 × 10–31 kg) and the one-dimensional box is about the size of an atom (d = 1 × 10–10 m), the allowed values of the energy are given by:
\[E_{k}=n^{\text{2}}\left( \dfrac{h^{\text{2}}}{\text{8}md^{\text{2}}} \right)=n^{\text{2}}\text{ }\times \text{ }\dfrac{\text{(6}\text{.624 }\times \text{ 10}^{-\text{34}}\text{ J s)}^{\text{2}}}{\text{8 }\times \text{ 9}\text{.1 }\times \text{ 10}^{-\text{31}}\text{ kg }\times \text{ 1 }\times \text{ 10}^{-\text{20}}\text{ m}^{\text{2}}} \nonumber \]
\[=n^{\text{2}}\text{ }\times \text{ 6}\text{.0 }\times \text{ 10}^{-\text{18}}\text{ }\dfrac{\text{J}^{\text{2}}\text{ s}^{\text{2}}}{\text{kg m}^{\text{2}}}=n^{\text{2}}\text{ }\times \text{ 6}\text{.0 }\times \text{ 10}^{-\text{18}}\text{ J} \nonumber \]
\[\text{ (recall: 1 J}=\text{1 kg m}^{\text{2}}\text{ s}^{-\text{2}}\text{)} \nonumber \]
Thus if n = 1, Ek = 12 × 6.0 × 10–18 J = 6.0 aJ
If n = 2, Ek = 22 × 6.0 × 10–18 J = 24.0 aJ
If n = 3, Ek = 32 × 6.0 × 10–18 J = 54.0 aJ
and so on.
This result means that by treating the electron as a wave, its energy is automatically restricted to certain specific values and not those in between. Although the electron can have an energy of 6.0 or 24.0 attojoules (aJ), it cannot have an intermediate energy such as 7.3 or 11.6 aJ. We describe this situation by saying that the energy of the electron is quantized. We can now begin to glimpse some of the advantages of looking at the electron in wave-mechanical terms. If an electron is in some sense a wave, then only certain kinds of motion, i.e., only certain kinds of wave patterns, governed by whole numbers, are possible. As we shall shortly see, when an electron is allowed to move in three dimensions around a nucleus, this kind of behavior easily translates into a shell structure. An electron can be in shell 1 or shell 2 but not in an intermediate shell like 1.386. One more property of the electron arises out of the wave analogy. If the electron in a box behaves like a guitar string, we can no longer state that the electron is located at a specific position within the box or is moving in one direction or the other. Indeed the electron seems to be all over the box at once! All we can say is that the wave (or the vibration of the string) has a certain intensity at any point along the box. This intensity is larger in some places than in others and is always zero at both ends of the box.


