3.8.3: Foods- Energy from Fats and Sugars
- Page ID
- 50734
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Energy from Fats & Sugars
Earlier we discussed the nature of fats and mentioned that fats typically provide 9 Cal/g of food energy, while sugars provide about 4 Cal/g. So in order to store the energy in 10 lb of fat, your body would need to store 22.5 lb of carbohydrates or sugars; but it's more extreme than that. Because sugars carry about their own weight of associated water in the body, 67.5 lb (31 kg) of hydrated glycogen has the energy equivalent of 10 lb (5 kg) of fat![1]
The food energy in various food types is given approximately in the following table [2], and you can find the fat content (as well as all other nutritional information) about nearly all foods in the United States Department of Agriculture's Bulletin #8 which has a searchable USDA Nutrient database. In the database, fats are list under "lipids"/"Fatty Acids" and then under "saturated" and "18:0", indicating the number of carbon atoms (18) in the fatty acid, and the number of double bonds (0) (see Example 1).
| Food component | Energy Density | |
|---|---|---|
| kJ/g | kcal/g | |
| Fat | 37 | 9 |
| Ethanol (drinking alcohol) | 29 | 7 |
| Proteins | 17 | 4 |
| Carbohydrates | 17 | 4 |
| Sorbitol, sugar alcohol sweeteners) | 10 | 2.4 |
| dietary Fiber | 8 | 2 |
These caloric values are measured in a "bomb calorimeter" like the one in the Figure.

A 1-2 g sample of food is sealed in a heavy walled steel cylinder (about 4" in diameter and 7" high), shown in the center of the Figure, which is then filled with pure oxygen at 30-40 atmospheres pressure, and immersed in a few liters of water. The sample is ignited electrically, and the heat released is determined by measuring the temperature increase of water that surrounds the "bomb".
Let's investigate the basis for these caloric values in terms of the chemical reactions that provide the energy. Energy changes which accompany chemical reactions are almost always expressed by thermochemical equations. The combustion of stearic acid, which is the main component of saturated fats, is written:
C18H36O2 (s) + 24 O2(g) → 18 CO2(g) + 18 H2O(l) (25°C, 1 atm pressure)
ΔHm = –11 407 kJ[3] (1)
Here the ΔHm (delta H subscript m) tells us whether heat energy is released or absorbed when the reaction occurs and also enables us to find the actual quantity of energy involved. By convention, if ΔHm is positive, heat is absorbed by the reaction; i.e., it is endothermic. More commonly, ΔHm is negative as in Eq. (1), indicating that heat energy is released rather than absorbed by the reaction, and that the reaction is exothermic. This convention as to whether ΔHm is positive or negative looks at the heat change in terms of the matter actually involved in the reaction rather than its surroundings. In the reaction in Eq. (1), the C, H, and O atoms have collectively lost energy and it is this loss which is indicated by a negative value of ΔHm.
It is important to notice that ΔHm is the enthalpy for the reaction as written. The quantity of heat released or absorbed by a reaction is proportional to the amount of each substance consumed or produced by the reaction. Thus Eq. (1) tells us that 890.4 kJ of heat energy is given off for every mole of C18H36O2 which is consumed. Alternatively, it tells us that 11407 kJ is released for every 18 mole of H2O produced, or for every 18 mol of carbon dioxide produced, or 24 mol of oxygen consumed. Seen in this way, ΔHm is a conversion factor enabling us to calculate the heat absorbed when a given amount of substance is consumed or produced. If q is the quantity of heat absorbed and n is the amount of substance involved, then
\(\Delta H_{\text{m}}=\frac{q}{n}\) (2)
EXAMPLE 1
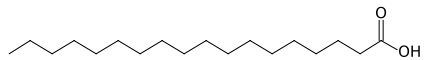
In the molecular model, each bend in the structure is occupied by a carbon atom, and each carbon atom has 4 bonds; missing bonds are to hydrogen atoms, which are not shown.
a. How much heat energy is obtained when 1 g of C18H36O2, is burned in oxygen according to the equation above? The molar mass of steric acid is 284.48 g/mol.
b. What is the caloric value of 1 g of stearic acid, given that ΔHm = –11407 kJ for equation (1)?
Solution
a. The mass of C18H36O2 is easily converted to the amount of C18H36O2 from which the heat energy q is easily calculated by means of Eq. (2). The value of ΔHm is –11407 kJ per mole of C18H36O2. The road map is
\(m_{\text{C}_{\text{18}}\text{H}_{\text{36}}\text{O}_{\text{2}}}\text{ }\xrightarrow{M}\text{ }n_{\text{C}_{\text{18}}\text{H}_{\text{36}}\text{O}_2}\text{ }\xrightarrow{\Delta H_{m}}\text{ }q\) so that \(q=\text{1.0 g C}_{\text{18}}\text{H}_{\text{36}}\text{O}_{2}\text{ }\times \text{ }\frac{\text{1 mol C}_{\text{18}}\text{H}_{\text{36}}\text{O}_2}{\text{284}\text{.48 g C}_{\text{18}}\text{H}_{\text{36}}\text{O}_{2}}\text{ }\times \text{ }\frac{-\text{11407 kJ}}{\text{1 mol C}_{\text{182}}\text{H}_{\text{36}}\text{O}_{2}}=\text{-40.09 kJ}\)
b. \(\text{-40.09 kJ}~\times~\frac{\text{1 kcal}}{\text{4.184 kJ}}~\times~\frac{\text{1 Cal}}{\text{1 kcal}}~=~-\text{9.84 Cal}\)
Note: By convention a negative value of q corresponds to a release of heat energy by the matter involved in the reaction.
This is close to the estimated 9 Cal/g for fats. We saw earlier that most fats are triglycerides, that is, they would have 3 fatty acid substituents (like stearic acid) attached to a glycerol "backbone" in a fat like glyceryl tristearate ("stearin") (C57H110O6, M = 891.48). Stearin has a heat of combustion of -35 663 kJ/mol, so 1 g produces (35 663 kJ/mol) / (891.48 g/mol) x (1 Cal / 4.184 kJ) = 9.57 Cal. When energy is required by our body, triglycerides are converted free fatty acids, and transported by serum albumin in the blood to cells where energy is required. Serum albumin is necessary because the solubility of fatty acids is low in water-based blood.[4]
In comparison, sucrose (C12H22O11) has a molar mass of 342.3 g/mol and a heat of combustion of -5645 kJ/mol, so it produces 16.49 kJ/g or 3.94 Cal/g, very close to the estimated value, by the combustion:
C12H22O11 (s) + 12 O2(g) → 12 CO2(g) + 11 H2O(l) (25°C, 1 atm pressure)
ΔHm = –5 645 kJ mol–1[5]
The quantity ΔHm is the enthalpy change for the reaction as written. In this context the symbol Δ (delta) signifies change in” while H is the symbol for the quantity being changed, namely the enthalpy. We will deal with the enthalpy in some detail in Chap. 15. For the moment we can think of it as a property of matter which increases when matter absorbs energy and decreases when matter releases energy.
It is important to realize that the value of ΔHm given in thermochemical equations like (1) depends on the physical state of both the reactants and the products. Thus, if water were obtained as a liquid instead of a gas in the reaction in Eq. (1), the value of ΔHm would be different from -890.4 kJ. It is also necessary to specify both the temperature and pressure since the value of ΔHm depends very slightly on these variables. If these are not specified [as in Eq. (3)] they usually refer to 25°C and to normal atmospheric pressure.
Two more characteristics of thermochemical equations arise from the law of conservation of energy. The first is that writing an equation in the reverse direction changes the sign of the enthalpy change. For example,
H2O(l) → H2O(g) ΔHm = 44 kJ (3a) tells us that when a mole of liquid water vaporizes, 44 kJ of heat is absorbed. This corresponds to the fact that heat is absorbed from your skin when perspiration evaporates, and you cool off. Condensation of 1 mol of water vapor, on the other hand, gives off exactly the same quantity of heat. H2O(g) → H2O(l) ΔHm = –44 kJ (3b) To see why this must be true, suppose that ΔHm [Eq. (4a)] = 44 kJ while ΔHm [Eq. (4b)] = –50.0 kJ. If we took 1 mol of liquid water and allowed it to evaporate, 44 kJ would be absorbed. We could then condense the water vapor, and 50.0 kJ would be given off. We could again have 1 mol of liquid water at 25°C but we would also have 6 kJ of heat which had been created from nowhere! This would violate the law of conservation of energy. The only way the problem can he avoided is for ΔHm of the reverse reaction to be equal in magnitude but opposite in sign from ΔHm of the forward reaction. That is, ΔHm forward = –ΔHm reverse
References
- en.Wikipedia.org/wiki/Fatty_a...tabolism#Fatty acids as an energy source
- en.Wikipedia.org/wiki/Food_Energy give
- home.fuse.net/clymer/rq/hoctable.html
- en.Wikipedia.org/wiki/Fatty_acid_metabolism
- home.fuse.net/clymer/rq/hoctable.html


