Cooling of Plutons and Young Earth Creationism
- Page ID
- 50853
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The dramatic eruptions of magma from volcanos only hints at the importance of heat energy in geology. We normally think of minerals as being resistant to melting (or "fusion"), but molten minerals are commonly found in the Earth's Mantle, and in manufacturing processes like glassworking,metal refining, and production of rock wool for insulation. Rock wool is made by heating a mixture of minerals to about 1500°C and spinning the wool in a device that works like the ones used to make cotton candy. Many minerals melt at much lower temperatures, down to 600-700 °C.

But mineral thermodynamics has also played a part in debates about the age of the Earth. A physicist, William Thompson (Lord Kelvin), calculated the age of the earth at between 20 and 400 Ma (mega annum or million years, also abbreviated myr for mega years) in 1862.[2]. His calculation was based on the assumption that the Earth was created molten and cooled gradually, with an ever-increasing insulating layer. The age of the Earth is now known to be 4.54 +/- 0.05 Ga (Ga=giga annum, billion years)[3], but young-earth creationists claim an age of 6,000-10,000 years, based on Biblical chronologies and an infallible scripture. [4]
Proponents of young-earth creationism have difficulty explaining the cooling of a pluton, an intrusive igneous rock crystallized from magma. [5]
The Earth now has a solid "inner core" where high pressures prevent melting, even though the temperature is the highest at 5,430 °C, surrounded by a molten "outer core" where the temperature falls to 4400°C. The next layer is the "mantle" of mostly solid, easily deformed rock and some molten minerals where the temperature falls to 500-900°C, and finally the solid surface layer called the crust.
Clearly, estimating the time required to cool the Earth to present temperatures is complicated. In 1892, Thomson revised his estimate of the age of the Earth to 100 Ma, but later reduced it to 20 Ma. In 1895, Thompson's assistant, John Perry added the effects of convection currents in the mantle, which increased the estimate to 2-3 Ga (giga annum, billion years). But neither scientist included the overwhelming effects of radioactive heating, because radioactivity was not discovered until 1896. Geologists realized that radioactivity made the calculations totally inaccurate, but also provided a means of accurately measuring the age of the earth, which we'll investigate later. In 1903 Pierre Curie and his associate Albert Laborde announced that radium produces enough heat to melt its own weight in ice in less than an hour (see below). Radioactivity generates about 2.47 x 1013 J/s of heat energy in the Earth's mantle. [6]
Let's try to understand the cooling of molten minerals in absence of radioactivity. This will establish a minimum age of the Earth, because radioactivity makes the cooling even slower, corresponding to an older earth.
Cooling Curve
This plot shows the cooling curve for zinc.
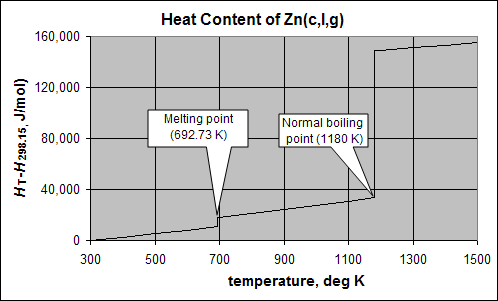
The vertical axis is HT-H25, or the Enthalpy (heat content) above that of one mole (65.4 g) of zinc metal at 25°C. Imagine heat being removed at a constant rate of 1 kJ/minute from an insulated sphere of molten zinc at 1100K (1373°C). Following the trace on the graph to the left from the 1100K gridline, the heat content drops by about 10 kJ of heat is removed, taking about 10 minutes, as the temperature decreases by 400K to the melting point (692.7°C). The rate of temperature decrease is about 40K per minute. At the melting point, the temperature does not change for 7 minutes as an additional 7323 J of heat is removed. Almost as much heat was removed, with no change in temperature, as in the 400K cooling process. The heat that must be removed at constant temperature to solidify a substance is called the heat (or enthalpy) of fusion,ΔHfus. Finally, after all the zinc has solidified, it begins to cool as heat is removed, losing another 10kJ of heat in 10 minutes as the solid cools from around 700 to 300 K (around room temperature).
Now imagine we were using the temperature decrease as a measure of the time that it took for the zinc sphere. We would predict 800K/40K/min = 20 minutes, but in reality, about 27kJ of heat was removed, which requires 27 minutes. Clearly, the heat of fusion accounts for a large part of the "heat content" of a molten substance.
A fairly complex formula [7] [8] is necessary to give the time in seconds for a body to cool to the ambient temperature (T0). For example, a diabasic intrusion the size of the Palisades Sill would require ~2000 years to solidify and longer than 10,000 years to cool to ambient. Creationists have ad hoc arguments to explain these facts, for example that plutons may have formed instantaneously [9], but cooling of plutons generally sets the earths age at millions of years.
The behavior of zinc demonstrates quite clearly that energy must be supplied to a solid in order to melt it, or a significant amount of energy must be removed to freeze it. On a microscopic level melting involves separating molecules or atoms which attract each other. This requires an increase in the potential energy of the molecules, and the necessary energy is supplied by the heating coil. The kinetic energy of the molecules (rotation, vibration, and limited translation) remains constant during phase changes, because the temperature does not change.
| Mineral | Formula | ΔHfus kJ mol-1 | Melting T |
|---|---|---|---|
| Quartz | SiO2 | 9.6 | - |
| Corundum | Al2O3 | 107 | 2345 |
| Zircon | ZrO2SiO2 | 144 | - |
| Halite | NaCl | 28.158 | 1073.8 |
| Sodium Metasilicate | Na2O,SiO2 | 51.8 | 1362 |
| Wollastonite | CaO, SiO2 | 27.4 | 1817 |
| Magnetite | Fe3O4 | 138.1 | 1870 |
| Ilmenite | FeO4,TiO2 | 90.67 | 1640 |
| Hematite | Fe2O3 | 74.9 | 1895 |
We noted above that a sample of radium produces enough heat to melt its own weight in ice in less than an hour. This is a well known quantity of heat, related to the heat of fusion of water:
\[\ce{H2O (s) -> H2O (l)} \nonumber\]
(0°C) ΔHm = 6.01 kJ mol–1 or 108.2 kJ/g
Selected molar enthalpies of fusion are tabulated below. Solids like ice which have strong intermolecular forces have much higher values than those like CH4 with weak ones.
Heat of Vaporization
When a liquid is boiled, the variation of temperature with the heat energy supplied is similar to that found for melting. When heat is supplied at a steady rate to a liquid at atmospheric pressure, the temperature rises until the boiling point is attained. After this the temperature remains constant until the enthalpy of vaporization has been supplied. Once all the liquid has been converted to vapor, the temperature again rises. In the case of water the molar enthalpy of vaporization is 40.67 kJ mol–1. In other words
\[\ce{H2O (l) -> H2O (g)} \nonumber\]
(100°C) ΔHv = 40.67 kJ mol–1
Note that the Heat vs. Temperature plot for zinc (above) shows the large heat of vaporization for zinc:
\[\ce{Zn (l) -> Zn (g)}\nonumber\]
(1180K, 1453°C) ΔHv = 115.330kJ mol–1
| Substance | Formula | ΔH(fusion) / kJ mol1 |
Melting Point / K | ΔH(vaporization) / kJ mol-1 | Boiling Point / K | (ΔHv/Tb) / JK-1 mol-1 |
|---|---|---|---|---|---|---|
| Neon | Ne | 0.33 | 24 | 1.80 | 27 | 67 |
| Oxygen | O2 | 0.44 | 54 | 6.82 | 90.2 | 76 |
| Methane | CH4 | 0.94 | 90.7 | 8.18 | 112 | 73 |
| Ethane | C2H6 | 2.85 | 90.0 | 14.72 | 184 | 80 |
| Chlorine | Cl2 | 6.40 | 172.2 | 20.41 | 239 | 85 |
| Carbon tetrachloride | CCl4 | 2.67 | 250.0 | 30.00 | 350 | 86 |
| Water* | H2O | 6.00678 at 0°C, 101kPa 6.354 at 81.6 °C, 2.50 MPa |
273.1 | 40.657 at 100 °C, 45.051 at 0 °C, 46.567 at -33 °C |
373.1 | 109 |
| n-Nonane | C9H20 | 19.3 | 353 | 40.5 | 491 | 82 |
| Mercury | Hg | 2.30 | 234 | 58.6 | 630 | 91 |
| Sodium | Na | 2.60 | 371 | 98 | 1158 | 85 |
| Aluminum | Al | 10.9 | 933 | 284 | 2600 | 109 |
| Lead | Pb | 4.77 | 601 | 178 | 2022 | 88 |
*http://www1.lsbu.ac.uk/water/data.html
Heat energy is absorbed when a liquid boils because molecules which are held together by mutual attraction in the liquid are jostled free of each other as the gas is formed. Such a separation requires energy. In general the energy needed differs from one liquid to another depending on the magnitude of the intermolecular forces. We can thus expect liquids with strong intermolecular forces to have larger enthalpies of vaporization. The list of enthalpies of vaporization given in the table bears this out.
Two other features of the table deserve mention. One is the fact that the enthalpy of vaporization of a substance is always higher than its enthalpy of fusion. When a solid melts, the molecules are not separated from each other to nearly the same extent as when a liquid boils. Second, there is a close correlation between the enthalpy of vaporization and the boiling point measured on the thermodynamic scale of temperature.Periodic trends in boiling point closely follow periodic trends in heat of vaporiation. If we divide the one by the other, we find that the result is often in the range of 75 to 90 J K–1 mol–1. To a first approximation therefore the enthalpy of vaporization of a liquid is proportional to the thermodynamic temperature at which the liquid boils. This interesting result is called Trouton’s rule. An equivalent rule does not hold for fusion. The energy required to melt a solid and the temperature at which this occurs depend on the structure of the crystal as well as on the magnitude of the intermolecular forces.
Thermodynamic data are very important in Geology, so several databases are available [11]. [12]
From ChemPRIME: 10.9: Enthalpy of Fusion and Enthalpy of Vaporization
References
- ↑ http://en.Wikipedia.org/wiki/Lava
- ↑ England, Philip C.; Molnar, Peter; Richter, Frank M. (2007). "Kelvin, Perry and the Age of the Earth". American Scientist 95 (4): 342–349. doi:10.1511/2007.66.3755. fckLR
- ↑ http://www.talkorigins.org/faqs/isochron-dating.html
- ↑ www.icr.org/index.php?module=discover
- ↑ http://gondwanaresearch.com/hp/paleosol.htm
- ↑ http://gondwanaresearch.com/hp/adam.htm
- ↑ http://gondwanaresearch.com/hp/paleosol.htm
- ↑
 where where x= width of the solidifying body, κ = thermal diffusivity, ts time to solidify and λ is a function described by
where where x= width of the solidifying body, κ = thermal diffusivity, ts time to solidify and λ is a function described by  where L=latent heat of fusion, c=specific heat, erf= error function and Tm-T0 temperature difference between the intrusion and the country rock.
where L=latent heat of fusion, c=specific heat, erf= error function and Tm-T0 temperature difference between the intrusion and the country rock. - ↑ "http://www.icr.org/pubs/imp/imp-326.htm">Snelling
- ↑ R. Leth-Miller, A. D. Jensen, P. Glarborg, L. M. Jensen, P. B. Hansen and S. B. Jørgensen Thermochimica Acta, 406 (2003) 129-142
- ↑ http://www.thermart.net/(FREED/ Free energy and Enthalpy Database)
- ↑ en.Wikipedia.org/wiki/Thermodynamic_databases_for_pure_substances
Contributors and Attributions
Ed Vitz (Kutztown University), John W. Moore (UW-Madison), Justin Shorb (Hope College), Xavier Prat-Resina (University of Minnesota Rochester), Tim Wendorff, and Adam Hahn.


