10.10: Enthalpy of Fusion and Enthalpy of Vaporization
- Page ID
- 49664
When heat is supplied to a solid (like ice) at a steady rate by means of an electrical heating coil, we find that the temperature climbs steadily until the melting point is reached and the first signs of liquid formation become evident, as can be seen on the graph below. Thereafter, even though we are still supplying heat to the system, the temperature remains constant as long as both liquid and solid are present. On the graph below, this is represented by the flat line, where energy is being added to the ice, but no change is occurring in the temperature. All energy added to the system at this stage is used to convert solid ice into liquid water.
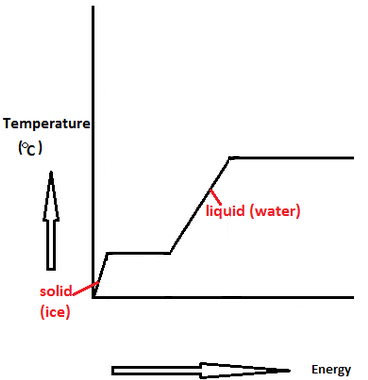
Image Credits: By Cawang via Wikimedia Commons
This macroscopic behavior demonstrates quite clearly that energy must be supplied to a solid in order to melt it. On a microscopic level melting involves separating molecules which attract each other. This requires an increase in the potential energy of the molecules, and the necessary energy is supplied by the heating coil. The kinetic energy of the molecules (rotation, vibration, and limited translation) remains constant during phase changes, because the temperature does not change.
The heat which a solid absorbs when it melts is called the enthalpy of fusion or heat of fusion and is usually quoted on a molar basis. (The word fusion means the same thing as “melting.”) When 1 mol of ice, for example, is melted, we find from experiment that 6.01 kJ are needed. The molar enthalpy of fusion of ice is thus +6.01 kJ mol–1, and we can write
\[\ce{ H2O(s) ->[0^{o} \text{C}] H2O(l)} \nonumber \]
\[\triangle H_m = 6.01 \frac {\text{kJ}}{\text{mol}} \nonumber \]
Selected molar enthalpies of fusion are tabulated in Table \(\PageIndex{1}\). Solids like ice which have strong intermolecular forces have much higher values than those like CH4 with weak ones. Note that the enthalpies of fusion and vaporization change with temperature.
When a liquid is boiled, the variation of temperature with the heat supplied is similar to that found for melting. When heat is supplied at a steady rate to a liquid at atmospheric pressure, the temperature rises until the boiling point is attained. After this the temperature remains constant until the enthalpy of vaporization (ΔHm) has been supplied. Once all the liquid has been converted to vapor, the temperature again rises. In the case of water the molar enthalpy of vaporization is 40.67 kJ mol–1. In other words
\[\ce{ H2O(l) ->[100^{o} \text{C}] H2O(g)} \nonumber \]
\[\triangle H_m = 40.67 \frac {\text{kJ}}{\text{mol}} \nonumber \]
heat is absorbed when a liquid boils because molecules which are held together by mutual attraction in the liquid are jostled free of each other as the gas is formed. Such a separation requires energy. In general the energy needed differs from one liquid to another depending on the magnitude of the intermolecular forces. We can thus expect liquids with strong intermolecular forces to have larger enthalpies of vaporization. The list of enthalpies of vaporization given in the table bears this out.
| Substance | Formula | ΔH(fusion) / kJ mol-1 |
Melting Point / K | ΔH(vaporization) / kJ mol-1 | Boiling Point / K | (ΔHv/Tb) / JK-1 mol-1 |
|---|---|---|---|---|---|---|
| Neon | Ne | 0.33 | 24 | 1.80 | 27 | 67 |
| Oxygen | O2 | 0.44 | 54 | 6.82 | 90.2 | 76 |
| Methane | CH4 | 0.94 | 90.7 | 8.18 | 112 | 73 |
| Ethane | C2H6 | 2.85 | 90.0 | 14.72 | 184 | 80 |
| Chlorine | Cl2 | 6.40 | 172.2 | 20.41 | 239 | 85 |
| Carbon tetrachloride | CCl4 | 2.67 | 250.0 | 30.00 | 350 | 86 |
| Water* | H2O | 6.00678 at 0°C, 101kPa 6.354 at 81.6 °C, 2.50 MPa |
273.1 | 40.657 at 100 °C, 45.051 at 0 °C, 46.567 at -33 °C |
373.1 | 109 |
| n-Nonane | C9H20 | 19.3 | 353 | 40.5 | 491 | 82 |
| Mercury | Hg | 2.30 | 234 | 58.6 | 630 | 91 |
| Sodium | Na | 2.60 | 371 | 98 | 1158 | 85 |
| Aluminum | Al | 10.9 | 933 | 284 | 2600 | 109 |
| Lead | Pb | 4.77 | 601 | 178 | 2022 | 88 |
*www1.lsbu.ac.uk/water/data.html
Two other features of the table deserve mention. One is the fact that the enthalpy of vaporization of a substance is always higher than its enthalpy of fusion. When a solid melts, the molecules are not separated from each other to nearly the same extent as when a liquid boils. Second, there is a close correlation between the enthalpy of vaporization and the boiling point measured on the thermodynamic scale of temperature. Periodic trends in boiling point closely follow periodic trends in heat of vaporization. If we divide the one by the other, we find that the result is often in the range of 75 to 90 J K–1 mol–1. To a first approximation therefore the enthalpy of vaporization of a liquid is proportional to the thermodynamic temperature at which the liquid boils. This interesting result is called Trouton’s rule. An equivalent rule does not hold for fusion. The energy required to melt a solid and the temperature at which this occurs depend on the structure of the crystal as well as on the magnitude of the intermolecular forces.
Compare the heat required to vaporize 100 g of lead to the energy required (1)to melt 100 g of lead; (2) to melt 100 g water; and (3) to vaporize 100 g of water.
Solution
To vaporize 100 g of lead:
\[\text{Pb}(l) \rightarrow\text{Pb}(g)\;\;\;\;\;\; (1749^{o} \text{C}) \;\;\;\;\triangle H_m = 178 \dfrac {\text{kJ}}{\text{mol}} \nonumber \]
\[100 g ~ \times ~\dfrac {1 \text{mol Pb}}{207.2 ~\text{g}~ \text{Pb}}~ \times ~\dfrac{178~ \text{kJ}}{\text{mol}} = 85.9~ \text{kJ} \nonumber \]
(1) To melt 100 g of lead:
\[\text{Pb}(s) \rightarrow\text{Pb}(l) \nonumber \]
\((328^{o}\text{C}) \)
\[\triangle H_m = 4.77 \frac {\text{kJ}}{\text{mol}} \nonumber \]
\[100 \text{g} ~\times~ \frac {1 \text{mol Pb}}{207.2 ~\text{g}~ \text{Pb}} ~\times ~\frac{4.77~ \text{kJ}}{\text{mol}} = 2.30~ \text{kJ} \nonumber \]
(2) To melt 100 g of water:
\[100 \text{g} ~\times~ \frac {1 \text{mol}}{18.0 ~\text{g}}~ \times~ \frac{6.01~ \text{kJ}}{\text{mol}} = 33.4~ \text{kJ} \nonumber \]
(3) To vaporize 100 g of water:
\[100 \text{g} ~\times ~\frac {1 \text{mol}}{18.0~ \text{g}} ~\times ~\frac{40.657~ \text{kJ}}{\text{mol}} = 226~ \text{kJ} \nonumber \]
It might be surprising that the heat required to melt or vaporize 100 g of lead is so much less than that require to melt or vaporized water. First, the temperature at which the substance melts has nothing to do with the enthalpy of fusion, although in practice we would have to add more heat to get lead to the melting point. The molar enthalpy of fusion is actually smaller for lead, because of smaller bonding energies between particles. The molar enthalpy of vaporization of lead is larger than that of water, but this problem reminds us that in some cases a mass-based result can be of practical value, showing that less heat is required to vaporize an equal mass of lead.


