6.02: The Chelate Effect (and Macrocycle Effect)

Saint Mary's College: CHEM 342
Bioinorganic Chemistry
Spring 2020: Dr. Kathryn Haas

Agenda   ●   Readings   ●  Worksheets   ●  Essays  ●  WiSEWIKI

---

Introduction

Monodentate ligands bind through only one donor atom. Monodentate means "one-toothed". The halides, phosphines, ammonia and amines seen previously are monodentate ligands.

Bidentate ligands bind through two donor sites. Bidentate means "two-toothed". An example of a bidentate ligand is bis(dimethylphosphino)propane. It can bind to a metal via two donor atoms at once: it uses one lone pair on each phosphorus atom.

Figure \(\PageIndex{1}\):

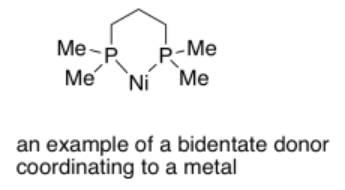
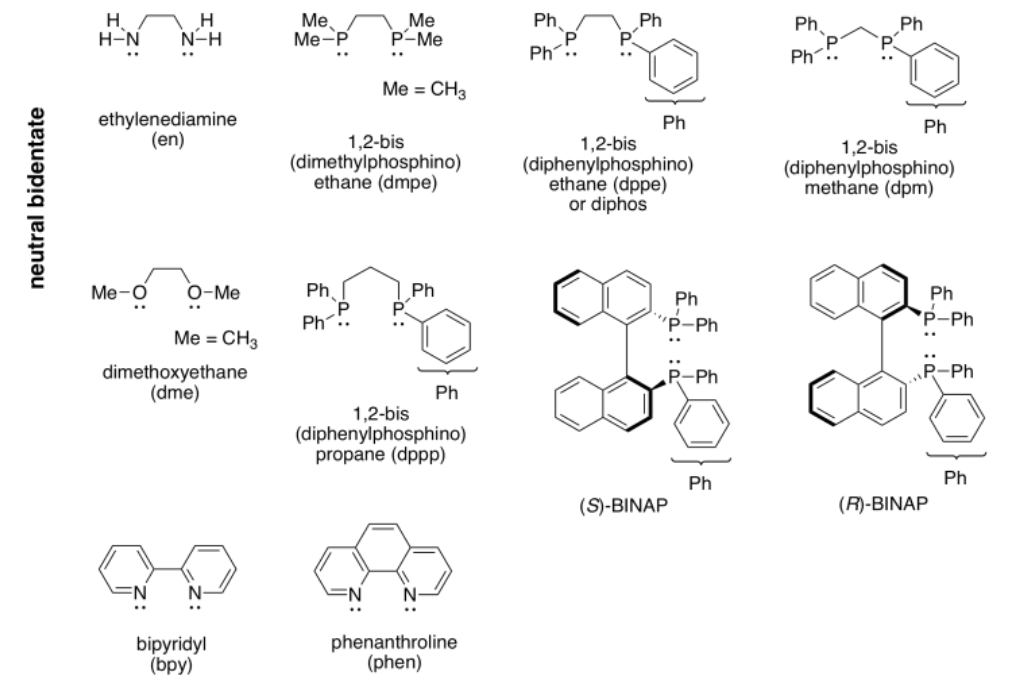
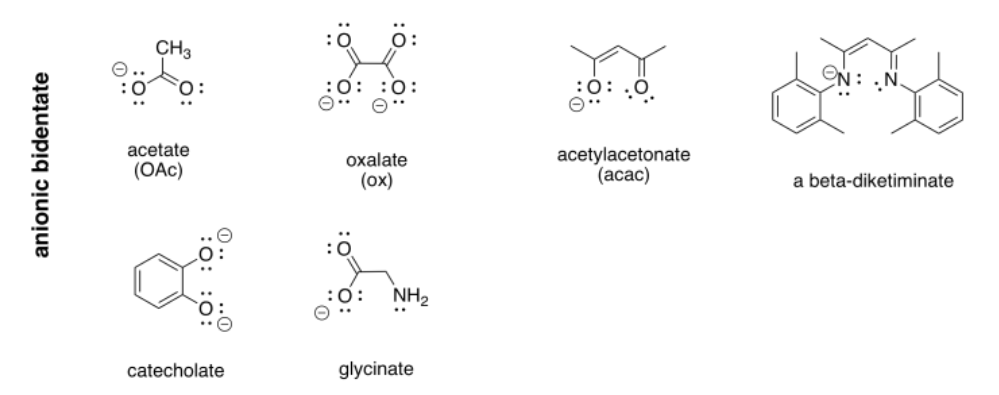
More examples of bidentate ligands are shown below. They all have at least two different atoms with lone pairs. In some cases, there are additional atoms with lone pairs, but only two of them are able to face the metal at one time. Oxalate and glycinate would act as bidentate donors, donating up to two sets of lone pairs at the same time.

Table CC \(\PageIndex{1}\) Some common bidentate ligands

Figure \(\PageIndex{2}\):

Figure \(\PageIndex{3}\):

 

The Chelate Effect

Chelating ligands have higher affinity for a metal ion than analogous monodentate ligands. The chelate effect is the enhanced affinity of a chelating ligand for a metal ion compared to its monodentate ligand counterpart(s).  This term comes from the Greek chelos, meaning "crab". A crab does not have any teeth at all, but it does have two claws for tightly holding onto something. A very simple analogy is that, if you are holding something with two hands rather than one, you are not as likely to drop it. For example, ethylenediamine (en, H₂NCH₂CH₂NH₂) is a bidentate ligand that binds metal ions more strongly than monodentate amine ligands like ammonia (NH₃) and methylamine (CH₃NH₂). Tridentate ligands, which bind through three donors, can bind even more tightly than bidentate, and so on.

Multidentate ligands bind more tightly because of the chelate effect

Chemical reasoning for the Chelate Effect

The chelate effect can be explained using principles of thermodynamics. Recall that reactions are spontaneous when the Gibbs Free Energy change is negative \(-\Delta G\); this is true when change in enthalpy is negative (\(-\Delta H\)) and the change in entropy is positive (disorder increases, \(+\Delta S\). (From the equation \(\Delta G = \Delta H - T\Delta S\).)

Consider the reaction shown below:

Figure \(\PageIndex{4}\): A reaction where one ethylenediamine (en) ligand replaces two amonia ligands in a Cu(II) complex. The equilibrium constant for this reaction is approximately 1000 (K \(\approx\) 10³), and so the equilibrium lies to the left.

Enthalpy 

In each the reactant Cu complex and product Cu-complex in Figure \(\PageIndex{4}\), there are two N-Cu bonds. Electronically, the ammonia and en ligands are very similar, since both bind through N and since the Lewis base strengths of their nitrogen atoms are similar. The enthalpy change due to breaking two H₃N-Cu binds and replacing them with two new N(en)-C bonds is almost zero. Thus, enthalpy is not a major driving factor in the chelate effect.

Entropy

In terms of entropy (disorder) there are two things to consider:

(1) The entropy from free rotation of the chelator. The chelator becomes somewhat constrained upon binding to the metal, and so this would result in small entropic penalty; loss in entropy. This is worth noting, but is a relatively small effect.

(2) The entropy from change in the number of molecules that can move freely. When a chelating ligand replaces several monodentate ligands, the result is an increase in the number of free molecules in the system, and a relatively large increase in entropy. This is the major energetic factor driving the chelate effect. 

When a chelating ligand replaces monodentate ligands, there is a relatively large increase in entropy (+\(\Delta S\)). This is the primary driving factor for the Chelate Effect.

For example, when en replaces two ammonia ligands (Figure \(\PageIndex{4}\)), the number of total molecules increases from two to three. Increasing the number of molecules by just one is enough to drive the reaction forward.

The example above is gives a case when just one bidentate ligand is involved. When multiple bidentate ligands are involved, or when denticity increases, the chelate effect is enhanced further. Consider the two complexation equilibria in aqueous solution, between the cobalt (II) ion, Co²⁺(aq) and ethylenediamine (en) on the one hand and ammonia, NH₃, on the other.

\[[Co(H_2O)_6]^{2+} + 6 NH_3 \rightleftharpoons [Co(NH_3)_6]^{2+} + 6 H_2O \ (1)\]

\[[Co(H_2O)_6]^{2+} + 3 en \rightleftharpoons [Co(en)_3]^{2+} + 6 H_2O \ (2)\]

This means that ΔH must be very similar for the two reactions, since six Co-N bonds are formed in each case. Interestingly however, we observe that the equilibrium constant is 100,000 times larger for the second reaction than it is for the first.

The big difference between these two reactions is that the second one involves "condensation" of fewer particles to make the complex. This means that the entropy changes for the two reactions are different. The first reaction has a ΔS value close to zero, because their is the same number of molecules on both sides of the equation. The second one has a positive ΔS° because four molecules come together but seven molecules are produced. The difference between them (ΔΔS) is about +100 J/mol-K. We can translate this into a ratio of equilibrium constants using:

\[K_f(en)/K_f(NH_3) = e^{-\Delta \Delta G^\circ/RT} \approx e^{+\Delta \Delta S^\circ/R} \approx e^{12} \approx 10^5​\]

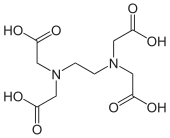
The bottom line is that the chelate effect is entropy-driven. It follows that the more binding groups a ligand contains, the more positive the ΔS° and the higher the K_f will be for complex formation. In this regard, the hexadentate ligand ethylenediamine tetraacetic acid (EDTA) is an optimal ligand for making octahedral complexes because it has six binding groups. In basic solutions where all four of the COOH groups are deprotonated, the chelate effect of the EDTA^4- ligand is approximately 10¹⁵. This means, for a given metal ion, K_f is 10¹⁵ times larger for EDTA^4- than it would be for the relevant monodentate ligands at the same concentration. EDTA^4-tightly binds essentially any 2+, 3+, or 4+ ion in the periodic table, and is a very useful ligand for both analytical applications and separations.

Figure \(\PageIndex{5}\): Ethylenediaminetetraaceticacid acid (EDTA), a hexadentate ligand

 

Exercise \(\PageIndex{1}\)

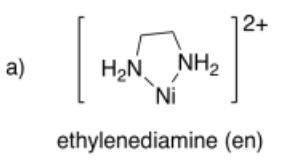
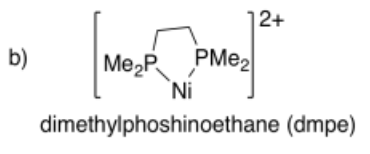
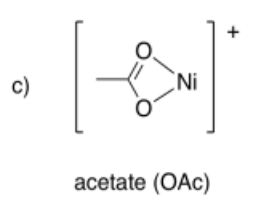
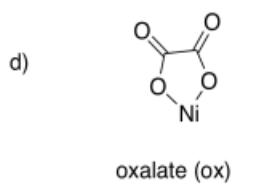
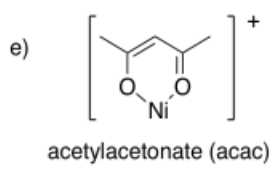
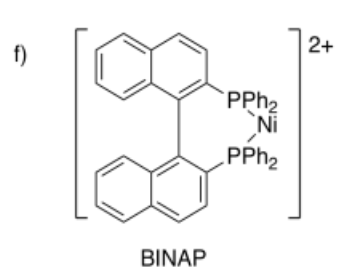
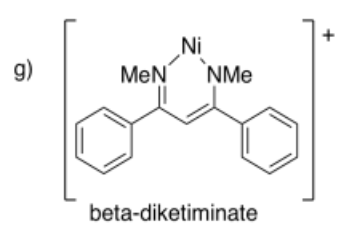
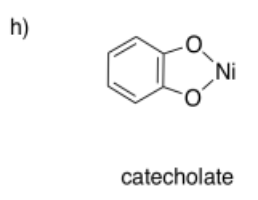
Draw metal complexes using the ligands below, binding to Ni(2+) in a bidentate mode.

Figure \(\PageIndex{1}\):

Answer a):

Answer b):

Answer c):

Answer d):

Answer e):

Answer f):

Answer g):

Answer h):

 

The Macrocycle Effect

The macrocyclic effect follows the same principle as the chelate effect, but the effect is further enhanced by the cyclic conformation of the ligand. Macrocyclic ligands are not only multi-dentate, but because they are covalently constrained to their cyclic form, they allow less conformational freedom. The ligand is said to be "pre-organized" for binding, and there is little entropy penalty for wrapping it around the metal ion. For example heme b is a tetradentate cyclic ligand which is strongly complexes transition metal ions, including (in biological systems) Fe⁺².

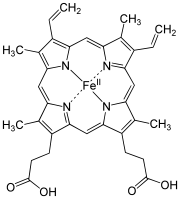
Figure 5.10.3: Heme b

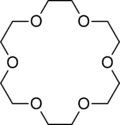
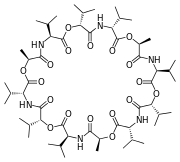
Some other common cyclic ligands are shown below:

• Crown ethers such as 18-crown-6 (below center) are cyclic hard bases that can complex alkali metal cations. Crowns can selectively bind Li⁺, Na⁺, or K⁺ depending on the number of ethylene oxide units in the ring.
• The chelating properties of crown ethers are mimetic of the natural antibiotic valinomycin (below right), which selectively transports K⁺ ions across bacterial cell membranes, killing the bacterium by dissipating its membrane potential. Like crown ethers, valinomycin is a cyclic hard base.

   

 

---

Attribution

This page was modified from:

• Chelation (by Chris Schaller)

• Adapted from the Wikibook constructed by Chemistry 310 students at Penn State University.

Curated or created by Kathryn Haas