Lab 1: Lab View (Analog Electronics)
- Page ID
- 2374
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Labview Page 1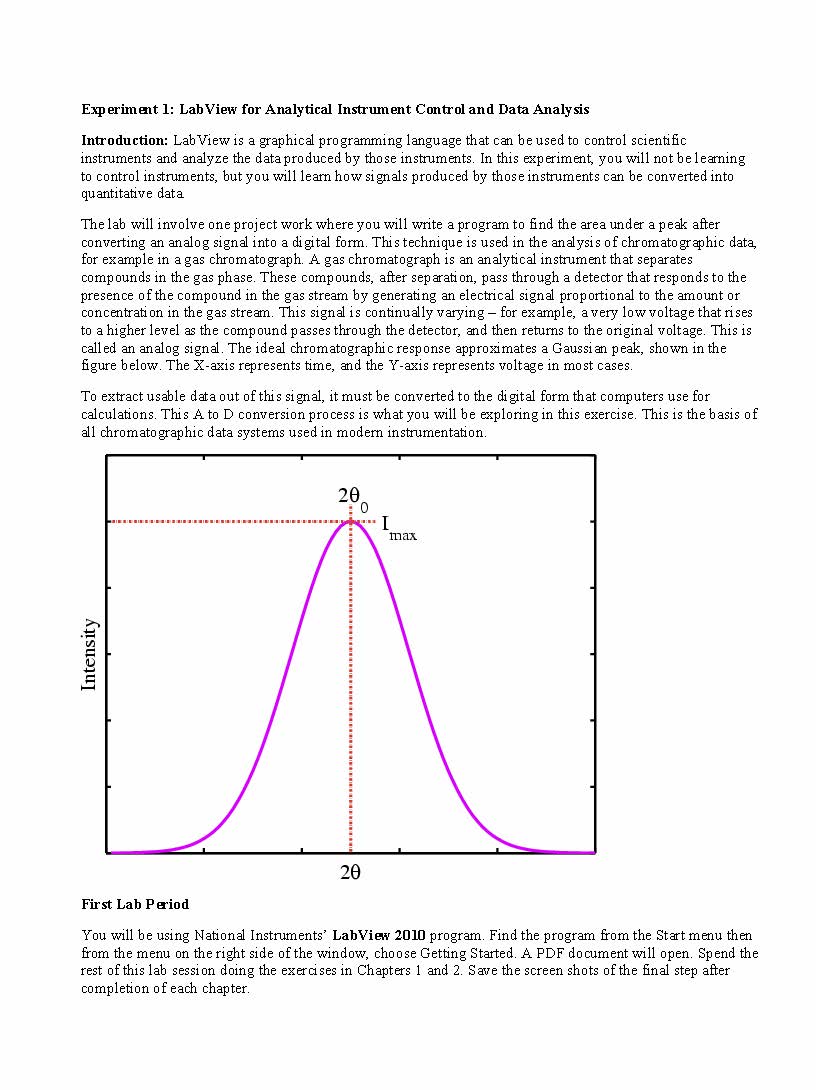
Labview Page 2
---------------------From this point forward is the traditional lab #1 Analog Electronics----------------------------------
In modern analytical chemistry, the quantity to be measured, for instance, the intensity of a light passing through a solution, is converted into an electrical signal which is then amplified or modified to operate a device which can visually display the numerical value of the measured quantity. A simple example is a pH meter in which the potential of the glass electrode responds to the concentration (more precisely, the activity) of hydrogen ions in solution. When an observed electrical response is continuous, it is called an analog response.
The Operational Amplifier
The most important element in analog instrumentation is the operational amplifier. In this experiment, we will examine the properties and uses of operational amplifiers. The operational amplifier is a device with two inputs and one output. It is normally represented by a triangle; the input designated (+) is non-inverting, and that designated (-) inverting (Fig. 1). The output voltage Vo is measured with respect to ground, the common terminal on the power supply. Vs is the voltage of the inverting input (-) with respect to the non-inverting one (+). The fundamental property of this amplifier is that the output voltage Vo is the inverted, amplified value of the voltage Vs; thus, if the gain of the amplifier is A, Vo = -AVs
Figure 1: Schematic of an operational amplifier; the numbers refer to pins on the 741 solid state type.
The power taken from the amplifier is derived from a power supply which usually delivers +12 V and -12 V in the case of solid-state devices. Note that the power supply is normally not shown in circuit diagrams. The following important properties are those of an ideal operational amplifier. It is important to keep them in mind in order to understand the applications: (a) The gain of the amplifier is infinity; real devices have values of A ranging from 104 to 108. In the ideal device, a very small value of Vs results in the maximum value of Vo which is determined by the limits of the power supply (± 12 V). (b) The input impedance is infinity; in real devices, this is in the range 105 to 1013 ?. (c) The output impedance is zero; in practice, it is determined by the power supply. (d) The properties of the device must be independent of time; in practice, real devices produce some noise and drift with respect to time. As a result, the amplifier must be periodically balanced using a separate nulling circuit.
Apparatus
The apparatus for this experiment consists of (a) A protoboard including a 741 operational amplifier for setting up the operational amplifier circuits connected to a +/-12 volt power supply (b) A variable d.c. power supply (c) A digital multimeter (d) A function generator for applying input voltages (e) An oscilloscope for observing the output voltage when it varies with time (f) An assortment of resistors, capacitors, and shielded cables with BNC connectors. For instructions on operation of the oscilloscope and function generator, see the appendix at the end of the experiment. The operational amplifier is a small integrated circuit about 1 cm2 in area with 8 numbered tabs in the following configuration. The tabs have the following purpose: 1. offset null 2. inverting input (-) 3. non-inverting input (+) 4. negative (-) terminal of power supply 5. offset null 6. output 7. positive (+) terminal of power supply 8. none The nulling circuit will not be attached in this experiment, and tabs 1 and 5 will not be used. Remember to define a common or ground terminal on the protoboard and to connect that point to the common terminal on the power supply.
EXPERIMENTAL ASSIGNMENTS
1. Reactive Circuits
Suppose that we are to measure a signal from a device by connecting the output of some transducer to a data acquisition or display device (such as an oscilloscope or an analog-to-digital converter). Most often we will use a shielded cable, such as typical coaxial “BNC” cable, which has a central conductor surrounded by insulation that is, in turn, wrapped by metal braid and more insulation. The metal braid serves as the circuit common return and, because electromagnetic waves do not penetrate into the enclosed metal objects, it also acts as a shield, greatly reducing noise pick-up by the inner conductor. Since the cable consists of two metal surfaces separated by an insulator, it has stray capacitance (as well as a small inductance). Capacitance (and inductance) in circuits causes frequency-dependent effects because the impedance of a capacitive circuit is dependent on the frequency of electric signals: XC = 1 / 2?fC (1) A simple circuit to allow the examination of the effect of capacitance is depicted below. When charging or discharging a capacitor, the voltages of the input Vin and output Vo,C are not proportional to each other, resulting in a phase shift: ? = arctan (Xc / R) (2) which is also frequency dependent.
Circuitry
The series RC circuit illustrates the effects of capacitance on signals of different frequencies. When the resister and capacitor are connected in series, the total voltage drop across the two components always must equal the instantaneous voltage applied as Vin. Since the capacitive reactance varies with frequency, however, the relative proportion of the voltage drops across the resistor and capacitor will vary. Looking across the resistor (at Vo,R), we have what is referred to as a high-pass filter. Looking across the capacitor (at Vo,C), we have a low-pass filter.
Procedure
First build a typical high-pass filter using C = 0.01 µF and R = 10 k?. Do not use the breadboard for this circuit. Use the holes in the wooden parts holder to support the circuit. Apply a 5.0 V p/p sinusoidal signal for Vin. Use the function generator as the power supply and the oscilloscope to make measurements of voltage and frequency. (Start at a frequency of ~10 kHz to set the voltage.) Be careful to make sure that the common leads from the O-scope and function generator are always together. Both devices connect their common points to earth ground via some circuitry. Unless these two common points are connected, ground loops can cause spurious behavior during your measurements. Thus, one has to re-wire the circuit a bit differently to measure across the capacitor or resistor. Refer to Figure 4 above. Measure Vo,R and Vo,C at frequencies of5.0 Hz, 10.0 Hz, 50 Hz, 100 Hz, 500 Hz, 1.00 kHz, 5.00 kHz, 10.0 kHz, and 50.0 kHz. Make sure to check your input amplitude each time you change frequencies, since the signal generator output will vary slightly, especially at low frequncies. Make a semi-log plot of Vo,R vs frequency and another plot using Vo,C vs frequency. Now apply a 5.0 V square wave input and sketch the waveform observed for both Vo,R and Vo,C at frequencies of 50 kHz, 10 kHz, 1 kHz, and 100 Hz. Discuss the results in terms of capacitor reactance and total circuit impedance, pulse width, and RC time constant.
2. THE VOLTAGE AMPLIFIER
Circuitry
Now we turn to basic operational amplifier circuits, the voltage amplifier. In order to operate the amplifier in a stable fashion, a feedback path is always provided between the output and inverting input (-). The amplifier then provides a current through the feedback path, if which maintains the voltage between the inputs, Vs at zero. A typical operating configuration is shown in Fig. 5. An input voltage Vi supplies an input current ii through the input impedance Zi. Similarly, the feedback current if is determined by the magnitude of the feedback impedance Zf. Since the non-inverting input has been connected to ground, the voltage Vs measured at S is zero. Now, by Kirchoff's law, the sum of the currents at S must be zero. Since no current flows into the amplifier when it behaves ideally if = -ii (3) Since if = Vo / Zf, and ii = Vi / Zi, the following relationship holds between the output and input voltages Vo = - Zf Zi Vi (4) The operational amplifier is now acting as a voltage amplifier, that is to say, the input voltage is multiplied by the ratio Zf / Zi and also inverted in sign. For example, if Zf is a 100 k? resistor and Zi, a 1 k? resistor, the amplification factor is 100. Obviously, the operational amplifier can also be used to attenuate the input voltage when Zf < Zi.
In summary, the following rules should be remembered in analyzing a circuit using an operational amplifier with feedback:
- No current flows into either input
- The voltage Vs between the two inputs is zero
- The input current ii is equal and opposite to the feedback current if at point S.
Procedure
In the first application, the impedances, Z, will be simple resistances and the input signals will be DC voltages. Figure 6 shows the breadboard set up as the circuit in Figure 5. Use the variable voltage supply (VVS) to apply -1.0 V for Vi. For Rf = 10 k??and 22 k?, measure Vo with either the DVM or the oscilloscope, with Ri equal to 4.7, 10, 22, 47 and 100 k?. (If you use the oscilloscope, remember to set it to DC.) Plot Vo against Rf / Ri. Discuss the slope and any errors in the measurements. Next, using Ri = 10 k? and Rf = 100 k?, apply a 0.1 V, 1.0 kHz sinusoidal input using the wave function generator. Increase the frequency of the input signal to 5.0 kHz, 10 kHz, 50 kHz, 100 kHz, 500 kHz and 1.0 MHz. Record the amplitude and phase relationship of the input and output signals. Explain any frequency dependence in terms of the bandwidth product (gain times bandwidth) and reactive impedances. Would this circuit be useful for amplifying 1 MHz signals from a piece of analytical instrumentation, such as a Fourier transform mass spectrometer?
3. DIFFERENTIAL AMPLIFIER
Circuitry
Figure 7 The purpose of this amplifier is to provide an output voltage proportional to the weighted difference between V1 and V2. The voltage at T which is equal to the voltage at S is given by VT = Vs = i2Rf = V2RfRi+Rf (5) The input current i1 is given by I1 = V1-Vs Ri = V1Ri - V2RfRi (Ri+Rf) (6) The feedback current if is given by if = = - (7) Since if = -i1, the following equation for Vo is obtained b. Procedure Set Ri = Rf = 10 k? and V1 = 1.2 V. Measure Vo for V2 = 10, 5, 2, 0, -3, -5, and -7 V. Change Rf to 47 k? and repeat with V2 = 3, 2, 1, 0 and -0.5 V. Plot Vo against V2 - V1, and comment on the behavior of this circuit. You should use the voltage divider made from the appropriate resistors and the fixed 12 volt power supply to provide the constant 1.2V for V1 4.
4. THE INTEGRATOR
Circuitry
Figure 8 The integrator produces an output voltage that is proportional to the integral of the input voltage with respect to time. When the reset switch is opened, the current through the feedback loop results in a charge Qf being built up on the feedback capacitor Cf. Qf = CfVo = o t if dt (9) Since if = -ii = -Vi / Ri, Vo = - 1RiCf o t Vi dt (10) If the input voltage does not change with time, then Vo = - (Vi / RiCf) t (11) where t is the time measured from the moment when the reset switch was opened. It follows that the output voltage varies linearly with time. When the reset switch is closed, the feedback loop contains no impedance and the output voltage drops to zero, that is, to the voltage at S. b)
Procedure
(i) (Note: Rf is not used in this step. Leave this resistor off the circuit. See above diagram.) First, the effect of the integrator circuit on a DC signal will be observed. Use the following values for the circuit: Ri = 1 M?, Cf = 1 ?F, and Vi = 0.5 VDC. Connect the output to the DVM (Digital Voltage Meter) and observe the change in Vo with respect to time when the reset switch/cable is unplugged. Record the value of Vo at 5-second intervals until it reaches a minimum value (after approximately 30 seconds). Compare the observed behavior of the system with that expected on the basis of equation (11). (ii) To observe the effect of the integrator circuit on an AC signal, use the Wavetech Signal Generator to generate the input voltage signal. The dual-channel oscilloscope is used to monitor both the input and the output voltage signals. To eliminate the integration of any small DC offset voltages, an additional resister is placed in the feedback loop. Use the following circuit and values: Ri = 10 k?, Cf = 0.01 ?F, Rf = 100 k? First set Vi to 1 V peak-to-peak at 10 kHz using the sinusoidal waveform. The input signal should appear as shown in the diagram below. Draw in the output voltage signal which you observe on the oscilloscope, paying close attention to any phase shift between Vi and Vo. Then switch Vi to the square wave function, as shown in the diagram below. Draw in the observed output signal. Last, repeat the measurement using the triangular waveform. Carefully estimate and record any phase shift between the input and output signals. Why is Rf chosen to be so much large than Ri?
Lab Report
Summarize the results in your lab book in an orderly fashion. Answer all questions at the appropriate point in the report, and discuss errors. How to write your Lab Report - See the general outline, as described on pages 1-3 of this lab manual - Your tables should include both calculated and experimental values for all sections.

