2.3: Isotopic Abundance and Atomic Weight
- Page ID
- 158405
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)learning objectives
- Define atomic weight
- Calculate atomic weight from percent abundance
- Manipulate the atomic weight equation to calculate various unknown variables
- Distinguish between atomic weight, atomic number, and mass number
Introduction
As mentioned in the previous section, atoms that have the same atomic number (number of protons), but different mass numbers (number of protons and neutrons) are called isotopes(nuclides). There are naturally occurring isotopes and isotopes that are artificially produced. Of all the elements on the periodic table, only 21 are pure elements. Pure, or monotopic, elements are those elements with only one naturally occurring isotope. The following lists the 21 pure elements:
| \(^{27}_{13}Al\) | \(^{75}_{33}As\) | \(^{9}_4Be\) | \(^{209}_{83}Bi\) | \(^{133}_{55}Cs\) | \(^{59}_{27}Co\) | \(^{19}_9F\) |
| \(^{197}_{79}Au\) | \(^{165}_{67}Ho\) | \(^{127}_{53}I\) | \(^{55}_{25}Mn\) | \(^{93}_{41}Nb\) | \(^{31}_{15}P\) | \(^{141}_{59}Pr\) |
| \(^{103}_{45}Rh\) | \(^{45}_{21}Sc\) | \(^{23}_{11}Na\) | \(^{159}_{65}Tb\) | \(^{232}_{90}Th\) | \(^{169}_{69}Tm\) | \(^{89}_{39}Y\) |
Table of the 2.3.1 Monotopic Elements
Isotopic Abundance
Isotopes of a given element do not all exist in equal ratios. Mercury, for example, has seven naturally occurring isotopes: \(^{196}Hg\), \(^{198}Hg\), \(^{199}Hg\), \(^{200}Hg\), \(^{201}Hg\), \(^{202}Hg\), \(^{204}Hg\); these have the percent natural abundances of 0.146%, 10.02%, 16.84%, 23.13%, 13.22%, 29.80%, and 6.85%, respectively. It is clear that \(^{202}Hg\) occurs with greatest abundance, and \(^{200}Hg\) is the next most abundant, but the other isotopes only occur in small traces.
Note: The sum of the percent natural abundances of all the isotopes of any given element must total 100%.
Some naturally occurring and artificially produced isotopes are radioactive. All atoms heavier than Bismuth (\(^{209}_{83}Bi\)) are radioactive. However, there are many lighter nuclides that are radioactive. For example, hydrogen has two naturally occurring stable isotopes, \(^{1}H\) and \(^{2}H\) (deuterium), and a third naturally occurring radioactive isotope, \(^{3}H\) (tritium).
It should not be surprising, but isotopic abundances (% of each isotope) can vary between samples. Here is an interesting IUPAC technical report, "Isotope-Abundance Variations of Selected Elements," which describes this, http://ciaaw.org/pubs/SNIF.pdf
How do we know what the percent abundance for each of the isotopes of a given element? Isotopes are separated through mass spectrometry; MS traces show the relative abundance of isotopes vs. mass number (mass : charge ratio).
Measuring Isotopic Abundances
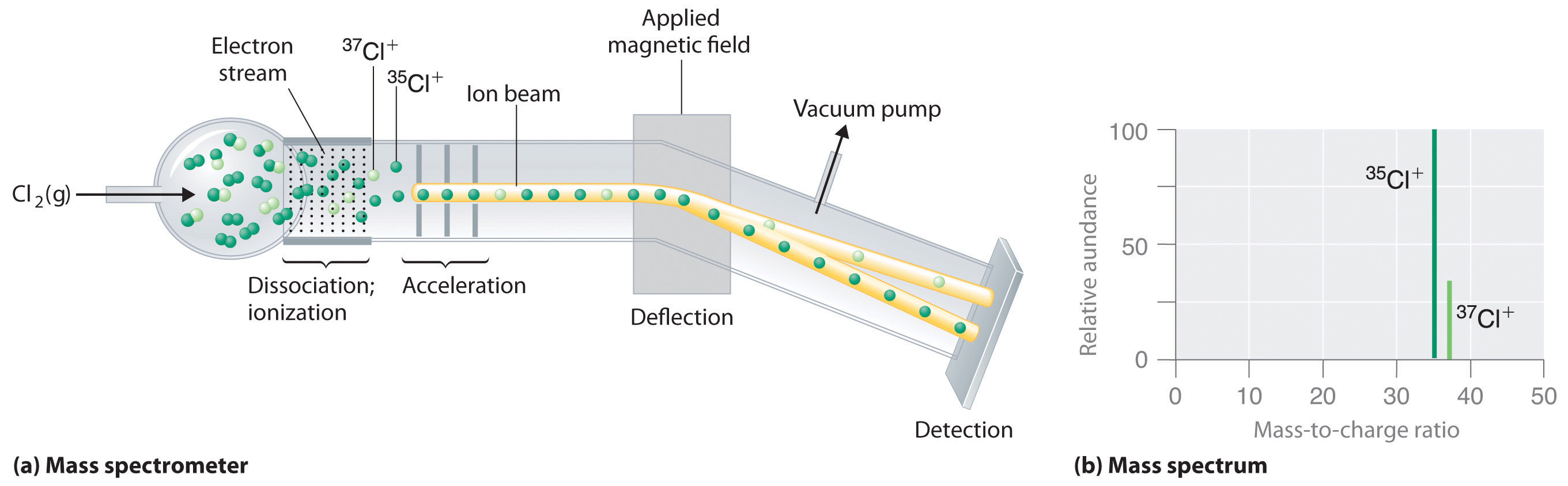
Although we cannot directly measure the mass of atoms, we can use Mass Spectrometer, an instrument that allows us to measure the mass to charge ratio. In figure 2.3.2 you can see chlorine gas entering an mass spectrometer. The chlorine has multiple isotopes and is hit with a stream of ionizing electrons which break the bond of Cl2 and strips electrons off the chlorine causing ions to form. These are then accelerated down the chamber until they reach a magnetic field that deflects the particles. The angle of deflection depends on both the mass of the particle and the magnetic field strength, with the lighter particles being deflected more (the lighter 35Cl+ ions are deflected more than the heavier 37Cl+ ions.) At the end of the chamber is an exit hole with a detector, and as the magnetic field intensity is increased the deflection angle changes, which separates the particles. Note, the mass spectrum in figure 2.3.2 (b) gives the relative abundance of each isotope, with the peak normalized to the isotope with the highest abundance. So if this ratio was 3:1 that means there are 3 particles of 35Cl for every particle of 37Cl, and the percent abundance would be 75% 35Cl and 25% 37Cl.
Figure 2.3.2 Determining Relative Atomic Masses Using a Mass Spectrometer
Below is a video from YouTube describing the mass spectrometer
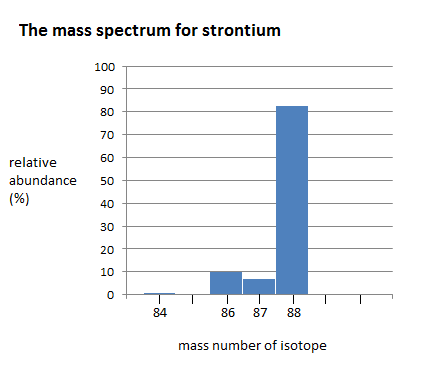
Here is a bar chart showing the relative abundance of 4 isotopes of strontium
Example:
The mass spectrum of strontium has four different peaks, varying in intensity. The four peaks indicate that there are four isotopes of strontium. The four isotopes of strontium have isotopic mass numbers of 84, 86, 87, and 88, and relative abundances of 0.56%, 9.86%, 7.00%, and 82.58%, respectively. The intensity of the peak corresponds to the abundance. \(^{84}Sr\) has the smallest peak, which corresponds to its relative abundance of 0.56%, whereas \(^{88}Sr\) has the largest peak, which corresponds to its relative abundance of 82.58%. This indicates that \(^{88}Sr\) is the isotope that occurs in highest amounts.
Average Atomic Mass
Once we collect the relative masses of each isotope from Mass Spectrometry data, we can use this information to calculate the average atomic mass(weight) of all atoms of an element taking into account the mass of each isotope present and the percent abundance for each isotope. This can be done through the following formula:
Average Atomic Mass = (Mass of Isotope 1 x Fractional Abundance of Isotope 1) + (Mass of Isotope 2 x Fractional Abundance of Isotope 2) + ......
The average atomic mass has been calculated in this fashion and can be found under every symbol in the periodic table. Let us see one such example of how we can calculate this information.
Calculating Average atomic mass
Problem 1 Average Atomic Mass: What is the average atomic mass of Neon, given that it has 3 isotopes with the follow percent abundances;
20Ne = 19.992 amu (90.51%), 21Ne = 20.993 amu (0.27%), 22Ne = 21.991 amu.
What we know: since you know what the element is, you can solve this without doing any math by using the periodic table, but you need to be able to do the math because it might be an unknown, and that is the only way you can figure out the correct significant figures.
Since Ne-20 has the greatest percent abundance, it should have the most impact on your average. Therefore, we expect the average atomic mass to be closer to the mass of Ne-20 (about 19.992 amu). Click the following video tutor to see if we estimated correctly.
Video Tutor:
Answer: According to the correct number of significant figures, we came up with 20.18 amu as the average atomic weight even thought the average atomic weight from the periodic table is 20.179 amu. However, it is still a good check to make sure that you are on the right path.
Check Yourself: We predicted earlier that our answer should be closer to the mass of Ne-20 (19.992 amu) instead of Ne-21 or Ne-22 because it has the greatest natural abundance, and thus, impacts the average more. We can see that the math does align with our logic!
Problem 2: Chlorine has two isotopes, with 75.53% being 35Cl with an isotopic mass of 34.969 amu, what is the mass of the other isotope?
What we know: In this case, you have the average atomic mass (from the periodic table). You are trying to find the mass of the individual isotope. You also know that the individual isotopes have to add up to 100%.
Video Tutor:
Answer: The correct answer is 36.9 amu.
Exercise \(\PageIndex{1}\)
A fictional element has two isotopes and an atomic mass of 131.244 amu. If the first isotope (Isotope 1) has a mass of 129.588amu and the second isotope (Isotope 2) has a mass of 131.912 amu, which isotope has the greatest natural abundance?
A) Isotope 1
B) Isotope 2
C) There are equal amounts.
D) Not enough information provided.
E) none of the above
- Answer
-
B) Isotope 2. Although it is algebraically possible to calculate the particular percent abundances for both isotopes, there is not need to spend that much time on this problem if you know the principle behind it. The average is 131.244 amu. It looks like the mass of Isotope 2 (131.912amu) is closer to the average than the mass of isotope 1 (129.588 amu). This indicates that isotope 2 impacted the average much more than isotope 1 and has a greater percent abundance.
Exercise \(\PageIndex{2}\)
The atomic weight of chorine is ______________and the atomic number of chlorine-35 is________________.
A) 35, 17
B) 17, 35
C) 35.4527; 17
D) 35.4527; 35
- Answer
-
C) the atomic weight is the average of mass of all isotopes of chlorine atoms and found below the symbol on the periodic table. The atomic number is the number of protons in all chlorine atoms and is found on the top of the symbol in the periodic table.
You should do the following worksheets, which were designed as in class activities for the prep course, and so give more step-by-step instructions than we are using.
Isotope Abundance Worksheets:
References
- Petrucci, Ralph H., William S. Harwood, F. Geoffrey Herring, and Jeffry D. Madura. General Chemistry: Principles and Modern Application. Ninth ed. New Jersey: Pearson Prentice Hall, 2007.
- Housecraft, Catherine E. and Alan G. Sharpe. Inorganic Chemistry. Third ed. England: Pearson Prentice Hall, 2008.
- Hoefs, Jochen. Stable Isotope Geochemistry. Sixth ed. Germany: Springer, 2009
Contributors
- Anonymous
- Modified by Joshua Halpern, Scott Sinex and Scott Johnson
- Bob Belford and November Palmer
- Ronia Kattoum (UALR)