4.7: Chemical Equations and Quantitative Analysis
- Page ID
- 51456
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Learning Objectives
- Differentiate between quantitative and qualitative analysis
- Determine the amount of unknown solute in a solution using the gravimetric analysis technique
- Determine the empirical formula of a hydrocarbon or an organic compound using the combustion analysis technique
Quantitative Analysis is a branch of analytical chemistry where you determine the "quantity" of an unknown, which is often contrasted to qualitative analysis, which seeks to identify the identity of unknown. There are many types of quantitative techniques that are based on stoichometry, and many of these deal with solutions, and will be covered in the next several sections of this Chapter
Gravimetric Analysis:
The identification of the quantity of an unknown solute in a solution by making it the limiting reagent of precipitation reaction, weighing the mass of the resulting precipitate and then using the stoichiometry of the precipitation reaction to determine the moles of the unknown. This technique takes advantage of the solubility rules, and the strategy is to use a double displacement reaction where where one of the products is soluble, while the other forms a precipitate
For example, if you wanted to identify the moles of soluble barium chloride in a solution, you could add excess sodium sulfate, the complete ionic equation would be
BaCl2(aq) + Na2SO4(aq) --> BaSO4(s) + 2NaCl(aq)
(limiting) (excess)
By adding excess sodium sulfate, you can force all the barium to form a precipitate, which can be filtered, dried, and weighed. Once the mass is known, the moles barium sulfate can be calculated, and from that the moles (or mass) of the barium chloride can be determined. For this to work, the unknown must be the limiting reagent.
Gravimetric Analysis of Lead (II) Nitrate
Lead was found in the drinking water at Flint Michigan, and one way of removing it is through the double displacement reaction with phosphate, to create lead(II) phosphate, which is an insoluble salt. Interestingly, this forms a protective coating in the pipe that prevents further corrosion. In fact, the city of Flint had removed phosphate from the treatment process, as it also prevents corrosion of iron, and that is when the lead started to dissolve in the first place, as described in the Chemical and Engineering News article of the American Chemical Society.
 |
 |
Figure \(\PageIndex{1}\): Images from Flint Michigan in response to lead in the drinking water. Left image of Texas National Guard from U.S. Department of Defense, right image uploaded to Flickr by Edward Kimmel.
By applying the solubility rules from section 3.4.2.1 one can not only see how phosphate can remove disolved lead from the water, but even be used in the lab to determine the concentration of lead in an unknown sample.
Example \(\PageIndex{1}\): Analysis of Lead in Water Sample.
What is the molar concentration of Pb+2 in contaminated water if 14.06 grams of Pb3(PO4)2(s) precipitates out of 1.00 liter of a lead(II)chloride solution
Solution
The unbalanced equation is: \[Pb(NO_3)_2(aq) + Na_3PO_4(aq) \rightarrow Pb_3(PO_4)_2(s) + NaNO_3(aq)\]
The net ionic equation is
\[ \underset{unknown}{3Pb^{+2}(aq)} + \underset{excess}{2PO_4^{-3}(aq)} \rightarrow \underset{m=14.06g}{Pb_3(PO_4)_2(s)}\]
The general strategy is:
- From mass of lead(II)phosphate calculate moles of lead phosphate that precipitated out of 1 liter of contaminated water.
- From formula of lead(II)phosphate calculate moles of phosphate ion
- Use net ionic equation to calculate moles lead(II)
- Divide into the volume of sample the lead precipitated out of (1.00l) to calculate lead(II) concentration
This is solved in video \(\PageIndex{1}\), and in one equation is:
\[\frac{14.06gPb_3(PO_4)_2\left ( \frac{1 \; mol \; Pb_3(PO_4)_2 }{811.543 \; g} \right )\left ( \frac{3 \; mol \; Pb^{+2}}{mol \; Pb_3(PO_4)_2} \right )}{1.00 \; L}=0.0519M \; Pb^{+2}\]
Exercise \(\PageIndex{1}\) Arsenic in Ground Water
You wish to detect the concentration of arsenate in ground water. You add silver nitrate to a 100 mL sample. The following table shows the concentration of silver arsenate as you add the silver nitrate to the arsenate
| Total mL silver nitrate added to 100 mL of water | mass precipitate |
| 0 | 0 |
| 1mL | 0.154g |
| 2mL | 0.308g |
| 3mL | 0.370g |
| 4mL | 0.370g |
From the above data it can be deduced that after 3 mL of silver nitrate was added, all the arsenate was removed from the water, and arsenate was the limiting reagent. What is the concentration of arsenate in the original sample?
- Answer
-
The net ionic equation for the reaction is
\[3Ag^+(aq) + AsO_4^{-3}(aq) \rightarrow Ag_3AsO_4(s)\]
The arsenate concentration is the number of moles arsenate in 100 mL of water, expressed in liters.
\[\frac{0.350gAg_3AsO_4\left ( \frac{molAg_3AsO_4}{462.52 g} \right )\left ( \frac{1 \; mole \;AsO_4^{-3}}{1 \;mol \;Ag_3AsO_4} \right )}{0.100L}= 0.00757M=7.57mM\]
Lead in Consumer Products
In 1980 lead(II)acetate was listed as safe for use in cosmetics like hair color products has been used in products like Grecian Formula (Figure \(\PageIndex{2}\)), and the FDA repealed is approval for use on October 30, 2018 for health concerns with the ban supposed to go into effect on December 3, 2018. The ban had a 30 day appeal period for anyone "adversely affected" by the ban and the manufacturer, Combe company requested a hearing claiming it was safe, and now the ban is on hold until this is resolved (see the Jan. 4, 2019 Environmental Defense Fund and Jan. 9, 2019 Consumer Report).
 |
 |
Figure \(\PageIndex{2}\) Image credit Bob Belford (left) and right the US FDA announcement repealing the approval of lead(II)acetate in hair products, https://www.fda.gov/cosmetics/cosmetic-products/lead-acetate-progressive-hair-dye-products.
Virtual Exercise \(\PageIndex{1}\)
Using Virtual Lab \(\PageIndex{1}\) to design and run an experiment to determine the lead acetate concentration of your unknown. Please watch http://www.chemcollective.org/chem/common/vlab_walkthrouh_html5.php for a quick guide on how to run the virtual lab. The answer for Unknown A is given below. You should look at the logic of exercise \(\PageIndex{1}\) Arsenic in Ground Water to help with coming up with a strategy to solve this problem. Students at UALR will be given one of the other 5 unknowns as a homework assignment.
- Answer
-
The reaction is:
\[Pb(C_2H_3O_2)_2(aq) + K_2CrO_4 \rightarrow PbCrO_4(s) + 2KC_3H_3O_2(aq)\]
Note we used the constant yield value of lead(II)chromate produced in excess potassium chromate for 100mL of unknown A
\[\frac{9.6966gPbCrO_4}{0.1000l}\left ( \frac{1mol \; PbCrO_4}{323.2g} \right )\left ( \frac{1mol \; Pb(C_2H_3O_2)_2}{1mol \; PbCrO_4} \right )=0.3000M \;Pb(C_2H_3O_2)_2\]
Virtual Lab \(\PageIndex{1}\): ChemCollective Virtual Lab developed by Dave Yaron of Carnegie Mellon University, (http://www.chemcollective.org/). NOTE: if the lab loads as "Default Lab Setup" (with a bunch of acids, bases and indicators), refresh the page . You want it to load the "Gravimetric Analysis of Unknown Leas Solutions", which contains sodium chloride, potassium chromate and six unknowns.
Acid Base Titration
Titrations are a very important analytical technique where you identify the quantity of an unknown (analyte) by reacting it with a titrant of known concentration until they are in stoichiometric proportions, and then use the balanced equation to determine the amount of the unknown analyte. These types of techniques often use visual indicators to indicate when they are complete, of which the indicators may be the reactants and products themselves, or other chemicals that react with the reactants and products. For example, an indicator in an acid-base neutralization reaction will have a color change over a specific pH range, and you choose an indicator that changes color at the pH indicative of where the acid and base are in stoichiometric proportions.
There are other types of titration, but the principle is the same.
Example \(\PageIndex{2}\): Acid Base Titration
A chemist needs to know the concentration of a 50 gallon drum of sulfuric acid. So the chemist uses a 25 mL volumetric pipette to transfer 25.00 mL of the unknown acid to an Erlenmeyer Flask and adds 2 drops of an indicator that changes color when the acid is neutralized. T his solution is then titrated with 0.100M sodium hydroxide and it takes 30.00 mL to neutralize the 25.00 mL of sulfuric acid. How concentrated is the sulfuric acid in the barrel?
Solution
Step 1: Write down the balanced equation and under each entity write what is given in the problem statement.
\[\underset{M=? \\ V=25.00mL}{H_2SO_4(aq)}+ \underset{M=0.100M \\ V=30.00mL}{2NaOH(aq)} \rightarrow Na_2SO_4(aq) +2H_2O(l)\]
Step 2: Calculate moles acid neutralized by adding 30.00mL of 0.100M NaOH.
\[0.03000LNaOH\left ( \frac{0.100molNaOH}{L} \right )\left ( \frac{1 \; mol \; H_2SO_4}{2 \; mol \; NaOH} \right ) = 0.0015 \; mol \; H_2SO_4\]
Step 3: Divide by the volume of acid titrated, and this gives the concentration of the acid in the 50 gallon drum
\[\frac{0.00150 \; mol}{0.02500 \; L}=0.0600M = 60.0mM\]
Combustion Analysis
This is a type of qualitative analysis that can be used to determine the empirical formula of an unknown organic compound consisting of carbon, hydrogen and oxygen. In combustion analysis a sample of known mass is burned in excess oxygen. It is then passed through H2O and CO2 absorbers that collect the hydrogen and carbon that where in the original sample. As we know the mass percent of hydrogen in water (2.01/18.01 or 11.2%) and the mass percent of carbon in carbon dioxide (12.01/44.01 or 27.3%) we can identify their masses from the water and carbon dioxide produced. Once those masses are known, the mass of the oxygen can be determined by substracting them from the original sample mass, and this becomes an empirical formula problem like the ones is Chapter 2.
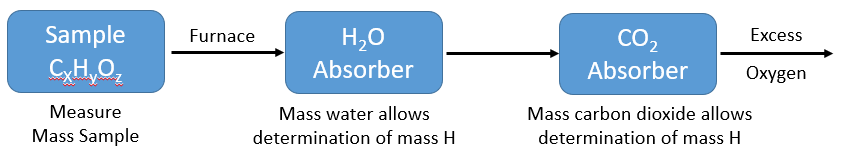
\(\PageIndex{2}\): Schema for obtaining mass analysis data from combustion analysis to determine an empirical formula
 |
 |
Figure \(\PageIndex{4}\): In 1670 John Wray published in the Philosophical Transactions of the Royal Society of London his observations and experiments dealing with the "Juyce to be Found in Ants" .doi:10.1098/rstl.1670.0052. Right, army ants in Uganda, image credit Bernard Dupont, Flickr
Example \(\PageIndex{3}\) Combustion Analysis of Acidum Formicum, Formic Acid
Formic acid, obtained through the distillation of Formica rufa (ants). What is the empirical formula of formic acid if 2.4527 g sample yielded 0.9696g water and 2.3482g carbon dioxide.?
Solution
Step 1: Calculate mass hydrogen in water
\[M_{H}=m_{H_{2}O}\left ( \text{fraction of hydrogen in water} \right ) \\
M_{H}=0.9696g \; H_2O\left ( \frac{2.016g \;H}{18.016g \; H_2O} \right )= 0.1067g \; H\]
Step 2: Calculate mass carbon in carbon dioxide
\[M_{C}=m_{CO_{2}}\left ( \text{fraction of carbon in water} \right ) \\
M_{H}=2.3482g \; CO_2\left ( \frac{12.011g \;C}{44.011g \; CO_2} \right )= 0.6404g \; C\]
Step 3: Calculate mass oxygen by subtracting mass hydrogen and carbon from mass of the sample
\[m_{oxygen}=m_{sample}-m_{hydrogen}-m_{carbon} \\ m_{oxygen} = 2.4527g-0.1067g-0.6404g=1.7056g\]
Now this is an empirical formula problem and is solved in video \(\PageIndex{3}\), giving an empirical formula of CH2O2. Please see section 2.11 if you need to review empirical formula problems.
Exercise \(\PageIndex{3}\)
Formaldehyde is composed of carbon, hydrogen and oxygen. If a 8.7355g sample undergoes combustion analysis and forms 12.806g of carbon dioxide and 5.2385g water, what is its empirical formula?
- Answer
-
CH2O
Test Yourself
Query \(\PageIndex{1}\)
Contributors and Attributions
Robert E. Belford (University of Arkansas Little Rock; Department of Chemistry). The breadth, depth and veracity of this work is the responsibility of Robert E. Belford, rebelford@ualr.edu. You should contact him if you have any concerns. This material has both original contributions, and content built upon prior contributions of the LibreTexts Community and other resources, including but not limited to:
- November Palmer & Ronia Kattoum (UALR)
- anonymous


