3.1: Problem Solving and Unit Conversions
- Page ID
- 365755
- Given a quantity, convert from one set of units to another using dimensional analysis showing canceling on units. This includes one factor conversions.
During your studies of chemistry (and physics also), you will note that mathematical equations are used in many different applications. Many of these equations have a number of different variables with which you will need to work. You should also note that these equations will often require you to use measurements with their units. Using the correct units is critical!
Converting Between Units with Conversion Factors
A conversion factor is a ratio used to convert one unit of measurement into another. A simple conversion factor can convert meters into centimeters, for example. Since most calculations require measurements to be in certain units, you will find many uses for conversion factors. Always remember that a conversion factor has to represent a fact; this fact can either be simple or more complex. For instance, you already know that 12 eggs equal 1 dozen. A more complex fact is that the speed of light is \(1.86 \times 10^5\) miles/\(\text{sec}\). Either one of these can be used as a conversion factor depending on what type of calculation you are working with.
| English Units | Metric Units | Quantity |
|---|---|---|
| 1 ounce (oz) | 28.35 grams (g) | *mass |
| 1 fluid once (oz) | 29.6 mL | volume |
| 2.205 pounds (lb) | 1 kilogram (kg) | *mass |
| 1 inch (in) | 2.54 centimeters (cm) | length |
| 0.6214 miles (mi) | 1 kilometer (km) | length |
| 1 gallon (gal) | 3.785 liters (L) | volume |
*Pounds and ounces are technically units of force, not mass, but this fact is often ignored by the non-scientific community.
Of course, there are other ratios which are not listed in Table \(\PageIndex{1}\). They may include:
- Ratios embedded in the text of the problem (using words such as per or in each, or using symbols such as / or %).
- Conversions in the SI system, as covered in the last chapter.
- Conversions in the English system (such as 12 inches \(=\) 1 foot or 4 quarts \(=\) 1 gallon).
- Time conversions (such as 60 seconds \(=\) 1 minute or 60 minutes \(=\) 1 hour).
If you learned the SI units and prefixes described, then you know that 1 cm is 1/100th of a meter.
\[ 1\; \rm{cm} = \dfrac{1}{100} \; \rm{m} = 10^{-2}\rm{m}\]
or
\[100\; \rm{cm} = 1\; \rm{m}\]
Suppose we divide both sides of the equation by \(1 \text{m}\) (both the number and the unit):
\[\mathrm{\dfrac{100\:cm}{1\:m}=\dfrac{1\:m}{1\:m}}\]
As long as we perform the same operation on both sides of the equals sign, the expression remains an equality. Look at the right side of the equation; it now has the same quantity in the numerator (the top) as it has in the denominator (the bottom). Any fraction that has the same quantity in the numerator and the denominator has a value of 1:
\[ \dfrac{ \text{100 cm}}{\text{1 m}} = \dfrac{ \text{1000 mm}}{\text{1 m}}= \dfrac{ 1\times 10^6 \mu \text{m}}{\text{1 m}}= 1\]
We know that 100 cm is 1 m, so we have the same quantity on the top and the bottom of our fraction, although it is expressed in different units.
Performing Dimensional Analysis
Dimensional analysis is amongst the most valuable tools that physical scientists use. Simply put, it is the conversion between an amount in one unit to the corresponding amount in a desired unit using various conversion factors.
Here is a simple example. How many centimeters are there in 3.55 m? Perhaps you can determine the answer in your head. If there are 100 cm in every meter, then 3.55 m equals 355 cm. To solve the problem more formally with a conversion factor, we first write the quantity we are given, 3.55 m. Then we multiply this quantity by a conversion factor, which is the same as multiplying it by 1. We can write 1 as \(\mathrm{\frac{100\:cm}{1\:m}}\) and multiply:
\[ 3.55 \; \rm{m} \times \dfrac{100 \; \rm{cm}}{1\; \rm{m}}\]
The 3.55 m can be thought of as a fraction with a 1 in the denominator. Because m, the abbreviation for meters, occurs in both the numerator and the denominator of our expression, they cancel out:
\[\dfrac{3.55 \; \cancel{\rm{m}}}{ 1} \times \dfrac{100 \; \rm{cm}}{1 \; \cancel{\rm{m}}}\]
The final step is to perform the calculation that remains once the units have been canceled:
\[ \dfrac{3.55}{1} \times \dfrac{100 \; \rm{cm}}{1} = 355 \; \rm{cm}\]
In the final answer, we omit the 1 in the denominator. Thus, by a more formal procedure, we find that 3.55 m equals 355 cm. A generalized description of this process is as follows:
quantity (in old units) × conversion factor = quantity (in new units)
You may be wondering why we use a seemingly complicated procedure for a straightforward conversion. The conversion problems you encounter will not always be so simple. If you master the technique of applying conversion factors, you will be able to solve a large variety of problems.
In the previous example, we used the fraction \(\frac{100 \; \rm{cm}}{1 \; \rm{m}}\) as a conversion factor. Does the conversion factor \(\frac{1 \; \rm m}{100 \; \rm{cm}}\) also equal 1? Yes, it does; it has the same quantity in the numerator as in the denominator (except that they are expressed in different units). Why did we not use that conversion factor? If we had used the second conversion factor, the original unit would not have canceled, and the result would have been meaningless. Here is what we would have gotten:
\[ 3.55 \; \rm{m} \times \dfrac{1\; \rm{m}}{100 \; \rm{cm}} = 0.0355 \dfrac{\rm{m}^2}{\rm{cm}}\]
For the answer to be meaningful, we have to construct the conversion factor in a form that causes the original unit to cancel out. Figure \(\PageIndex{1}\) shows a concept map for constructing a proper conversion.
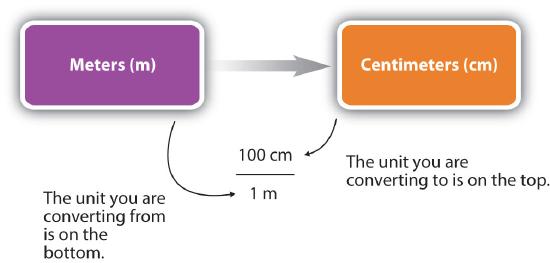
- Identify the "given" information in the problem. Look for a number with units to start this problem with.
- What is the problem asking you to "find"? In other words, what unit will your answer have?
- Use ratios and conversion factors to cancel out the units that aren't part of your answer, and leave you with units that are part of your answer.
- When your units cancel out correctly, you are ready to do the math. You are multiplying fractions, so you multiply the top numbers and divide by the bottom numbers in the fractions.
Significant Figures in Conversions
How do conversion factors affect the determination of significant figures?
- Numbers in conversion factors based on SI definitions, such as kilograms to grams, are not considered in the determination of significant figures in a calculation because the numbers in such conversion factors are exact.
- Conversions within the English system are also exact. The numbers in conversion factors from one English unit to another English unit do not effect the significant figures in the answer.
- Counted numbers are also exact. If there are 16 students in a classroom, the number 16 is exact.
- In contrast, conversion factors that come from measurements (such as density, as we will see shortly) have a limited number of significant figures and should be considered in determining the significant figures of the final answer.
- Conversions between the SI and English systems are not exact. The significant figures in the conversion must be considered when determining the significant figures in the answer. The one exception is the conversion between inches and centimeters (1 in = 2.54 cm) which is exact.
| Example \(\PageIndex{1}\) | Example \(\PageIndex{2}\) | |
|---|---|---|
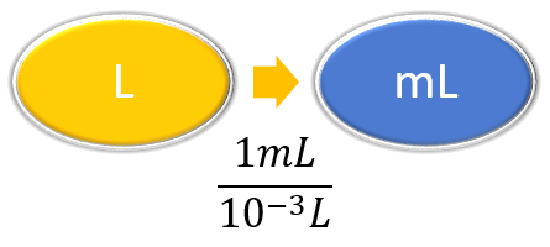
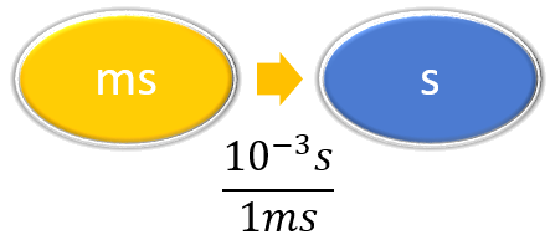
| Steps for Problem Solving | The average volume of blood in an adult male is 4.7 L. What is this volume in milliliters? | A hummingbird can flap its wings once in 18 ms. How many seconds are in 18 ms? |
| Identify the "given" information and what the problem is asking you to "find." | Given: 4.7 L Find: mL |
Given: 18 ms Find: s |
| List other known quantities. | \(1\, mL = 10^{-3} L \) | \(1 \,ms = 10^{-3} s \) |
|
Prepare a concept map and use the proper conversion factor. |
 |
 |
| Cancel units and calculate. |
\( 4.7 \cancel{\rm{L}} \times \dfrac{1 \; \rm{mL}}{10^{-3}\; \cancel{\rm{L}}} = 4,700\; \rm{mL}\) \( 4.7 \cancel{\rm{L}} \times \dfrac{1,000 \; \rm{mL}}{1\; \cancel{\rm{L}}} = 4,700\; \rm{mL}\) or 4.7 x 103 mL 2SF, not ambiguous |
\( 18 \; \cancel{\rm{ms}} \times \dfrac{10^{-3}\; \rm{s}}{1 \; \cancel{\rm{ms}}} = 0.018\; \rm{s}\) or \( 18 \; \cancel{\rm{ms}} \times \dfrac{1\; \rm{s}}{1,000 \; \cancel{\rm{ms}}} = 0.018\; \rm{s}\) |
| Think about your result. | The amount in mL should be 1000 times larger than the given amount in L. | The amount in s should be 1/1000 the given amount in ms. |
Perform each conversion.
- 101,000 ns to seconds
- 32.08 kg to grams
- 1.53 grams to cg
- Answer a:
- \(1.01 x 10^{-4} s \)
- Answer b:
- \(3.208 x 10^{4} g \)
- Answer c:
- \(1.53 x 10^{2} cg \)
Contributions & Attributions
This page was constructed from content via the following contributor(s) and edited (topically or extensively) by the LibreTexts development team to meet platform style, presentation, and quality:
Henry Agnew (UC Davis)

