Equilibrium Constant
For the hypothetical chemical reaction:
\[aA + bB \rightleftharpoons cC + dD\]
the equilibrium constant is defined as:
\[K_C = \dfrac{[C]^c[D]^d}{[A]^a[B]^b}\]
where the notation [A] signifies the molar concentration of species A. Note that the expression for the equilibrium constant includes only solutes and gases; pure solids and liquids do not appear in the expression. For example, the equilibrium expression for the reaction
\[\ce{CaH_2 (s) + 2H_2O (g) \rightleftharpoons Ca(OH)_2 (s) + 2H_2 (g)} \]
is the following:
\[K_C = \dfrac{[H_2]^2}{[H_2O]^2}\]
Observe that the gas-phase species \(\ce{H2O}\) and \(\ce{H2}\) appear in the expression but the solids \(\ce{CaH2}\) and \(\ce{Ca(OH)2}\) do not appear.
The equilibrium constant is most readily determined by allowing a reaction to reach equilibrium, measuring the concentrations of the various solution-phase or gas-phase reactants and products, and substituting these values into the Law of Mass Action.
Free Energy
The interaction between enthalpy and entropy changes in chemical reactions is best observed by studying their influence on the equilibrium constants of reversible reactions. To this end a new thermodynamic function called Free Energy (or Gibbs Free Energy), symbol \(ΔG\), is defined as shown in the first equation below. Two things should be apparent from this equation. First, in cases where the entropy change is small, \(ΔG ≅ ΔH\). Second, the importance of \(ΔS\) in determining \(ΔG\) increases with increasing temperature.
\[ΔGº = ΔHº – TΔSº\]
where the temperature is measured in absolute temperature (K).
The free energy function provides improved insight into the thermodynamic driving forces that influence reactions. A negative \(ΔGº\) is characteristic of an exergonic reaction, one which is thermodynamically favorable and often spontaneous, as is the melting of ice at 1 ºC. Likewise a positive \(ΔGº\) is characteristic of an endergonic reaction, one which requires an input of energy from the surroundings.
For an example of the relationship of free energy to enthalpy consider the decomposition of cyclobutane to ethene, shown in the following equation. The standard state for all the compounds is gaseous.
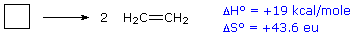
This reaction is endothermic, but the increase in number of molecules from one (reactants) to two (products) results in a large positive ΔSº.
At 25 ºC (298 K):
ΔGº = 19 kcal/mol – 298(43.6) cal/mole = 19 – 13 kcal/mole = +6 kcal/mole.
Thus, the entropy change opposes the enthalpy change, but is not sufficient to change the sign of the resulting free energy change, which is endergonic. Indeed, cyclobutane is perfectly stable when kept at room temperature.
Because the entropy contribution increases with temperature, this energetically unfavorable transformation can be made favorable by raising the temperature. At 200 ºC (473 K),
\[\begin{align} ΔGº &= 19\, kcal/mol – 473(43.6)\, cal/mole \\[4pt] &= 19 – 20.6\, kcal/mole \\[4pt] &= –1.6 kcal/mole.\end{align}\]
This is now an exergonic reaction, and the thermal cracking of cyclobutane to ethene is known to occur at higher temperatures.
\[ΔGº = –RT \ln K = –2.303 RT \log_{10} K \label{eq2}\]
where R = 1.987 cal/ K mole T = temperature in K and K = equilibrium constant
Note
Equation \ref{eq2} is important because it demonstrates the fundamental relationship of \(ΔGº\) to the equilibrium constant, \(K\). Because of the negative logarithmic relationship between these variables, a negative ΔGº generates a K>1, whereas a positive ΔGº generates a K<1. When ΔGº = 0, K = 1. Furthermore, small changes in ΔGº produce large changes in K. A change of 1.4 kcal/mole in ΔGº changes K by approximately a factor of 10. This interrelationship may be explored with the calculator on the right. Entering free energies outside the range -8 to 8 kcal/mole or equilibrium constants outside the range 10-6 to 900,000 will trigger an alert, indicating the large imbalance such numbers imply.
Applications to Organic Reactions
The equation below can also be useful without performing any calculations.
\[ΔGº = –RT \ln K = –2.303 RT \log_{10} K \label{eq2}\]
Conceptually, this equation helps us compare the energetics of reaction mechanisms to predict the major products. For example, if ΔG° < 0, then K > 1, and products are favored over reactants. If ΔG° > 0, then K < 1, and reactants are favored over products. If ΔG° = 0, then K = 1, and the system is at equilibrium. Recognizing the underlying energetics of equilibrium, the stability of charged reactants and products can be used to predict reaction equilibrium. Reaction conditions can also be adjusted or controlled to shift the equilibrium in the desired direction by a range of experimental methods. A theoretical understanding of the reaction free energy and equilibrium helps us predict and design the optimum reaction conditions for a desired product.
Exercises
- At 155°C, the equilibrium constant, Keq, for the reaction
\[\ce{\sf{CH3CO2H + CH3CH2OH <=> CH3CO2CH2CH3 + H2O}}\]
has a value of 4.0. Calculate ΔG° for this reaction at 155°C.
- Acetylene (C2H2) can be converted into benzene (C6H6) according to the equation:
\[\ce{\sf{3H-C#C-H (g) <=> C6H6 (l)}}\]
At 25°C, ΔG° for this reaction is −503 kJ and ΔH° is −631 kJ. Determine ΔS° and indicate whether the size of ΔS° agrees with what you would have predicted simply by looking at the chemical equation.
- Answer
-
Δ G ° = − R T ln K eq = − ( 8.314 J ⋅ K − 1 ⋅ mol − 1 ) ( 428 K ) ln ( 4.0 ) = − ( 8.314 J ⋅ K − 1 ⋅ mol − 1 ) ( 428 K ) ( 1.386 ) = − 4.9 × 10 3 J ⋅ mol − 1 = − 4.9 KJ ⋅ mol − 1 Δ G ° = Δ H ° − T Δ S ° Δ S ° = ( Δ H ° − Δ G ° ) T = − 631 kJ − ( − 503 kJ ) 298 K = − 128 kJ 298 K = − 0.430 KJ ⋅ mol − 1 = − 430 J ⋅ mol − 1
The entropy change is negative, as one would expect from looking at the chemical equation, since three moles of reactants yield one mole of product; that is, the system becomes much more “ordered” as it goes from reactants to products.