9.1: Overview of Titrimetry
- Page ID
- 220733
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In titrimetry we add a reagent, called the titrant, to a solution that contains another reagent, called the titrand, and allow them to react. The type of reaction provides us with a simple way to divide titrimetry into four categories: acid–base titrations, in which an acidic or basic titrant reacts with a titrand that is a base or an acid; complexometric titrations , which are based on metal–ligand complexation; redox titrations, in which the titrant is an oxidizing or reducing agent; and precipitation titrations, in which the titrand and titrant form a precipitate.
We will deliberately avoid the term analyte at this point in our introduction to titrimetry. Although in most titrations the analyte is the titrand, there are circumstances where the analyte is the titrant. Later, when we discuss specific titrimetric methods, we will use the term analyte where appropriate.
Despite their difference in chemistry, all titrations share several common features. Before we consider individual titrimetric methods in greater detail, let’s take a moment to consider some of these similarities. As you work through this chapter, this overview will help you focus on the similarities between different titrimetric methods. You will find it easier to understand a new analytical method when you can see its relationship to other similar methods.
Equivalence Points and End Points
If a titration is to give an accurate result we must combine the titrand and the titrant in stoichiometrically equivalent amounts. We call this stoichiometric mixture the equivalence point. Unlike precipitation gravimetry, where we add the precipitant in excess, an accurate titration requires that we know the exact volume of titrant at the equivalence point, Veq. The product of the titrant’s equivalence point volume and its molarity, MT, is equal to the moles of titrant that react with the titrand.
\[\text { moles titrant }=M_{T} \times V_{e q} \nonumber\]
If we know the stoichiometry of the titration reaction, then we can calculate the moles of titrand.
Unfortunately, for most titration reactions there is no obvious sign when we reach the equivalence point. Instead, we stop adding the titrant at an end point of our choosing. Often this end point is a change in the color of a substance, called an indicator, that we add to the titrand’s solution. The difference between the end point’s volume and the equivalence point’s volume is a determinate titration error. If the end point and the equivalence point volumes coincide closely, then this error is insignificant and is safely ignored. Clearly, selecting an appropriate end point is of critical importance.
Volume as a Signal
Instead of measuring the titrant’s volume, we may choose to measure its mass. Although generally we can measure mass more precisely than we can measure volume, the simplicity of a volumetric titration makes it the more popular choice.
Almost any chemical reaction can serve as a titrimetric method provided that it meets the following four conditions. The first condition is that we must know the stoichiometry between the titrant and the titrand. If this is not the case, then we cannot convert the moles of titrant used to reach the end point to the moles of titrand in our sample. Second, the titration reaction effectively must proceed to completion; that is, the stoichiometric mixing of the titrant and the titrand must result in their complete reaction. Third, the titration reaction must occur rapidly. If we add the titrant faster than it can react with the titrand, then the end point and the equivalence point will differ significantly. Finally, we must have a suitable method for accurately determining the end point. These are significant limitations and, for this reason, there are several common titration strategies.
Depending on how we are detecting the endpoint, we may stop the titration too early or too late. If the end point is a function of the titrant’s concentration, then adding the titrant too quickly leads to an early end point. On the other hand, if the end point is a function of the titrant’s concentration, then the end point exceeds the equivalence point.
A simple example of a titration is an analysis for Ag+ using thiocyanate, SCN–, as a titrant.
\[\mathrm{Ag}^{+}(a q)+\mathrm{SCN}^{-}(a q)\rightleftharpoons\mathrm{Ag}(\mathrm{SCN})(s) \nonumber\]
This reaction occurs quickly and with a known stoichiometry, which satisfies two of our requirements. To indicate the titration’s end point, we add a small amount of Fe3+ to the analyte’s solution before we begin the titration. When the reaction between Ag+ and SCN– is complete, formation of the red-colored Fe(SCN)2+ complex signals the end point. This is an example of a direct titration since the titrant reacts directly with the analyte.
This is an example of a precipitation titration. You will find more information about precipitation titrations later in this chapter.
If the titration’s reaction is too slow, if a suitable indicator is not available, or if there is no useful direct titration reaction, then an indirect analysis may be possible. Suppose you wish to determine the concentration of formaldehyde, H2CO, in an aqueous solution. The oxidation of H2CO by \(\text{I}_3^-\)
\[\mathrm{H}_{2} \mathrm{CO}(a q)+\mathrm{I}_{3}^-(a q)+3 \mathrm{OH}^{-}(a q)\rightleftharpoons\mathrm{HCO}_{2}^{-}(a q)+3 \mathrm{I}^{-}(a q)+2 \mathrm{H}_{2} \mathrm{O}(1) \nonumber\]
is a useful reaction, but it is too slow for a titration. If we add a known excess of \(\text{I}_3^-\) and allow its reaction with H2CO to go to completion, we can titrate the unreacted \(\text{I}_3^-\) with thiosulfate, \(\text{S}_2\text{O}_3^{2-}\).
\[\mathrm{I}_{3}^{-}(a q)+2 \mathrm{S}_{2} \mathrm{O}_{3}^{2-}(a q)\rightleftharpoons\mathrm{S}_{4} \mathrm{O}_{6}^{2-}(a q)+3 \mathrm{I}^{-}(a q) \nonumber\]
The difference between the initial amount of \(\text{I}_3^-\) and the amount in excess gives us the amount of \(\text{I}_3^-\) that reacts with the formaldehyde. This is an example of a back titration.
This is an example of a redox titration. You will find more information about redox titrations later in this chapter.
Calcium ions play an important role in many environmental systems. A direct analysis for Ca2+ might take advantage of its reaction with the ligand ethylenediaminetetraacetic acid (EDTA), which we represent here as Y4–.
\[\mathrm{Ca}^{2+}(a q)+\mathrm{Y}^{4-}(a q)\rightleftharpoons\mathrm{CaY}^{2-}(a q) \nonumber\]
Unfortunately, for most samples this titration does not have a useful indicator. Instead, we react the Ca2+ with an excess of MgY2–
\[\mathrm{Ca}^{2+}(a q)+\mathrm{MgY}^{2-}(a q)\rightleftharpoons\mathrm{Ca} \mathrm{Y}^{2-}(a q)+\mathrm{Mg}^{2+}(a q) \nonumber\]
releasing an amount of Mg2+ equivalent to the amount of Ca2+ in the sample. Because the titration of Mg2+ with EDTA
\[\mathrm{Mg}^{2+}(a q)+\mathrm{Y}^{4-}(a q)\rightleftharpoons\mathrm{MgY}^{2-}(a q) \nonumber\]
has a suitable end point, we can complete the analysis. The amount of EDTA used in the titration provides an indirect measure of the amount of Ca2+ in the original sample. Because the species we are titrating was displaced by the analyte, we call this a displacement titration.
MgY2– is the Mg2+–EDTA metal–ligand complex. You can prepare a solution of MgY2– by combining equimolar solutions of Mg2+ and EDTA. This is an example of a complexation titration. You will find more information about complexation titrations later in this chapter.
If a suitable reaction with the analyte does not exist it may be possible to generate a species that we can titrate. For example, we can determine the sulfur content of coal by using a combustion reaction to convert sulfur to sulfur dioxide
\[\mathrm{S}(s)+\mathrm{O}_{2}(g) \rightarrow \mathrm{SO}_{2}(g) \nonumber\]
and then convert the SO2 to sulfuric acid, H2SO4, by bubbling it through an aqueous solution of hydrogen peroxide, H2O2.
\[\mathrm{SO}_{2}(g)+\mathrm{H}_{2} \mathrm{O}_{2}(a q) \longrightarrow \mathrm{H}_{2} \mathrm{SO}_{4}(a q) \nonumber\]
Titrating H2SO4 with NaOH
\[\mathrm{H}_{2} \mathrm{SO}_{4}(a q)+2 \mathrm{NaOH}(a q)\rightleftharpoons2 \mathrm{H}_{2} \mathrm{O}(l )+\mathrm{Na}_{2} \mathrm{SO}_{4}(a q) \nonumber\]
provides an indirect determination of sulfur.
This is an example of an acid–base titration. You will find more information about acid–base titrations later in this chapter.
Titration Curves
To find a titration’s end point, we need to monitor some property of the reaction that has a well-defined value at the equivalence point. For example, the equivalence point for a titration of HCl with NaOH occurs at a pH of 7.0. A simple method for finding the equivalence point is to monitor the titration mixture’s pH using a pH electrode, stopping the titration when we reach a pH of 7.0. Alternatively, we can add an indicator to the titrand’s solution that changes color at a pH of 7.0.
Why a pH of 7.0 is the equivalence point for this titration is a topic we will cover later in the section on acid–base titrations.
Suppose the only available indicator changes color at a pH of 6.8. Is the difference between this end point and the equivalence point small enough that we safely can ignore the titration error? To answer this question we need to know how the pH changes during the titration.
A titration curve provides a visual picture of how a property of the titration reaction changes as we add the titrant to the titrand. The titration curve in Figure \(\PageIndex{1}\), for example, was obtained by suspending a pH electrode in a solution of 0.100 M HCl (the titrand) and monitoring the pH while adding 0.100 M NaOH (the titrant). A close examination of this titration curve should convince you that an end point pH of 6.8 produces a negligible titration error. Selecting a pH of 11.6 as the end point, however, produces an unacceptably large titration error.
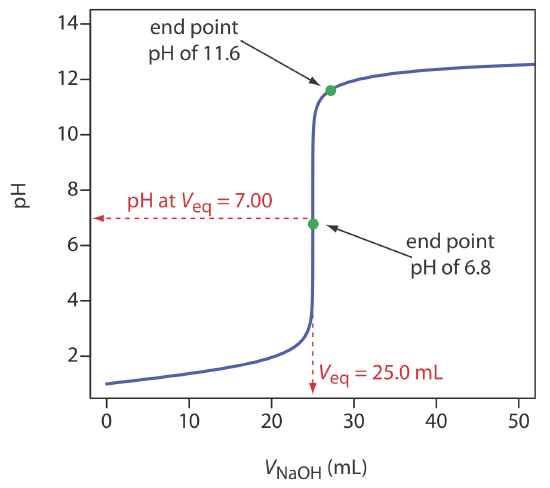
For the titration curve in Figure \(\PageIndex{1}\), the volume of titrant to reach a pH of 6.8 is 24.99995 mL, a titration error of \(-2.00 \times 10^{-4}\)% relative to the equivalence point of 25.00 mL. Typically, we can read the volume only to the nearest ±0.01 mL, which means this uncertainty is too small to affect our results. The volume of titrant to reach a pH of 11.6 is 27.07 mL, or a titration error of +8.28%. This is a significant error.
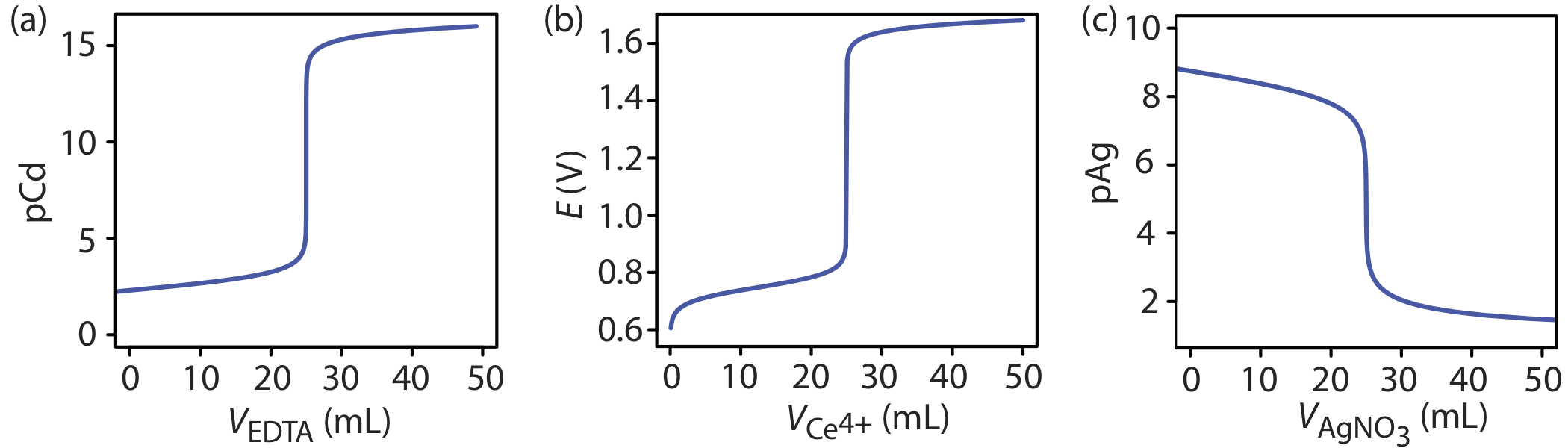
The shape of the titration curve in Figure \(\PageIndex{1}\) is not unique to an acid–base titration. Any titration curve that follows the change in concentration of a species in the titration reaction (plotted logarithmically) as a function of the titrant’s volume has the same general sigmoidal shape. Several additional examples are shown in Figure \(\PageIndex{2}\).
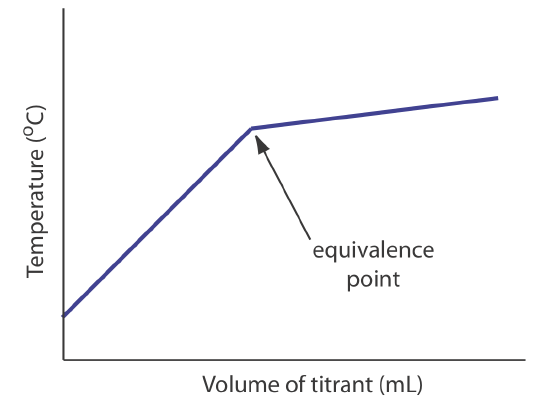
The titrand’s or the titrant’s concentration is not the only property we can use to record a titration curve. Other parameters, such as the temperature or absorbance of the titrand’s solution, may provide a useful end point signal. Many acid–base titration reactions, for example, are exothermic. As the titrant and the titrand react, the temperature of the titrand’s solution increases. Once we reach the equivalence point, further additions of titrant do not produce as exothermic a response. Figure \(\PageIndex{3}\) shows a typical thermometric titration curve where the intersection of the two linear segments indicates the equivalence point.
The Buret
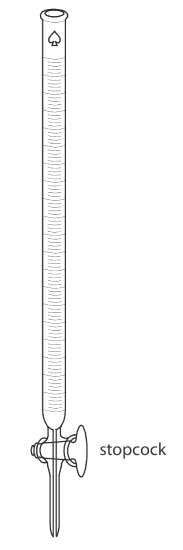
The only essential equipment for an acid–base titration is a means for delivering the titrant to the titrand’s solution. The most common method for delivering titrant is a buret (Figure \(\PageIndex{4}\)), which is a long, narrow tube with graduated markings and equipped with a stopcock for dispensing the titrant. The buret’s small internal diameter provides a better defined meniscus, making it easier to read precisely the titrant’s volume. Burets are available in a variety of sizes and tolerances (Table \(\PageIndex{1}\)), with the choice of buret determined by the needs of the analysis. You can improve a buret’s accuracy by calibrating it over several intermediate ranges of volumes using the method described in Chapter 5 for calibrating pipets. Calibrating a buret corrects for variations in the buret’s internal diameter.
An automated titration uses a pump to deliver the titrant at a constant flow rate (Figure \(\PageIndex{5}\)). Automated titrations offer the additional advantage of using a microcomputer for data storage and analysis.



