5.6: Diastereomers
- Page ID
- 67098
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)After completing this section, you should be able to
- calculate the maximum number of stereoisomers possible for a compound containing a specified number of chiral carbon atoms.
- draw wedge-and-broken-line structures for all possible stereoisomers of a compound containing two chiral carbon atoms, with or without the aid of molecular models.
- assign R,S configurations to wedge-and-broken-line structures containing two chiral carbon atoms, with or without the aid of molecular models.
- determine, with or without the aid of molecular models, whether two wedge-and-broken-line structures containing two chiral carbon atoms are identical, represent a pair of enantiomers, or represent a pair of diastereomers.
- draw the wedge-and-broken-line structure of a specific stereoisomer of a compound containing two chiral carbon atoms, given its IUPAC name and R,S configuration.
Make certain that you can define, and use in context, the key term below.
- diastereomer
Diastereomers are stereoisomers that are not related as object and mirror image and are not enantiomers. Unlike enatiomers which are mirror images of each other and non-sumperimposable, diastereomers are not mirror images of each other and non-superimposable. Diastereomers can have different physical properties and reactivity. They have different melting points and boiling points and different densities. They have two or more stereocenters.
Introduction
It is easy to mistake between diasteromers and enantiomers. For example, we have four steroisomers of 3-bromo-2-butanol. The four possible combination are SS, RR, SR and RS (Figure 5.6.1). One of the molecule is the enantiomer of its mirror image molecule and diasteromer of each of the other two molecule (SS is enantiomer of RR and diasteromer of RS and SR). SS's mirror image is RR and they are not superimposable, so they are enantiomers. RS and SR are not mirror image of SS and are not superimposable to each other, so they are diasteromers.
.png?revision=1&size=bestfit&width=720&height=519)
Figure 5.6.1
Diastereomers vs. Enantiomers
Tartaric acid, C4H6O6, is an organic compound that can be found in grape, bananas, and in wine. The structures of tartaric acid itself is really interesting. Naturally, it is in the form of (R,R) stereocenters. Artificially, it can be in the meso form (R,S), which is achiral. R,R tartaric acid is enantiomer to is mirror image which is S,S tartaric acid and diasteromers to meso-tartaric acid (Figure 5.6.2).
(R,R) and (S,S) tartaric acid have similar physical properties and reactivity. However, meso-tartaric acid have different physical properties and reactivity. For example, melting point of (R,R) & (S,S) tartaric is about 170 degree Celsius, and melting point of meso-tartaric acid is about 145 degree Celsius.
.bmp?revision=1&size=bestfit&width=720&height=567)
Figure 5.6.2
We turn our attention next to molecules which have more than one stereocenter. We will start with a common four-carbon sugar called D-erythrose.
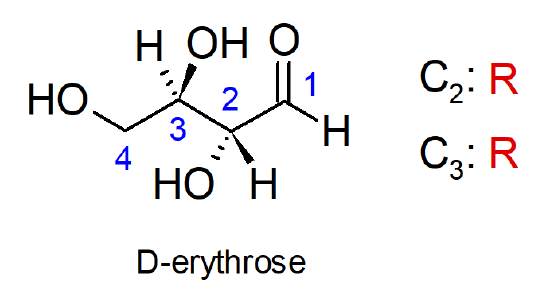
A note on sugar nomenclature: biochemists use a special system to refer to the stereochemistry of sugar molecules, employing names of historical origin in addition to the designators 'D' and 'L'. You will learn about this system if you take a biochemistry class. We will use the D/L designations here to refer to different sugars, but we won't worry about learning the system.
As you can see, D-erythrose is a chiral molecule: C2 and C3 are stereocenters, both of which have the R configuration. In addition, you should make a model to convince yourself that it is impossible to find a plane of symmetry through the molecule, regardless of the conformation. Does D-erythrose have an enantiomer? Of course it does – if it is a chiral molecule, it must. The enantiomer of erythrose is its mirror image, and is named L-erythrose (once again, you should use models to convince yourself that these mirror images of erythrose are not superimposable).
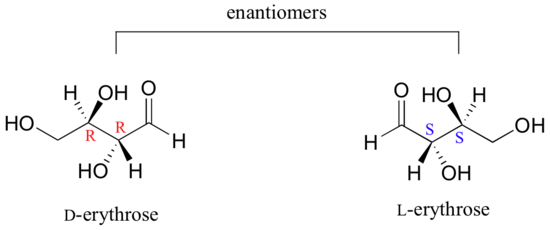
Notice that both chiral centers in L-erythrose both have the S configuration.
In a pair of enantiomers, all of the chiral centers are of the opposite configuration.
What happens if we draw a stereoisomer of erythrose in which the configuration is S at C2 and R at C3? This stereoisomer, which is a sugar called D-threose, is not a mirror image of erythrose. D-threose is a diastereomer of both D-erythrose and L-erythrose.
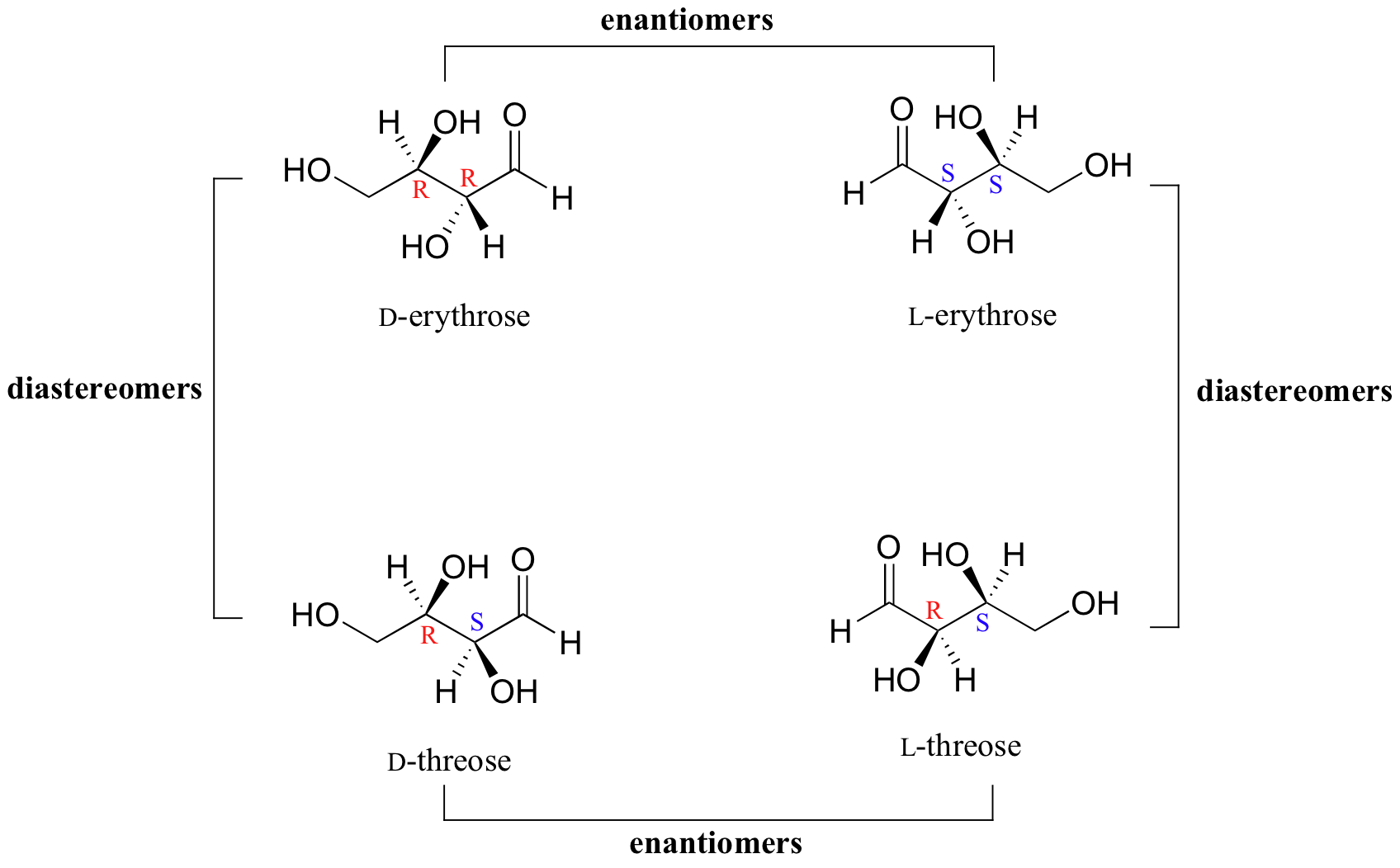
The definition of diastereomers is simple: if two molecules are stereoisomers (same molecular formula, same connectivity, different arrangement of atoms in space) but are not enantiomers, then they are diastereomers by default. In practical terms, this means that at least one - but not all - of the chiral centers are opposite in a pair of diastereomers. By definition, two molecules that are diastereomers are not mirror images of each other.
L-threose, the enantiomer of D-threose, has the R configuration at C2 and the S configuration at C3. L-threose is a diastereomer of both erythrose enantiomers.
In general, a structure with n stereocenters will have 2n different stereoisomers. (We are not considering, for the time being, the stereochemistry of double bonds – that will come later). For example, let's consider the glucose molecule in its open-chain form (recall that many sugar molecules can exist in either an open-chain or a cyclic form). There are two enantiomers of glucose, called D-glucose and L-glucose. The D-enantiomer is the common sugar that our bodies use for energy. It has n = 4 stereocenters, so therefore there are 2n = 24 = 16 possible stereoisomers (including D-glucose itself).
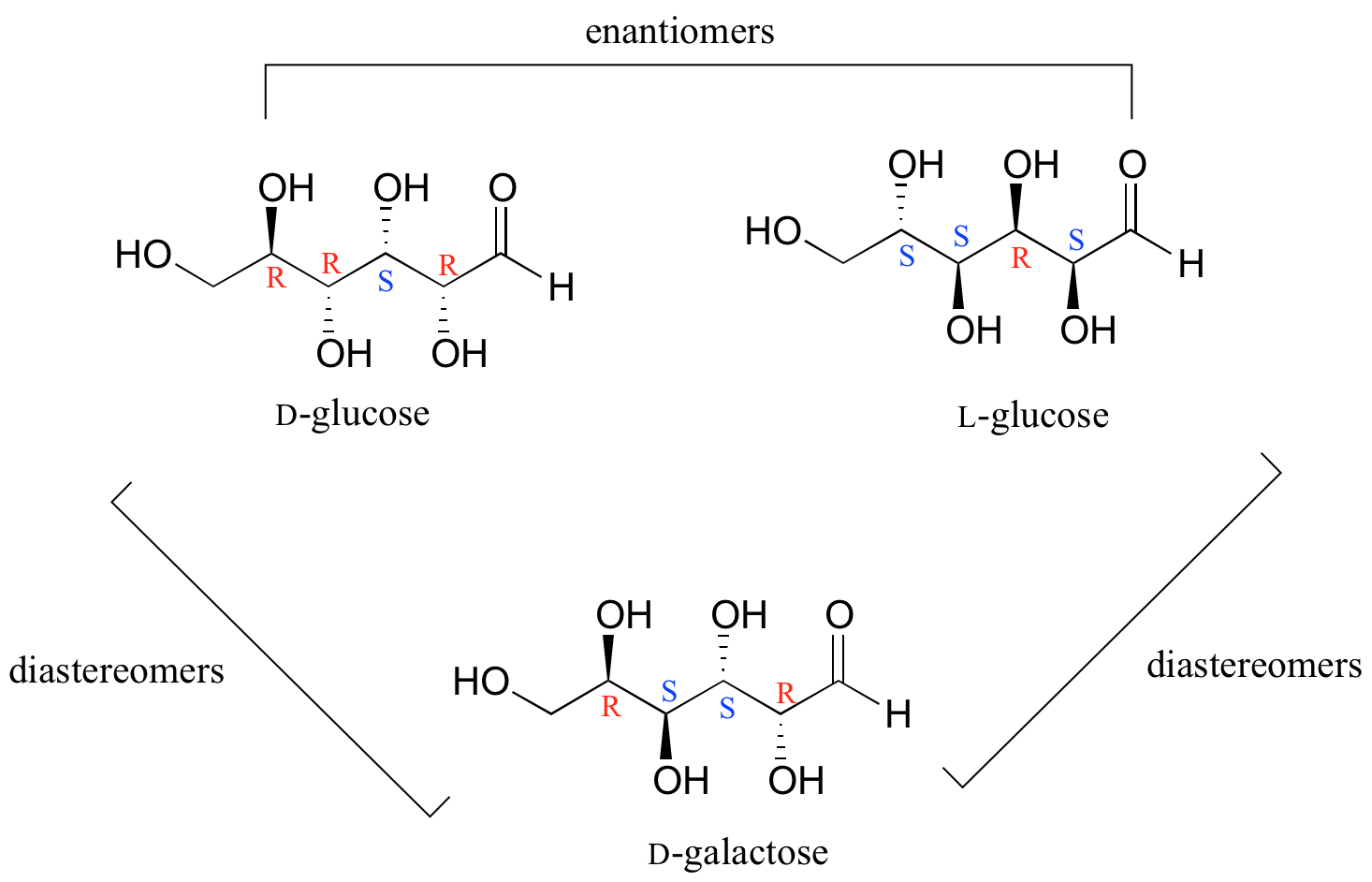
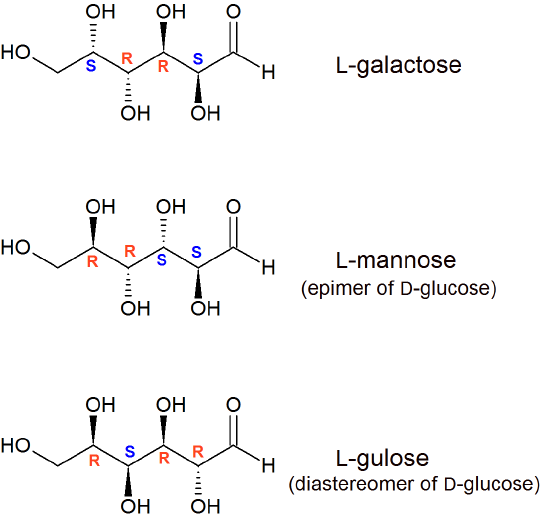
In L-glucose, all of the stereocenters are inverted relative to D-glucose. That leaves 14 diastereomers of D-glucose: these are molecules in which at least one, but not all, of the stereocenters are inverted relative to D-glucose. One of these 14 diastereomers, a sugar called D-galactose, is shown above: in D-galactose, one of four stereocenters is inverted relative to D-glucose. Diastereomers which differ in only one stereocenter (out of two or more) are called epimers. D-glucose and D-galactose can therefore be refered to as epimers as well as diastereomers.
Draw the structure of L-galactose, the enantiomer of D-galactose.
Draw the structure of two more diastereomers of D-glucose. One should be an epimer.
- Answer
Erythronolide B, a precursor to the 'macrocyclic' antibiotic erythromycin, has 10 stereocenters. It’s enantiomer is that molecule in which all 10 stereocenters are inverted.
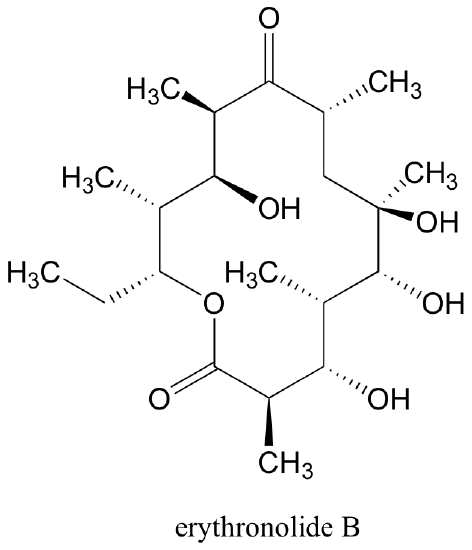
In total, there are 210 = 1024 stereoisomers in the erythronolide B family: 1022 of these are diastereomers of the structure above, one is the enantiomer of the structure above, and the last is the structure above.
We know that enantiomers have identical physical properties and equal but opposite degrees of specific rotation. Diastereomers, in theory at least, have different physical properties – we stipulate ‘in theory’ because sometimes the physical properties of two or more diastereomers are so similar that it is very difficult to separate them. In addition, the specific rotations of diastereomers are unrelated – they could be the same sign or opposite signs, and similar in magnitude or very dissimilar.
Exercises
Determine the number of stereoisomers a molecule can have with…
- 3 chiral centers
- 1 chiral center
- 6 chiral centers
- Answer
-
Since a molecule with n chiral centers can have 2n stereoisomers…
- 23 = 8 possible stereoisomers
- 21 = 2 possible stereoisomers
- 26 = 64 possible stereoisomers
What is the relationship between enantiomers?
- Answer
-
They are mirror images of each other and when 2 or more chiral centers are present, every stereocenter is the opposite in its enantiomer.
How does the stereochemistry in diastereomers differ from each other?
- Answer
-
In diastereomers, one or more of the chiral centers is the opposite but they all can’t be the opposite or else they’d be enantiomers.
What are epimers?
- Answer
-
Epimers are when only one chiral center is the opposite (in molecules with 2 or more chiral centers) in its diastereomer.
Draw the structure of (2R,3R) 2-fluoro-3-methylpentane.
- Answer
-
Draw both diastereomers of (2R,3R) 2-fluoro-3-methylpentane.
- Answer
-
Draw the enantiomer of (2R,3R) 2-fluoro-3-methylpentane.
- Answer
-
Draw the structure of L-galactose, the enantiomer of D-galactose.
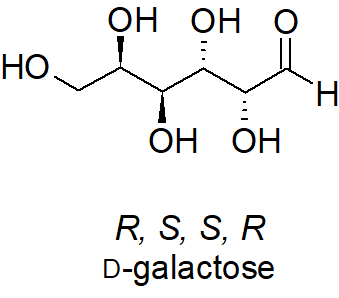
- Answer
-
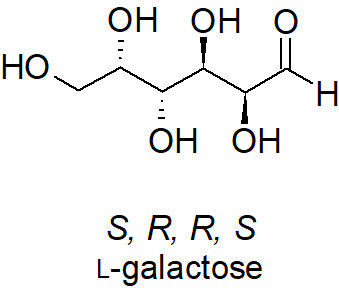
Draw a diastereomer of D-galactose that is an epimer.
- Answer
-
You can draw an epimer by drawing D-galactose with 1 (and only 1) of its chiral centers reversed. Here’s an example when you switch only the first chiral center (in red). (There are 3 other epimers that could be drawn as long as you only swap a single chiral center in the diastereomer that you use.)
Identify if the following diastereomer of galactose is an epimer of D- galactose or L- galactose.
- Answer
-
Since the diastereomer above only varies from L-galactose by 1 chiral center, the above is an epimer in relationship to L-galactose. Since it varies from D-galactose by 3 chiral centers, it is not an epimer but a diastereomer. Since not all of the chiral centers are swapped, it is not an enantiomer!
For the compound shown below, label each chiral center as R or S.
- Answer
-
How many stereoisomers are possible for the compound in part a)?
- Answer
-
Since there are 3 chiral centers, 23 = 8 possible stereoisomers.
Consider the stereoisomers below.
- Which is/are an enantiomer of i?
- Which is/are a diastereomer of ii?
- Which is/are an epimer of i?
- Answer
-
- iv is an enantiomer of i since both chiral centers are switched and they are non superimposable mirror images.
- i & iv are diastereomers of ii since they are stereoisomers that are not mirror images.
- ii and iii are epimers of i since they are diastereomers with only 1 chiral center switched and the other one the same.
Consider the 8 stereoisomers below.
- Which is/are an enantiomer of i?
- Which is/are a diastereomer of i?
- Which is/are an epimer of i?
- Answer
-
- v is an enantiomer since all three chiral centers are switched and they are non superimposable mirror images.
- ii, iii, iv, vi, vii & viii are diastereomers of i since they are stereoisomers that are not mirror images.
- ii,iii & viii are epimers of i since they are diastereomers with only 1 chiral center switched and the other chiral centers the same.
Contributors and Attributions
Organic Chemistry With a Biological Emphasis by Tim Soderberg (University of Minnesota, Morris)
Dr. Dietmar Kennepohl FCIC (Professor of Chemistry, Athabasca University)

