10.7: Periodic Trends

⚙️ Learning Objectives

• Be able to state how certain properties of atoms vary based on their relative position on the periodic table.

One of the reasons the periodic table is so useful is because its structure allows us to qualitatively determine how some properties of the elements vary versus their position on the periodic table. The variations of properties versus positions on the periodic table are called periodic trends. There is no other tool in science that allows us to judge relative properties of a class of objects like this, which makes the periodic table a very useful tool. Many periodic trends are general. There may be a few points where an opposite trend is seen, but there is an overall trend when considered across a whole row or down a whole column of the periodic table.
 

Atomic Size

The first periodic trend we will consider is atomic radius. The atomic radius is an indication of the size of an atom. Although the concept of a definite radius of an atom is a bit fuzzy, atoms behave as if they have a certain radius. Such radii can be estimated from various experimental techniques, such as the x-ray crystallography of crystals.

As mentioned in Section 10.5, orbitals get larger with more energy. The energy level with the lowest energy, designated as n = 1, also has the smallest orbital and its electrons are therefore closest to the nucleus. As the value of the energy level (n) increases, the orbitals become larger. In addition, a higher energy level has more orbitals than a lower energy level and hence can hold more electrons. This is in agreement with predictions made by Bohr that the maximum number of electrons allowed in a particular energy level, n, would be 2n². As we build atoms with more and more electrons, those electrons need to move to higher energy levels as lower ones are filled. For main group elements, the highest energy level containing its valence electrons corresponds to the row that the atom is in on the periodic table. Thus, magnesium's two valence electrons are in n = 3, and iodine's seven valence electrons are in n = 5. 

We can use these concepts of energy levels and orbital size to describe two trends seen experimentally. The first is that as you go down a column of the periodic table, the atomic radii increase. This is because as we move down a column, the valence electrons occupy higher energy levels. The higher energy level will have larger orbitals, so the valence electrons are physically farther away from the nucleus. This trend can be summarized as follows:

\(\mathrm{as}\downarrow\mathrm{PT},\mathrm{atomic}\;\mathrm{radius}\uparrow\)

where PT stands for periodic table.

The second trend is observed when going across a row on the periodic table, from left to right. Even though the valence electrons are going into the same energy level, the number of protons—and hence the positive charge of the nucleus—is increasing as you go across the row. The increasing positive charge casts a tighter grip on the valence electrons, so as you go across the periodic table, the atomic radii decrease. Again, we can summarize this trend as follows:

\(\mathrm{as}\rightarrow\mathrm{PT},\mathrm{atomic}\;\mathrm{radius}\downarrow\)

Combining these two trends together, we see that the smallest atoms will be in the upper right corner of the periodic table and the largest atoms will be in the lower left corner. Figure \(\PageIndex{1}\) shows spheres representing the atoms of the main group elements from the periodic table to scale, showing the two trends for the atomic radius.
 

Figure \(\PageIndex{1}\): Atomic radii trends on the periodic table. Although there are some reversals in the trend (e.g., see Po in the bottom row), atoms generally get smaller as you go across the periodic table and larger as you go down any one column. Numbers are the radii in pm.

 

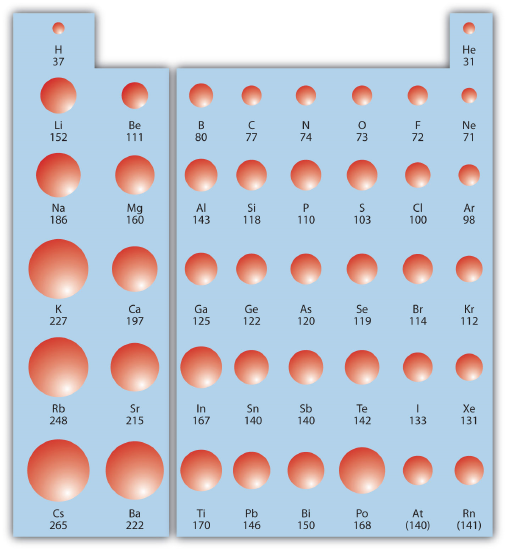
✅ Example \(\PageIndex{1}\): Atomic Radii

Referring only to a periodic table and not to Figure \(\PageIndex{1}\), which atom is larger in each pair?

1. Si or S
2. S or Te

Solution

1. Si is to the left of S on the periodic table; it is larger because as you go across the row, the atoms get smaller.
2. S is above Te on the periodic table; Te is larger because as you go down the column, the atoms get larger.

 

✏️ Exercise \(\PageIndex{1}\): Atomic Radii

Referring only to a periodic table and not to Figure \(\PageIndex{1}\), which atom is smaller, Ca or Br?

Answer

Br

Ionization Energy

Another property of atoms that exhibits periodic trends is ionization energy. Ionization energy (IE) is the amount of energy required to remove an electron from an atom in the gas phase:

\[\mathrm A(g)\;\rightarrow\;\mathrm A^+(g)\;+\;e^–\]

IE is usually expressed in kJ/mol of atoms. It is always positive because the removal of an electron always requires that energy be put in (i.e., it is endothermic). If the atom contains more electrons, the ionization energy for the removal of each additional electron can also be determined. For the purposes of this text, we will only consider the ionization energy for the removal of the first electron, known as the first ionization energy or IE₁.

The amount of energy that is required for IE₁ will depend on how strongly the electron is attracted to the nucleus. Electrons that are close to the nucleus will be held more tightly than electrons located farther away from the nucleus. Thus, as you go down the periodic table, it becomes easier to remove an electron from an atom (i.e., IE₁ decreases) because the valence electron is farther away from the nucleus. 

\(\mathrm{as}\downarrow\mathrm{PT},\;\mathrm{IE}\downarrow\)

Electrons will also be held more tightly by a greater positive charge. This is reflected in the trend observed as you go across the periodic table. The greater positive charge of the nucleus draws the electrons closer in, and it takes more energy to remove an electron. As a result, IE₁ increases.

\(\mathrm{as}\rightarrow\mathrm{PT},\;\mathrm{IE}\uparrow\)

Combining these two trends together for the main group elements, we see that the greatest first ionization energy occurs with the smallest atoms in the upper right corner of the periodic table while the lowest first ionization energy is found in the largest atoms in the lower right corner. Figure \(\PageIndex{2}\) shows values of IE₁ versus position on the periodic table. Again, the trend is not absolute, but the general trends going across and down the periodic table should be obvious.
 

Figure \(\PageIndex{2}\): Ionization energy on the periodic table. Values are in kJ/mol. Note that the larger atoms (based on the size of the spheres) tend to have the smallest IE values.

 

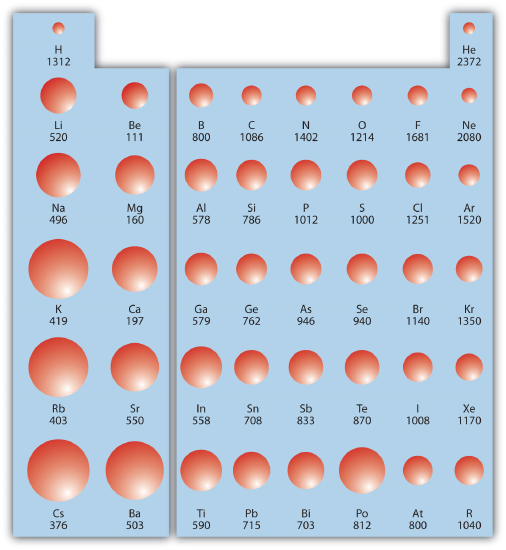
✅ Example \(\PageIndex{2}\): Ionization Energies

Which atom in each pair has the larger first ionization energy?

1. Ca or Sr
2. P or Cl

Solution

1. Because Sr is below Ca on the periodic table, it is easier to remove an electron from it; thus, Ca has the higher IE₁.
2. Because Cl is farther to the right, it has a more positive charge in its nucleus and it will be harder to remove an electron from it, so its IE₁ is larger than that of P. 

 

✏️ Exercise \(\PageIndex{2}\): Ionization Energies

Which atom has the lower ionization energy, C or F?

Answer

C

Metallic Character

The metallic character is used to define the chemical properties that metallic elements present. Generally, metals tend to lose electrons to form cations. Thus, atoms with low first ionization energies will be more likely to lose their valence electrons and have chemical properties of metallic elements. They also have a high oxidation potential—they are easily oxidized (lose electrons) and are strong reducing agents. Metals also form basic oxides; the more basic the oxide, the higher the metallic character. In contrast, nonmetals have high first ionization energies making it very difficult for them to form cations. Instead, they will tend to gain electrons to fill up their energy level and form anions.  

This metallic character is reflected in the regions of the periodic table described previously. Small atoms with greater first ionization energies are in the upper right corner of the periodic table. This area was designated as the nonmetals. Large atoms with lower first ionization energies are in the lower left corner of the periodic table in the region designated as the metals. The metallic character of the elements will therefore increase as we move down and to the left on the periodic table. 
 

Figure \(\PageIndex{3}\): Summary of periodic trends involving atomic size, first ionization energy, and metallic character. Arrows show how each property increases along rows and columns.

 

Summary

• Certain properties—notably atomic radius, ionization energies, and metallic character—can be qualitatively understood by the positions of the elements on the periodic table. The major trends are summarized in the Figure \(\PageIndex{3}\) above. 
• There are two factors that help in the prediction of the trends in the periodic table: energy level of the valence electrons and the number of protons in the nucleus.

 

 

---

This page is shared under a CK-12 license and was authored, remixed, and/or curated by Vicki MacMurdo (Anoka-Ramsey Community College), Melissa Alviar-Agnew, Henry Agnew, and Lance S. Lund (Anoka-Ramsey Community College). Original source: https://www.ck12.org/c/chemistry/.

LICENSED UNDER