13.7: The Gibbs Free Energy of Formation and Equilibrium in Ideal Gas Reactions
- Page ID
- 152382
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In Section 13.6, we develop a relationship between the standard Gibbs free energy change for a reaction and the equilibrium constant for that reaction. The standard Gibbs free energy change for a reaction is a specific quantity of energy, which depends only on the temperature. In Chapter 11, we develop the Gibbs free energy of formation for a substance in its standard state. We assert that it is convenient to set the Gibbs free energy of every substance in its standard state equal to its Gibbs free energy of formation. Now we can see the reason: When we express the molar Gibbs free energy of an ideal gas as
\[{\overline{G}}_A\left(P_A\right)={\Delta }_fG^o\left(A,P^o\right)+RT{ \ln \frac{P_A}{P^o}\ } \nonumber \]
\({\overline{G}}_A\left(P_A\right)\) is the Gibbs free energy change for producing one mole of ideal gas \(A\), at pressure \(P_A\), from the elements at the same temperature. Because the Gibbs free energies of the reactants and products are measured from a common starting point, we can use them to calculate the Gibbs free energy change for their reaction.
To demonstrate the role of our choice of the elements as the reference state for the Gibbs free energies of chemical substances, we need only expand the Gibbs free energy cycle in Figure 4. In Figure 6, we introduce the Gibbs free energy change for producing the separate components of the very large system.
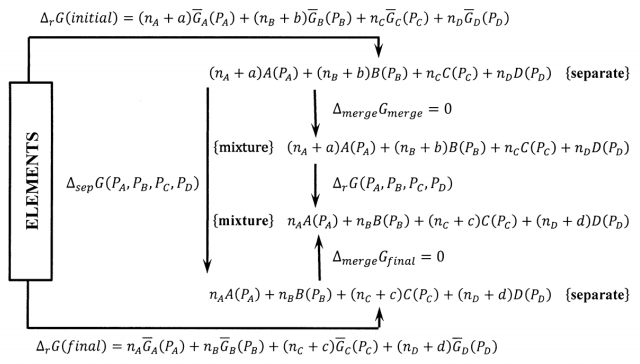
Computing the Gibbs free energy change in a clockwise direction around the cycle in which the elements are converted first to isolated reactants, then to components of a large equilibrium system, then to separated products, and finally back to the elements, we find
\[\mathrm{0=}{\mathrm{\Delta }}_fG\left(initial\right) + {\mathrm{\Delta }}_{merge}G_{initial} \nonumber \] \[ + {\mathrm{\Delta }}_rG\left(P_A\mathrm{,\ }P_B\mathrm{,\ }P_C\mathrm{,\ }P_D\right) + {\mathrm{\Delta }}_{merge}G_{final} + {\mathrm{\Delta }}_fG\left(final\right) \nonumber \]
Since the partial pressures \(P_A\), \(P_B\), \(P_C\), and \(P_D\) characterize the system at equilibrium, \({\mathrm{\Delta }}_rG\left(P_A\mathrm{,\ }P_B\mathrm{,\ }P_C\mathrm{,\ }P_D\right)\mathrm{=0}\). Also, \({\mathrm{\Delta }}_{merge}G_{initial} = {\mathrm{\Delta }}_{merge}G_{final}\)= 0, so the equation for the Gibbs free energy change around the cycle simplifies to
\[\begin{aligned} 0 & ={\Delta }_fG\left(initial\right)-{\Delta }_fG\left(final\right) \\ ~ & =a \overline{G}_A\left(p_A\right)+b \overline{G}_B\left(p_B\right)-c \overline{G}_C\left(p_C\right)-d \overline{G}_D\left(p_D\right) \\ ~ & =a\ {\Delta }_fG^o\left(A,P^o\right)+b\ {\Delta }_fG^o\left(B,P^o\right)-c {\Delta }_fG^o\left(C,P^o\right) -d {\Delta }_fG^o\left(D,P^o\right) +RT \ln p^a_A\ +RT \ln p^b_B -RT \ln p^c_C-RT \ln p^d_D \\ ~ & =-{\Delta }_rG^o-RT \ln \frac{p^c_Cp^d_D}{p^a_Ap^b_B} \end{aligned} \nonumber \]
So that we have
\[{\Delta }_rG^o=-RT{ \ln \frac{p^c_Cp^d_D}{p^a_Ap^b_B}\ } \nonumber \]
To illustrate the application of these ideas, let us consider equilibrium in the oxidation of nitric oxide to nitrogen dioxide
\[2\ NO+O_2\rightleftharpoons 2\ NO_2 \nonumber \]
At 800 K, the standard Gibbs free energy of formation of nitric oxide is \(+81.298\ \mathrm{kJ}\ {\mathrm{mol}}^{-1}\), and that of nitrogen dioxide is \(+83.893\ \mathrm{kJ}\ {\mathrm{mol}}^{-1}\). Hence, the standard Gibbs free energy change for the oxidation is \(+5,190\ \mathrm{J}\ {\mathrm{mol}}^{-1}\), and the equilibrium constant is
\[K_P=\mathrm{exp}\left(-\frac{{\Delta }_rG^o}{RT}\right)=0.458 \nonumber \]
Suppose that one mole of \(NO_2\) and one mole of \(O_2\) are mixed, that the mixture is thermostated at 800 K, and that the applied pressure remains constant at 10 bar while the reaction goes to equilibrium.
Let the number of moles of \(NO\) present at equilibrium be \(n_{NO}\). Then the moles of \(NO_2\) and \(O_2\) present at equilibrium are \(n_{NO_2}\mathrm{=1-}n_{NO}\) and \(n_{O_2}=1+{n_{NO}}/{2}\). The partial pressures are \(P_{NO}={n_{NO}RT}/{V}\), \(P_{NO_2}={n_{NO_2}RT}/{V}\), and \(P_{O_2}={n_{O_2}RT}/{V}\), so that
\[K_P=\frac{{\left(\mathrm{1-}n_{NO}\mathrm{\ }\right)}^2}{n^2_{NO}\left(1+{n_{NO}}/{2}\right)\left({RT}/{V}\right)} \nonumber \] The value of \(RT/V\) depends on the total pressure; we have
\[P=P_{NO}+P_{NO_2}+P_{O_2}=\left(2+{n_{NO}}/{2}\right)\left({RT}/{V}\right)=10\ \mathrm{bar} \nonumber \] so that
\[\frac{RT}{V}=\frac{20}{4+n_{NO}} \nonumber \]
and
\[K_P=\frac{{\left(\mathrm{1-}n_{NO}\mathrm{\ }\right)}^2\left(4+n_{NO}\right)}{10n^2_{NO}\left(2+n_{NO}\right)}=0.458 \nonumber \]
Solving, we find \(n_{NO}=0.388\).


