13.4: The Gibbs Free Energy Change for Reaction at Constant Partial Pressures
- Page ID
- 151742
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Now we can compare the difference between the Gibbs free energies of reactants and products in the reaction
\[a\ A+b\ B\ \rightleftharpoons \ c\ C+d\ D \nonumber \]
when all of the gases are present in the same system to the same difference when each gas is in its own container.
In the first case, the gases are present in a mixture, and their partial pressures remain constant at \(P_A\), \(P_B\), \(P_C\), and \(P_D\) respectively. We called the Gibbs free energy change under these conditions \({\Delta }_rG\). These conditions can be satisfied if we suppose that the system is very large. That is, \({\Delta }_rG\) is the limiting Gibbs free energy change for the conversion of an initial mixture into a final mixture. The initial mixture contains \(\left(n_A+a\right)\) moles of \(A\), \(\left(n_B+b\right)\) moles of \(B\), \(n_C\) moles of \(C\), and \(n_D\) moles of D. The final mixture contains \(n_A\) moles of \(A\), \(n_B\) moles of \(B\), \(\left(n_C+c\right)\) moles of \(C\), and \(\left(n_D+d\right)\) moles of \(D\). \({\Delta }_rG\) is the Gibbs free energy change for this conversion in the limit as \(n_A\), \(n_B\), \(n_C\), and \(n_D\) become arbitrarily large. In this limit, we have \(n_A\gg a\), \(n_B\gg b\), \(n_C\gg c\), , and \(n_D\gg d\). Since the partial pressures remain (essentially) constant, the \(n_i\) must also satisfy
\[P_A={n_A}/{\left(n_A+n_B+n_C+n_D\right)} \nonumber \] \[P_B={n_B}/{\left(n_A+n_B+n_C+n_D\right)} \nonumber \] \[P_C={n_C}/{\left(n_A+n_B+n_C+n_D\right)} \nonumber \] \[P_D={n_D}/{\left(n_A+n_B+n_C+n_D\right)} \nonumber \]
When the ideal gases are separated from one another, the Gibbs free energy difference is the Gibbs free energy of \(c\) moles of gas \(C\) (at pressure \(P_C\)) plus the Gibbs free energy of \(d\) moles of gas \(D\) (at pressure \(P_D\)) minus the Gibbs free energy of \(a\) moles of gas \(A\) (at pressure \(P_A\)) and minus the Gibbs free energy of \(b\) moles of gas \(B\) (at pressure \(P_B\)). In §2, we call the Gibbs free energy change under these conditions \({\Delta }_{sep}G\).
In Section 13.2, we assert that \({\Delta }_{sep}G={\Delta }_rG\). Now we can see why this is so. The cycle shown in Figure 4 relates these two Gibbs free energy differences.
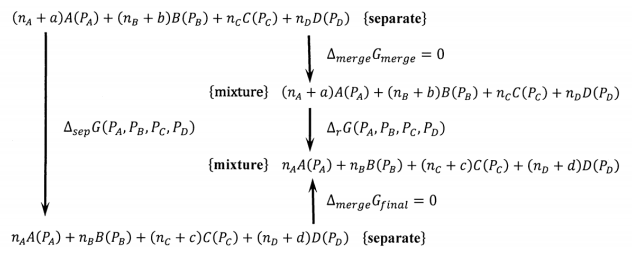
We have
\[{\Delta }_{sep}G\left(P_A,P_B,P_C,P_D\right)+{\Delta }_{merge}G_{final} \nonumber \] \[={\Delta }_{merge}G_{initial}+{\Delta }_rG\left(P_A,P_B,P_C,P_D\right) \nonumber \]
and since
\[{\Delta }_{merge}G_{final}={\Delta }_{merge}G_{initial} \nonumber \] we have
\[{\Delta }_{sep}G\left(P_A,P_B,P_C,P_D\right)={\Delta }_rG\left(P_A,P_B,P_C,P_D\right) \nonumber \]
Letting \({\overline{G}}_A\left(P_A\right)\) be the Gibbs free energy of one mole of pure ideal gas \(A\) at pressure \(P_A\), \({\overline{G}}_B\left(P_B\right)\) be the Gibbs free energy of one mole of pure ideal gas \(B\) at pressure \(P_B\), etc., we have
\[\begin{aligned} {\Delta }_{sep}G\left(P_A,P_B,P_C,P_D\right) & = n_A \overline{G}_A\left(P_A\right)+n_B\ \overline{G}_B\left(P_B\right) \\ ~ & +\left(n_C+c\right)\ \overline{G}_C\left(P_C\right)+\left(n_D+d\right)\overline{G}_D\left(P_D\right) \\ ~ & -\left(n_A+a\right) \overline{G}_A\left(P_A\right)-\left(n_B+b\right) \overline{G}_B\left(P_B\right) \\ ~ & -n_C\ \overline{G}_C\left(P_C\right)-n_D \overline{G}_D\left(P_D\right) \\ ~ & =c \overline{G}_C\left(P_C\right)+d \overline{G}_D\left(P_D\right)-a\ \overline{G}_A\left(P_A\right)-b\ \overline{G}_B\left(P_B\right) \end{aligned} \nonumber \]
Since \({\Delta }_{sep}G={\Delta }_rG\), we have
\[{\Delta }_rG=c\ \overline{G}_C\left(P_C\right)+d\ \overline{G}_D\left(P_D\right)-a\ \overline{G}_A\left(P_A\right)-b\ \overline{G}_B\left(P_B\right) \nonumber \]


