11.13: Absolute Zero is Unattainable
- Page ID
- 152360
The third law postulates that the entropy of a substance is always finite and that it approaches a constant as the temperature approaches zero. The value of this constant is independent of the values of any other state functions that characterize the substance. For any given substance, we are free to assign an arbitrarily selected value to the zero-temperature limiting value. However, we cannot assign arbitrary zero-temperature entropies to all substances. The set of assignments we make must be consistent with the experimentally observed zero-temperature limiting values of the entropy changes of reactions among different substances. For perfectly crystalline substances, these reaction entropies are all zero. We can satisfy this condition by assigning an arbitrary value to the zero-temperature molar entropy of each element and stipulating that the zero-temperature entropy of any compound is the sum of the zero-temperature entropies of its constituent elements. This calculation is greatly simplified if we let the zero-temperature entropy of every element be zero. This is the essential content of the third law.
The Lewis and Randall statement incorporates this selection of the zero-entropy reference state for entropies, specifying it as “a crystalline state” of each element at zero degrees. As a result, the entropy of any substance at zero degrees is greater than or equal to zero. That is, the Lewis and Randall statement includes a convention that fixes the zero-temperature limiting value of the entropy of any substance. In this respect, the Lewis and Randall statement makes an essentially arbitrary choice that is not an intrinsic property of nature. We see, however, that it is an overwhelmingly convenient choice.
We have discussed alternative statements of the first and second laws. A number of alternative statements of the third law are also possible. We consider the following:
It is impossible to achieve a temperature of absolute zero.
This statement is more general than the Lewis and Randall statement. If we consider the application of this statement to the temperatures attainable in processes involving a single substance, we can show that it implies, and is implied by, the Lewis and Randall statement.
The properties of the heat capacity, \(C_P\), play a central role in these arguments. We have seen that \(C_P\) is a function of temperature. While it is not useful to do so, we can apply the defining relationship for \(C_P\) to a substance undergoing a phase transition and find \(C_P=\infty\). If we think about a substance whose heat capacity is less than zero, we encounter a contradiction of our basic ideas about heat and temperature: If \(q>0\) and \({q}/{\Delta T}<0\), we must have \(\Delta T<0\); that is, heating the substance causes its temperature to decrease. In short, the theory we have developed embeds premises that require \(C_P>0\) for any system on which we can make measurements.
Let us characterize a pure-substance system by its pressure and temperature and consider reversible constant-pressure processes in which only pressure–volume work is possible. Then \({\left({\partial S}/{\partial T}\right)}_P={C_P}/{T}\) and \(dS={C_PdT}/{T}\). We now want to show: the Lewis and Randall stipulation that the entropy is always finite requires that the heat capacity go to zero when the temperature goes to zero. (Since we are going to show that the third law prohibits measurements at absolute zero, this conclusion is consistent with our conclusion in the previous paragraph.) That the heat capacity goes to zero when the temperature goes to zero is evident from \(S={C_PdT}/{T}.\) If \(C_P\) does not go to zero when the temperature goes to zero, \(dS\) becomes arbitrarily large as the temperature goes to zero, which contradicts the Lewis and Randall statement.
To develop this result more explicitly, we let the heat capacities at temperatures \(T\) and zero be \(C_P\left(T\right)\) and \(C_P\left(0\right)\), respectively. Since \(C_P\left(T\right)>0\) for any \(T\ >\ 0\), we have \(S\left(T\right)-S\left(T^*\right)>0\) for any \(T>T^*>0\). Since the entropy is always finite, \(\infty >S\left(T\right)-S\left(T^*\right)>0\), so that
\[\infty >{\mathop{\mathrm{lim}}_{T^*\to 0} \left[S\left(T\right)-S\left(T^*\right)\right]\ }>0 \nonumber \]
and
\[\infty >{\mathop{\mathrm{lim}}_{T^*\to 0} \int^T_{T^*}{\frac{C_P}{T}}\ }dT>0 \nonumber \]
For temperatures in the neighborhood of zero, we can expand the heat capacity, to arbitrary accuracy, as a Taylor series polynomial in \(T\):
\[C_P\left(T\right)=C_P\left(0\right)+\left(\frac{\partial C_P\left(0\right)}{\partial T}\right)_PT +\frac{1}{2} \left(\frac{{\partial }^2C_P\left(0\right)}{\partial T^2}\right)_PT^2+\dots \nonumber \]
The inequalities become
\[\infty >{\mathop{\mathrm{lim}}_{T^*\to 0} \left\{C_P\left(0\right){ \ln \frac{T}{T^*}\ }+{\left(\frac{\partial C_P\left(0\right)}{\partial T}\right)}_P\left(T-T^*\right)+\frac{1}{4}{\left(\frac{{\partial }^2C_P\left(0\right)}{\partial T^2}\right)}_P{\left(T-T^*\right)}^2+\dots \right\}\ }>0 \nonumber \]
The condition on the left requires \(C_P\left(0\right)=0\).
We could view the third law as a statement about the heat capacities of pure substances. We infer not only that \(C_P>0\) for all \(T>0\), but also that
\[{\mathop{\mathrm{lim}}_{T\to 0} \left(\frac{C_P}{T}\right)=0\ } \nonumber \]
More generally, we can infer corresponding assertions for closed reversible systems that are not pure substances: \({\left({\partial H}/{\partial T}\right)}_P>0\) for all \(T>0\), and \({\mathop{\mathrm{lim}}_{T\to 0} T^{-1}{\left({\partial H}/{\partial T}\right)}_P=0\ }\). (The zero-temperature entropies of such systems are not zero, however.) In the discussion below, we describe the system as a pure substance. We can make essentially the same arguments for any system; we need only replace \(C_P\) by \({\left({\partial H}/{\partial T}\right)}_P\). The Lewis and Randall statement asserts that the entropy goes to a constant at absolute zero, irrespective of the values of any other thermodynamic functions. It follows that the entropy at zero degrees is independent of the value of the pressure. For any two pressures, \(P_1\) and \(P_2\), we have \(S\left(P_2,0\right)-S\left(P_1,0\right)=0\). Letting \({P=P}_1\) and \(P_2=P+\Delta P\) and, we have
\[\frac{S\left(P+\Delta P,0\right)-S\left(P,0\right)}{\Delta P}=0 \nonumber \]
for any \(\Delta P\). Hence, we have
\[{\left(\frac{\partial S}{\partial P}\right)}_{T=0}=0 \nonumber \]
In Chapter 10, we find \({\left({\partial S}/{\partial }P\right)}_T=-{\left({\partial V}/{\partial T}\right)}_P\), so both the entropy and the volume approach their zero-temperature values asymptotically.
When we say that absolute zero is unattainable, we mean that no system can undergo any change in which its final temperature is zero. To see why absolute zero must be unattainable, let us consider processes that can decrease the temperature of a system. In general, we have heat reservoirs available at various temperatures. We can select the available reservoir whose temperature is lowest, and bring the system to this temperature by simple thermal contact. This much is trivial; clearly, the challenge is to decrease the temperature further. To do so, we must effect some other change. Whatever this change may be, it cannot be aided by an exchange of heat with the surroundings. Once we have brought the system to the temperature of the coldest available portion of the surroundings, any further exchange of heat with the surroundings can only be counter-productive. We conclude that any process suited to our purpose must be adiabatic. Since an adiabatic process exchanges no heat with the surroundings, \(\Delta \hat{S}=0\).
The process must also be a possible process, so that \(\Delta S+\Delta \hat{S}\ge 0\), and since it is adiabatic, \(\Delta S\ge 0\). Let us consider a reversible process and an irreversible process in which the same system\({}^{2}\) goes from the state specified by \(P_1\) and \(T_1\) to a second state in which the pressure is \(P_2\). The final temperatures and the entropy changes of these processes are different. For the reversible process, \(\Delta S=0\); we designate the final temperature as \(T_2\). For the irreversible process, \(\Delta S>0\); we designate the final temperature as \(T^*_2\). As it turns out, the temperature change is less for the irreversible process than for the reversible process; that is, \(T_2-T_1<t^*_2-t_1\)>. Equivalently, the reversible process reaches a lower temperature: \({T_2<t}^*_2\)>. From
\[dS=\frac{C_P}{T}dT-{\left(\frac{\partial V}{\partial T}\right)}_PdP \nonumber \]
we can calculate the entropy changes for these processes. For the reversible process, we calculate \[\Delta S^{rev}=S\left(P_2,T_2\right)-S\left(P_1,T_1\right) \nonumber \]
To do so, we first calculate
\[{\left(\Delta S\right)}_T=S\left(P_2,T_1\right)-S\left(P_1,T_1\right) \nonumber \]
for the isothermal reversible transformation from state \(P_1\), \(T_1\) to the state specified by \(P_2\) and \(T_1\). For this step, \(dT\) is zero, and so
\[{\left(\Delta S\right)}_T=\int^{P_2}_{P_1}{{\left(\frac{\partial V}{\partial T}\right)}_PdP} \nonumber \]
We then calculate
\[{\left(\Delta S\right)}_P=S\left(P_2,T_2\right)-S\left(P_2,T_1\right) \nonumber \]
for the isobaric reversible transformation from state \(P_2\), \(T_1\) to state \(P_2\), \(T_2\). For this transformation, \(dP\) is zero, and
\[{\left(\Delta S\right)}_P=-\int^{T_2}_{T_1}{\frac{C_P}{T}dT} \nonumber \]
Then,
\[\Delta S^{rev}=S\left(P_2,T_2\right)-S\left(P_1,T_1\right)=\int^{T_2}_{T_1}{\frac{C_P}{T}dT}-\int^{P_2}_{P_1}{{\left(\frac{\partial V}{\partial T}\right)}_PdP}=0 \nonumber \]
Because \(\Delta S^{rev}=0\), the reversible process is unique; that is, given \(P_1\), \(T_1\), and \(P_2\), the final temperature of the system is determined. We find \(T_2\) from
\[\int^{T_2}_{T_1}{\frac{C_P}{T}dT}=\int^{P_2}_{P_1}{{\left(\frac{\partial V}{\partial T}\right)}_PdP} \nonumber \]
To understand the entropy change for the irreversible process, we note first that there are an infinite number of such processes. There is nothing unique about the final temperature. Given \(P_1\), \(T_1\), and \(P_2\), the final temperature, \(T^*_2\), can have any value consistent with the properties of the substance. To specify a particular irreversible process, we must specify all four of the quantities \(P_1\), \(T_1\), \(P_2\), and \(T^*_2\). Having done so, however, we can calculate the entropy change for the irreversible process,
\[\Delta S^{irrev}=S\left(P_2,T^*_2\right)-S\left(P_1,T_1\right)>0 \nonumber \]
by computing the entropy changes as we reversibly carry the system along the isothermal two-step path from \(P_1\), \(T_1\) to \(P_2\), \(T_1\) and then along the isobaric path from \(P_2\), \(T_1\) to \(P_2\), \(T^*_2\). The calculation of \(\Delta S^{irrev}\) for this reversible path from \(P_1\), \(T_1\) to \(P_2\), \(T^*_2\) employs the same logic as the calculation, in the previous paragraph, of \(\Delta S\) for the reversible path from \(P_1\), \(T_1\) to \(P_2\), \(T_2\). The difference is that \(T^*_2\) replaces \(T_2\) as the upper limit in the temperature integral. The pressure integral is the same. We have
\[\Delta S^{irrev}=S\left(P_2,T^*_2\right)-S\left(P_1,T_1\right)=\int^{T^*_2}_{T_1}{\frac{C_P}{T}dT}-\int^{P_2}_{P_1}{{\left(\frac{\partial V}{\partial T}\right)}_PdP} >0 \nonumber \]
From \(\Delta S^{irrev}>\Delta S^{rev}\), we have
\[\int^{T^*_2}_{T_1}{\frac{C_P}{T}dT}>\int^{T_2}_{T_1}{\frac{C_P}{T}dT} \nonumber \]
Since the integrands are the same and positive, it follows that \(T^*_2>T_2\), as asserted above.
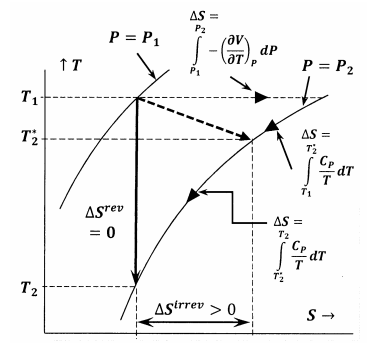
Figure 6 shows the relationships among the various quantities discussed in this argument. In the first instance, Figure 6 shows a plot of two of the system’s isobars in temperature—entropy space. That is, the line labeled \(P=P_1\) depicts the set of temperature—entropy points at which the equilibrated system has pressure \(P_1\); the line labeled \(P=P_2\), depicts the equilibrium positions at pressure \(P_2\). Other lines in this sketch represent paths along which the system can undergo reversible changes at constant entropy or constant temperature. The dotted line represents the irreversible process in which the system goes from the state specified by \(P_1\), \(T_1\) to the state specified by \(P_2\), \(T^*_2\). This line is dotted to represent the fact that the system’s temperature may not be well defined during the irreversible process.
Effective cooling can be achieved using pressure changes if the system is a gas. However, for liquids and solids, \({\left({\partial V}/{\partial T}\right)}_P\) is small; consequently, the temperature change for a reversible pressure change is also small. At temperatures near absolute zero, nearly all substances are solid; to achieve effective cooling we must change a thermodynamic variable for which a solid’s temperature coefficient is as large as possible. To consider the general problem of decreasing the temperature of a system by varying something other than pressure, we must consider a system in which some form of non-pressure–volume work is possible. Such a system is subject to an additional force, and its energy changes as this force changes.
Adiabatic Demagnetization
The practical method by which extremely low temperatures are achieved is called adiabatic demagnetization. This method exploits the properties of paramagnetic solids. In such solids, unpaired electrons localized on individual atoms give rise to a magnetic moment. Quantum mechanics leads to important conclusions about the interaction between such magnetic moments and an applied magnetic field: In an applied magnetic field, the magnetic moment of an individual atom is quantized. In the simplest case, it can be aligned in only two directions; it must be either parallel or anti-parallel to the applied magnetic field. When an atom’s magnetic moment is parallel to the magnetic field the energy of the system is less than when the alignment is anti-parallel. The applied magnetic field exerts a force on the magnetic moments associated with individual atoms. The energy of the system depends on the magnitude of the applied magnetic field.
Rather than focus on the particular case of adiabatic demagnetization, let us consider the energy and entropy changes associated with changes in a generalized potential, \({\mathit{\Phi}}_{\theta }\), and its generalized displacement, \(\theta\). (For adiabatic demagnetization, \(\theta\) would be the applied magnetic field.) Three variables are required to describe reversible changes in this system. We can express the energy and entropy as functions of temperature, pressure, and \(\theta\):
\(E=E\left(T,P,\theta \right)\) and \(S=S\left(T,P,\theta \right)\). The total differential of the entropy includes a term that specifies the dependence of entropy on \(\theta\). We have
\[dS={\left(\frac{\partial S}{\partial T}\right)}_{P,\theta }dT+{\left(\frac{\partial S}{\partial P}\right)}_{T,\theta }dP+{\left(\frac{\partial S}{\partial \theta }\right)}_{T,P}d\theta =\frac{C\left(T,P,\theta \right)}{T}dT-{\left(\frac{\partial V}{\partial T}\right)}_{P,\theta }dP+{\left(\frac{\partial S}{\partial \theta }\right)}_{T,P}d\theta \nonumber \]
where we write \(C\left(T,P,\theta \right)\) to emphasize that our present purposes now require that we measure the heat capacity at constant pressure and constant \(\theta\).
For constant pressure, P, and constant displacement, \(\theta\), the entropy depends on temperature as
\[S\left(T,P,\theta \right)=S\left(0,P,\theta \right)+\int^T_0 \left(\frac{\partial S}{\partial T}\right)_{P,\theta }dT=S\left(0,P,\theta \right)+\int^T_0 \frac{C\left(T,P,\theta \right)}{T}dT \nonumber \]
The postulate that the entropy be finite at any temperature implies that the pressure- and \(\theta\)-dependent heat capacity becomes zero at absolute zero. That is, at absolute zero, the heat capacity vanishes whatever the values of P and \(\theta\). The argument is exactly the same as before. Earlier, we wrote \(C_P\left(0\right)=0\); for the present generalized case, we write \(C\left(0,P,\theta \right)=0\).
Similarly, from the postulate that the entropy goes to a constant at absolute zero for all values of the other thermodynamics variables, it follows that, for any two pressures \(P_1\) and \(P_2\), and for any two values of the generalized displacement, \({\theta }_1\) and \({\theta }_2\),
\[S\left(0,P_1,{\theta }_1\right)=S\left(0,P_2,{\theta }_1\right)=S\left(0,P_1,{\theta }_2\right)=S\left(0,P_2,{\theta }_2\right)=0 \nonumber \]
and hence that
\[{\left(\frac{\partial S}{\partial P}\right)}_{T=0,\theta }={\left(\frac{\partial S\left(0,P,\theta \right)}{\partial P}\right)}_{T,\theta }=0 \nonumber \] and \[{\left(\frac{\partial S}{\partial \theta }\right)}_{T=0,P}={\left(\frac{\partial S\left(0,P,\theta \right)}{\partial \theta }\right)}_{T,P}=0 \nonumber \]
We want to consider a process in which a system goes from the lowest temperature available in the surroundings to a still lower temperature. To minimize the final temperature, this process must be carried out adiabatically. It must also be a possible process, so that \(dS\ge 0\). For simplicity, let us now assume that we carry out this process at a constant pressure, \(P\), and that the system goes from the state specified by \(P\), \(T_1\), \({\theta }_1\) to the state specified by \(P\), \(T_2\), \({\theta }_2\) where \(T_1>T_2\). The entropies of these two states are
\[S\left(T_1,P,{\theta }_1\right)=S\left(0,P,{\theta }_1\right)+\int^{T_1}_0{\frac{C\left(T,P,{\theta }_1\right)}{T}}dT \nonumber \] and \[S\left(T_2,P,{\theta }_2\right)=S\left(0,P,{\theta }_2\right)+\int^{T_2}_0{\frac{C\left(T,P,{\theta }_2\right)}{T}}dT \nonumber \]
The entropy change for this process is
\[S\left(T_2,P,{\theta }_2\right)-S\left(T_1,P,{\theta }_1\right)=S\left(0,P,{\theta }_2\right)-S\left(0,P,{\theta }_1\right) \nonumber \] \[+\int^{T_2}_0{\frac{C\left(T,P,{\theta }_2\right)}{T}}dT-\int^{T_1}_0{\frac{C\left(T,P,{\theta }_1\right)}{T}}dT\ge 0 \nonumber \]
Now, let us suppose that the final temperature is zero; that is, \(T_2=0\), so that
\[\int^{T_2}_0{\frac{C\left(T,P,{\theta }_2\right)}{T}}dT=0 \nonumber \] It follows that \[S\left(0,P,{\theta }_2\right)-S\left(0,P,{\theta }_1\right)\ge \int^{T_1}_0{\frac{C\left(T,P,{\theta }_1\right)}{T}}dT>0 \nonumber \]
where the inequality on the right follows from the fact that that \(C\left(T,P,{\theta }_1\right)>0\). Then, it follows that
\[S\left(0,P,{\theta }_2\right)-S\left(0,P,{\theta }_1\right)>0 \nonumber \]
which contradicts the Lewis and Randall statement of the third law. The assumption that the system can reach absolute zero leads to a contradiction of the Lewis and Randall statement of the third law. Therefore, if the Lewis and Randall statement is true, absolute zero is unattainable.
The converse applies also; that is, from the proposition that absolute zero is unattainable, we can show that the Lewis and Randall statement is true. To do so, we rearrange the above equation for \(\Delta S\),
\[\int^{T_2}_0{\frac{C\left(T,P,{\theta }_2\right)}{T}}dT\ge \nonumber \] \[\int^{T_1}_0{\frac{C\left(T,P,{\theta }_1\right)}{T}}dT-S\left(0,P,{\theta }_2\right)+S\left(0,P,{\theta }_1\right) \nonumber \]
If we now assume that the Lewis and Randall statement is false, the expression on the right can be less than or equal to zero. The integral on the left can then be zero, in which case the system can reach absolute zero. If the Lewis and Randall statement is false, it is true that the system can reach absolute zero. Therefore: If the system cannot reach absolute zero, the Lewis and Randall statement is true.
Figures 7 and 8 depict these ideas using contour plots in temperature–entropy space. Each figure shows two contour lines. One of these contour lines is a set of temperature and entropy values along which the pressure is constant at \(P\) and \(\theta\) is constant at \({\theta }_1\). The other contour line is a set of temperature and entropy values along which the pressure is constant at \(P\) and \(\theta\) is constant at \({\theta }_2\). The slope of a contour line is
\[{\left(\frac{\partial T}{\partial S}\right)}_{P,\theta }=\frac{T}{C\left(T,P,\theta \right)} \nonumber \]
Because the heat capacity is always positive, this slope is always positive.
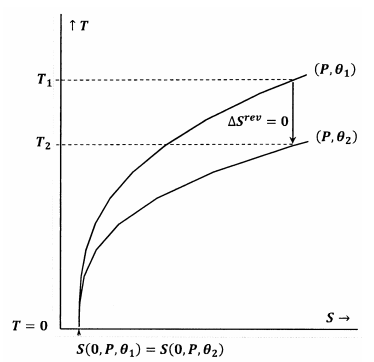
In Figure 7, the Lewis and Randall statement is satisfied. When the temperature goes to zero, the contour lines meet at the same value of the entropy; these contours satisfy the relationship
\[S\left(0,P,{\theta }_1\right)=S\left(0,P,{\theta }_2\right) \nonumber \]
An adiabatic (vertical) path from the contour for \(P\) and \({\theta }_1\) meets the contour for \(P\) and \({\theta }_2\) at a positive temperature, \(T_2>0\). Since this is evidently true for any \(P\) and any \({\theta }_2\), the final state for any adiabatic process will have \(T_2>0\). Because the Lewis and Randall statement is satisfied, the system cannot reach absolute zero, and vice versa.
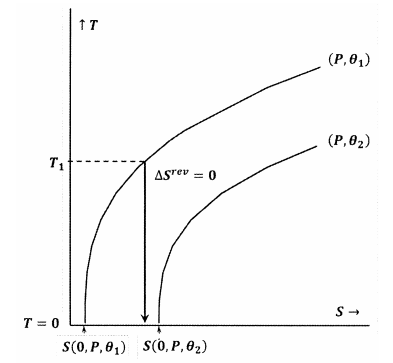
In Figure 8, the Lewis and Randall statement is violated, because we have \(S\left(0,P,{\theta }_1\right)\). In this case, an adiabatic process initiated from a low enough initial temperature, \(T_1\), will reach absolute zero without intersecting the contour for constant \(P\) and \({\theta }_2\). Because the Lewis and Randal statement is violated, the system can reach absolute zero, and vice versa.