Quantum Mechanical H Atom
- Page ID
- 1708
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)A hydrogen atom consists of a single proton orbited by a single electron. When compared to the electron, the proton has such a large mass that it may be considered stationary while the electron circles around it.
Introduction
It is known that this model is acceptable when the reduced mass of the system is used. Reduced mass is defined below for two masses 1 and 2.
\[ \mu = \dfrac{m_1m_2}{m_1+m_2}\]
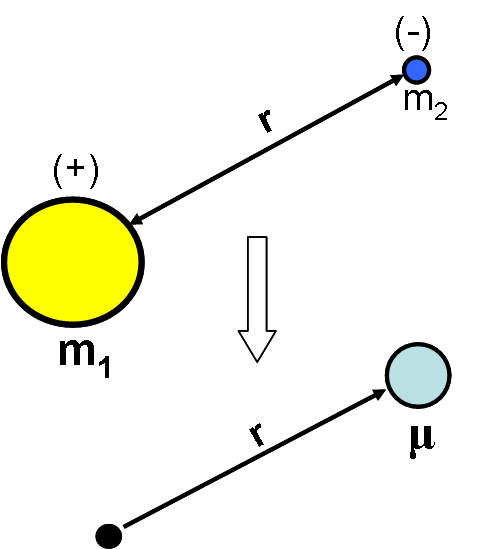
Using the reduced mass effectively converts the two-body problem (two moving and interacting bodies in space) into a one-body problem (a single electron moving about a fixed point). The basic Schrödinger equation is
\[\hat{H}\Psi=E\Psi\]
where \(\hat{H}\) is the Hamiltonian operator, E is the energy of the particle and \(\Psi\) is the particle's wavefunction that describes its spatial probability. The Hamiltonian operator is the sum of the kinetic energy operator and potential energy operator. The kinetic energy operator is the same for all models but the potential energy changes and is the defining parameter.
Hydrogen Atom Schrödinger Equation
The hydrogen atom's electron wavefunctions can be described using a variation of the rigid rotor-harmonic oscillator (RRHO) model. Rather than following a parabolic potential energy surface, the electron associated with the hydrogen experiences an exponential coulombic interaction.
This RRHO Hamiltonian combines the kinetic energy elements of both previous models as well as an associated potential energy (as that in the harmonic oscillator scenario). The Schrödinger Equation for the RRHO model involves the Hamiltonian operator acting on a wavefunction that similarly reflects both the rigid rotor and harmonic oscillator models.
In order for the Hamiltonian to fit the model, all component Hamiltonian elements need only be added together.
\[\hat{H}_{RRHO}=\hat{H}_{RR}(\theta{,}\phi{})+\hat{H}_{HO}(r)\]
\[\hat{H}_{RRHO}=\frac{1}{2mr_0^2}\hat{L}^2(\theta{,}\phi{})+\frac{-\hbar{^2}}{2\mu{}}\frac{d^2}{dr^2}+V(r)\]
As shown below, the solution wavefunction will be a multiplicative combination of the two model solutions.
\[\psi{_{RRHO}}=\psi{}_{RR}*\psi{}_{OH}\]
\((\hat{H}_{RR}+\hat{H}_{HO})(\psi{_{RR}}*\psi{_{HO}})=\psi{_{HO}}\hat{H}_{RR}\psi{_{RR}}+\psi{_{RR}}\hat{H}_{HO}\psi{_{HO}}\)
\((\hat{H}_{RR}+\hat{H}_{HO})(\psi{_{RR}}*\psi{_{HO}})=\psi{_{HO}}*E_{RR}\psi{_{RR}}+\psi{_{RR}}*E_{HO}\psi{_{HO}}\)
\[(] (\hat{H}_{RR}+\hat{H}_{HO})(\psi_{RR}*\psi_{HO})=E_{RR}(\psi{_{RR}}*\psi{_{HO}})+E_{HO}(\psi_{RR}*\psi_{HO}) \]
\(\hat{H}_{RRHO}\psi{_{RRHO}}=E_{RR}\psi{_{RRHO}}+E_{HO}\psi{_{RRHO}}") }}
Thus, \( E_{RRHO}=E_{RR}+E_{HO}\)
After further refinement the Hamiltonian operator for the hydrogen atom is found to be
\[\hat{H}=-\dfrac{\hbar{^2}}{2m_e}\bigtriangledown{^2}-\dfrac{e^2}{4\pi{}\epsilon{r}}\]
where the Laplacian operator is defined as
\[ \bigtriangledown{^2}=\dfrac{\partial{^2}}{\partial{x^2}}+\dfrac{\partial{^2}}{\partial{y^2}}+\dfrac{\partial{^2}}{\partial{z^2}}\]
To solve the Schrödinger Equation for the hydrogen atom, it is simplest to perform the quantum mechanical calculations using spherical coordinates (based on the three variables r, \(\theta\) and \(\phi\)). After appropriate adjustments are made to compensate for the change of variables, the Schrödinger equation becomes:
\[-\hbar{^2}\dfrac{\partial{}}{\partial{r}}\left(r^2\dfrac{\partial{}\psi{}}{\partial{r}}\right)+\hat{L}^2\psi{}+2m_er^2[V(r)-E]\psi{(}r,\theta{,}\phi{)}=0\]
where \( V(r)=\dfrac{e^2}{4\pi{}\epsilon{_0}r}\) is the Coulombic (electrostatic) potential between the nucleus and electron and \(\hat{L}^2\) is the angular momentum operator also found from the quantum mechanical rigid rotor model.
Additionally, the assumption must be made that the wavefunction is of a form such that it can be arranged as the product of two functions using different variables (separation of variables). It is found that the wavefunction can be described as
\[ \psi{(}r,\theta{,}\phi{)}=R_{nl}(r)Y_l^m(\theta{,}\phi{)}\]
where \(R_{nl}(r)\) is the radial function and is defined as \(R_{nl}(r)=N_{nl}e^{r/na_0}r^lL_{n+l}^{2l+1}\left(\frac{2r}{na_0}\right)\)
Y is a spherical harmonic function, identical to the set of solutions to the rigid rotor quantum mechanical model. The function \( L_{n+l}^{2l+1}\left(\frac{2r}{na_0}\right) \) is an associated Laguerre polynomial, determined by the two quantum numbers \(l")}} and n. However, simply keeping the notation of R(r) will suffice for the following mathematics.
If this separated wavefunction is used in the Schrödinger Equation and the substitution \(\hat{L}^2Y_l^{m_l}(\theta{,}\phi{)}=\hbar{^2}l(l+1)Y_l^{m_l}(\theta{,}\phi{)})\) is carried out, it is found that all of the angular elements of the equation cancel out to yield an equation containing solely radial functions.
\(-\hbar{^2}\dfrac{\partial{}}{\partial{r}}\left(r^2\dfrac{\partial{}}{\partial{r}}\left(R(r)Y_l^{m_l}(\theta{,}\phi{})\right)\right)+R(r)\hbar{^2}l(l+1)Y_l^{m_l}(\theta{},\phi{})+2m_er^2[V(r)-E]R(r)Y_l^{m_l}(\theta{},\phi{})\)
\(-\hbar{^2}\dfrac{d}{dr}\left(r^2\dfrac{\partial{R}}{\partial{r}}\right)Y_l^{m_l}(\theta{},\phi{})+\hbar{^2}l(l+1)R(r)Y_l^{m_l}(\theta{},\phi{})+2m_er^2[V(r)-E]R(r)Y_l^{m_l}(\theta{},\phi{})=0\)
\[ -\hbar{^2}\dfrac{d}{dr}\left(r^2\dfrac{\partial{R}}{\partial{r}}\right)+\hbar{^2}l(l+1)R(r)+2m_er^2[V(r)-E]R(r)=0\]
\[ \dfrac{-\hbar{^2}}{2m_er^2}\dfrac{d}{dr}\left(r^2\dfrac{dR}{dr}\right)+\left[\dfrac{\hbar{^2}l(l+1)}{2m_er^2}+V(r)-E\right]R(r)=0\]
To solve this Schrödinger equation the power series method, the same method used to solve the harmonic oscillator, has to be used.
References
- McQuarrie, Donald A. Quantum Chemistry. 2nd ed. United States Of America: University Science Books, 2008. 321-24.
Outside Links
- Link to outside sources. Wikipedia entries should probably be referenced here.
Problems
1. Show that the energy is dependent only on the radial portion of the wavefunction.

