Van Der Waals Interactions
- Page ID
- 1664
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Van der Waals forces are driven by induced electrical interactions between two or more atoms or molecules that are very close to each other. Van der Waals interaction is the weakest of all intermolecular attractions between molecules. However, with a lot of Van der Waals forces interacting between two objects, the interaction can be very strong.
Introduction
Here is a chart to compare the relative weakness of Van der Waals forces to other intermolecular attractions.
| Force | Strength (kJ/mol) | Distance (nm) |
|---|---|---|
| Van der Waals | 0.4-4.0 | 0.3-0.6 |
| Hydrogen Bonds | 12-30 | 0.3 |
| Ionic Interactions | 20 | 0.25 |
| Hydrophobic Interactions | <40 | varies |
Causes of Van der Waals Forces
Quantum Mechanics strongly emphasizes the constant movement of electrons in an atom through the Schrödinger Equation and the Heisenberg’s Uncertainty Principle. The Heisenberg’s Uncertainty Principle proposes that the energy of the electron is never zero; therefore, it is constantly moving around its orbital. The square of the Schrödinger Equation for a particle in a box suggests that it is probable of finding the electron (particle) anywhere in the orbital of the atom (box).
These two important aspects of Quantum Mechanics strongly suggest that the electrons are constantly are moving in an atom, so dipoles are probable of occurring. A dipole is defined as molecules or atoms with equal and opposite electrical charges separated by a small distance.
It is probable to find the electrons in this state:
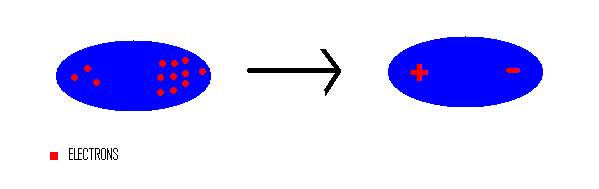
This is how spontaneous (or instantaneous) dipoles occur. When groups of electrons move to one end of the atom, it creates a dipole. These groups of electrons are constantly moving so they move from one end of the atom to the other and back again continuously. Therefore, the opposite state is as probable of occurring.
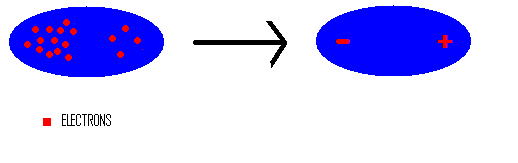
Opposite state due to fluctuation of dipoles:
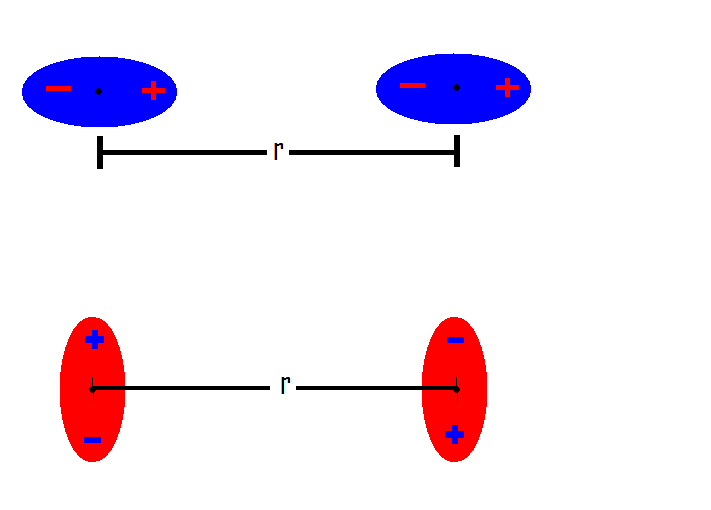
Dipole-Dipole Interaction
Dipole-Dipole interactions occur between molecules that have permanent dipoles; these molecules are also referred to as polar molecules. The figure below shows the electrostatic interaction between two dipoles.
The potential energy of the interaction for the top pair of the image above is represented by the equation:
\[ V = -\dfrac{2\mu_A\mu_B}{4\pi\epsilon_o r^3} \tag{1} \]
The potential energy of the interaction for the bottom pair is represented by the equation:
\[ V = -\dfrac{\mu_A\mu_B}{4\pi\epsilon_o r^3} \tag{2}\]
with
- \( V \) is the potential energy
- \( \mu \) is the dipole moment
- \( \epsilon_o \) is the vacuum permittivity
- \( r \) is the length between the two nuclei
The negative sign indicates that energy is released out of the system, because energy is released when bonds are formed, even weak bonds. The negative sign also suggests that the interaction is driven by an attractive force (a positive sign would indicate a repulsion force between the two molecules). If the conditions of these two samples are the same except for their orientation, the second pair of the electron will always have a larger potential energy, because both the negative and positive ends are involve in the interactions.
Induced Dipoles
An induced dipole moment is a temporary condition during which a neutral nonpolar atom (i.e. Helium) undergo a separation of charges due to the environment. When an instantaneous dipole atom approaches a neighboring atom, it can cause that atom to also produce dipoles. The neighboring atom is then considered to have an induced dipole moment.
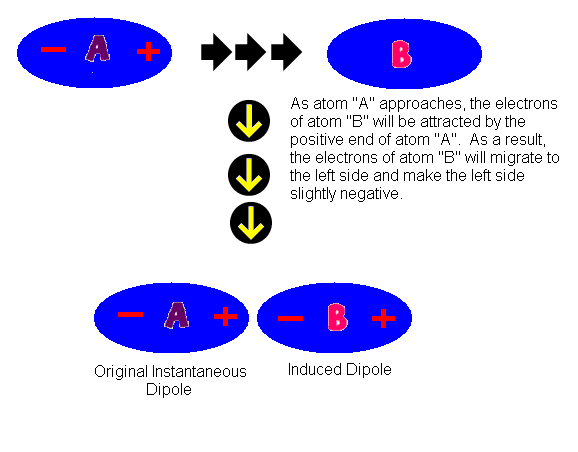
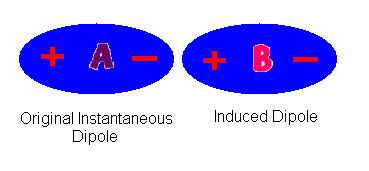
Even though these two atoms are interacting with each other, their dipoles may still fluctuate. However, they must fluctuate in synchrony in order to maintain their dipoles and stay interacted with each other. Result of synchronizing fluctuation of dipoles:
The potential energy representing the dipole-induced dipole interaction is:
\[ V = -\dfrac{\alpha\mu^2}{4\pi\epsilon_o r^6} \tag{4}\]
- \( \alpha \) = polarizability of the nonpolar molecule
Polarizability defines how easy the electron density of an atom or a molecule can be distorted by an external electric field.
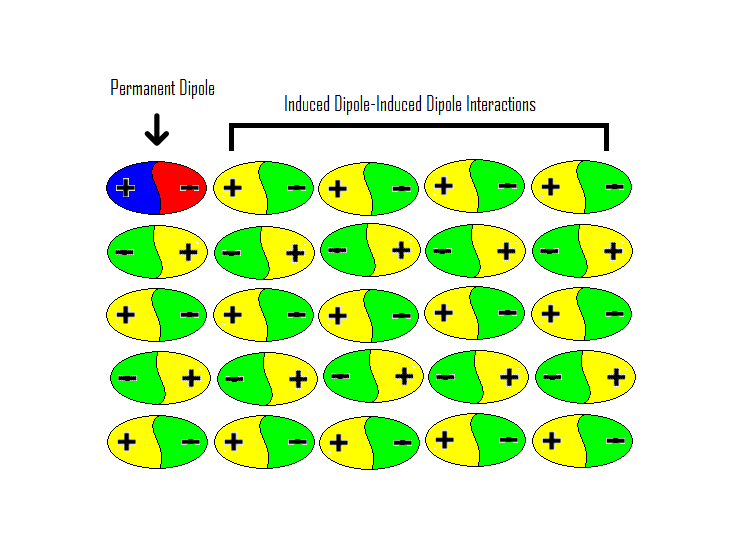
Spontaneous Dipole-Induced Dipole Interaction
Spontaneous dipole-induced dipole interactions are also known as dispersion or London forces (name after the German physicist Fritz London). They are large networks of intermolecular forces between nonpolar and non-charged molecules and atoms (i.e. alkanes, noble gases, and halogens). Molecules that have induced dipoles may also induce neighboring molecules to have dipole moments, so a large network of induced dipole-induced dipole interactions may exist. The image below illustrates a network of induced dipole-induced dipole interactions.
The potential energy of an induced dipole-induced dipole interaction is represented by this equation:
\[ V = -\dfrac{3}{2}\dfrac{I_aI_b}{I_a + I_b}\dfrac{\alpha_a\alpha_b}{r^6} \tag{5}\]
- \( I \) = The first ionization energy of the molecule
The radius is a huge determinant of how large the potential energy is since the potential energy is inversely proportional to \( r^6 \). A small increase in the radius, would greatly decrease the potential energy of the interaction.
References
- Atkins, Peter and Julio de Paula. Physical Chemistry for the Life Sciences. Oxford, UK: Oxford University Press. 2006. 458.
- Chang, Raymond. Physical Chemistry for the Biosciences. Sausalito, CA: Edwards Brothers, Inc. 2005. 492-498.
- Garrett, Reginald H. and Charles M. Grisham. Biochemistry. Belmont, CA: Thomas Brooks/Cole. 2005. 13.
- Petrucci, Ralph H. et. al. General Chemistry. Eight Edition. Upper Saddle River, NJ: Prentice-Hall, Inc. 2002. 497-500.
Contributors and Attributions
- Justin Than (UCD)