2.1.5: Spectrophotometry
- Page ID
- 1431
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Spectrophotometry is a method to measure how much a chemical substance absorbs light by measuring the intensity of light as a beam of light passes through sample solution. The basic principle is that each compound absorbs or transmits light over a certain range of wavelength. This measurement can also be used to measure the amount of a known chemical substance. Spectrophotometry is one of the most useful methods of quantitative analysis in various fields such as chemistry, physics, biochemistry, material and chemical engineering and clinical applications.
Introduction
Every chemical compound absorbs, transmits, or reflects light (electromagnetic radiation) over a certain range of wavelength. Spectrophotometry is a measurement of how much a chemical substance absorbs or transmits. Spectrophotometry is widely used for quantitative analysis in various areas (e.g., chemistry, physics, biology, biochemistry, material and chemical engineering, clinical applications, industrial applications, etc). Any application that deals with chemical substances or materials can use this technique. In biochemistry, for example, it is used to determine enzyme-catalyzed reactions. In clinical applications, it is used to examine blood or tissues for clinical diagnosis. There are also several variations of the spectrophotometry such as atomic absorption spectrophotometry and atomic emission spectrophotometry.
A spectrophotometer is an instrument that measures the amount of photons (the intensity of light) absorbed after it passes through sample solution. With the spectrophotometer, the amount of a known chemical substance (concentrations) can also be determined by measuring the intensity of light detected. Depending on the range of wavelength of light source, it can be classified into two different types:
- UV-visible spectrophotometer: uses light over the ultraviolet range (185 - 400 nm) and visible range (400 - 700 nm) of electromagnetic radiation spectrum.
- IR spectrophotometer: uses light over the infrared range (700 - 15000 nm) of electromagnetic radiation spectrum.
In visible spectrophotometry, the absorption or the transmission of a certain substance can be determined by the observed color. For instance, a solution sample that absorbs light over all visible ranges (i.e., transmits none of visible wavelengths) appears black in theory. On the other hand, if all visible wavelengths are transmitted (i.e., absorbs nothing), the solution sample appears white. If a solution sample absorbs red light (~700 nm), it appears green because green is the complementary color of red. Visible spectrophotometers, in practice, use a prism to narrow down a certain range of wavelength (to filter out other wavelengths) so that the particular beam of light is passed through a solution sample.
Devices and mechanism
Figure 1 illustrates the basic structure of spectrophotometers. It consists of a light source, a collimator, a monochromator, a wavelength selector, a cuvette for sample solution, a photoelectric detector, and a digital display or a meter. Detailed mechanism is described below. Figure 2 shows a sample spectrophotometer (Model: Spectronic 20D).
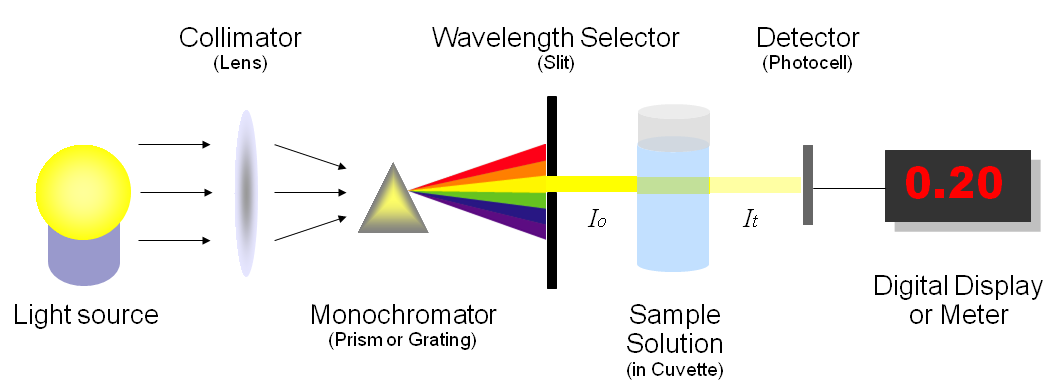
A spectrophotometer, in general, consists of two devices; a spectrometer and a photometer. A spectrometer is a device that produces, typically disperses and measures light. A photometer indicates the photoelectric detector that measures the intensity of light.
- Spectrometer: It produces a desired range of wavelength of light. First a collimator (lens) transmits a straight beam of light (photons) that passes through a monochromator (prism) to split it into several component wavelengths (spectrum). Then a wavelength selector (slit) transmits only the desired wavelengths, as shown in Figure 1.
- Photometer: After the desired range of wavelength of light passes through the solution of a sample in cuvette, the photometer detects the amount of photons that is absorbed and then sends a signal to a galvanometer or a digital display, as illustrated in Figure 1.

You need a spectrometer to produce a variety of wavelengths because different compounds absorb best at different wavelengths. For example, p-nitrophenol (acid form) has the maximum absorbance at approximately 320 nm and p-nitrophenolate (basic form) absorb best at 400nm, as shown in Figure 3.
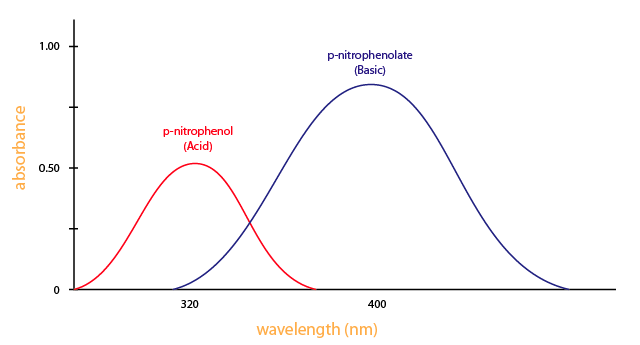
Looking at the graph that measures absorbance and wavelength, an isosbestic point can also be observed. An isosbestic point is the wavelength in which the absorbance of two or more species are the same. The appearance of an isosbestic point in a reaction demonstrates that an intermediate is NOT required to form a product from a reactant. Figure 4 shows an example of an isosbestic point.

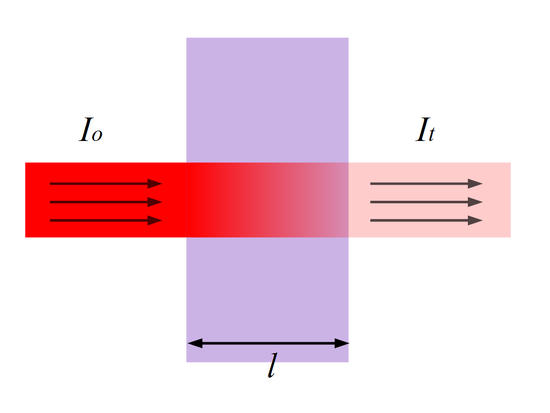
Referring back to Figure 1 (and Figure 5), the amount of photons that goes through the cuvette and into the detector is dependent on the length of the cuvette and the concentration of the sample. Once you know the intensity of light after it passes through the cuvette, you can relate it to transmittance (T). Transmittance is the fraction of light that passes through the sample. This can be calculated using the equation:
\(Transmittance (T) = \dfrac{I_t}{I_o}\)
Where It is the light intensity after the beam of light passes through the cuvette and Io is the light intensity before the beam of light passes through the cuvette. Transmittance is related to absorption by the expression:
\(Absorbance (A) = - log(T) = - log(\dfrac{I_t}{I_o})\)
Where absorbance stands for the amount of photons that is absorbed. With the amount of absorbance known from the above equation, you can determine the unknown concentration of the sample by using Beer-Lambert Law. Figure 5 illustrates transmittance of light through a sample. The length \(l\) is used for Beer-Lambert Law described below.
Beer-Lambert Law
Beer-Lambert Law (also known as Beer's Law) states that there is a linear relationship between the absorbance and the concentration of a sample. For this reason, Beer's Law can only be applied when there is a linear relationship. Beer's Law is written as:
\(A = \epsilon{lc}\)
where
- \(A\) is the measure of absorbance (no units),
- \(\epsilon\) is the molar extinction coefficient or molar absorptivity (or absorption coefficient),
- \(l\) is the path length, and
- \(c\) is the concentration.
The molar extinction coefficient is given as a constant and varies for each molecule. Since absorbance does not carry any units, the units for \(\epsilon\) must cancel out the units of length and concentration. As a result, \(\epsilon\) has the units: L·mol-1·cm-1. The path length is measured in centimeters. Because a standard spectrometer uses a cuvette that is 1 cm in width, \(l\) is always assumed to equal 1 cm. Since absorption, \(\epsilon\), and path length are known, we can calculate the concentration \(c\) of the sample.
Guanosine has a maximum absorbance of 275 nm. \(\epsilon_{275} = 8400 M^{-1} cm^{-1}\) and the path length is 1 cm. Using a spectrophotometer, you find the that \(A_{275}= 0.70\). What is the concentration of guanosine?
Solution
To solve this problem, you must use Beer's Law.
\[A = \epsilon lc \nonumber \]
0.70 = (8400 M-1 cm-1)(1 cm)(\(c\))
Next, divide both side by [(8400 M-1 cm-1)(1 cm)]
\(c\) = 8.33x10-5 mol/L
There is a substance in a solution (4 g/liter). The length of cuvette is 2 cm and only 50% of the certain light beam is transmitted. What is the absorption coefficient?
Solution
Using Beer-Lambert Law, we can compute the absorption coefficient. Thus,
\(- \log \left(\dfrac{I_t}{I_o} \right) = - \log(\dfrac{0.5}{1.0}) = A = {8} \epsilon\)
Then we obtain that
\(\epsilon\) = 0.0376
In example 2 above, how much is the beam of light is transmitted when 8 g/liter ?
Solution
Since we know \(\epsilon\), we can calculate the transmission using Beer-Lambert Law. Thus,
\(\log(1) - \log(I_t) = 0 - \log(I_t)\) = 0.0376 x 8 x 2 = 0.6016
\(\log(I_t)\) = -0.6016
Therefore, \(I_t\) = 0.2503 = 25%
In example 2 above, what is the molar absorption coefficient if the molecular weight is 100?
Solution
It can simply obtained by multiplying the absorption coefficient by the molecular weight. Thus,
\(\epsilon\) = 0.0376 x 100 = 3.76 L·mol-1·cm-1
The absorption coefficient of a glycogen-iodine complex is 0.20 at light of 450 nm. What is the concentration when the transmission is 40 % in a cuvette of 2 cm?
Solution
It can also be solved using Beer-Lambert Law. Therefore,
\[- \log(I_t) = - \log(0.4) = 0.20 \times c \times 2 \nonumber \]
Then \(c\) = 0.9948
References
- Atkins, Peter and Julio de Paula. Physical Chemistry for the Life Sciences. New York: Oxford University Press, 2006.
- Chang, Raymond. Physical Chemistry for the Biosciences. USA: University Science Books, 2005.
- Gore, Michael. Spectrophotometry & Spectrofluorimetry. New York: Oxford University Press, 2000.
- Price, Nicholas and Dwek, Raymond and Wormald, Mark. Principles and Problems in Physical Chemistry for Biochemists. R. G. Ratcliffe. New York: Oxford University Press, 1997.
- Irwin H. Segel, Biochemical Calculations (How to Solve Mathematical Problems in General Biochemistry), 2nd edition, John Wiley & Sons, 1975
- http://www.nist.gov/pml/div685/grp03/spectrophotometry.cfm
Contributors and Attributions
- Kevin Vo (UCD)

