10.25: Gaussian Trial Wave Function for the Helium Atom

Last updated
Save as PDF

Page ID: 136975

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Gaussian trial wave function:

\[ \Psi (r, \beta ) := ( \frac{2 \beta}{ \pi})^{ \frac{3}{4}} exp (- \beta r^2) \nonumber \]

Demonstrate the wave function is normalized.

\[ \int_{0}^{ \infty} \Psi (r, \beta )^2 4 \pi r^2 dr |_{simplify}^{assume,~ \beta > 0 } \rightarrow 1 \nonumber \]

The terms contributing to the total electronic energy of the helium atom are the kinetic energy of each electron, each electronʹs interaction with the nucleus, and the interaction of the electrons with each other.

Calculate kinetic energy:

\[ 2 \int_{0}^{ \infty} \Psi (r, \beta ) [ \frac{-1}{2r} \frac{d^2}{dr^2} (r \Psi (r, \beta ))] 4 \pi r^2 dr |_{simplify}^{assume,~ \beta >0} \rightarrow 3 \beta] \nonumber \]

Calculate electron-nucleus potential energy:

\[ 2 \int_{0}^{ \infty} \Psi (r, \beta ) \frac{-2}{r} \Psi (r, \beta ) 4 \pi r^2 dr |_{simplify}^{assume,~ \beta > 0} \rightarrow (-8) \frac{2 ^{ \frac{1}{2}}}{ \pi} ( \beta \pi )^{ \frac{1}{2}} \nonumber \]

Calculation of electron-electron potential energy:

a. Calculate the electric potential of one of the electrons in the presence of the other:

\[ \frac{1}{r} \int_{0}^{r} \Psi (x, \beta )^2 4 \pi x^2 dx + \int_{r}^{ \infty} \frac{ \Psi (x, \beta )^2 4 \pi x^2}{x} dx |_{simplify}^{assume,~ \beta > 0 } \rightarrow \frac{erf(r 2^{ \frac{1}{2}} \beta ^{ \frac{1}{2}})}{r} \nonumber \]

b. Calculate the electron-electron potential energy using result of part a:

\[ \int_{0}^{ \infty} \Psi (r, \beta )^2 ( \frac{erf(r 2^{ \frac{1}{2}} \beta ^{ \frac{1}{2}})}{r}) 4 \pi r^2 dr |_{simplify}^{assume,~ \beta > 0} /rightarrow \frac{2}{ \pi} ( \beta \pi )^{ \frac{1}{2}} \nonumber \]

Write the equation for the total electronic energy in terms of the variational parameter \( \beta\):

\[ E( \beta ) := 3 \beta + (-8) \frac{2^{ \frac{1}{2}}}{ \pi} ( \beta \pi )^{ \frac{1}{2}} + \frac{2}{ \pi} ( \beta \pi )^{ \frac{1}{2}} simplify \rightarrow \frac{-[ (-3) \beta \pi + (8) 2^{ \frac{1}{2}} ( \beta \pi )^{ \frac{1}{2} - 2 ( \beta \pi )^{ \frac{1}{2}}}]}{ \pi} \nonumber \]

Minimize the energy with respect to the variational parameter \( \beta\).

\[ \frac{d}{d \beta} E( \beta ) = 0~~~solve,~ \beta \rightarrow \frac{-1}{9} \frac{(-33) + 8 (2)^{ \frac{1}{2}}}{ \pi} = 0.767~~~E(.767) = -2.301 \nonumber \]

Compare the variational calculation to the Hartree-Fock limit: E_HF := -2.8617

\[ \frac{E_{HF}- E(.767)}{E_{HF}} = 19.594 \nonumber \]

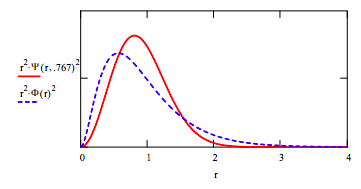
Compare optimized trial wave function with the Hartree-Fock wave function (see McQuarrie and Simon, page 283) by plotting the radial distribution functions.

\[ \Phi (r) := .75738exp( -1.430 r) + .43658exp(-2.4415r) + .17295exp(-4.0996r) - .02730exp(-6.4843r) + .06675exp(-7.978) \nonumber \]

Screen Shot 2019-02-14 at 12.29.37 PM.png