17.4: Heat Capacity at Constant Volume is the Change in Internal Energy with Temperature

The heat capacity at constant volume (\(C_V\)) is defined to be the change in internal energy with respect to temperature:

\[C_V = \left( \dfrac{\partial U}{\partial T} \right)_{N, V} \label{Eq3.26} \]

Since:

\[E = -\dfrac{\partial \ln{Q(N, V, \beta)}}{\partial \beta} \label{Eq3.27} \]

We see that:

\[\begin{align} C_V &= \dfrac{\partial U}{\partial T} \nonumber \\[4pt] &= \dfrac{\partial U}{\partial \beta} \dfrac{\partial \beta}{\partial T}  \nonumber \\[4pt] &= \dfrac{1}{kT^2} \dfrac{\partial^2}{\partial \beta^2} \: \ln Q(N, V, \beta) \nonumber\\[4pt] &= k \beta^2 \dfrac{\partial^2}{\partial \beta^2} \: \ln Q(N, V, \beta) \label{Eq3.28} \end{align} \]

where \(k\) is the Boltzmann constant. Energy can be stored in materials/gases via populating the specific degrees of freedom that exist in the sample. Understanding how this occurs requires the usage of Quantum Mechanics.

Dulong-Petit Law on the Heat Capacities of Solids

Pierre Louis Dulong and Alexis Thèrèse Petit conducted experiments in 1819 on three dimensional solid crystals to determine the heat capacities of a variety of these solids (Heat capacity is the solids ability to absorb and retain heat). Dulong and Petit discovered that all investigated solids had a heat capacity of approximately 2.49 x 10⁴ J kilomole^-1 K^-1 at around 298 K or room temperature. The result from their experiment was explained by considering every atom inside the solid as an oscillator with six degrees of freedom (an oscillator can be thought of as a spring connecting all the atoms in the solid lattice). These springs extend into three dimensional space. The more energy that is added to the solid the more these springs vibrate. Each atom has an energy of \(\frac{1}{2}kT\), where \(k\) is the Boltzmann constant and \(T\) is the absolute temperature. Thus,

\[ C_V=\dfrac{6R}{2}=3R \label{1}\tag{Dulong-Petit Law} \]

The number 6 in this equation is the number of degrees of freedom for the molecule. Petit and Dulong suggested that these results supported their foundation for the heat capacity of solids. The explanation for Petit and Dulong's experiment was not sufficient when it was discovered that heat capacity decreased as temperature approached absolute zero. The degrees of freedom do not slow down or cease to move when the solid reaches a sufficiently cold temperature. An additional model was proposed to explain this deviance. Two main theories were developed to explain this puzzling deviance in the heat capacity experiments. The first was constructed by Einstein and the second was authored by Debye.

Figure 17.4.1 : In a simple model of a solid element, each atom is attached to others by six springs, two for each possible motion: x, y, and z. Each of the three motions corresponds to two degrees of freedom, one for kinetic energy and one for potential energy. (CC BY-SA 4.0; OpenStax).

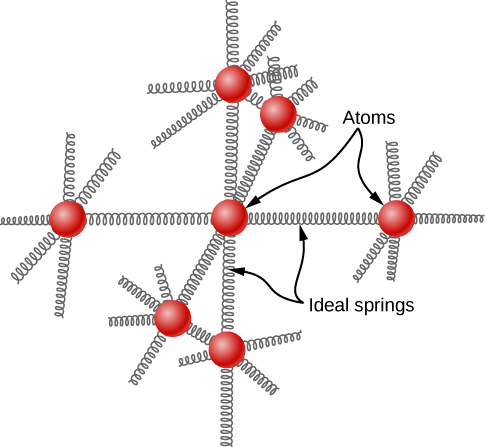
Einstein's Theory on the Heat Capacities of Solids

Einstein assumed three things when he investigated the heat capacity of solids. First, he assumed that each solid was composed of a lattice structure consisting of \(N\) atoms. Each atom was treated as moving independently in three dimensions within the lattice (3 degrees of freedom). This meant that the entire lattice's vibrational motion could be described by a total of \(3N\) motions, or degrees of freedom. Secondly, he assumed that the atoms inside the solid lattice did not interact with each other and thirdly, all of the atoms inside the solid vibrated at the same frequency. The third point highlights the main difference in Einstein's and Debye's two models.

Einstein's first point is accurate because the experimental data supported his hypothesis, however his second point is not because if atoms inside a solid could not interact, sound could not propagate through it. For example, a tuning fork's atoms, when struck, interact with one another to create sound which travels through air to the listener's ear. Atoms also interact in a solid when they are heated. Take for example a frying pan. If the pan is heated on one side, the heat transfers throughout the metal effectively warming the entire pan. Molecules that make up the frying pan interact to transfer heat. Much in the same way the oscillators in a solid interact when energy is added to the system. The extent of these interactions lead to the physically observed heat capacity.

The heat capacity of a solid at a constant volume is

\[ \begin{align*} C_V &= \left(\dfrac{\partial{U}}{\partial{T}}\right)_V \\[4pt] &=3Nk\left(\dfrac{\theta_E}{T}\right)^2 \label{2} \\[4pt] &= \dfrac{\exp \left(\dfrac{\theta_E}{T} \right)}{\left(\exp \dfrac{\theta_E}{T} -1\right)^2} \end{align*} \]

where

• \(\theta_E=\dfrac{hv}{k}\) is the Einstein temperature,
• \(h\) is Planck's constant,
• \(k\) is Boltzmann's constant, and
• \(\nu\) is the oscillator frequency of the atoms inside the solid.

The Einstein temperature's accessibility of the vibrational energy inside of a solid molecule determines the heat capacity of that solid. The greater the accessibility the greater the heat capacity. If the vibrational energy is easily accessible the collisions in the molecule have a greater probability of exciting the atom into an upper vibrational level. This is displayed below.

Figure 17.4.2 : This Figure illustrates the effect of the Einstein temperature on the likelihood of an oscillator absorbing energy from a collision and transferring that energy into stored heat or heat capacity. As the Einstein temperature increases, the greater the probability of an oscillator being excited to the next vibrational state. (CC BY-NC 4.0; Ümit Kaya via LibreTexts)

So the Einstein temperature specifically indicates the probability that a molecule has in its degrees of freedom to store energy in its atomic oscillators (or bonds). Comparing the Einstein temperature to the traditional classical values of Heat capacity will illustrate the differences the specific strengths (high temperature) and weaknesses (low temperature) of the Einstein model.

Figure 17.4.3 :This graph shows the accuracy of the of the Einstein temperature at low temperatures. The classical model is effective at temperatures above 10 Einstein Temperatures. (Cc BY-NC; Ümit Kaya via LibreTexts)

Thus, in the high temperature limit (\(\dfrac{\theta_E}{T}\ll 1\)) (i.e., the temperature is very large compared to the Einstein temperature) then

\[ C_V \approx 3Nk=3nR. \label{4} \]

Einstein's model reveals the accuracy of the Petit and Dulong model and models high temperatures accurately. However, just as Petit and Dulong's model decreased in accuracy as the temperature decreased, so followed Einstein's.

When examining the extremely low temperature limit: \(\dfrac{\theta_E}{T}\gg1\), it can be seen:

\[ C_V=3Nk\left(\dfrac{\theta_E}{T}\right)^2e^\dfrac{-\theta_E}{T} \label{5} \]

As temperature (\(T\)) goes to zero, the exponential portion of the above equation goes to zero and therefore \(C_V\) also approaches zero. This supports the experimental values as temperature approaches zero the heat capacity of the solid likewise decreases to zero.

Einstein's theory also explains solids that exhibit a low heat capacity even at relatively high temperatures. An example of such a solid is diamond. The heat capacity of diamond approaches \(3Nk\) as temperature greatly increases. Einstein's model supports this through the definition of an Einstein temperature. As the Einstein temperature increases, \(\nu\) must increase likewise. This is the equivalent of each atom possessing more energy and therefore vibrating more rapidly within the solid itself. The oscillator frequency, \(\nu\), can be written as:

\[ \nu=\dfrac{1}{2\pi}\sqrt{\dfrac{\kappa}{\mu}} \label{6} \]

where \(\kappa\) is the force constant and \(\mu\) is the reduced mass. This formula better predicts solids with high force constants or low reduced masses. This corrects deviations from the Petit and Dulong model.

Figure 17.4.4 . This graph indicates the heat capacity of several solid metals as a function of the Einstein temperature: \(\theta_E=\dfrac{hv}{k_B}\).

Essentially the Einstein temperature allows for the heat capacity equation and the vibrational frequencies in the solid to change as the temperature changes. This effectively adjusts for the deviations seen in the Petit/Dulong model. As the temperature increases or decreases, the Einstein temperature increases or decreases likewise to mirror the actual physical activity within the solid.

Figure 17.4.5 . This plot shows the heat capacity of lead compared to the expected values calculated from Einstein's model.

Einstein's model predicts relatively low temperatures well. However, when decreasing from approximately 15 K, Einstein's model deviates from experimental values. Also, this can be observed, although not as dramatically, for temperatures from 25 K to 30 K. Clearly a term or correction is still missing from Einstein's model to increase its accuracy.