4.3: The Particle-in-a-Box Model
- Page ID
- 4496
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\(\newcommand{\ket}[1]{\left| #1 \right>} \)
\( \newcommand{\bra}[1]{\left< #1 \right|} \)
\( \newcommand{\braket}[2]{\left< #1 \vphantom{#2} \right| \left. #2 \vphantom{#1} \right>} \)
\( \newcommand{\qmvec}[1]{\mathbf{\vec{#1}}} \)
\( \newcommand{\op}[1]{\hat{\mathbf{#1}}}\)
\( \newcommand{\expect}[1]{\langle #1 \rangle}\)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The particle-in-a box model is used to approximate the Hamiltonian operator for the \(\pi\) electrons because the full Hamiltonian is quite complex. The full Hamiltonian operator for each electron consists of the kinetic energy term \(\dfrac {-\hbar ^2}{2m} \dfrac {d^2}{dx^2}\) and the sum of the Coulomb potential energy terms \(\dfrac {q_1q_2}{4\pi \epsilon _0 r_{12}}\) for the interaction of each electron with all the other electrons and with the nuclei (\(q\) is the charge on each particle and \(r\) is the distance between them). Considering these interactions, the Hamiltonian for electron i given below.
\[ \hat {H} _i = \dfrac {- \hbar ^2}{2m} \dfrac {d^2}{dx^2} + \underset{\text{sum over electrons}}{ \sum _{j } \dfrac {e^2}{4 \pi \epsilon _0 r_{i, j}}} - \underset{\text{sum over nuclei}}{ \sum _{n} \dfrac {e^2 Z_n}{4 \pi \epsilon _0 r_{i,n}} } \label {4-1}\]
The Schrödinger equation obtained with this Hamiltonian cannot be solved analytically by anyone because of the electron-electron interaction terms. Some approximations for the potential energy must be made.
We want a model for the dye molecules that has a particularly simple potential energy function because we want to be able to solve the corresponding Schrödinger equation easily. The particle-in-a-box model has the necessary simple form. It also permits us to get directly at understanding the most interesting feature of these molecules, their absorption spectra.
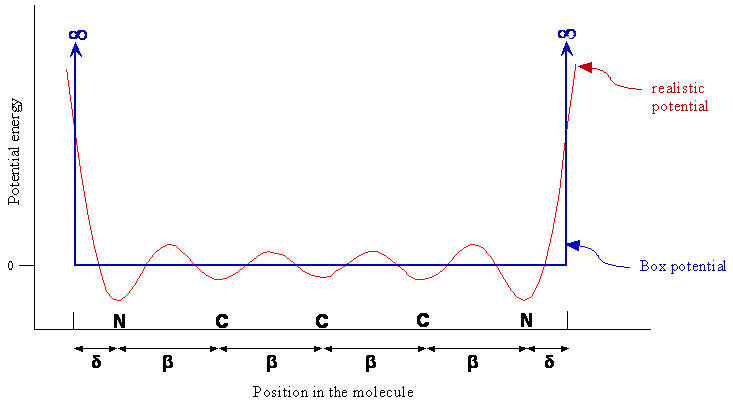
As mentioned in the previous section, we assume that the π-electron motion is restricted to left and right along the chain in one dimension. The average potential energy due to the interaction with the other electrons and with the nuclei is taken to be a constant except at the ends of the molecule. At the ends, the potential energy increases abruptly to a large value; this increase in the potential energy keeps the electrons bound within the conjugated part of the molecule. Figure \(\PageIndex{1}\) shows the classical particle-in-a-box potential function and the more realistic potential energy function. We have defined the constant potential energy for the electrons within the molecule as the zero of energy. One end of the molecule is set at \(x = 0\), the other at \(x = L\), and the potential energy is goes to infinity at these points.
For one electron located within the box, i.e. between \(x = 0\) and \(x = L\), the Hamiltonian is
\[\hat {H} = \dfrac {-\hbar ^2}{2m} \dfrac {d^2}{dx^2}\]
because \(V =0\), and the (time-independent) Schrödinger equation that needs to be solved is then
\[\dfrac {- \hbar ^2}{2m} \dfrac {d^2}{dx^2} \psi (x) = E \psi (x) \label {4-2}\]
We need to solve this differential equation to find the wavefunction and the energy. In general, differential equations have multiple solutions (solutions that are families of functions), so actually by solving this equation, we will find all the wavefunctions and all the energies for the particle-in-a-box. There are many ways of solving differential equations, and you will see some of them illustrated here and in subsequent chapters. One way is to recognize functions that might satisfy the equation. This equation says that differentiating the function twice produces the function times a constant. What kinds of functions have you seen that regenerate the function after differentiating twice? Exponential functions and sine and cosine functions come to mind.
Example \(\PageIndex{1}\)
Use \(\sin(kx)\), \(\cos(kx)\), and \(e^{ikx}\) for the possible wavefunctions in Equation \(\ref{4-2}\) and differentiate twice to demonstrate that each of these functions satisfies the Schrödinger equation for the particle-in-a-box.
Exercise \(\PageIndex{1}\) leads you to the following three equations.
\[\dfrac {\hbar ^2 k^2}{2m} \sin (kx) = E \sin (kx) \label {4-3}\]
\[\dfrac {\hbar ^2 k^2}{2m} \cos (kx) = E \cos (kx) \label {4-4}\]
\[\dfrac {\hbar ^2 k^2}{2m} e^{ikx} = E e^{ikx} \label {4-5}\]
For the equalities expressed by these equations to hold, \(E\) must be given by
\[E = \dfrac {\hbar ^2 k^2}{2m} \label {4-6}\]
Kinetic energy is the momentum squared divided by twice the mass \(p^2/2m\), so we conclude from Equation \(\ref{4-6}\) that \(ħ^2k^2 = p^2\).
Solutions to differential equations that describe the real world also must satisfy conditions imposed by the physical situation. These conditions are called boundary conditions.
For the particle-in-a-box, the particle is restricted to the region of space occupied by the conjugated portion of the molecule, between \(x = 0\) and \(x = L\). If we make the large potential energy at the ends of the molecule infinite, then the wavefunctions must be zero at \(x = 0\) and \(x = L\) because the probability of finding a particle with an infinite energy should be zero. Otherwise, the world would not have an energy resource problem. This boundary condition therefore requires that \(ψ(0) = ψ(L) = 0\).
Example \(\PageIndex{2}\)
Which of the functions \(sin(kx)\), \(cos(kx)\), or \(e^{ikx}\) is 0 when x = 0?
As you discovered in Exercise \(\PageIndex{2}\) for these three functions, only \(sin(kx) = 0\) when \(x = 0\). Consequently only \(sin(kx)\) is a physically acceptable solution to the Schrödinger equation.
The boundary condition described above also requires us to set \(ψ(L) = 0\).
\[ψ(L) = \sin(kL) = 0 \label {4.7}\]
The sine function will be zero if \(kL = nπ\) with \(n = 1,2,3, \cdots\). In other words,
\[ k = \dfrac {n \pi}{L} \label {4-8}\]
with \(n = 1, 2, 3 \cdots\)
Note that \(n = 0\) is not acceptable here because this makes the wave vector zero \(k = 0\), so \(\sin(kx) = 0\), and thus \(ψ(x)\) is zero everywhere. If the wavefunction were zero everywhere, it means that the probability of finding the electron is zero. This clearly is not acceptable because it means there is no electron.
Example \(\PageIndex{3}\)
Show that \(\sin(kx) = 0\) at \(x = L\) if \(k = nπ/L\) and \(n\) is an integer.
Negative Quantum Numbers
It appears that a negative integer also would work for \(n\) because
\[\sin \left ( \dfrac {-n \pi}{L} x \right ) = - \sin \left ( \dfrac {n \pi}{L} x \right ) \label {4.9}\]
which also satisfies the boundary condition at \(x = L\). The reason negative integers are not used is a bit subtle. Changing \(n\) to \(–n\) just changes the sign (also called the phase) of the wavefunction from + to -, and does not produce a function describing a new state of the particle. Note that the probability density for the particle is the absolute square of the function, and the energies are the same for \(n\) and \(–n\). Also, since the wave vector k is associated with the momentum (p = ħk), n > 0 means k > 0 corresponding to momentum in the positive direction, and \(n < 0\) means \(k < 0\) corresponding to momentum in the negative direction. By using Euler’s formula one can show that the sine function incorporates both \(k\) and \(–k\) since
\[ \sin (kx) = \dfrac {1}{2i} ( e^{ikx} - e^{-ikx} ) \label {4-10}\]
so changing \(n\) to \(–n\) and \(k\) to \(–k\) does not produce a function describing new state, because both momentum states already are included in the sine function.
The set of wavefunctions that satisfies both boundary conditions is given by
\[ \psi _n (x) = N \sin \left ( \dfrac {n \pi}{L} x \right ) \text {with } n = 1, 2, 3, \cdots \label {4-11}\]
The normalization constant N is introduced and evaluated to satisfy the normalization requirement.
\[ \int \limits _0^L \psi ^* (x) \psi (x) dx = 1 \label {4- 12}\]
\[N^2 \int \limits _0^L \sin ^2 \left ( \dfrac {n \pi x}{L} \right ) dx = 1 \label {4-13}\]
\[N = \sqrt{ \dfrac{1}{\int \limits _0^L \sin ^2 \dfrac {n\pi x}{L} dx} } \label {4-14}\]
\[ N = \sqrt{ \dfrac {2}{L}} \label {4-15}\]
Finally we write the wavefunction:
\[ \psi _n (x) = \sqrt{ \dfrac {2}{L} } \sin \left ( \dfrac {n \pi}{L} x \right ) \label {4-16}\]
Example \(\PageIndex{4}\)
Evaluate the integral in Equation \(\ref{4-13}\) and show that \(N = (2/L)^{1/2}\).
By finding the solutions to the Schrödinger equation and imposing boundary conditions, we have found a whole set of wavefunctions and corresponding energies for the particle-in-a box. The wavefunctions and energies depend upon the number n, which is called a quantum number. In fact there are an infinite number of wavefunctions and energy levels, corresponding to the infinite number of values for n \(n = 1 \rightarrow \infty\). The wavefunctions are given by Equation \(\ref{4-16}\) and the energies by Equation \(\ref{4-6}\). If we substitute the expression for k from Equation \(\ref{4-8}\) into Equation \(\ref{4-6}\), we obtain the equation for the energies \(E_n\)
\[ E_n = \dfrac {n^2 \pi ^2 \hbar ^2}{2mL^2} = n^2 \left (\dfrac {h^2}{8mL^2} \right ) \label {4-17}\]
Example \(\PageIndex{4}\)
Substitute the wavefunction, Equation \(\ref{4-16}\), into Equation \(\ref{4.2}\) and differentiate twice to obtain the expression for the energy given by Equation \(\ref{4-17}\).
From Equation \(\ref{4-17}\) we see that the energy is quantized in units of \(\dfrac {h^2}{8mL^2}\); i.e. only certain values for the energy of the particle are possible. This quantization, the dependence of the energy on integer values for n, results from the boundary conditions requiring that the wavefunction be zero at certain points. We will see in other chapters that quantization generally is produced by boundary conditions and the presence of Planck’s constant in the equations.
The lowest-energy state of a system is called the ground state. Note that the ground state (\(n = 1\)) energy of the particle-in-a-box is not zero. This energy is called the zero-point energy.
Example \(\PageIndex{5}\)
Here is a neat way to deduce or remember the expression for the particle-in-a-box energies. The momentum of a particle has been shown to be equal to \(ħk\). Show that this momentum, with \(k\) constrained to be equal to \(nπ/L\), combined with the classical expression for the kinetic energy in terms of the momentum \((p^2/2m)\) produces Equation \(\ref{4.17}\). Determine the units for \(\dfrac {h^2}{8mL^2}\) from the units for \(h\), \(m\), and \(L\).
Example \(\PageIndex{6}\)
Why must the wavefunction for the particle-in-a-box be normalized? Show that φ(x) in Equation \(\ref{4-16}\) is normalized.
Example \(\PageIndex{6}\)
Use a spreadsheet program, Mathcad, or other suitable software to construct an accurate energy level diagram and to plot the wavefunctions and probability densities for a particle-in-a-box with \(n = 1\) to \(6\). You can make your graphs universal, i.e. apply to any particle in any box, by using the quantity \((h^2/8mL^2)\) as your unit of energy and \(L\) as your unit of length. To make these universal graphs, plot \(n^2\) on the y-axis of the energy-level diagram, and plot \(x/L\) from \(0\) to \(1\) on the x-axis of your wavefunction and probability density graphs.
Example \(\PageIndex{7}\)
How does the energy of the electron depend on the size of the box and the quantum number n? What is the significance of these variations with respect to the spectra of cyanine dye molecules with different numbers of carbon atoms and pi electrons? Plot \(E(n_2)\), \(E(L_2)\), and \(E(n)\) on the same figure and comment on the shape of each curve.
The quantum number serves as an index to specify the energy and wavefunction or state. Note that \(E_n\) for the particle-in-a-box varies as \(n^2\) and as \(1/L^2\), which means that as \(n\) increases the energies of the states get further apart, and as \(L\) increases the energies get closer together. How the energy varies with increasing quantum number depends on the nature of the particular system being studied; be sure to take note of the relationship for each case that is discussed in subsequent chapters.
David M. Hanson, Erica Harvey, Robert Sweeney, Theresa Julia Zielinski ("Quantum States of Atoms and Molecules")


