Fundamentals of Chirality

Last updated
Save as PDF

Page ID: 798

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Stereoisomers are isomers that differ in spatial arrangement of atoms, rather than order of atomic connectivity. One of their most interesting type of isomer is the mirror-image stereoisomers, a non-superimposable set of two molecules that are mirror image of one another. The existance of these molecules are determined by concept known as chirality. The word "chiral" was derived from the Greek word for hand, because our hands display a good example of chirality since they are non-superimposable mirror images of each other.

Introduction

The opposite of chiral is achiral. Achiral objects are superimposable with their mirror images. For example, two pieces of paper are achiral. In contrast, chiral molecules, like our hands, are non superimposable mirror images of each other.

Try to line up your left hand perfectly with your right hand, so that the palms are both facing in the same directions. Spend about a minute doing this. Do you see that they cannot line up exactly? The same thing applies to some molecules

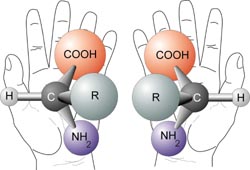
A Chiral molecule has a mirror image that cannot line up with it perfectly- the mirror images are non superimposable. The mirror images are called enantiomers.

But why are chiral molecules so interesting? A chiral molecule and its enantiomer have the same chemical and physical properties(boiling point, melting point,polarity, density etc...). It turns out that many of our biological molecules such as our DNA, amino acids and sugars, are chiral molecules.

It is pretty interesting that our hands seem to serve the same purpose but most people are only able to use one of their hands to write. Similarily this is true with chiral biological molecules and interactions. Just like your left hand will not fit properly in your right glove, one of the enantiomers of a molecule may not work the same way in your body.

This must mean that enantiomers have properties that make them unique to their mirror images. One of these properties is that they cannot have a plane of symmetry or an internal mirror plane. So, a chiral molecule cannot be divided in two mirror image halves. Another property of chiral molecules is optical activity.

Optical Activity

As mentioned before, chiral molecules are very similar to each other since they have the same components to them. The only thing that obviously differs is their arrangement in space. As a result of this similarity, it is very hard to distinguish chiral molecules from each other when we try to compare their properties such as boiling points, melting points and densities.

However, we can differentiate them by their optical activity. When a plane-polarized light is passed through one of the 2 enantiomers of a chiral molecule that molecule rotates light in a certain direction. When the same plane polarized light is passed through the other enantiomer, that enantionmer rotates light by the same amount but in the opposite direction.

If one enantiomer rotates the light counterclockwise, the other would rotate it clockwise. Because chiral molecules are able to rotate the plane of polarization differently by interacting with the electric field differently, they are said to be optically active. In general molecules that rotate light in differen directions are called optical isomers.

Dextrorotatory (+ enantiomer) – rotates the plane polarized light clockwise (when viewing towards the light source)
Levorotatory (- enantiomer) – rotates the plane polarized light counterclockwise

Circular Dichroism

Another property of chiral molecules is called circular dichroism (CD). This pertains to their differential absorption of left and right circularly polarized light. When left and right circularly polarized light passes through chiral molecules, the absorption coefficients differ so that the change in absorption coefficients does not equal zero.

240px-Polarisation_(Circular).svg.png

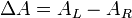
$\Delta A=A_L-A_R \,$

where ΔA is the difference between absorbance of left circularly polarized (LCP) and right circularly polarized (RCP) light (this is what is usually measured). Where [J] = molar concentration of the sample and l is the path length.

The CD signals of chiral molecules can give important information and this information can be used for visible and ultraviolet spectroscopy. Every chiral molecule shows a particular CD spectrum. By looking at the distinctive spectra of molecules such as proteins and DNA, we can obtain useful information about their secondary structures and see how they differ. An example of a CD spectrum comparing the differences between A-DNA and B-DNA.

Inside Links

References

Atkins, Peter and de Paula, Julio. Physical Chemistry for the Life Sciences. New York, N.Y.: W. H. Freeman Company, 2006. (565-566).
Croft, William J. Under the Microscope: a brief history of microscopy. Singapore: Mainland Press, 2006. (39).
Vollhardt, K. Peter C. and Schore, Neil E. Organic Chemistry: Structure and Function. Fourth Edition. New York, N.Y.: W. H. Freeman Company, 2003. (165-169).
Wagniere, George H. On Chirality and the Universal Asymmetry: Reflection on Image and Mirror Image. Zurich, Switzerland, 2007. (3-7).

Contributors

Kristeen Pareja (UCD), Ifemayowa Aworanti (University Of Maryland Baltimore County)

Search

Text Color

Text Size

Margin Size

Font Type