8.4: Degrees of Unsaturation
- Page ID
- 45189
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Learning Objectives
- calculate the Degrees of Unsaturation (DU) and apply it to alkene structure
Saturated and Unsaturated Molecules
In the lab, saturation may be thought of as the point when a solution cannot dissolve anymore of a substance added to it. In terms of degrees of unsaturation, a molecule only containing single bonds with no rings is considered saturated.
| CH3CH2CH3 | 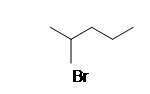 |
.bmp?revision=1&size=bestfit&width=113&height=128) |
1-methyoxypentane |
Unlike saturated molecules, unsaturated molecules contain double bond(s), triple bond(s) and/or ring(s).
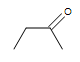
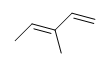
| CH3CH=CHCH3 | 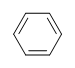 |
 |
 |
3-chloro-5-octyne |
There are many ways one can go about determining the structure of an unknown organic molecule. Although, nuclear magnetic resonance (NMR) and infrared radiation (IR) are the primary ways of determining molecular structures, these techniques require expensive instrumentation and are not always readily available. Fortunately, calculating the degrees of unsaturation provides useful information about the structure. The degree of unsaturation indicates the total number of pi bonds and rings within a molecule which makes it easier for one to figure out the molecular structure.
DU = Degrees of Unsaturation = (number of pi bonds) + (number of rings)
Alkenes (R2C=CR2) and alkynes (R–C≡C–R) are called unsaturated hydrocarbons because they have fewer hydrogen atoms than does an alkane with the same number of carbon atoms, as is indicated in the following general formulas:
Calculating The Degree of Unsaturation (DU)
If the molecular formula is given, plug in the numbers into this formula:
\[ DoU= \dfrac{2C+2+N-X-H}{2} \tag{7.2.1}\]
- \(C\) is the number of carbons
- \(N\) is the number of nitrogens
- \(X\) is the number of halogens (F, Cl, Br, I)
- \(H\) is the number of hydrogens
The molecular formula of a hydrocarbon provides information about the possible structural types it may represent. A saturated molecule contains only single bonds and no rings. Another way of interpreting this is that a saturated molecule has the maximum number of hydrogen atoms possible to be an acyclic alkane. Thus, the number of hydrogens can be represented by 2C+2, which is the general molecular representation of an alkane. As an example, for the molecular formula C3H4 the number of actual hydrogens needed for the compound to be saturated is 8
[2C+2=(2x3)+2=8.]
The compound needs 4 more hydrogens in order to be fully saturated (expected number of hydrogens-observed number of hydrogens=8-4=4). Degrees of unsaturation is equal to 2, or half the number of hydrogens the molecule needs to be classified as saturated. Hence, the DoB formula divides by 2. The formula subtracts the number of X's because a halogen (X) replaces a hydrogen in a compound. For instance, in chloroethane, C2H5Cl, there is one less hydrogen compared to ethane, C2H6. For example, consider compounds having the formula C5H8. The formula of the five-carbon alkane pentane is C5H12 so the difference in hydrogen content is 4. This difference suggests such compounds may have a triple bond, two double bonds, a ring plus a double bond, or two rings. Some examples are shown here, and there are at least fourteen others!
For a compound to be saturated, there is one more hydrogen in a molecule when nitrogen is present. Therefore, we add the number of nitrogens (N). This can be seen with C3H9N compared to C3H8. Oxygen and sulfur are not included in the formula because saturation is unaffected by these elements. As seen in alcohols, the same number of hydrogens in ethanol, C2H5OH, matches the number of hydrogens in ethane, C2H6.
The following chart illustrates the possible combinations of the number of double bond(s), triple bond(s), and/or ring(s) for a given degree of unsaturation. Each row corresponds to a different combination.
- One degree of unsaturation is equivalent to 1 ring or 1 double bond (1 \( \pi \) bond).
- Two degrees of unsaturation is equivalent to 2 double bonds, 1 ring and 1 double bond, 2 rings, or 1 triple bond (2 \( \pi \) bonds).
When the DU is 4 or greater, the presence of benzene rings is very likely.
|
DU
|
Possible combinations of rings/ bonds
|
||
|---|---|---|---|
|
|
# of rings
|
# of double bonds
|
# of triple bonds
|
|
1
|
1
|
0
|
0
|
|
|
0
|
1
|
0
|
|
2
|
2
|
0
|
0
|
|
|
0
|
2
|
0
|
|
|
0
|
0
|
1
|
|
|
1
|
1
|
0
|
Remember, the degrees of unsaturation only gives the sum of double bonds, triple bonds and/or rings. For instance, a degree of unsaturation of 3 can contain 3 rings, 2 rings+1 double bond, 1 ring+2 double bonds, 1 ring+1 triple bond, 1 double bond+1 triple bond, or 3 double bonds.
Example: Benzene
What is the Degree of Unsaturation for Benzene?
Solution
The molecular formula for benzene is C6H6. Thus,
DU= 4, where C=6, N=0,X=0, and H=6. 1 DoB can equal 1 ring or 1 double bond. This corresponds to benzene containing 1 ring and 3 double bonds.

However, when given the molecular formula C6H6, benzene is only one of many possible structures (isomers). The following structures all have DU of 4 and have the same molecular formula as benzene. However, these compounds are very rare, unlike benzene. We will learn more about the reasons for benzen's high stability when we studey aromaticity in later chapters.

Exercises
- Are the following molecules saturated or unsaturated:
 (b.)
(b.)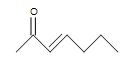 (c.)
(c.) 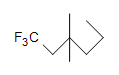 (d.) C10H6N4
(d.) C10H6N4
- Using the molecules from (1) above, give the degrees of unsaturation for each.
- Calculate the degrees of unsaturation, classify the compound as saturated or unsaturated, and list all the ring/pi bond combination possible for the following molecular formulas: (a.) C9H20 (b.) C7H8 (c.) C5H7Cl (d.) C9H9NO4
- Calculate degrees of unsaturation (DoU) for the following, and propose a structure for each.
a) C5H8
b) C4H4
-
Calculate the degree of unsaturation (DoU) for the following molecules
a) C5H5N
b) C5H5NO2
c) C5H5Br
-
The following molecule is caffeine (C8H10N4O2), determine the degrees of unsaturation (DoU).
- Answer
-
1.
(a.) unsaturated (Even though rings only contain single bonds, rings are considered unsaturated.)
(b.) unsaturated
(c.) saturated
(d.) unsaturated
2. If the molecular structure is given, the easiest way to solve is to count the number of double bonds, triple bonds and/or rings. However, you can also determine the molecular formula and solve for the degrees of unsaturation by using the formula.
(a.) 2
(b.) 2 (one double bond and the double bond from the carbonyl)
(c.) 0
(d.) 10
3.
(a.) DU =0 ; saturated (Remember-a saturated molecule only contains single bonds)
(b.) DU = 4; unsaturated The molecule can contain any of these combinations of rings and pi bonds that add up to 4, such as (i) 4 double bonds (ii) 4 rings (iii) 2 double bonds+2 rings (iv) 1 double bond+3 rings (v) 3 double bonds+1 ring (vi) 1 triple bond+2 rings (vii) 2 triple bonds (viii) 1 triple bond+1 double bond+1 ring (ix) 1 triple bond+2 double bonds
(c.) DU = 2; unsaturated (i) 1 triple bond (ii) 1 ring+1 double bond (iii) 2 rings (iv) 2 double bonds
(d.) DU = 6; (i) 3 triple bonds (ii) 2 triple bonds+2 double bonds (iii) 2 triple bonds+1 double bond+1 ring (iv)... (As you can see, the degrees of unsaturation only gives the sum of double bonds, triple bonds and/or ring. Thus, the formula may give numerous possible structures for a given molecular formula.)
4.
5. a) 4 b) 4 c) 3
6. DU = 6

