5.2: Theory of NMR

Objectives

After reading this section, you should be able to

• understand why NMR works
• understand why different hydrogens do not all show up in the same location on a spectrum

Introduction

Nuclear magnetic resonance (NMR) plays an important role in the fields chemistry, materials science, physics and engineering. In 1946, NMR was co-discovered by Purcell, Pound and Torrey of Harvard University and Bloch, Hansen and Packard of Stanford University. The discovery first came about when it was noticed that magnetic nuclei, such as ¹H and ³¹P (read: proton and Phosphorus 31) were able to absorb radiofrequency energy when placed in a magnetic field of a strength that was specific to the nucleus. Upon absorption, the nuclei begin to resonate and different atoms within a molecule resonated at different frequencies. This observation allowed a detailed analysis of the structure of a molecule. Since then, NMR has been applied to solids, liquids and gasses, kinetic and structural studies, resulting in 6 Nobel prizes being awarded in the field of NMR. 

Nuclear magnetic resonance, NMR, is a physical phenomenon of resonance transition between magnetic energy levels, happening when atomic nuclei are immersed in an external magnetic field and an applied electromagnetic radiation with specific frequency. By detecting the absorption signals, one can acquire NMR spectrum. According to the positions, intensities and fine structure of resonance peaks, people can study the structures of molecules quantitatively. The size of molecules of interest varies from small organic molecules, to biological molecules of middle size, and even to some macromolecules such as nucleic acids and proteins. Apart from these commonly utilized applications in organic compounds, NMR also plays an important role in analyzing inorganic molecules, which makes NMR spectroscopy a powerful technique.

But the a major question still remains- Why does NMR work? Some types of atomic nuclei act as though they spin on their axis similar to the Earth. Since they are positively charged they generate an electromagnetic field just as the Earth does. In effect, they will act as tiny bar magnetics. Not all nuclei act this way, but fortunately both ¹H and ¹³C do have nuclear spins and will respond to this technique.

The concept of spin is regularly addressed in subatomic particle physics. However, to most people spin seems like an abstract concept. This is due to the fact there is no macroscopic equivalent of what spin is. However, for those people who have taken an introduction to chemistry course have seen the concept of spin in electrons. Electrons are subatomic particles which have spin intrinsic to them. The nucleus is not much different. Spin is just another form of angular momentum. The nucleus consists of protons and neutrons and neutrons and protons are comprised of subatomic particles known as quarks and gluons. The neutron has 2 quarks with a -e/3 charge and one quark with a +2e/3 charge resulting in a total charge of 0. The proton however, has 2 quarks with +2e/3 charge and only one quark with a -e/3 charge giving it a net positive charge. Both protons and neutrons are spin=1/2.

Figure \(\PageIndex{1}\): The atomic nucleus (black) of ²H. The proton (green) and neutron (red) are composed of quarks (purple and teal) which have a charge and spin (arrow).

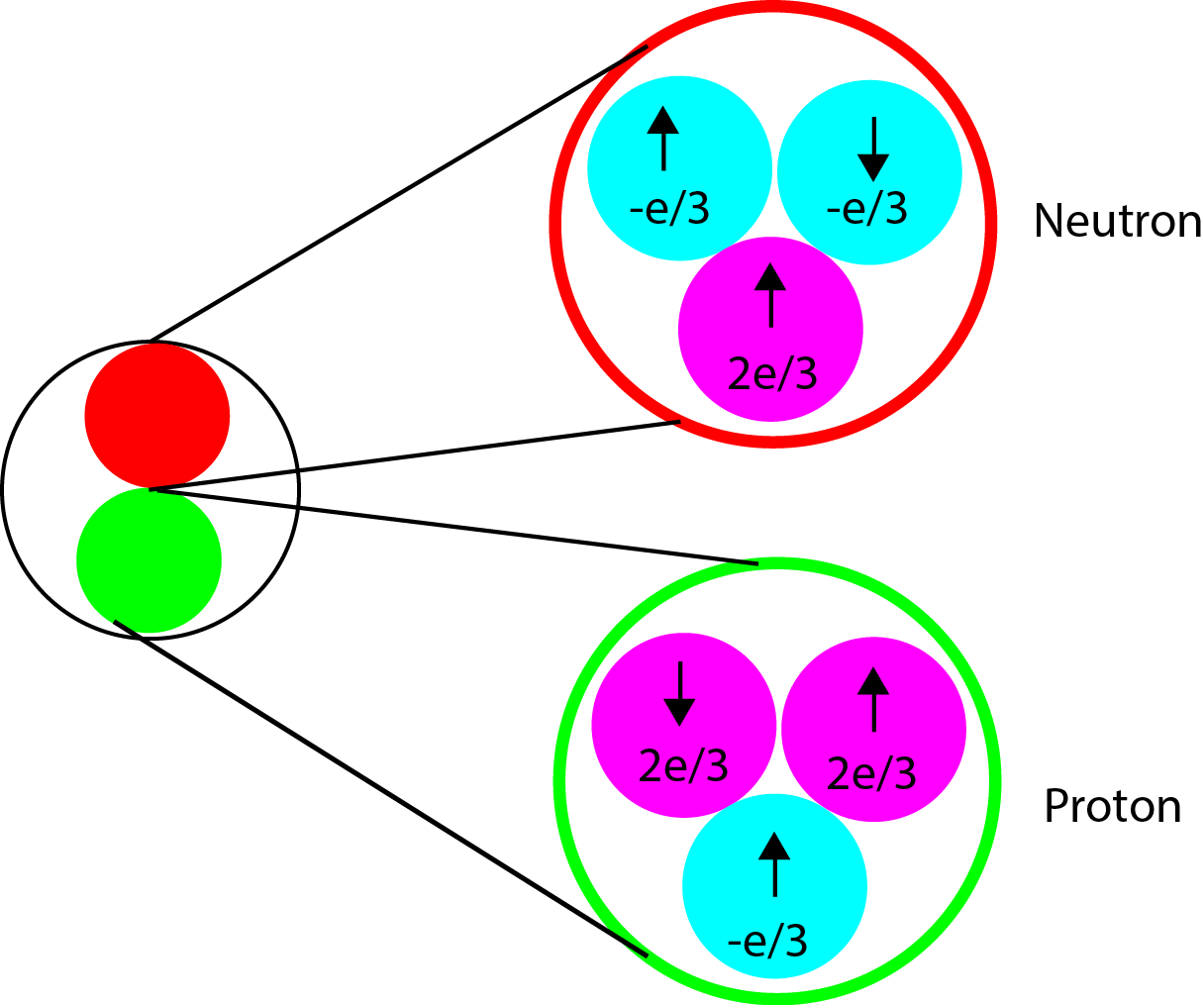
For any system consisting of n multiple parts, each with an angular momentum the total angular momentum can be described by J where

\[J=|J_1+J_2+...+J_n|, |J_1+J_2+...+J_n| -1,...|J_1-J_2-...-J_n|\]

\[a=mx^2\]

Here are some examples using the isotopes of hydrogen

• ¹H = 1 proton so J=1/2
• ²H= 1 proton and 1 neutron so \(J=1\) or 0.

For larger nuclei, it is not immediately evident what the spin should be as there are a multitude of possible values. For the remainder of the discussion we will attribute the spin of the nucleus, I, to be an intrinsic value. There are some rules that the nuclei do follow with respect to nuclear spin. They are summarized in the table below.

Table \(\PageIndex{1}\): General rules for determination of nuclear spin quantum numbers

Mass Number	Number of Protons	Number of Neutrons	Spin (I)	Example
Even	Even	Even	0	^16O
	Odd	Odd	Integer (1,2,...)	^2H
Odd	Even	Odd	Half-Integer (1/2, 3/2,...)	^13C
	Odd	Even	Half-Integer (1/2, 3/2,...)	^15N

Atomic nuclei with even numbers of protons and neutrons have zero spin and all the other atoms with odd numbers have a non-zero spin. Furthermore, all molecules with a non-zero spin have a magnetic moment, \(\mu\), given by

\[\mu=\gamma I\]

where \(\gamma\) is the gyromagnetic ratio, a proportionality constant between the magnetic dipole moment and the angular momentum, specific to each nucleus (Table 2). In other words, ¹H and ¹³C are not unique in their ability to undergo NMR. All nuclei with an odd number of protons (¹H, ²H, ¹⁴N, ¹⁹F, ³¹P) or nuclei with an odd number of neutrons (i.e. ¹³C) show the magnetic properties required for NMR. Only nuclei with even number of both protons and neutrons (¹²C and ¹⁶O) do not have the required magnetic properties.

Table \(\PageIndex{2}\): The gyromagnetic ratios for several common nuclei

Nuclei	Spin	Gyromagetic Ratio (MHz/T)	Natural Abundance (%)
^1H	1/2	42.576	99.9985
^13C	1/2	10.705	1.07
^31P	1/2	17.235	100
^27Al	5/2	11.103	100
^23Na	3/2	11.262	100
^7Li	3/2	16.546	92.41
^29Si	1/2	-8.465	4.68
^17O	5/2	5.772	0.038
^15N	1/2	-4.361	0.368

The magnetic moment of the nucleus forces the nucleus to behave as a tiny bar magnet. In the absence of an external magnetic field, each magnet is randomly oriented (as can be seen in the figure below on the left). During the NMR experiment the sample is placed in an external magnetic field, \(B_0\), which forces the bar magnets to align with (low energy) or against (high energy) the \(B_0\). The nuclear spins will adopt specific orientations like a compass needle responds to the Earth’s magnetic field and aligns with it. Two possible orientations are possible, with the external field (i.e. parallel to and in the same direction as the external field) or against the field (i.e. antiparallel to the external field) as can be seen in the figure below on the right. 

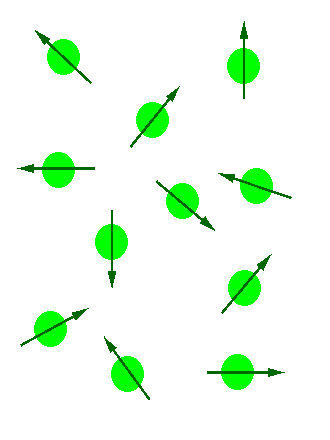
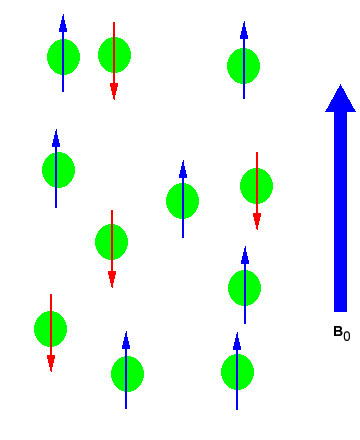
(Left) Random nuclear spin without an external magnetic field. (Right) Ordered nuclear spin in an external magnetic field

 

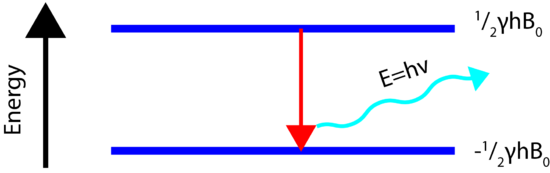
If the ordered nuclei are now subjected to electromagnetic (EM) radiation, specifically radiofrequency, of the proper frequency the nuclei aligned with the field will absorb energy and "spin-flip" to align themselves against the field, a higher energy state. When this spin-flip occurs the nuclei are said to be in "resonance" with the field, hence the name for the technique, Nuclear Magentic Resonance.

Figure \(\PageIndex{2}\): Absorption of radio frequency radiation to promote a transition between nuclear energy levels, called a spin flip.

 

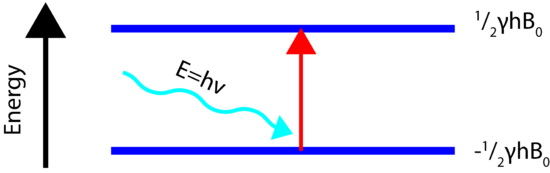
In order for the NMR experiment to work, a spin flip between the energy levels must occur. The energy difference between the two states corresponds to the energy of the electromagnetic radiation that causes the nuclei to change their energy levels. For most NMR spectrometers, \(B_0\) is on the order of Tesla (T) while \(\gamma\) is on the order of \(10^7\). Consequently, the electromagnetic radiation required is on the order of 100's of MHz and even GHz. The energy of a photon is represented by

\[E=h\nu\]

and thus the frequency necessary for absorption to occur is represented as:

\[\nu=\dfrac{\gamma B_0}{2\pi}\]

For the beginner, the NMR experiment measures the resonant frequency that causes a spin flip. For the more advanced NMR users, the sections on NMR detection and Larmor frequency should be consulted.

The amount of energy, and hence the exact frequency of EM radiation required for resonance to occur is dependent on both the strength of the magnetic field applied and the type of the nuclei being studied. As the strength of the magnetic field increases the energy difference between the two spin states increases and a higher frequency (more energy) EM radiation needs to be applied to achieve a spin-flip (see image below).

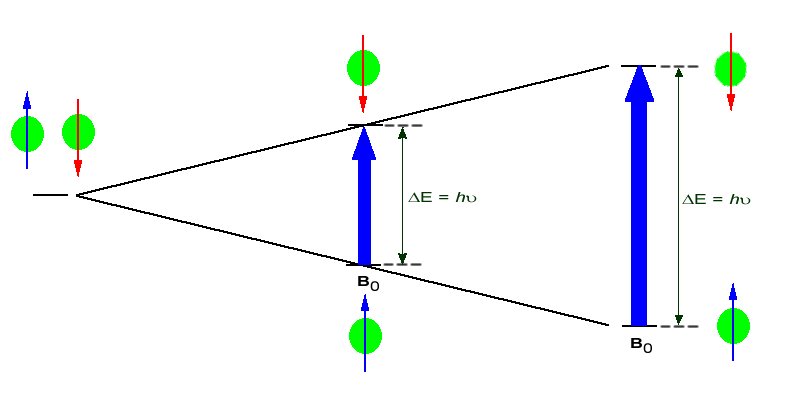
Superconducting magnets can be used to produce very strong magnetic field, on the order of 21 tesla (T). Lower field strengths can also be used, in the range of 4 - 7 T. At these levels the energy required to bring the nuclei into resonance is in the MHz range and corresponds to radio wavelength energies, i.e. at a field strength of 4.7 T 200 MHz bring ¹H nuclei into resonance and 50 MHz bring ¹³C into resonance. This is considerably less energy then is required for IR spectroscopy, ~10^-4 kJ/mol versus ~5 - ~50 kJ/mol.

If all ¹H nuclei have the same gyromagnetic ratio, shouldn't all the ¹H absorb the same energy, so would all show up at the same spot? Luckily, no. The power of NMR is based on the concept of nuclear shielding, which allows for structural assignments. Every atom is surrounded by electrons, which orbit the nucleus. Charged particles moving in a loop will create a magnetic field which is felt by the nucleus. Therefore the local electronic environment surrounding the nucleus will slightly change the magnetic field experienced by the nucleus, which in turn will cause slight changes in the energy levels! This is known as shielding. Nuclei that experience different magnetic fields due to the local electronic interactions are known as nonequivalent nuclei (B_experienced = B₀ - B_isigma). The change in the energy levels requires a different frequency to excite the spin flip, which as will be seen below, creates a new peak in the NMR spectrum. The shielding allows for structural determination of molecules.

Figure \(\PageIndex{3}\): The effect that shielding from electrons has on the splitting of the nuclear energy levels. Electrons impart their own magnetic field which shields the nucleus from the externally applied magnetic field. This effect is greatly exaggerated in this illustration.

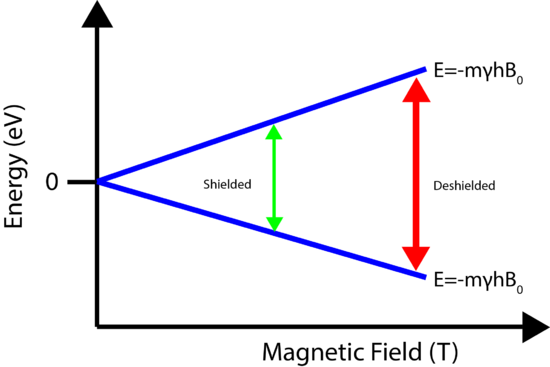
The shielding of the nucleus allows for chemically nonequivalent environments to be determined by Fourier Transforming the NMR signal. The result is a spectrum, shown below, that consists of a set of peaks in which each peak corresponds to a distinct chemical environment. 

Figure \(\PageIndex{4}\): ³¹P spectrum of phosphinic acid. Each peak corresponds to a distinct chemical environment while the area under the peak is proportional to the number of nuclei in a given environment.

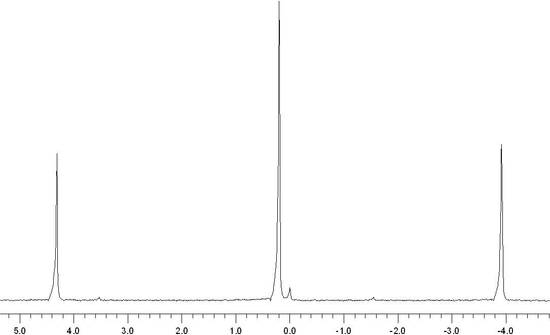
In ¹H NMR spectrum, hydrogen atoms bound to a carbon consisting of a double bond are typically found in low field of the NMR spectrum and the hydrogens are considered deshielded. The cause for this is due to the movement of the electrons in the pi bond of the carbon-carbon double bond and how the magnetic field effects the protons bound to the pi bond.

The pi system creates an external magnetic field that is perpendicular to the double bond axis and causes the electrons in the pi bond to enter a circular motion (shown in red). The circular motion actually reinforces the external field at the edge of the double bond on both sides of the pi bond but creates a local field (shown in purple and green) that opposes the external field in the center of the double bond. Because of this pulling force within the pi bond across the double bond which reinforces the regions occupied by alkenyl hydrogens, the alkenyl hydrogens are strongly deshielded.

B_experienced = B₀ - B_isigma + B_ipi

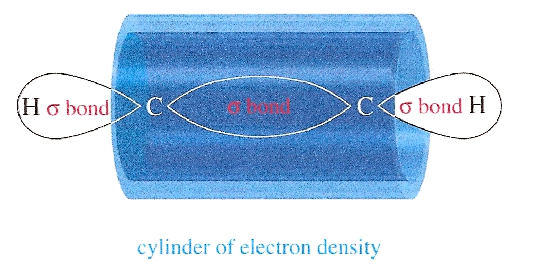
Unlike alkenyl hydrogens, alkynyl hydrogens give rise to shielded hydrogens, or relatively high field chemical shifts for H-NMR when subjected to an external magnetic field (B_experienced = B₀ - B_isigma - B_ipi). The molecules are tumbling all around in solution, but for the alkyne the triple bond has a cylindrical pi cloud around the carbon-carbon triple bond. When the two bonds are subjected to an external magnetic field, these electrons will enter into a cylindrical motion that results in this strong shielding effect with high field chemical shifts. This means that the alkynyl hydrogens will be experiencing less of the external magnetic field. This electron cloud can be seen in the figure below.

Relaxation

Relaxation refers to the phenomenon of nuclei returning to their thermodynamically stable states after being excited to higher energy levels. The energy absorbed when a transition from a lower energy level to a high energy level occurs is released when the opposite happens. This can be a fairly complex process based on different timescales of the relaxation. The two most common types of relaxation are spin lattice relaxation (T₁) and spin spin relaxation (T₂). 

Figure \(\PageIndex{5}\): The process of relaxation

To understand relaxation, the entire sample must be considered. By placing the nuclei in an external magnetic field, the nuclei create a bulk magnetization along the z-axis. The spins of the nuclei are also coherent. The NMR signal may be detected as long as the spins are coherent with one another. The NMR experiment moves the bulk magnetization from the z-axis to the x-y plane, where it is detected.

• Spin-Lattice Relaxation (\(T_1\)): T₁ is the time it takes for the 37% of bulk magnetization to recovery along Z-axis from the x-y plane. The more efficient the relaxation process, the smaller relaxation time (T₁) value you will get. In solids, since motions between molecules are limited, the relaxation time (T₁) values are large. Spin-lattice relaxation measurements are usually carried out by pulse methods.
• Spin-Spin Relaxation (\(T_2\)): T₂ is the time it takes for the spins to lose coherence with one another. T₂ can either be shorter or equal to T₁.

In the next sections, the topics to be discussed will build up to actually analyzing NMR spectra. 

Exercise \(\PageIndex{1}\)

Where is radiofrequency (RF) radiation on the energy scale of the electromagnetic spectrum?

Answer

Radiofrequency (RF) radiation, which includes radio waves and microwaves, is at the low-energy end of the electromagnetic spectrum. It is a type of non-ionizing radiation.

Exercise \(\PageIndex{2}\)

If in a field strength of 4.7 T, ¹H requires 200 MHz of energy to maintain resonance. If atom X requires 150 MHz, calculate the amount of energy required to spin flip atom X’s nucleus. Is this amount greater than the energy required for hydrogen?

Answer

E = hυ

E = (6.62 × 10⁻³⁴)(150 MHz)

E = 9.93 × 10⁻²⁶ J

The energy is equal to 9.93x10^-26 J. This value is smaller than the energy required for hydrogen (1.324 × 10⁻²⁵ J).

Exercise \(\PageIndex{3}\)

Calculate the energy required to spin flip at 400 MHz. Does changing the frequency to 500 MHz decrease or increase the energy required? What about 300 MHz.

Answer

E = hυ

E = (6.62 × 10⁻³⁴)(400 MHz)

E = 2.648 × 10⁻²⁵ J

The energy would increase if the frequency would increase to 500 MHz, and decrease if the frequency would decrease to 300 MHz.

Exercise \(\PageIndex{4}\)

What “things” in a molecule generate magnetic fields that will influence B_o for a particular hydrogen nucleus?

Answer

The electrons in bonds can generate magnetic fields that will influence B_O.

References

• Atta-ur-Rahman. Nuclear Magnetic Resonance. New York: Springer-Verlag, 1986.
• Freeman, Ray. Magnetic Resonance in Chemistry and Medicine. New York: Oxford University Press, 2003.
• Lambert, Joseph B and Eugene P Mazzola. Nuclear Magnetic Resonance Spectroscopy: An Introduction to Princliples, Applications, and Experimental Methods. Upper Saddle River: Pearson Education, 2004.
• Chang, Raymond. Physical Chemistry for the Biosciences. University Science Books, 2005

Contributors and Attributions

• Derrick Kaseman (UC Davis) and Revathi Srinivasan Ganesh Iyer (UCD)